Introduction

Recent years have witnessed a growing tendency to study nonreciprocal structures and devices in many different areas1,2,3. Inspired by the development of photoelectric diode4, 5, researchers have successively invented the acoustic diode6, 7 and the thermal diode8, 9. According to Lorentz reciprocity theorem10, the light propagation process is usually reversible. Therefore, it is difficult to achieve non-reciprocity in optical field. However, several effective ways have been figured out to overcome this problem in recent years. For example, a metal-silicon waveguide system was proposed in 2011, which enabled light to propagate unidirectionally on silicon chips by adjusting light potential energy11. In nonlinear optics, an all-optical diode made of a defect embedded in lithium niobate channel waveguide was numerically demonstrated with a spatially nonreciprocal response12, 13. External electric field can be employed to further improve the isolating performance for this structure14,15,16, and multiple quasi-phase-matching (QPM) technique was also introduced to realize all-optical isolating action17.

In these researches, numerical computation is the main approach used to study the nonreciprocal process, which may not directly explain why it shows a nonreciprocal response as well as how to get a bigger response. In this paper, we have studied the above 1D defective structure theoretically and obtained a series of analytical solutions not only for the second-harmonic output but also for the nonreciprocal response. Further derivations have been implemented and some new results have been revealed from the solutions. The role of the non-reciprocity parameter P as well as its corresponding realization conditions has been discussed in detail.

SHG output solutions and simulations for forward and backward processes

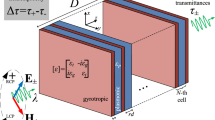
It is well known that the field distributions of the SHG process in homogenous nonlinear crystals can be solved exactly with the help of Jacobi elliptic functions18. In this paper we show that the nonreciprocal SHG process in 1D defective nonlinear photonics crystal (NPC) structures can be solved in a similar manner. The schematic of the defective NPC structure we studied is shown in Fig. 1, where the up and down arrows represent the domain poling directions. Supposing the whole length of the NPC is L, a defect with a width of δL is embedded at the position x = L 1. Theoretically, the whole structure can be treated as three sections, where the first and third sections have the same period (denoted as Λ) but different initial conditions and the second section is the embedded defect inducing phase shift between the fundamental wave (FW) and second-harmonic wave (SHW). The nonreciprocal responses of the structure can be revealed by studying the forward and backward SHG processes separately, which can be treated analytically with the nonlinear coupled equations.

Figure 1
figure 1

A schematic of the forward and backward SHG processes in a 1D nonlinear photonic crystal with a defect embedded.

Full size image

Firstly, we focus on the forward SHG process. The nonlinear coupled equations for this process are usually expressed as19:

$$\{\begin{array}{rcl}\frac{d{A}_{1}}{dx} & = & -iKf(x){A}_{2}{A}_{1}^{\ast }\exp (-i{\rm{\Delta }}kx)\\ \frac{d{A}_{2}}{dx} & = & -\frac{1}{2}iKf(x){A}_{1}^{2}\exp (i{\rm{\Delta }}kx)\end{array}$$
(1)

where A 1, A 2 refer to the field distributions of FW and SHW, respectively. K is the nonlinear coupling coefficient and f(x) represents the structure function of the NPC. Δk = k 2 − 2k 1 describes the phase mismatching in the SHG process, where k 1 and k 2 are respectively the wave vectors of FW and SHW.

For the first section of the NPC (0 ~ L 1), when the QPM condition is satisfied20,21,22, Eq. (1) can be simplified as:

$$\{\begin{array}{rcl}\frac{d{A}_{1}}{dx} & = & -i{K}_{1}{A}_{2}{A}_{1}^{\ast }\\ \frac{d{A}_{2}}{dx} & = & -\frac{1}{2}i{K}_{1}{A}_{1}^{2}\end{array}$$
(2)

where K 1 = K · g m , and g m is the Fourier coefficient of the reciprocal vector used in the QPM process. Eq. (2) can be solved easily with the initial conditions of A 1(0) = A 0, A 2(0) = 0, and the following solutions for A 1 and A 2 at the position x = L 1 can be obtained17:

$$\{\begin{array}{rcl}{A}_{1}({L}_{1}) & = & {A}_{0}\,{\rm sech}(\frac{\sqrt{2}}{2}{A}_{0}{K}_{1}{L}_{1})\\ {A}_{2}({L}_{1}) & = & -\frac{\sqrt{2}}{2}i{A}_{0}\,\tanh (\frac{\sqrt{2}}{2}{A}_{0}{K}_{1}{L}_{1})\end{array}$$
(3)

For the second section (L 1 ~ L 1 + δL), a phase shift (denoted as Δφ) is induced between the FW and SHW, which can be written as Δφ = (2δL/Λ − 1)π.

For the third section (L 1 + δL ~ L), the simplified coupled equations under the QPM condition are similar with Eq. (2) except additional terms exp(iΔφ) and exp(−iΔφ) resulting from the second section, which can be expressed as:

$$\{\begin{array}{rcl}\frac{d{A}_{1}}{dx} & = & -i{K}_{1}{A}_{2}{A}_{1}^{\ast }\exp (i{\rm{\Delta }}\phi )\\ \frac{d{A}_{2}}{dx} & = & -\frac{1}{2}i{K}_{1}{A}_{1}^{2}\exp (-i{\rm{\Delta }}\phi )\end{array}$$
(4)

In this situation, the initial conditions can be determined by Eq. (3), where not only the FW but also the SHW has a non-zero initial value.

If we concentrate on the variation of amplitudes and phases respectively, Eq. (4) can be further reduced to the following ones:

$$\{\begin{array}{rcl}\frac{d{y}_{1}}{dx} & = & -{K}_{1}{y}_{1}{y}_{2}\,\sin \,\theta \\ \frac{d{y}_{2}}{dx} & = & \frac{1}{2}{K}_{1}{y}_{1}^{2}\,\sin \,\theta \end{array}$$
(5a)
$$\{\begin{array}{rcl}\frac{d{\phi }_{1}}{dx} & = & -{K}_{1}{y}_{2}\,\cos \,\theta \\ \frac{d{\phi }_{2}}{dx} & = & -\frac{1}{2}{K}_{1}\frac{{y}_{1}^{2}}{{y}_{2}}\,\cos \,\theta \end{array}$$
(5b)

where y 1, y 2 represent the amplitudes of the FW and SHW respectively while φ 1, φ 2 represent the corresponding phases and θ = 2φ 1 − φ 2 − Δφ.

From the above equations and QPM conditions, two first integrals can be drawn. They are

$$\{\begin{array}{rcl}\cos \,\theta \cdot {y}_{1}^{2}{y}_{2} & = & {\rm{\Gamma }}\\ {|{y}_{{\rm{1}}}|}^{{\rm{2}}}+2{|{y}_{{\rm{2}}}|}^{{\rm{2}}} & = & {A}_{0}^{2}\end{array}$$
(6)

where Γ is a constant and its value can be obtained by substituting Eq. (3) to the above equation:

$${\rm{\Gamma }}=\frac{\sqrt{2}}{2}{A}_{0}^{3}\,\sin \,{\rm{\Delta }}\phi {\rm sech}^{2}(\frac{\sqrt{2}}{2}{A}_{0}{K}_{1}{L}_{1})\tanh (\frac{\sqrt{2}}{2}{A}_{0}{K}_{1}{L}_{1})$$
(7)

On the basis of Eqs (6) and (5a) can be further solved as:

$${\int }_{{y}_{2}({L}_{1})}^{{y}_{2}(L)}\frac{d({y}_{2}^{2})}{\sqrt{{({A}_{0}^{2}-2{y}_{2}^{2})}^{2}{y}_{2}^{2}-{{\rm{\Gamma }}}^{2}}}=\pm {K}_{1}(L-{L}_{1})$$
(8)

Supposing that the term under the square root sign can be written as \({({A}_{0}^{2}-2{y}_{2}^{2})}^{2}{y}_{2}^{2}-{{\rm{\Gamma }}}^{2}=4({y}_{2}^{2}-A)\) \(({y}_{2}^{2}-B)({y}_{2}^{2}-C)\), the parameters A, B, C can be expressed as23, 24:

$$\{\begin{array}{rcl}A & = & \frac{{A}_{0}^{2}}{3}+\frac{{A}_{0}^{2}}{3}\,\cos (\zeta +\frac{2\pi }{3})\\ B & = & \frac{{A}_{0}^{2}}{3}+\frac{{A}_{0}^{2}}{3}\,\cos (\zeta +\frac{4\pi }{3})\\ C & = & \frac{{A}_{0}^{2}}{3}+\frac{{A}_{0}^{2}}{3}\,\cos \,\zeta \end{array}$$
(9)

where \(\zeta =\arccos (-1+27{{\rm{\Gamma }}}^{2}/{A}_{0}^{6})/3\) and A ≤ B ≤ C. Here A, B, C are all real numbers since \(0\le 27{{\rm{\Gamma }}}^{2}/{A}_{0}^{6}\le 2\) is always satisfied and thus obviously ζ ∈ [0, π/3]. Eq. (8) can be further solved as:

$${{y}_{2}}^{2}(L)={a}^{2}{{\rm{sn}}}^{2}[b{K}_{1}(L-{L}_{1})\pm F(\gamma ,\varphi ),\gamma ]+A$$
(10)

where \(F(\gamma ,\varphi )=F(a/b,\arcsin (\sqrt{{y}_{2}^{2}({L}_{1})-A}/a))\) is the Legendre’s incomplete elliptic integral of the first kind, and a, b are two real constants which are defined by \(a=\sqrt{B-A}\) and \(b=\sqrt{C-A}\). sn(z,γ) represents Jacobi elliptic function with modulus γ = a/b. In Eq. (10), we should choose a “+” sign if y 2 is to increase with increasing x and a “−” sign if y 2 is to decrease with increasing x.

Secondly, for the backward transmission, the results are similar to the forward ones, thus the amplitude for SHW can be expressed as:

$${{y}_{2}}^{2}(0)={a}^{^{\prime} 2}{{\rm{sn}}}^{2}[b^{\prime} {K}_{1}{L}_{1}\pm F(\gamma ^{\prime} ,\varphi ^{\prime} ),\gamma ^{\prime} ]+A^{\prime} $$
(11)

where \(F(\gamma ^{\prime} ,\varphi ^{\prime} )=F(a^{\prime} /b^{\prime} ,\arcsin (\sqrt{{y}_{2}^{2}(L-{L}_{1})-A^{\prime} }/a^{\prime} ))\). Parameters a′, b′, A′ can be obtained by repeating the above deduction processes with the defect position L − L 1 instead of L 1.

Numerical calculations have been conducted together with the above analytical solutions to demonstrate the nonreciprocal response of the defective NPC structure. Assuming that the defect position is L 1 = 0.2L and δL = 5Λ/6, that is the phase shift Δφ equals to 2π/3, the normalized amplitudes of FW and SHW in the NPC are shown in Fig. 2. Figure 2(a) and (b) show the forward and backward transmission processes calculated on the basis of the original coupled equations Eq. (1). Figure 2(c) and (d) are corresponding processes obtained by numerical calculation of the simplified equations Eqs (2) and (4). Whereas Fig. 2(e) and (f) show the corresponding results of the analytical solutions Eqs (10) and (11). We can see that they match well with each other.

Figure 2
figure 2

Normalized amplitudes of FW and SHW for the forward and backward processes in the defective NPC. (a–f) are the corresponding forward and backward propagations calculated on the basis of the original coupled equations, the simplified coupled equations and the analytical solutions, respectively.

Full size image

Analytical and numerical derivations of the non-reciprocity parameter

According to [12–15], the non-reciprocity parameter P in this SHG process can be defined as:

$$P=\frac{|{y}_{2}^{-}|-|{y}_{2}^{+}|}{|{y}_{2}^{-}|+|{y}_{2}^{+}|}$$
(12)

Here, superscripts “+” and “−” represent the forward and backward processes, respectively. In the situation that L − L 1 ≫ L 1, Eq. (12) can be simplified as:

$$P=\frac{1-{\eta }^{+}}{1+{\eta }^{+}}$$
(13)

where \({({\eta }^{+})}^{2}=2{|{y}_{2}^{+}|}^{2}/{A}_{0}^{2}\) describes the SHG conversion efficiency of the forward process.

Substituting Eq. (10) into Eq. (13), it is found that when the forward SHG output reaches its minimum, the non-reciprocity achieves the maximum, and vice versa. According to the properties of Jacobi elliptic function, when the forward output achieves the minimum or maximum, the following expressions for the total length of the crystal L can be obtained respectively:

$$L=\frac{{\rm{1}}}{b{K}_{1}}[nT(\gamma )\pm F(\gamma ,\arcsin \frac{\sqrt{{y}_{2}^{2}({L}_{1})-A}}{a})+b{K}_{1}{L}_{1}]$$
(14)

where T(γ) = F(γ, π/2) describes the period of the first kind of complete elliptic integral and n represents positive integers. When n is an even number, the forward SHG output reaches the minimum value \(\sqrt{A}\) and otherwise achieves the maximum value \(\sqrt{B}\). The corresponding extremes of P can be solved as

$$\{\begin{array}{rcl}{P}_{\min } & = & \frac{\sqrt{3}-2\,\sin (\frac{\zeta }{2}+\frac{\pi }{6})}{\sqrt{3}+2\,\sin (\frac{\zeta }{2}+\frac{\pi }{6})}\\ {P}_{\max } & = & \frac{\sqrt{3}-2\,\sin (\frac{\pi }{6}-\frac{\zeta }{2})}{\sqrt{3}+2\,\sin (\frac{\pi }{6}-\frac{\zeta }{2})}\end{array}$$
(15)

It can be analytically solved from Eq. (15) that P max equals to P min when the condition \(27{{\rm{\Gamma }}}^{2}/{A}_{0}^{6}=2\) is satisfied. That means with specific values of A 0 K 1 L 1 and Δφ, no matter what the length of the total crystal, the value of P keeps the same.

Surface plot of the extremes of is calculated and shown in Fig. 3. It can be seen that high nonreciprocal responses can be obtained when Δφ is close to 0 or π, which corresponds to the situation that Γ is small. Furthermore, if Γ → 0, ideal non-reciprocity can be obtained. The expression of P max can be approximately simplified as follows:

$${P}_{\max }=1-\frac{2\sqrt{2}{\rm{\Gamma }}}{{A}_{0}^{3}}$$
(16)
Figure 3
figure 3

Surface plot of the extremes of the non-reciprocity parameter with different structure parameters.

Full size image

In this situation, γ can be expressed as \(\gamma =1-3\sqrt{2}{\rm{\Gamma }}/{A}_{0}^{3}\). Substituting Eq. (16) into Eq. (14), it can be concluded that the total NPC length needed increases logarithmically with P max since the corresponding period T(γ) can be approximately expressed as

$$T(\gamma )=\frac{1}{2}\,\mathrm{ln}\,\frac{16}{1-\gamma }$$
(17)

Figure 4 shows the dependence of P max and Δφ, where Δφ varies in the range of 0 and π, and A 0 K 1 L 1 is set to be 0.3π. Figure 4(b) is the partial magnification of Fig. 4(a). It can be seen that the non-reciprocity parameter P is periodically distributed for a given Δφ, which is resulted from the periodicity of the Jacobi elliptic function. Besides, when Δφ → 0 or Δφ → π, the corresponding period will increase logarithmically, which matches well with the analytical discussions.

Figure 4
figure 4

Distribution of non-reciprocity parameter with different values of Δφ under the condition that A 0 K 1 L 1 = 0.3π. Different color represents different nonreciprocal responses.

Full size image

Simulation results show that the contrast ratio of the all-optical diode can maintain close to 1. Thus, a near complete nonreciprocal all-optical diode based on a NPC can be realized. As is shown in the Fig. 4(b), the same color describes the same nonreciprocal response. It can be seen that for any target non-reciprocity, a NPC structure with a defect embedded can be designed with specific values of Δφ and A 0 K 1 L 1, which are realized by adjusting the defect width, the crystal length and the FW input. As Δφ moves away from zero, the same nonreciprocal response is kept as long as the total length of the crystal decreases. It means that the target non-reciprocity could be achieved with a shorter crystal length and a lower FW input by adjusting the defect width. For practical applications in a defective NPC structured on the lithium niobate (LN), the nonlinear coefficient d 33 = 27 pm/V. If a 70% nonreciprocal contrast is required, that is P max = 0.7, A 0 K 1 L ≈ 6.191 and Δφ ≈ 0.532 can be carried out according to Eqs (7) and (14), sketched by point ‘A’ in Fig. 4(b). The data show that if we set the FW (1064 nm) intensity to be 60 MW/cm 2, the needed total length is 1.3 cm and the required defect width is 3.8 μm with the QPM period being 6.6 μm. Similarly, if an 80% nonreciprocal contrast is required, the corresponding parameters can be obtained by the same method, shown by point ‘B’ in the figure. Discussions above have explained a universal design of nonlinear crystals. In comparison with previously reported NPC diodes, our results reveal that the design of diodes can be more flexible and easier to control.

Conclusion

In all, we have theoretically studied the non-reciprocity properties of SHG process realized by a defective 1D NPC. Exact solutions for the SHG output in both forward and backward transmission processes have been derived with elliptic function forms. According to the property of the elliptic function, it can be concluded that for specific values of Δφ and A 0 K 1 L 1, the non-reciprocity parameter has a maximum value as well as a minimum value. It is found that the complete non-reciprocity cannot be realized in this structure but can be approached infinitely. In this case, the crystal length needed increases with the nonreciprocal parameter at a logarithmic rate. Any target non-reciprocity can be obtained by adjusting the defect width, the crystal length and the FW input. These results may lead to a variety of relevant applications, including all-optical diode, optical isolator, amplifiers and so on.

Methods

It is obvious that there is no non-reciprocity when the defect is at the middle position, which means L 1 = L/2. Asymmetric structures should be adopted for significant responses. Hereafter we present a detailed analysis under the condition that L − L 1 ≫ L 1. In this situation, it is found that the SHG output in backward transmission process will tend to \(\sqrt{2}{A}_{0}/2\) as long as the intensity of the input FW is sufficiently high. Thus Eq. (12) can be simplified as:

$$P=\frac{\frac{\sqrt{2}}{2}{A}_{0}-|{y}_{2}^{+}|}{\frac{\sqrt{2}}{2}{A}_{0}+|{y}_{2}^{+}|}$$
(18)

Ordering \({({\eta }^{+})}^{2}=2{|{y}_{2}^{+}|}^{2}/{A}_{0}^{2}\) to describe the SHG conversion efficiency of the forward process, Eq. (18) can be written as \(P=\frac{1-{\eta }^{+}}{1+{\eta }^{+}}\). As a result, complete reciprocity and non-reciprocity are corresponding to P = 0 and P = 1, respectively.

Figures for the discussion of the non-reciprocity parameter are all calculated by MATLAB on the basis of the analytical solutions. Figure 3 is obtained with Eq. (15) under the approximate condition that L − L 1 ≫ L 1 while Fig. 4 is obtained by accurately substituting the Eqs (10) and (11) into Eq. (12). The digital trends for the corresponding points match well between the two figures, which demonstrated that the approximation above is reasonable.