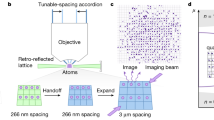
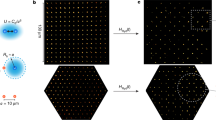
Abstract
Spontaneous symmetry breaking underlies much of our classification of phases of matter and their associated transitions1,2,3. The nature of the underlying symmetry being broken determines many of the qualitative properties of the phase; this is illustrated by the case of discrete versus continuous symmetry breaking. Indeed, in contrast to the discrete case, the breaking of a continuous symmetry leads to the emergence of gapless Goldstone modes controlling, for instance, the thermodynamic stability of the ordered phase4,5. Here, we realize a two-dimensional dipolar XY model that shows a continuous spin-rotational symmetry using a programmable Rydberg quantum simulator. We demonstrate the adiabatic preparation of correlated low-temperature states of both the XY ferromagnet and the XY antiferromagnet. In the ferromagnetic case, we characterize the presence of a long-range XY order, a feature prohibited in the absence of long-range dipolar interaction. Our exploration of the many-body physics of XY interactions complements recent works using the Rydberg-blockade mechanism to realize Ising-type interactions showing discrete spin rotation symmetry6,7,8,9.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
Data availability
The data are available from the corresponding author on reasonable request.
References
Landau, L. D. On the theory of phase transitions. I. Zh. Eksp. Teor. Fiz. 11, 19 (1937).
Landau, L. D. & Ginzburg, V. L. On the theory of superconductivity. Zh. Eksp. Teor. Fiz. 20, 1064 (1950).
Kepler, J. De Nive Sexangula (Gottfried Tampach, 1611).
Goldstone, J. Field theories with ‘Superconductor’ solutions. Il Nuovo Cimento 19, 154–164 (1961).
Tasaki, H. Physics and Mathematics of Quantum Many-Body Systems (Springer International Publishing, 2020).
Schauß, P. et al. Crystallization in Ising quantum magnets. Science 347, 1455–1458 (2015).
Guardado-Sanchez, E. et al. Probing the quench dynamics of antiferromagnetic correlations in a 2D quantum Ising spin system. Phys. Rev. X 8, 021069 (2018).
Scholl, P. et al. Quantum simulation of 2D antiferromagnets with hundreds of Rydberg atoms. Nature 595, 233–238 (2021).
Ebadi, S. et al. Quantum phases of matter on a 256-atom programmable quantum simulator. Nature 595, 227–232 (2021).
Bloch, F. Zur Theorie des Ferromagnetismus. Zeitschrift Phys. 61, 206–219 (1930).
Peierls, R. Quelques propriétés typiques des corps solides. Annales de l’institut Henri Poincaré 5, 177–122 (1935).
Mermin, N. D. & Wagner, H. Absence of ferromagnetism or antiferromagnetism in one- or two-dimensional isotropic Heisenberg models. Phys. Rev. Lett. 17, 1133–1136 (1966).
Hohenberg, P. C. Existence of long-range order in one and two dimensions. Phys. Rev. 158, 383–386 (1967).
Bruno, P. Absence of spontaneous magnetic order at nonzero temperature in one- and two-dimensional Heisenberg and XY systems with long-range interactions. Phys. Rev. Lett. 87, 137203 (2001).
Defenu, N. et al. Long-range interacting quantum systems. Preprint at https://arxiv.org/abs/2109.01063 (2021).
Dyson, F. J. Existence of a phase-transition in a one-dimensional Ising ferromagnet. Commun. Math. Phys. 12, 91–107 (1969).
Kunz, H. & Pfister, C. E. First order phase transition in the plane rotator ferromagnetic model in two dimensions. Commun. Math. Phys. 46, 245–251 (1976).
Maleev, S. V. Dipole forces in two-dimensional and layered ferromagnets. Soviet J. Exp. Theor. Phys. 43, 1240 (1976).
Fröhlich, J., Israel, R., Lieb, E. H. & Simon, B. Phase transitions and reflection positivity. I. General theory and long range lattice models. Commun. Math. Phys. 62, 1–34 (1978).
Diep, H. T. (ed.) Frustrated Spin Systems 2nd edn (World Scientific, 2013).
Castelnovo, C., Moessner, R. & Sondhi, S. L. Magnetic monopoles in spin ice. Nature 451, 42–45 (2008).
Yao, N. Y., Zaletel, M. P., Stamper-Kurn, D. M. & Vishwanath, A. A quantum dipolar spin liquid. Nat. Phys. 14, 405–410 (2018).
Keleş, A. & Zhao, E. Absence of long-range order in a triangular spin system with dipolar interactions. Phys. Rev. Lett. 120, 187202 (2018).
Keleş, A. & Zhao, E. Renormalization group analysis of dipolar Heisenberg model on square lattice. Phys. Rev. B 97, 245105 (2018).
De’Bell, K., MacIsaac, A. B. & Whitehead, J. P. Dipolar effects in magnetic thin films and quasi-two-dimensional systems. Rev. Mod. Phys. 72, 225–257 (2000).
Taroni, A., Bramwell, S. T. & Holdsworth, P. C. W. Universal window for two-dimensional critical exponents. J. Phys. Condens. Matter 20, 275233 (2008).
Peter, D., Müller, S., Wessel, S. & Büchler, H. P. Anomalous behavior of spin systems with dipolar interactions. Phys. Rev. Lett. 109, 025303 (2012).
Mazurenko, A. et al. A cold-atom Fermi-Hubbard antiferromagnet. Nature 545, 462–466 (2017).
Yan, B. et al. Observation of dipolar spin-exchange interactions with lattice-confined polar molecules. Nature 501, 521–525 (2013).
Christakis, L. et al. Probing site-resolved correlations in a spin system of ultracold molecules. Nature 614, 64–69 (2023).
Chomaz, L. et al. Dipolar physics: a review of experiments with magnetic quantum gases. Rep. Prog. Phys. 86, 026401 (2022).
Leo, N. et al. Collective magnetism in an artificial 2D XY spin system. Nat. Commun. 9, 2850 (2018).
Richerme, P. et al. Non-local propagation of correlations in quantum systems with long-range interactions. Nature 511, 198–201 (2014).
Jurcevic, P. et al. Quasiparticle engineering and entanglement propagation in a quantum many-body system. Nature 511, 202–205 (2014).
Maghrebi, M. F., Gong, Z.-X. & Gorshkov, A. V. Continuous symmetry breaking in 1D long-range interacting quantum systems. Phys. Rev. Lett. 119, 023001 (2017).
Feng, L. et al. Continuous symmetry breaking in a trapped-ion spin chain. Preprint at https://arxiv.org/abs/2211.01275 (2022).
Yang, C. N. Concept of off-diagonal long-range order and the quantum phases of liquid He and of superconductors. Rev. Mod. Phys. 34, 694–704 (1962).
Berezinskiǐ, V. L. Destruction of long-range order in one-dimensional and two-dimensional systems having a continuous symmetry group I. Classical systems. Soviet J. Exp. Theor. Phys. 32, 493 (1971).
Berezinskiǐ, V. L. Destruction of long-range order in one-dimensional and two-dimensional systems possessing a continuous symmetry group. II. Quantum systems. Soviet J. Exp. Theor. Phys. 34, 610 (1972).
Kosterlitz, J. M. & Thouless, D. J. Ordering, metastability and phase transitions in two-dimensional systems. J. Phys. C. Solid State Phys. 6, 1181–1203 (1973).
Kosterlitz, J. M. The critical properties of the two-dimensional XY model. J. Phys. C Solid State Phys. 7, 1046–1060 (1974).
Giachetti, G., Defenu, N., Ruffo, S. & Trombettoni, A. Berezinskii-Kosterlitz-Thouless phase transitions with long-range couplings. Phys. Rev. Lett. 127, 156801 (2021).
Browaeys, A. & Lahaye, T. Many-body physics with individually controlled Rydberg atoms. Nat. Phys. 16, 132 (2020).
Sørensen, A. S. et al. Adiabatic preparation of many-body states in optical lattices. Phys. Rev. A. 81, 061603 (2010).
Sandvik, A. W. & Hamer, C. J. Ground-state parameters, finite-size scaling, and low-temperature properties of the two-dimensional S = 1/2 XY model. Phys. Rev. B 60, 6588–6593 (1999).
White, S. R. Density matrix formulation for quantum renormalization groups. Phys. Rev. Lett. 69, 2863–2866 (1992).
Hauschild, J. & Pollmann, F. Efficient numerical simulations with tensor networks: Tensor Network Python (TeNPy). SciPost Physics Lecture Notes 5 (2018).
Anderson, P. W. An approximate quantum theory of the antiferromagnetic ground state. Phys. Rev. 86, 694–701 (1952).
Anderson, P. W. Basic Notions of Condensed Matter Physics (Perseus Publishing, 2010).
Tasaki, H. Long-range order, ‘tower’ of states, and symmetry breaking in lattice quantum systems. J. Stat. Phys. 174, 735–761 (2019).
Beekman, A., Rademaker, L. & van Wezel, J. An introduction to spontaneous symmetry breaking. SciPost Physics Lecture Notes 11 (2019).
Comparin, T., Mezzacapo, F. & Roscilde, T. Robust spin squeezing from the tower of states of U(1)-symmetric spin Hamiltonians. Phys. Rev. A 105, 022625 (2022).
Barredo, D., de Léséleuc, S., Lienhard, V., Lahaye, T. & Browaeys, A. An atom-by-atom assembler of defect-free arbitrary 2D atomic arrays. Science 354, 1021–1023 (2016).
de Léséleuc, S., Barredo, D., Lienhard, V., Browaeys, A. & Lahaye, T. Analysis of imperfections in the coherent optical excitation of single atoms to Rydberg states. Phys. Rev. A 97, 053803 (2018).
Kennedy, T., Lieb, E. H. & Shastry, B. S. in Statistical Mechanics (eds Nachtergaele, B. et al.) 327–329 (Springer, 1988).
Björnberg, J. E. & Ueltschi, D. Reflection positivity and infrared bounds for quantum spin systems. In The Physics and Mathematics of Elliott Lieb 77–108 (EMS Press, 2022).
Stoudenmire, E. & White, S. R. Studying two-dimensional systems with the density matrix renormalization group. Ann. Rev. Condens. Matter Phys. 3, 111–128 (2012).
Hastings, M. B. & Koma, T. Spectral gap and exponential decay of correlations. Commun. Math. Phys. 265, 781–804 (2006).
Lienhard, V. et al. Observing the space- and time-dependent growth of correlations in dynamically tuned synthetic Ising antiferromagnets. Phys. Rev. X 8, 021070 (2018).
de Léséleuc, S. et al. Observation of a symmetry-protected topological phase of interacting bosons with Rydberg atoms. Science 365, 775–780 (2019).
Jensen, P. J., Bennemann, K. H., Morr, D. K. & Dreyssé, H. Two-dimensional Heisenberg antiferromagnet in a transverse field. Phys. Rev. B 73, 144405 (2006).
Kar, S., Wierschem, K. & Sengupta, P. Magnons in a two-dimensional transverse-field XXZ model. Phys. Rev. B 96, 045126 (2017).
Gu, S.-J. Fidelity approach to quantum phase transitions. Int. J. Mod. Phys. B 24, 4371–4458 (2010).
Zaletel, M. P., Mong, R. S. K., Karrasch, C., Moore, J. E. & Pollmann, F. Time-evolving a matrix product state with long-ranged interactions. Phys. Rev. B 91, 165112 (2015).
Mermin, N. D. Crystalline order in two dimensions. Phys. Rev. 176, 250–254 (1968).
Fröhlich, J. & Pfister, C. On the absence of spontaneous symmetry breaking and of crystalline ordering in two-dimensional systems. Commun. Math. Phys. 81, 277–298 (1981).
Tobochnik, J. & Chester, G. V. Monte Carlo study of the planar spin model. Phys. Rev. B 20, 3761–3769 (1979).
Ueda, A. & Oshikawa, M. Resolving the Berezinskii-Kosterlitz-Thouless transition in the two-dimensional XY model with tensor-network-based level spectroscopy. Phys. Rev. B 104, 165132 (2021).
Ding, H.-Q. & Makivić, M. S. Kosterlitz-Thouless transition in the two-dimensional quantum XY model. Phys. Rev. B 42, 6827–6830 (1990).
Ding, H.-Q. Phase transition and thermodynamics of quantum XY model in two dimensions. Phys. Rev. B 45, 230–242 (1992).
Romano, S. Computer simulation study of a long-range plane-rotator system in two dimensions. Nuovo Cim, B 100, 447–466 (1987).
Romano, S. Computer-simulation study of a disordered plane-rotator system in two dimensions with long-range ferromagnetic interactions. Phys. Rev. B 42, 8647–8650 (1990).
Fisher, M. E., Ma, S.-k & Nickel, B. G. Critical exponents for long-range interactions. Phys. Rev. Lett. 29, 917–920 (1972).
Sak, J. Recursion relations and fixed points for ferromagnets with long-range interactions. Phys. Rev. B 8, 281–285 (1973).
Stoudenmire, E. M. & White, S. R. Minimally entangled typical thermal state algorithms. New J. Phys. 12, 055026 (2010).
Binder, M. & Barthel, T. Symmetric minimally entangled typical thermal states for canonical and grand-canonical ensembles. Phys. Rev. B 95, 195148 (2017).
Gubernatis, J., Kawashima, N. & Werner, P. Quantum Monte Carlo Methods: Algorithms for Lattice Models 1st edn (Cambridge Univ. Press, 2016).
Syljuasen, O. F. & Sandvik, A. W. Quantum Monte Carlo with directed loops. Phys. Rev. E 66, 046701 (2002).
Calabrese, P. & Cardy, J. Time dependence of correlation functions following a quantum quench. Phys. Rev. Lett. 96, 136801 (2006).
Acknowledgements
We acknowledge the insights of and discussions with M. Aidelsburger, L. Henriet, V. Lienhard, J. Moore, C. Laumann, B. Halperin, E. Altman, B. Ye, E. Davis and M. Block. We are especially indebted to H.P. Büchler for insightful comments and discussions about the role of dipolar interactions in the XY model. The computational results presented were performed in part using the FASRC Cannon cluster supported by the FAS Division of Science Research Computing Group at Harvard University, the Savio computational cluster resource provided by the Berkeley Research Computing programme at the University of California, Berkeley and the Vienna Scientific Cluster. This work is supported by the European Union’s Horizon 2020 research and innovation programme under grant agreement no. 817482 (PASQuanS), the Agence Nationale de la Recherche (ANR, project nos. RYBOTIN and ANR-22-PETQ-0004, project QuBitAF) and the European Research Council (advanced grant no. 101018511-ATARAXIA). J.H. acknowledges support from the NSF OIA Convergence Accelerator programme under award number 2040549, and the Munich Quantum Valley, which is supported by the Bavarian state government with funds from the Hightech Agenda Bayern Plus. M.S. and A.M.L. acknowledge support by the Austrian Science Fund (FWF) through grant no. I 4548. D.B. acknowledges support from grant no. MCIN/AEI/10.13039/501100011033 (grant nos. RYC2018- 025348-I, PID2020-119667GA-I00 and European Union NextGenerationEU PRTR-C17.I1). M.P.Z. acknowledges support from the Department of the Environment (DOE) Early Career programme and the Alfred P. Sloan foundation. N.Y.Y. acknowledges support from the Army Research Office (ARO) (grant no. W911NF-21-1-0262), the AFOSR MURI programme (grant no. W911NF-20-1-0136), the David and Lucile Packard foundation, and the Alfred P. Sloan foundation. M.B. and V.L. acknowledge support from NSF QLCI programme (grant no. OMA-2016245). S.C. acknowledges support from the ARO through the MURI programme (grant no. W911NF-17-1-0323) and from the US DOE, Office of Science, Office of Advanced Scientific Computing Research, under the Accelerated Research in Quantum Computing programme.
Author information
Authors and Affiliations
Contributions
C.C., G.B., M.B. and G.E. contributed equally to this work. C.C., G.B., G.E., P.S. and D.B. carried out the experiments. M.B., L.L., V.S.L., J.H., S.C. and M.S. conducted the theoretical analysis and simulations. A.M.L., M.P.Z., T.L., N.Y.Y. and A.B. supervised the work. All authors contributed to the data analysis, progression of the project and on both the experimental and theoretical side. All authors contributed to the writing of the manuscript.
Corresponding author
Ethics declarations
Competing interests
A.B. and T.L. are cofounders and shareholders of PASQAL. The remaining authors declare no competing interests.
Peer review
Peer review information
Nature thanks the anonymous reviewers for their contribution to the peer review of this work.
Additional information
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Extended data figures and tables
Extended Data Fig. 1 Experimental procedures and sequence.
a, Fluorescence image of the atoms in a fully assembled 6 × 7 array. b, Scheme for the preparation of the initial staggered state. c, Detected staggered state, corresponding to the situation for which all the atoms in sublattice A are in \(\left|\uparrow \right\rangle \), and all the atoms in sublattice B are in \(\left|\downarrow \right\rangle \). d, Schematics of the atomic level diagram. e, Experimental sequence.
Extended Data Fig. 2 Simplified error tree associated to the preparation of the initial Néel state, for a the atoms in sublattice A (non-addressed), and b in sublattice B (addressed).
For simplicity, the events with a probability of order 2 or higher in the ηi, ϵ, \({{\epsilon }}^{{\prime} }\) are disregarded.
Extended Data Fig. 3 Time dependence of the correlations along x in the FM case for a 10 × 10 lattice.
a, Time evolution of the nearest-neighbour correlations along x (different colors correspond to different times). b, Spatial correlations as a function of distance, measured at different times t = {0.0, 0.5, 1.0, 2.0, 8.0} μs indicated by dashed lines in a.
Extended Data Fig. 4 DMRG ground state calculations.
a, Real-space correlation profile ∣Cx(d)∣ on L × L square clusters with open boundary conditions. The ground state of \({H}_{{\rm{XY}}}^{{\rm{FM}}}\) clearly exhibits XY LRO at all system sizes. For \({H}_{{\rm{XY}}}^{{\rm{AFM}}}\) and Hnn, the correlations decrease at long distances, but this decay is reduced as L increases. b, Finite-size scaling of the magnetization \({m}_{{\rm{FM}}/{\rm{AFM}}}^{2}\). All three models are consistent with \({m}_{{\rm{FM}}/{\rm{AFM}}}^{2} > 0\) as L → ∞. c, Dependence of \({m}_{{\rm{FM}}/{\rm{AFM}}}^{2}\) on the interaction distance cutoff \({R}_{\max }\). At each system size, the ground state correlations are well-converged by \({R}_{\max }\approx 4\). d–f, Ground state properties of HXY + HZ as a function of δ. There is a smooth crossover from the XY ordered state at δ = 0 to the staggered paramagnet as δ → ∞. The − dm2/dδ peaks (f) are finite-size incarnations of the quantum phase transition expected in the thermodynamic limit; we use their centers to define the crossover point ħδc/J.
Extended Data Fig. 5 Excitation gap for two adiabatic preparation protocols.
a, Minimal energy gaps of \({H}_{{\rm{XY}}}^{{\rm{AFM(FM)}}}+{H}_{{\rm{Z}}}\) to the lowest excited state in the Mz = 0 sector as a function of ħδ/J. We here only consider gaps among states with momentum \({\bf{k}}=0\) and fully symmetric under the lattice point-group, which reflects the setup in the (ideal) experiment. Blue (red) curves show the results for the AFM (FM) model. Darker colors correspond to larger system sizes. The inset shows a sketch of the expected phase diagram. b, Same as a, but for the protocol with Hamiltonian \({H}_{{\rm{XY}}}^{{\rm{AFM(FM)}}}+{H}_{{\rm{X}}}\). Here we cannot restrict the analysis to a single Mz sector since it is not conserved. c, Cumulatively integrated \(1/{\Delta }_{\min }^{2}\) [starting from the largest value ħδ/J = 24] for the gaps shown in a. The values at ħδ/J = 0 measure how difficult it is to prepare the ground state of \({H}_{{\rm{XY}}}^{{\rm{AFM(FM)}}}\) by sweeping δ. d, Same as c but for the gaps along Ω, as shown in b. The inset shows a sketch of the expected phase diagrams for \({H}_{{\rm{XY}}}^{{\rm{AFM(FM)}}}+{H}_{{\rm{X}}}\).
Extended Data Fig. 6 Numerical simulation of the adiabatic preparation for the 6 × 7 lattice.
We compare the predictions from the t-MPS simulations (disorder ensemble average in dark teal, standard deviation in light teal) to the experimental data (grey), as measured at light-shift δ(t) = δ. We also show the ground-state expectation value from DMRG (purple) a, The staggered polarization Pz of the FM. Theory and experiment agree remarkably well, except for an offset at small δ, due to the light-shift-induced depumping. Inset: ramp δ(t) used for the FM simulation. b, The ferromagnetic magnetization \({m}_{{\rm{FM}}}^{2}(\delta )\). We find excellent agreement between experiment and numerics for δ > 2, including near the phase transition at \({\delta }_{c}^{{\rm{FM}}}=5.5\) (red dashed line). The two diverge somewhat at smaller δ (later times), likely due to decoherence and unmodeled systematic measurement errors. c, d, Corresponding results for the AFM. For Pz, the t-MPS simulation accurately reproduces the experimental data across the whole δ(t) sweep. For δ far above \({\delta }_{c}^{{\rm{AFM}}}\,=\,0.6\) (blue dashed line), there are many-body Rabi oscillations characteristic of the paramagnetic phase. c, Inset: ramp δ(t) used for the AFM simulation. d, Inset: zoom-in of lower left corner. At small δ (late times), the magnetization \({m}_{{\rm{AFM}}}^{2}\) measured in experiment is below that predicted from the simulations.
Extended Data Fig. 7 Energetics of the simulated adiabatic preparation.
a, b, Interaction energy density \({E}_{{\rm{XY}}}/\bar{N}(\delta )\) in the t-MPS simulations of the 6 × 7 lattice. The teal line and envelope are the disorder ensemble average and standard deviation, respectively. Following a single state with minimal initialization errors (pink line), we see that EXY tightly follows the DMRG ground state value (purple), confirming that diabatic errors are negligible. c, d, Energy gaps Δ0 between the ground state and the first excited state in the Sz = 0 sector, obtained from DMRG. For the near-ideal initial state, the final energy density (pink dotted line) falls below the gap in both the FM and AFM case.
Extended Data Fig. 8 Finite-temperature properties of HXY + HZ.
a, Phase diagram of \({H}_{{\rm{XY}}}^{{\rm{FM}}}+{H}_{{\rm{Z}}}\) at finite temperature T and light-shift δ, computed from METTS on a 6 × 7 array in the Mz = 0 sector. We also include T = 0 points calculated from DMRG. The region with large magnetization \({m}_{{\rm{FM}}}^{2}\) at small δ and small T should correspond to the LRO phase in the thermodynamic limit. The colorbar is chosen so that dark red corresponds to the final \({m}_{{\rm{FM}}}^{2}\) calculated in the t-MPS simulation, absent measurement errors. Thin black lines are equal-magnitude contours to guide the eye. b,c, Estimated temperature of a quench experiment with final light-shift δf and quench magnitude δq, taking the pre-quench configuration to be either the DMRG ground state (b) or the t-MPS ramp simulation ensemble (c). The oscillatory behavior seen in c stems from the paramagnetic Rabi oscillations discussed in Sec. D3. d, e Corresponding magnetization \({m}_{{\rm{FM}}}^{2}\) of the system at temperature Teff(δf, δq). f-j Analogous results for the antiferromagnet. The region with finite magnetization \({m}_{{\rm{AFM}}}^{2}\) is expected to become an algebraic-ordered (BKT) phase in the thermodynamic limit.
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Chen, C., Bornet, G., Bintz, M. et al. Continuous symmetry breaking in a two-dimensional Rydberg array. Nature 616, 691–695 (2023). https://doi.org/10.1038/s41586-023-05859-2
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41586-023-05859-2
This article is cited by
-
Experimental observation of spontaneous symmetry breaking in a quantum phase transition
Science China Physics, Mechanics & Astronomy (2024)
-
Continuous symmetry breaking in a trapped-ion spin chain
Nature (2023)
-
Exploring large-scale entanglement in quantum simulation
Nature (2023)
-
Scalable spin squeezing in a dipolar Rydberg atom array
Nature (2023)
-
Dipolar quantum solids emerging in a Hubbard quantum simulator
Nature (2023)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.