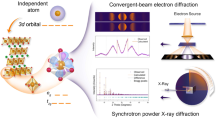
Abstract
The electrification of heavy-duty transport and aviation will require new strategies to increase the energy density of electrode materials1,2. The use of anionic redox represents one possible approach to meeting this ambitious target. However, questions remain regarding the validity of the O2−/O− oxygen redox paradigm, and alternative explanations for the origin of the anionic capacity have been proposed3, because the electronic orbitals associated with redox reactions cannot be measured by standard experiments. Here, using high-energy X-ray Compton measurements together with first-principles modelling, we show how the electronic orbital that lies at the heart of the reversible and stable anionic redox activity can be imaged and visualized, and its character and symmetry determined. We find that differential changes in the Compton profile with lithium-ion concentration are sensitive to the phase of the electronic wave function, and carry signatures of electrostatic and covalent bonding effects4. Our study not only provides a picture of the workings of a lithium-rich battery at the atomic scale, but also suggests pathways to improving existing battery materials and designing new ones.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout



Similar content being viewed by others
Data availability
The experimental data and theoretical simulations that support the findings of this study are available from the corresponding authors upon reasonable request.
References
Sripad, S. & Viswanathan, V. Performance metrics required of next-generation batteries to make a practical electric semi-truck. ACS Energy Lett. 2, 1669–1673 (2017).
Viswanathan, V. & Knapp, B. M. Potential for electric aircraft. Nat. Sustainability 2, 88–89 (2019).
Radin, M. D., Vinckeviciute, J., Seshadri, R. & Van der Ven, A. Manganese oxidation as the origin of the anomalous capacity of Mn-containing Li-excess cathode materials. Nat. Energy 4, 639–646 (2019).
Suzuki, K. et al. Extracting the redox orbitals in Li battery materials with high-resolution x-ray Compton scattering spectroscopy. Phys. Rev. Lett. 114, 087401 (2015).
Krauskopf, T., Richter, F. H., Zeier, W. G. & Janek, J. Physicochemical concepts of the lithium metal anode in solid-state batteries. Chem. Rev. 120, 7745–7794 (2020).
Salah, M. et al. Pure silicon thin-film anodes for lithium-ion batteries: a review. J. Power Sources 414, 48–67 (2019).
Jia, H. et al. Hierarchical porous silicon structures with extraordinary mechanical strength as high-performance lithium-ion battery anodes. Nat. Commun. 11, 1474 (2020).
Yabuuchi, N. et al. High-capacity electrode materials for rechargeable lithium batteries: Li3NbO4-based system with cation-disordered rocksalt structure. Proc. Natl Acad. Sci. USA 112, 7650–7655 (2015).
Seo, D.-H. et al. The structural and chemical origin of the oxygen redox activity in layered and cation-disordered Li-excess cathode materials. Nat. Chem. 8, 692–697 (2016).
Yabuuchi, N. et al. Origin of stabilization and destabilization in solid-state redox reaction of oxide ions for lithium-ion batteries. Nat. Commun. 7, 13814 (2016).
Li, B. & Xia, D. Anionic redox in rechargeable lithium batteries. Adv. Mater. 29, 1701054 (2017).
Okubo, M. & Yamada, A. Molecular orbital principles of oxygen-redox battery electrodes. ACS Appl. Mater. Interfaces 9, 36463–36472 (2017).
Assat, G. & Tarascon, J.-M. Fundamental understanding and practical challenges of anionic redox activity in Li-ion batteries. Nat. Energy 3, 373–386 (2018).
Clément, R., Lun, Z. & Ceder, G. Cation-disordered rocksalt transition metal oxides and oxyfluorides for high energy lithium-ion cathodes. Energy Environ. Sci. 13, 345–373 (2020).
Naylor, A. J. et al. Depth-dependent oxygen redox activity in lithium-rich layered oxide cathodes. J. Mater. Chem. A Mater. Energy Sustain. 7, 25355–25368 (2019).
Li, M. et al. Cationic and anionic redox in lithium-ion based batteries. Chem. Soc. Rev. 49, 1688–1705 (2020).
Xu, J. et al. Elucidating anionic oxygen activity in lithium-rich layered oxides. Nat. Commun. 9, 947 (2018).
Mukai, K., Nonaka, T., Uyama, T. & Nishimura, Y. F. In situ X-ray Raman spectroscopy and magnetic susceptibility study on Li [Li0.15Mn1.85] O4 oxygen anion redox reaction. Chem. Commun. 56, 1701–1704 (2020).
Hong, J. et al. Metal–oxygen decoordination stabilizes anion redox in Li-rich oxides. Nat. Mater. 18, 256–265 (2019).
Tygesen, A. S., Chang, J. H., Vegge, T. & García-Lastra, J. M. Computational framework for a systematic investigation of anionic redox process in Li-rich compounds. npj Comp. Mater. 6, 65 (2020).
Bhowmik, A. et al. A perspective on inverse design of battery interphases using multi-scale modelling, experiments and generative deep learning. Energy Storage Materials 21, 446–456 (2019).
Barbiellini, B. et al. Identifying a descriptor for d-orbital delocalization in cathodes of Li batteries based on x-ray Compton scattering. Appl. Phys. Lett. 109, 073102 (2016).
Suzuki, K. et al. Non-destructive measurement of in-operando lithium concentration in batteries via X-ray Compton scattering. J. Appl. Phys. 119, 025103 (2016).
Suzuki, K. et al. In operando quantitation of Li concentration for a commercial Li-ion rechargeable battery using high-energy X-ray Compton scattering. J. Synchrotron Radiat. 24, 1006–1011 (2017).
Suzuki, K. et al. Dependency of the charge–discharge rate on lithium reaction distributions for a commercial lithium coin cell visualized by Compton scattering imaging. Condensed Matter 3, 27 (2018).
Suzuki, K. et al. High-energy X-ray Compton scattering imaging of 18650-type lithium-ion battery cell. Condensed Matter 4, 66 (2019).
Kaplan, I., Barbiellini, B. & Bansil, A. Compton scattering beyond the impulse approximation. Phys. Rev. B 68, 235104 (2003).
Hafiz, H. et al. Visualizing redox orbitals and their potentials in advanced lithium-ion battery materials using high-resolution X-ray Compton scattering. Sci. Adv. 3, e1700971 (2017).
Hafiz, H. et al. Identification of ferrimagnetic orbitals preventing spinel degradation by charge ordering in LixMn2O4. Phys. Rev. B 100, 205104 (2019).
Sakurai, Y. et al. Imaging doped holes in a cuprate superconductor with high-resolution Compton scattering. Science 332, 698–702 (2011).
Schwarz, W. E. Measuring orbitals: provocation or reality? Angew. Chem. Int. Edn 45, 1508–1517 (2006).
Cooper, M. et al. X-ray Compton Scattering (Oxford Univ. Press, 2004).
Isaacs, E. et al. Covalency of the hydrogen bond in ice: a direct x-ray measurement. Phys. Rev. Lett. 82, 600 (1999).
Eijt, S. W. H. et al. Electronic coupling of colloidal CdSe nanocrystals monitored by thin-film positron-electron momentum density methods. Appl. Phys. Lett. 94, 091908 (2009).
Hoffmann, L., Shukla, A., Peter, M., Barbiellini, B. & Manuel, A. Linear and non-linear approaches to solve the inverse problem: applications to positron annihilation experiments. Nucl. Instrum. Methods Phys. Res. A 335, 276–287 (1993).
Hiraoka, N. et al. A new x-ray spectrometer for high-resolution Compton profile measurements at Spring-8. J. Synchrotron Radiat. 8, 26–32 (2001).
Itou, M. et al. Present status of the Cauchois-type Compton scattering spectrometer at Spring8. Nucl. Instrum. Methods Phys. Res. A 467–468, 1109–1112 (2001).
Sakurai, Y. & Itou, M. A Cauchois-type X-ray spectrometer for momentum density studies on heavy-element materials. J. Phys. Chem. Solids 65, 2061–2064 (2004).
Kresse, G. & Joubert, D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B 59, 1758–1775 (1999).
Kresse, G. & Furthmuller, J. Efficiency of ab-initio total energy calculations for metals and semiconductors using a plane-wave basis set. Comput. Mater. Sci. 6, 15–50 (1996).
Kresse, G. & Furthmuller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 54, 11169–11186 (1996).
Perdew, J. P., Burke, K. & Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 77, 3865–3868 (1996).
Sun, J., Ruzsinszky, A. & Perdew, J. P. Strongly constrained and appropriately normed semilocal density functional. Phys. Rev. Lett. 115, 036402 (2015).
van de Walle, A. et al. Efficient stochastic generation of special quasirandom structures. Calphad 42, 13–18 (2013).
van de Walle, A., Asta, M. & Ceder, G. The alloy theoretic automated toolkit: a user guide. Calphad 26, 539–553 (2002).
Makkonen, I., Hakala, M. & Puska, M. Calculation of valence electron momentum densities using the projector augmented-wave method. J. Phys. Chem. Solids 66, 1128–1135 (2005).
Duncanson, W. E. & Coulson, C. A. Theoretical shape of the Compton profile for atoms from H to Ne. Proc. Phys. Soc. 57, 190 (1945).
Biggs, F., Mendelsohn, L. B. & Mann, J. B. Hartree-Fock Compton profiles for the elements. Atomic Data Nucl. Data Tables 16, 201–309 (1975).
Kaijser, P. & Smith, V. H. Jr Evaluation of momentum distributions and Compton profiles for atomic and molecular systems. Adv. Quantum Chem. 10, 37–76 (1977).
Weyrich, W., Pattison, P. & Williams, B. G. Fourier analysis of the Compton profile: atoms and molecules. Chem. Phys. 41, 271–284 (1979).
Yu, M. & Trinkle, D. R. Accurate and efficient algorithm for Bader charge integration. J. Chem. Phys. 134, 064111 (2011).
Maintz, S., Deringer, V. L., Tchougreeff, A. L., Dronskowski, R. & Dronskowski, R. LOBSTER: a tool to extract chemical bonding from plane-wave based DFT. J. Comput. Chem. 37, 1030–1035 (2016).
Acknowledgements
We thank M. Radin for providing feedback on the manuscript; A. Terasaka (Gunma University) for technical support with the Compton scattering experiment; and M. Nakayama (Nagoya Institute of Technology) for sharing insights into COOP analysis. K.S. was supported by Japan Society for the Promotion of Science (JSPS) KAKENHI grants JP15K17873 and JP19K05519. Compton scattering experiments were performed with the approval of the Japan Synchrotron Radiation Research Institute (JASRI; proposals 2017A1122 and 2019B1668). The work at Northeastern University was supported by US Department of Energy (DOE), Office of Science, Basic Energy Sciences grant DE-FG02-07ER46352; and benefited from Northeastern University’s Advanced Scientific Computation Center (ASCC), and the allocation of time at the National Energy Research Scientific Computing Center (NERSC)’s supercomputing center through DOE grant DE-AC02-05CH11231. The work at Carnegie Mellon University was supported by the Assistant Secretary for Energy Efficiency and Renewable Energy, Office of Vehicle Technologies of the US DOE through the Advanced Battery Materials Research (BMR) program under contract no. DE-EE0007810.
Author information
Authors and Affiliations
Contributions
H.H. performed all of the theoretical simulations and analysed the data. K.S., H.S., N.T and Y.S. performed the X-ray Compton scattering experiment and data analyses. N.Y., K.Y., Y.O. and Y.U. synthesized and characterized the materials. H.H. and B.B. wrote the manuscript. V.V. and A.B. contributed to the theory and oversaw the research and writing of the manuscript. All authors were involved in revising the manuscript.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Peer review information Nature thanks B. L. Ahuja, Stephen Dugdale and the other, anonymous, reviewer(s) for their contribution to the peer review of this work.
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Extended data figures and tables
Extended Data Fig. 1 Experimental valence Compton profiles of LixTi0.4Mn0.4O2 with different lithium concentrations.
Lithium concentrations ranged from x = 0 to x = 1.2.
Extended Data Fig. 2 Comparison of experimental CPDs in LTMO with theoretical models and fits.
CPDs are denoted as ∆J. The blue line shows the result of linear combination of atomic oxygen 2p orbitals; the cyan line represents the atomic lithium 2s orbitals; and the yellow line shows a model Coulomb repulsion profile for manganese 3d orbitals (see text). The experimental CPD is the difference in the Compton profiles for lithium concentrations of x = 0.8 minus x = 0.4. The model Coulomb repulsion profile was obtained analytically to take into account the effects of localization of the atomic manganese 3d orbitals using normalized Slater-type orbitals. The inset shows manganese 3d Compton profiles for two different effective values of Zeff. The fitting curve (red) and ∆J are both normalized to one. The experimental error bars were obtained from a statistical analysis of the curves in Extended Data Fig. 1 and have a total length of 2σ.
Extended Data Fig. 3 Spin-dependent PDOS associated with manganese eg, manganese t2g and oxygen p orbitals in LixTi0.4Mn0.4O2 for lithium concentrations of x = 0.4 and x = 0.8.
a, x = 0.4. b, x = 0.8. The vertical dashed lines mark the Fermi energy (EF). Up and down arrows indicate the contributions of spin-up and spin-down, respectively, to the PDOS.
Extended Data Fig. 4 COOP analysis between oxygen 2p and manganese 3d states for a lithium concentration of x = 0.4.
The vertical dashed line marks the Fermi energy (EF). COOP analysis indicates an antibonding character (green shaded area) for localized oxygen 2p states right above the Fermi level, as shown in the PDOS in Fig. 2 and Extended Data Fig. 3a. Here, localized oxygen 2p holes point in the direction of a lithium-atom vacancy along the direction of the Li–O–Li axis.
Extended Data Fig. 5 Fourier transform, B(r), of the CPD.
The reciprocal form factor B(r) was computed for the CPD (black solid line), the fitted curve (red line) and the Coulomb profile (yellow line) shown in Extended Data Fig. 2. The B(r) for experimental Coulomb profile (black dashed line) was obtained by subtracting the B(r) of atomic oxygen 2p (blue line) from the experimental B(r) (black solid line). Agreement between the fitted B(r) (red line) and the experimental B(r) (black solid line) was used to assess the goodness of fit of Extended Data Fig. 2.
Rights and permissions
About this article
Cite this article
Hafiz, H., Suzuki, K., Barbiellini, B. et al. Tomographic reconstruction of oxygen orbitals in lithium-rich battery materials. Nature 594, 213–216 (2021). https://doi.org/10.1038/s41586-021-03509-z
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41586-021-03509-z
This article is cited by
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.