Abstract
The nature of the pseudogap phase of the copper oxides (‘cuprates’) remains a puzzle. Although there are indications that this phase breaks various symmetries, there is no consensus on its fundamental nature1. Fermi-surface, transport and thermodynamic signatures of the pseudogap phase are reminiscent of a transition into a phase with antiferromagnetic order, but evidence for an associated long-range magnetic order is still lacking2. Here we report measurements of the thermal Hall conductivity (in the x–y plane, κxy) in the normal state of four different cuprates—La1.6−xNd0.4SrxCuO4, La1.8−xEu0.2SrxCuO4, La2−xSrxCuO4 and Bi2Sr2−xLaxCuO6+δ. We show that a large negative κxy signal is a property of the pseudogap phase, appearing at its critical hole doping, p*. It is also a property of the Mott insulator at p ≈ 0, where κxy has the largest reported magnitude of any insulator so far3. Because this negative κxy signal grows as the system becomes increasingly insulating electrically, it cannot be attributed to conventional mobile charge carriers. Nor is it due to magnons, because it exists in the absence of magnetic order. Our observation is reminiscent of the thermal Hall conductivity of insulators with spin-liquid states4,5,6, pointing to neutral excitations with spin chirality7 in the pseudogap phase of cuprates.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
Data availability
The data that support the plots within this paper and other findings of this study are available from the corresponding authors upon reasonable request.
References
Keimer, B. et al. From quantum matter to high-temperature superconductivity in copper oxides. Nature 518, 179–186 (2015).
Proust, C. & Taillefer, L. The remarkable underlying ground states of cuprate superconductors. Annu. Rev. Condens. Matter Phys. 10, 409–429 (2019).
Ideue, T. et al. Giant thermal Hall effect in multiferroics. Nat. Mater. 16, 797–802 (2017).
Kasahara, Y. et al. Unusual thermal Hall effect in a Kitaev spin liquid candidate α-RuCl3. Phys. Rev. Lett. 120, 217205 (2018).
Watanabe, D. et al. Emergence of nontrivial magnetic excitations in a spin-liquid state of kagomé volborthite. Proc. Natl Acad. Sci. USA 113, 8653–8657 (2016).
Doki, H. et al. Spin thermal Hall conductivity of a Kagome antiferromagnet. Phys. Rev. Lett. 121, 097203 (2018).
Lee, H., Han, J. H. & Lee, P. A. Thermal Hall effect of spins in a paramagnet. Phys. Rev. B 91, 125413 (2015).
Monthoux, P., Pines, D. & Lonzarich, G. G. Superconductivity without phonons. Nature 450, 1177–1183 (2007).
Kyung, B. et al. Pseudogap induced by short-range spin correlations in a doped Mott insulator. Phys. Rev. B 73, 165114 (2006).
Scheurer, M. S. et al. Topological order in the pseudogap metal. Proc. Natl Acad. Sci. USA 115, E3665–E3672 (2018).
Nasu, J., Yoshitake, J. & Motome, Y. Thermal transport in the Kitaev model. Phys. Rev. Lett. 119, 127204 (2017).
Hirschberger, M. et al. Large thermal Hall conductivity of neutral spin excitations in a frustrated quantum magnet. Science 348, 106–109 (2015).
Zhang, Y. et al. Giant enhancement of the thermal Hall conductivity κ xy in the superconductor YBa2Cu3O7. Phys. Rev. Lett. 86, 890–893 (2001).
Durst, A. C., Vishwanath, A. & Lee, P. A. Weak-field thermal Hall conductivity in the mixed state of d-wave superconductors. Phys. Rev. Lett. 90, 187002 (2003).
Cvetkovic, V. & Vafek, O. Berry phases and the intrinsic thermal Hall effect in high-temperature cuprate superconductors. Nat. Commun. 6, 6518 (2015).
Grissonnanche, G. et al. Wiedemann-Franz law in the underdoped cuprate superconductor YBa2Cu3Oy. Phys. Rev. B 93, 064513 (2016).
Collignon, C. et al. Fermi-surface transformation across the pseudogap critical point of the cuprate superconductor La1.6−xNd0.4SrxCuO4. Phys. Rev. B 95, 224517 (2017).
Kawasaki, S. et al. Carrier-concentration dependence of the pseudogap ground state of superconducting Bi2Sr2−xLaxCuO6 revealed by 63,65Cu-nuclear magnetic resonance in very high magnetic fields. Phys. Rev. Lett. 105, 137002 (2010).
Strohm, C., Rikken, G. L. J. A. & Wyder, P. Phenomenological evidence for the phonon Hall effect. Phys. Rev. Lett. 95, 155901 (2005).
Sugii, K. et al. Thermal Hall effect in a spin-phonon glass Ba3CuSb2O9. Phys. Rev. Lett. 118, 145902 (2017).
Nachumi, B. et al. Muon spin relaxation study of the stripe phase order in La1.6−xNd0.4SrxCuO4 and related 214 cuprates. Phys. Rev. B 58, 8760–8772 (1998).
Katsura, H., Nagaosa, N. & Lee, P. A. Theory of the thermal Hall effect in quantum magnets. Phys. Rev. Lett. 104, 066403 (2010).
Hentrich, R. et al. Large thermal Hall effect in α-RuCl3: evidence for heat transport by Kitaev-Heisenberg paramagnons. Phys. Rev. B 99, 085136 (2019).
Kasahara, Y. et al. Majorana quantization and half-integer thermal quantum Hall effect in a Kitaev spin liquid. Nature 559, 227–231 (2018).
Kivelson, S. A., Rokhsar, D. S. & Sethna, J. P. Topology of the resonating valence-bond state: solitons and high-T c superconductivity. Phys. Rev. B 35, 8865 (1987).
Varma, C. M. Theory of the pseudogap state of the cuprates. Phys. Rev. B 73, 155113 (2006).
Samajdar, R., Chatterjee, S., Sachdev, S. & Scheurer, M. Thermal Hall effect in square-lattice spin liquids: a Schwinger boson mean-field study. Phys. Rev. B 99, 165126 (2019).
Onose, Y. et al. Observation of the magnon Hall effect. Science 329, 297–299 (2010).
Cyr-Choinière, O. et al. Pseudogap temperature T* of cuprate superconductors from the Nernst effect. Phys. Rev. B 97, 064502 (2018).
Michon, B. et al. Thermodynamic signatures of quantum criticality in cuprate superconductors. Nature 567, 218–222 (2019).
Klauss, H.-H. et al. From antiferromagnetic order to static magnetic stripes: the phase diagram of (La, Eu)2−xSrxCuO4. Phys. Rev. Lett. 85, 4590–4593 (2000).
Hücker, M. et al. Coupling of stripes to lattice distortions in cuprates and nickelates. Physica C 460–462, 170–173 (2007).
Ono, S. & Ando, Y. Evolution of the resistivity anisotropy in Bi2Sr2−xLaxCuO6+δ single crystals for a wide range of hole doping. Phys. Rev. B 67, 104512 (2003).
Uchida, S. et al. Electric and magnetic properties of La2CuO4. Jpn. J. Appl. Phys. 26, L445 (1987).
Komiya, S., Ando, Y., Sun, X. F. & Lavrov, A. N. c-axis transport and resistivity anisotropy of lightly to moderately doped La2−xSrxCuO4 single crystals: implications on the charge transport mechanism. Phys. Rev. B 65, 214535 (2002).
Keimer, B. et al. Soft phonon behavior and magnetism at the low temperature structural phase transition of La1.65Nd0.35CuO4. Z. Phys. B 91, 373–382 (1993).
Hess, C. et al. Magnon heat transport in doped La2CuO4. Phys. Rev. Lett. 90, 197002 (2003).
Lavrov, A. N., Ando, Y., Komiya, S. & Tsukada, I. Unusual magnetic susceptibility anisotropy in untwinned La2−xSrxCuO4 single crystals in the lightly doped region. Phys. Rev. Lett. 87, 017007 (2001).
Li, Q. J. et al. Phonon-glass-like behavior of magnetic origin in single crystal Tb2Ti2O7. Phys. Rev. B 87, 214408 (2013).
Michon, B. et al. Wiedemann-Franz law and abrupt change in conductivity across the pseudogap critical point of a cuprate superconductor. Phys. Rev. X 8, 041010 (2018).
Acknowledgements
We thank L. Balents, K. Behnia, S. Chatterjee, B. D. Gaulin, H. J. Han, S. M. Hayden, C. Hess, S. A. Kivelson, H. Y. Kee, P. A. Lee, Y. S. Lee, A. Rosch, S. Sachdev, M. Scheurer, T. Senthil, A.-M. S. Tremblay, C. M. Varma and S. Verret for helpful and stimulating discussions. L.T. acknowledges support from the Canadian Institute for Advanced Research (CIFAR) as a CIFAR Fellow and funding from the Natural Sciences and Engineering Research Council of Canada (NSERC), the Fonds de recherche du Québec–Nature et Technologies (FRQNT), the Canada Foundation for Innovation (CFI), and a Canada Research Chair. This research was undertaken thanks in part to funding from the Canada First Research Excellence Fund. Part of this work was funded by the Gordon and Betty Moore Foundation’s EPiQS Initiative (grant GBMF5306 to L.T.). J.-S.Z was supported by NSF MRSEC DMR-1720595 in the US.
Reviewer information
Nature thanks Kwang-yong Choi, Patrick Lee and the other anonymous reviewer(s) for their contribution to the peer review of this work.
Author information
Authors and Affiliations
Contributions
G.G., A.L., S.B., E.L., V.Z., M.L., F.L., A.G. and N.D.-L. performed the thermal Hall conductivity measurements. G.G., A.L., S.B., E.L., V.Z., M.L. and N.D.-L. performed the electrical Hall conductivity measurements. J.-S.Z. grew the Nd-LSCO single crystals. S.P., T.T. and H.T. grew the Eu-LSCO and LSCO single crystals. S.O. grew the Bi2201 single crystal. G.G., N.D.-L. and L.T. wrote the manuscript, in consultation with all authors. L.T. supervised the project.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Extended data figures and tables
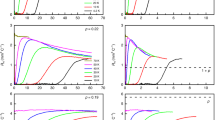
Extended Data Fig. 1 Magnetic field dependence of κxx.
a–f, Field dependence of κxx in Eu-LSCO p = 0.08 (top panels) and LSCO p = 0.06 (bottom panels), displayed in three ways. a, d, Plot of κxx/T versus T at H = 1 T (blue) and H = 15 T (red) (data points). The difference between the two curves is very small, not visible by eye. b, e, Plot of the change in κxx with field measured relative to its value at H = 1 T, that is, [κxx(H) − κxx(1 T)] versus H, for various temperatures as indicated (data points). c, f, Change in κxx between 15 T and 1 T, plotted as [κxx(H) − κxx(1 T)]/T versus T (blue, right axis), compared to κxy(15 T)/T versus T (red, left axis) (data points). Markers represent data and the line is a guide to the eye. Note how at low T the transverse response grows to be as large, if not larger, than the longitudinal response.
Extended Data Fig. 2 Comparison of cuprates to other oxides.
a, Thermal conductivity of two isostructural oxides, plotted as κxx/T versus T at H = 0, namely Y2Ti2O7 (red) and Tb2Ti2O7 (blue) (data points39). The presence of disordered magnetic moments in Tb2Ti2O7 produces a strong scattering of phonons, seen as a massive suppression of κxx (15-fold at T = 15 K). b, Field dependence of κxx, plotted as Δκxx(H)/κxx(0) versus H, with Δκxx = κxx(H) − κxx(0), at T = 15 K (blue data points12). The strong effect of field (30% in 8 T) is a direct signature of the strong coupling between phonons and spins in Tb2Ti2O7. Also shown is the transverse response in Tb2Ti2O7 at T = 15 K, plotted as κxy/T versus H (red data points12). Note that in Y2Ti2O7, κxy = 0 (ref. 12). c, Thermal conductivity of two Nd-LSCO samples, on either side of p* (red, p = 0.24; blue, p = 0.21), plotted as κxx/T versus T at H = 18 T (data points). We see that contrary to Tb2Ti2O7 (a), the appearance of the negative κxy signal in Nd-LSCO below p* is not accompanied by a large suppression of κxx (see Extended Data Fig. 3). d, Same as b but for LSCO p = 0.06, with the same x-axis and y-axis scales and data taken at (nearly) the same temperature (data points). We see that the situation in LSCO is very different to that found in Tb2Ti2O7 (b): instead of having a small κxy and a large Δκxx (b), we now have a large κxy and a small Δκxx. Quantitatively, κxy/Δκxx ≈ 1 in LSCO and approximately 0.01 in Tb2Ti2O7, at T = 15 K and H = 8 T (Table 1).
Extended Data Fig. 3 Change in phonon κxx across p* in Nd-LSCO.
a, Thermal conductivity of Nd-LSCO at four different dopings, above p* (p = 0.24) and below p* (p = 0.20, 0.21, 0.22), plotted as κxx/T versus T, at H = 18 T (data points). We see that κxx increases below p*. b, Same as a but for Nd-LSCO p = 0.21 (blue; H = 18 T) and LSCO p = 0.06 (green, H = 16 T). We see that κxx continues to increase as we lower p further. This shows that phonons conduct better at lower p. A natural explanation is that they are less scattered by charge carriers as the material becomes less metallic. c, Same data as in a for Nd-LSCO p = 0.21 (blue data points) and p = 0.24 (red data points), compared to the electrical conductivity of those same samples, plotted as L0/ρ versus T (lines; measured at H = 33 T (ref. 17)). The latter curves are a reasonable estimate of the electronic thermal conductivity \({\kappa }_{xx}^{{\rm{el}}}\), exact at T → 0 (since the Wiedemann–Franz law is satisfied40), as seen in Fig. 2a. d, Estimate of the phonon conductivity, defined as \({\kappa }_{xx}^{{\rm{ph}}}={\kappa }_{xx}-{L}_{0}T/\rho \), plotted as \({\kappa }_{xx}^{{\rm{ph}}}/T\) versus T (using data from c) (data points). We see that \({\kappa }_{xx}^{{\rm{ph}}}(T)\) increases upon crossing below p*, most probably because electron–phonon scattering is weakened by the loss of carrier density. There is no evidence that the phonons suddenly suffer from the onset of strong spin scattering below p* (which would cause \({\kappa }_{xx}^{{\rm{ph}}}(T)\) to drop below p*), such as would be required to explain the appearance of the large negative κxy signal below p* (Fig. 3) as being due to phonon transport.
Extended Data Fig. 4 Magnetic field dependence of κxy in LSCO.
a, Field dependence of the thermal Hall conductivity of LSCO at p = 0.06, plotted as κxy versus H at various temperatures, as indicated (data points). The dependence of κxy on H is linear at high T and it becomes sublinear at lower T. b, Deviation from linearity displayed by plotting κxy/(TH) versus T at four different fields H, as indicated (data points).
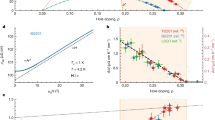
Extended Data Fig. 5 Magnon thermal conductivity in La2CuO4.
Thermal conductivity of magnons in La2CuO4, plotted as κmag/T versus T (blue data points, right axis; ref. 37). The solid blue line is a fit to the data using the standard calculation for two magnon branches in 2D, with gaps as measured by neutron inelastic scattering36, namely Δ1 = 26 K and Δ2 = 58 K. Below T ≈ 5 K, thermally excited magnons are exponentially rare and κmag/T ≈ 0. In sharp contrast, the thermal Hall conductivity of La2CuO4, |κxy/T| (red data points, left axis; the red line is a guide to the eye; Fig. 1b), is largest at T ≈ 5 K. This comparison shows that the κxy signal in La2CuO4 cannot come from magnon transport.
Extended Data Fig. 6 Electrical resistivity in La2CuO4.
Electrical resistivity, ρxx, of two of our samples—La2CuO4 (LCO, red) and LSCO at p = 0.06 (blue)—compared with published data for La2CuO4 (yellow34) and LSCO at p = 0.01 (green35). This shows that our LCO sample is very close to the Mott insulator La2CuO4, being more insulating than LSCO with p = 0.01 and much more insulating than our LSCO sample with p = 0.06.
Rights and permissions
About this article
Cite this article
Grissonnanche, G., Legros, A., Badoux, S. et al. Giant thermal Hall conductivity in the pseudogap phase of cuprate superconductors. Nature 571, 376–380 (2019). https://doi.org/10.1038/s41586-019-1375-0
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41586-019-1375-0
This article is cited by
-
Phonon chirality from impurity scattering in the antiferromagnetic phase of Sr2IrO4
Nature Physics (2024)
-
Thermal Hall effects due to topological spin fluctuations in YMnO3
Nature Communications (2024)
-
The phonon thermal Hall angle in black phosphorus
Nature Communications (2023)
-
Electrical and thermal transport properties of kagome metals AV3Sb5 (A = K, Rb, Cs)
Tungsten (2023)
-
Unconventional short-range structural fluctuations in cuprate superconductors
Scientific Reports (2022)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.