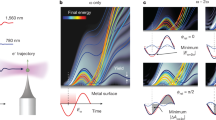
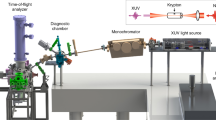
Abstract
Photoemission spectroscopy is central to understanding the inner workings of condensed matter, from simple metals and semiconductors to complex materials such as Mott insulators and superconductors1. Most state-of-the-art knowledge about such solids stems from spectroscopic investigations, and use of subfemtosecond light pulses can provide a time-domain perspective. For example, attosecond (10−18 seconds) metrology allows electron wave packet creation, transport and scattering to be followed on atomic length scales and on attosecond timescales2,3,4,5,6,7. However, previous studies could not disclose the duration of these processes, because the arrival time of the photons was not known with attosecond precision. Here we show that this main source of ambiguity can be overcome by introducing the atomic chronoscope method, which references all measured timings to the moment of light-pulse arrival and therefore provides absolute timing of the processes under scrutiny. Our proof-of-principle experiment reveals that photoemission from the tungsten conduction band can proceed faster than previously anticipated. By contrast, the duration of electron emanation from core states is correctly described by semiclassical modelling. These findings highlight the necessity of treating the origin, initial excitation and transport of electrons in advanced modelling of the attosecond response of solids, and our absolute data provide a benchmark. Starting from a robustly characterized surface, we then extend attosecond spectroscopy towards isolating the emission properties of atomic adsorbates on surfaces and demonstrate that these act as photoemitters with instantaneous response. We also find that the tungsten core-electron timing remains unchanged by the adsorption of less than one monolayer of dielectric atoms, providing a starting point for the exploration of excitation and charge migration in technologically and biologically relevant adsorbate systems.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout



Similar content being viewed by others
Data availability
The data that support the findings of this study are available from the corresponding authors upon request.
References
Damascelli, A. Probing the electronic structure of complex systems by ARPES. Phys. Scr. T 109, 61–62 (2004).
Cavalieri, A. L. et al. Attosecond spectroscopy in condensed matter. Nature 449, 1029–1032 (2007).
Neppl, S. et al. Direct observation of electron propagation and dielectric screening on the atomic length scale. Nature 517, 342–346 (2015).
Seiffert, L. et al. Attosecond chronoscopy of electron scattering in dielectric nanoparticles. Nat. Phys. 13, 766–770 (2017).
Siek, F. et al. Angular momentum–induced delays in solid-state photoemission enhanced by intra-atomic interactions. Science 357, 1274–1277 (2017).
Chen, C. et al. Distinguishing attosecond electron–electron scattering and screening in transition metals. Proc. Natl Acad. Sci. USA 114, E5300–E5307 (2017).
Tao, Z. et al. Direct time-domain observation of attosecond final-state lifetimes in photoemission from solids. Science 353, 62–67 (2016).
Kienberger, R. et al. Atomic transient recorder. Nature 427, 817–821 (2004).
Muller, H. G. Reconstruction of attosecond harmonic beating by interference of two-photon transitions. Appl. Phys. B 74, s17–s21 (2002).
Schultze, M. et al. Delay in photoemission. Science 328, 1658–1662 (2010).
Pazourek, R., Nagele, S. & Burgdörfer, J. Attosecond chronoscopy of photoemission. Rev. Mod. Phys. 87, 765–802 (2015).
Lucchini, M. et al. Light-matter interaction at surfaces in the spatiotemporal limit of macroscopic models. Phys. Rev. Lett. 115, 137401 (2015).
Locher, R. et al. Energy-dependent photoemission delays from noble metal surfaces by attosecond interferometry. Optica 2, 405–410 (2015).
Kasmi, L. et al. Effective mass effect in attosecond electron transport. Optica 4, 1492–1497 (2017).
Ossiander, M. et al. Attosecond correlation dynamics. Nat. Phys. 13, 280–285 (2017).
Palacios, A., McCurdy, C. W. & Rescigno, T. N. Extracting amplitudes for single and double ionization from a time-dependent wave packet. Phys. Rev. A 76, 043420 (2007).
Pazourek, R., Feist, J., Nagele, S. & Burgdörfer, J. Attosecond streaking of correlated two-electron transitions in helium. Phys. Rev. Lett. 108, 163001 (2012).
Magerl, E. et al. A flexible apparatus for attosecond photoelectron spectroscopy of solids and surfaces. Rev. Sci. Instrum. 82, 063104 (2011).
Cavalieri, A. L. et al. Intense 1.5-cycle near infrared laser waveforms and their use for the generation of ultra-broadband soft-X-ray harmonic continua. New J. Phys. 9, 242 (2007).
Eisenbud, L. The Formal Properties of Nuclear Collisions. PhD thesis, Princeton Univ. (1948).
Wigner, E. P. Lower limit for the energy derivative of the scattering phase shift. Phys. Rev. 98, 145–147 (1955).
Smith, F. T. Lifetime matrix in collision theory. Phys. Rev. 118, 349–356 (1960).
Huppert, M., Jordan, I., Baykusheva, D., von Conta, A. & Wörner, H. J. Attosecond delays in molecular photoionization. Phys. Rev. Lett. 117, 093001 (2016).
Heuser, S. et al. Angular dependence of photoemission time delay in helium. Phys. Rev. A 94, 063409 (2016).
Neppl, S. et al. Attosecond time-resolved photoemission from core and valence states of magnesium. Phys. Rev. Lett. 109, 087401 (2012).
Tanuma, S., Powell, C. J. & Penn, D. R. Calculations of electron inelastic mean free paths. II. Data for 27 elements over the 50–2000 eV range. Surf. Interface Anal. 17, 911–926 (1991).
Krasovskii, E. E. Attosecond spectroscopy of solids: streaking phase shift due to lattice scattering. Phys. Rev. B 84, 195106 (2011).
Mirhosseini, H., Flieger, M. & Henk, J. Dirac-cone-like surface state in W(110): dispersion, spin texture and photoemission from first principles. New J. Phys. 15, 033019 (2013).
Pi, T.-W., Hong, L.-H. & Cheng, C.-P. Synchrotron-radiation photoemission study of Ba on W(110). Phys. Rev. B 58, 4149–4155 (1998).
Riemensberger, J. Time-Frequency-Resolved Absolute Time Delay of the Photoelectric Effect. PhD Thesis, Technische Universität München (2018).
Yakovlev, V. S., Gagnon, J., Karpowicz, N. & Krausz, F. Attosecond streaking enables the measurement of quantum phase. Phys. Rev. Lett. 105, 073001 (2010).
Kazansky, A. K. & Echenique, P. M. Theoretical study of the ionization of an alkali atom adsorbed on a metal surface by a laser-assisted subfemtosecond pulse. Phys. Rev. B 81, 075440 (2010).
Jones, R. G. Halogen adsorption on solid surfaces. Prog. Surf. Sci. 27, 25–160 (1988).
Jones, R. G. & Dowben, P. A. Reply to comments on “A re-interpretation of the LEED structures formed by iodine on W(110)” by P. A. Dowben and R. G. Jones. Surf. Sci. 116, L228–L231 (1982).
Dowben, P. A. & Jones, R. G. A re-interpretation of the LEED structures formed by iodine on W(110). Surf. Sci. 105, 334–346 (1981).
Shirley, D. High-resolution X-ray photoemission spectrum of the valence bands of gold. Phys. Rev. B 5, 4709–4714 (1972).
Quéré, F., Mairesse, Y. & Itatani, J. Temporal characterization of attosecond XUV fields. J. Mod. Opt. 52, 339–360 (2005).
Cutler, J. N., Bancroft, G. M., Sutherland, D. G. & Tan, K. H. Chemical dependence of core-level linewidths and ligand-field splittings: high-resolution core-level photoelectron spectra of I4d levels. Phys. Rev. Lett. 67, 1531–1534 (1991).
Neppl, S. Attosecond Time-Resolved Photoemission from Surfaces and Interfaces (Technische Universität München, München, 2012).
Gagnon, J., Goulielmakis, E. & Yakovlev, V. S. The accurate FROG characterization of attosecond pulses from streaking measurements. Appl. Phys. B 92, 25–32 (2008).
Dunin von Przychowski, M., Wiechert, H., Marx, G. K. L. & Schönhense, G. Real-space observation of xenon adsorption and desorption kinetics on graphite (0001) by photoemission electron microscopy. Surf. Sci. 541, 46–58 (2003).
Schmidt, M. W. et al. General atomic and molecular electronic structure system. J. Comput. Chem. 14, 1347–1363 (1993).
Schuchardt, K. L. et al. Basis Set Exchange: a community database for computational sciences. J. Chem. Inf. Model. 47, 1045–1052 (2007).
Feller, D. The role of databases in support of computational chemistry calculations. J. Comput. Chem. 17, 1571–1586 (1996).
Barbieri, P. L., Fantin, P. A. & Jorge, F. E. Gaussian basis sets of triple and quadruple zeta valence quality for correlated wave functions. Mol. Phys. 104, 2945–2954 (2006).
Campos, C. T. & Jorge, F. E. Triple zeta quality basis sets for atoms Rb through Xe: application in CCSD(T) atomic and molecular property calculations. Mol. Phys. 111, 167–173 (2013).
Yanai, T., Tew, D. P. & Handy, N. C. A new hybrid exchange correlation functional using the Coulomb-attenuating method (CAM-B3LYP). Chem. Phys. Lett. 393, 51–57 (2004).
Perdew, J. P. & Zunger, A. Self-interaction correction to density-functional approximations for many-electron systems. Phys. Rev. B 23, 5048–5079 (1981).
Natalense, A. P. P. & Lucchese, R. R. Cross section and asymmetry parameter calculation for sulfur 1s photoionization of SF6. J. Chem. Phys. 111, 5344–5348 (1999).
Gianturco, F. A., Lucchese, R. R. & Sanna, N. Calculation of low energy elastic cross sections for electron-CF4 scattering. J. Chem. Phys. 100, 6464–6471 (1994).
Hockett, P., Frumker, E., Villeneuve, D. M. & Corkum, P. B. Time delay in molecular photoionization. J. Phys. B 49, 095602 (2016).
Nahon, L., Svensson, A. & Morin, P. Experimental study of the 4d ionization continuum in atomic iodine by photoelectron and photoion spectroscopy. Phys. Rev. A 43, 2328–2337 (1991).
Olney, T. N., Cooper, G. & Brion, C. Quantitative studies of the photoabsorption (4.5–488 eV) and photoionization (9–59.5 eV) of methyl iodide using dipole electron impact techniques. Chem. Phys. 232, 211–237 (1998).
Amusia, M. Y., Cherepkov, N. A., Chernysheva, L. V. & Manson, S. T. Photoionization of atomic iodine and its ions. Phys. Rev. A 61, 020701 (2000).
Fano, U. Effects of configuration interaction on intensities and phase shifts. Phys. Rev. 124, 1866–1878 (1961).
Burgdörfer, J. Dynamical image charge effects on convoy electron emission from solid surfaces. Nucl. Instrum. Methods B 24–25, 139–142 (1987).
Weaver, J. H., Olson, C. G. & Lynch, D. W. Optical properties of crystalline tungsten. Phys. Rev. B 12, 1293–1297 (1975).
Connerade, J. P. in Giant Resonances in Atoms, Molecules, and Solids (eds Connerade, J. P., Esteva, J. M. & Karnatak, R. C.) 3–23 (NATO Sci. Ser. B, Vol. 151, Springer Science+Business Media, New York, 1987).
Kresse, G. & Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 54, 11169–11186 (1996).
Kresse, G. & Joubert, D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B 59, 1758–1775 (1999).
Kresse, G. & Hafner, J. Ab initio molecular dynamics for liquid metals. Phys. Rev. B 47, 558–561 (1993).
Kresse, G. & Hafner, J. Ab initio molecular-dynamics simulation of the liquid-metal–amorphous-semiconductor transition in germanium. Phys. Rev. B 49, 14251–14269 (1994).
Lemell, C., Solleder, B., Tőkési, K. & Burgdörfer, J. Simulation of attosecond streaking of electrons emitted from a tungsten surface. Phys. Rev. A 79, 062901 (2009).
Salvat, F., Jablonski, A. & Powell, C. J. ELSEPA — Dirac partial-wave calculation of elastic scattering of electrons and positrons by atoms, positive ions and molecules. Comput. Phys. Commun. 165, 157–190 (2005).
Acknowledgements
We acknowledge discussions with M. Schultze, experimental support by A. Kim and A. Schiffrin and infrastructural support by F. Krausz. This work was supported by the Max Planck Society, the Deutsche Forschungsgemeinschaft Cluster of Excellence, Munich Centre for Advanced Photonics, a Consolidator Grant from the European Research Council (ERC-2014-CoG AEDMOS), LASERLAB-EUROPE (grant agreement number 654148, European Union’s Horizon 2020 research and innovation programme), FWF Austria (SFB-041 ViCoM, SFB-049 NextLite) and COST Action CM1204 (XLIC). Calculations were performed using the Vienna Scientific Cluster (VSC).
Reviewer information
Nature thanks M. Chini and T. Fennel for their contribution to the peer review of this work.
Author information
Authors and Affiliations
Contributions
M.O., J.R., S.N., M.M., M. Schäffer, A.D., M.S.W., R.H., M.W., M.G. and M. Schnitzenbaumer carried out the experiments. M.O. and J.R. analysed the experimental data. F.L., C.L. and J.B. performed the electron transport and DFT calculations. M.O. wrote the initial manuscript. J.V.B., P.F. and R.K. supervised the study. All authors discussed and reviewed the manuscript.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Extended data figures and tables
Extended Data Fig. 1 I/W(110) and W(110) measurements.
a, Main panel, XUV I/W(110) photoelectron spectra taken at 105 eV central photon energy for different adsorbate surface coverages (key in top left inset). Three effects are observable with decreasing iodine surface coverage: a decrease of the I4d peak intensity, an increase of the W4f peak intensity due to reduced inelastic scattering, and a shape change of the valence-electron peak towards a clean tungsten spectrum. The iodine surface density was calibrated by taking a full monolayer I/W(110) photoelectron spectrum as a reference before thermal desorption and comparing the I4d photoelectron flux before and after the thermal desorption of iodine. Because the iodine surface coverage saturates, this allows for a reliable coverage calibration. Top centre inset, illustration of the employed Shirley background (BG) subtraction scheme; top right inset, the magnified valence photoelectron spectrum. b, Relative photoemission delays for I/W(110). Both W4f to I4d (blue) and valence to I4d (red) delays are shown. All individual measurements are depicted by crosses, averages for individual coverages are depicted by circles. Vertical error bars mark 95% confidence assuming a Student’s t-distribution and horizontal error bars mark maximum errors. The blue line represents a linear regression to the W4f to I4d delay (blue line), the shaded area represents the 95% confidence interval of this model. c, Attosecond streaking delay measurements on a pristine W(110) surface. The W4f−CB emission delay (blue circles and histogram) as a function of the time of measurement after the preparation of a pristine surface (yellow) reveals a small deviation of the centre (red, dashed line) of the normal distribution (red, solid line) fit to all measurements from the extrapolation to an instantaneous measurement due to surface contamination. The large number of measurements allows the extraction of the photoemission timing of the clean surface by extrapolation.
Extended Data Fig. 2 Typical streaking spectrogram measured for I/W(110) at 32% of the saturated iodine surface coverage and its reconstruction.
The first column shows the experimental data, the second column the results of the reconstruction algorithm and the third column their difference. The residual mainly consists of background independent of the XUV−NIR-delay, which is cancelled during the retrieval by differentiating along the delay axis. The three rows focus, from top to bottom, on the streaking of the valence, W4f and I4d photoemission peaks. The XUV−NIR-delay difference between consecutive spectra in the spectrogram is 200 as. The photoemission delay results extracted from this sample spectrogram are \({\rm{\Delta }}{\tau }_{{\rm{W}}4f-{\rm{I}}4d}=81\hspace{1pt}{\rm{as}}\) and \({\rm{\Delta }}{\tau }_{{\rm{Valence}}-{\rm{I}}4d}=14\hspace{1.5pt}{\rm{as}}\).
Extended Data Fig. 3 Gas-phase iodine/helium measurements.
a, Unstreaked XUV photoelectron spectra of iodoethane (red) and an iodoethane/helium mixture (blue) recorded at 105 eV central photon energy. Electrons emitted through the N4,5VV Auger process are spectrally separated from all timed photoelectron peaks. b, Relative I4d−He1s photoemission delay for different mixture compositions of iodoethane and helium. Shown are individual measurements (blue circles), linear regression (red line) and the 95% confidence interval of the regression (shaded area). c, Histograms (blue) of the individual relative photoemission delay measurements (yellow) between He1s and I4d electrons in iodomethane and iodoethane at 105 eV photon energy and normal-distribution fits to the data (red).
Extended Data Fig. 4 Results of DFT calculations.
a, DFT-derived electron density in the surface bandgap of a clean W(110) surface. A 2D cut through the \((1\bar{1}0)\) plane, perpendicular to the (110) surface, is shown. Tungsten atoms are indicated as black circles. Second-layer atoms are projected onto the plane of the cut. The inelastic mean free path λIMFP for conduction-band photoelectrons is marked as a guide to the eye. b, Energy-resolved density of states for an iodine-covered W(110) surface. The red dashed line represents the density of states in the proximity of the top-layer tungsten atoms and the blue dashed line represents the density of states near the iodine adsorbates. The full lines are folded with the spectrum of the experimental XUV pulse. The yellow line represents the full density of states of the iodine covered tungsten surface folded with the experimental XUV spectrum. See Methods for details of ‘jellium edge’ and EF.
Rights and permissions
About this article
Cite this article
Ossiander, M., Riemensberger, J., Neppl, S. et al. Absolute timing of the photoelectric effect. Nature 561, 374–377 (2018). https://doi.org/10.1038/s41586-018-0503-6
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41586-018-0503-6
Keywords
This article is cited by
-
Attosecond metrology of the two-dimensional charge distribution in molecules
Nature Physics (2024)
-
Tunnelling of electrons via the neighboring atom
Light: Science & Applications (2024)
-
Raman time-delay in attosecond transient absorption of strong-field created krypton vacancy
Nature Communications (2024)
-
Sub-cycle multidimensional spectroscopy of strongly correlated materials
Nature Photonics (2024)
-
Deep learning with coherent VCSEL neural networks
Nature Photonics (2023)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.