Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

  • Article
  • Published:

Quantum advantage with noisy shallow circuits

Nature Physics volume 16, pages 1040–1045 (2020)Cite this article

Subjects

Abstract

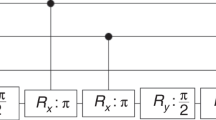
As increasingly sophisticated prototypes of quantum computers are being developed, a pressing challenge is to find computational problems that can be solved by an intermediate-scale quantum computer, but are beyond the capabilities of existing classical computers. Previous work in this direction has introduced computational problems that can be solved with certainty by quantum circuits of depth independent of the input size (so-called ‘shallow’ circuits) but cannot be solved with high probability by any shallow classical circuit. Here we show that such a separation in computational power persists even when the shallow quantum circuits are restricted to geometrically local gates in three dimensions and corrupted by noise. We also present a streamlined quantum algorithm that is shown to achieve a quantum advantage in a one-dimensional geometry. The latter may be amenable to experimental implementation with the current generation of quantum computers.

This is a preview of subscription content, access via your institution

Access options

Buy this article

  • Purchase on Springer Link
  • Instant access to full article PDF
Buy now

Prices may be subject to local taxes which are calculated during checkout

Fig. 1: A variant of the magic square game21,22.
Fig. 2: Quantum circuit for the 1D Magic Square Problem.
Fig. 3: Encoded 1D Magic Square circuit.

Similar content being viewed by others

Data availability

Data sharing is not applicable to this article as no datasets were generated or analysed during the current study.

References

  1. Shor, P. W. Polynomial-time algorithms for prime factorization and discrete logarithms on a quantum computer. SIAM Rev. 41, 303–332 (1999).

    Article  ADS  MathSciNet  Google Scholar 

  2. Aaronson, S. & Arkhipov, A. The computational complexity of linear optics. In Proc. 43rd Annual ACM Symposium on Theory of Computing (STOC ’11) 333–342 (Association for Computing Machinery, 2011).

  3. Bremner, M. J., Montanaro, A. & Shepherd, D. J. Achieving quantum supremacy with sparse and noisy commuting quantum computations. Quantum 1, 8 (2017).

    Article  Google Scholar 

  4. Farhi, E. & Harrow, A. W. Quantum supremacy through the quantum approximate optimization algorithm. Preprint at http://arXiv.org/abs/1602.07674 (2016).

  5. Bremner, M. J., Montanaro, A. & Shepherd, D. J. Average-case complexity versus approximate simulation of commuting quantum computations. Phys. Rev. Lett. 117, 080501 (2016).

    Article  ADS  MathSciNet  Google Scholar 

  6. Bermejo-Vega, J. et al. Architectures for quantum simulation showing a quantum speedup. Phys. Rev. X 8, 021010 (2018).

    Google Scholar 

  7. Terhal, B. M. & DiVincenzo, D. P. Adaptive quantum computation, constant depth quantum circuits and Arthur-Merlin games. Quantum Inf. Comput. 4, 134–145 (2004).

    MathSciNet  MATH  Google Scholar 

  8. Aaronson, S. Quantum computing, postselection, and probabilistic polynomial-time. Proc. R. Soc. A 461, 3473–3482 (2005).

  9. Boixo, S. et al. Characterizing quantum supremacy in near-term devices. Nat. Phys. 14, 595–600 (2018).

    Article  Google Scholar 

  10. Arute, F. et al. Quantum supremacy using a programmable superconducting processor. Nature 574, 505–510 (2019).

    Article  ADS  Google Scholar 

  11. Pednault, E. et al. Breaking the 49-qubit barrier in the simulation of quantum circuits. Preprint at https://arxiv.org/abs/1710.05867 (2017).

  12. Boixo, S., Isakov, S. V., Smelyanskiy, V. N. & Neven, H. Simulation of low-depth quantum circuits as complex undirected graphical models. Preprint at https://arxiv.org/abs/1712.05384 (2017).

  13. Li, R. et al. Quantum supremacy circuit simulation on Sunway TaihuLight. Preprint at https://arxiv.org/abs/1804.04797 (2018).

  14. Chen, J. et al. Classical simulation of intermediate-size quantum circuits. Preprint at https://arxiv.org/abs/1805.01450 (2018).

  15. Bravyi, S. et al. Simulation of quantum circuits by low-rank stabilizer decompositions. Quantum 3, 181 (2019).

    Article  Google Scholar 

  16. Pednault, E. et al. Leveraging secondary storage to simulate deep 54-qubit Sycamore circuits. Preprint at https://arxiv.org/abs/1910.09534 (2019).

  17. Bravyi, S., Gosset, D. & Koenig, R. Quantum advantage with shallow circuits. Science 362, 308–311 (2018).

    Article  ADS  MathSciNet  Google Scholar 

  18. Coudron, M., Stark, J. & Vidick, T. Trading locality for time: certifiable randomness from low-depth circuits. Preprint at https://arxiv.org/abs/1810.04233 (2018).

  19. Gall, F. L. Average-case quantum advantage with shallow circuits. In Proc. 34th Computational Complexity Conference (CCC’19) (Ed. Shpilka, A.) 21:1–21:20 (Dagstuhl Publishing, 2019).

  20. Watts, A. B., Kothari, R., Schaeffer, L. & Tal, A. Exponential separation between shallow quantum circuits and unbounded fan-in shallow classical circuits. In Proc. 51st Annual ACM SIGACT Symposium on Theory of Computing—STOC’19 515–526 (Association for Computing Machinery, 2019).

  21. Peres, A. Incompatible results of quantum measurements. Phys. Lett. A 151, 107–108 (1990).

    Article  ADS  MathSciNet  Google Scholar 

  22. Mermin, N. D. Simple unified form for the major no-hidden-variables theorems. Phys. Rev. Lett. 65, 3373–3376 (1990).

    Article  ADS  MathSciNet  Google Scholar 

  23. Gottesman, D. Fault-tolerant quantum computation with constant overhead. Preprint at https://arxiv.org/abs/1310.2984 (2013).

  24. Fawzi, O., Grospellier, A. & Leverrier, A. Constant overhead quantum fault-tolerance with quantum expander codes. In Proc. 59th IEEE Annual Symposium on Foundations of Computer Science (FOCS’18) 743–754 (IEEE, 2018).

  25. Bravyi, S. & Kitaev, A. Quantum codes on a lattice with boundary. Preprint at https://arxiv.org/abs/quant-ph/9811052 (1998).

  26. Dennis, E., Kitaev, A., Landahl, A. & Preskill, J. Topological quantum memory. J. Math. Phys. 43, 4452–4505 (2002).

    Article  ADS  MathSciNet  Google Scholar 

  27. Fowler, A. G., Stephens, A. M. & Groszkowski, P. High-threshold universal quantum computation on the surface code. Phys. Rev. A 80, 052312 (2009).

    Article  ADS  Google Scholar 

  28. Bombín, H. Single-shot fault-tolerant quantum error correction. Phys. Rev. X 5, 031043 (2015).

    Google Scholar 

  29. Raussendorf, R., Bravyi, S. & Harrington, J. Long-range quantum entanglement in noisy cluster states. Phys. Rev. A 71, 062313 (2005).

    Article  ADS  Google Scholar 

  30. Moussa, J. E. Transversal Clifford gates on folded surface codes. Phys. Rev. A 94, 042316 (2016).

    Article  ADS  Google Scholar 

  31. Calderbank, A. R. & Shor, P. W. Good quantum error-correcting codes exist. Phys. Rev. A 54, 1098–1105 (1996).

    Article  ADS  Google Scholar 

  32. Steane, A. Multiple-particle interference and quantum error correction. Proc. R. Soc. Lond. A 452, 2551–2577 (1996).

    Article  ADS  MathSciNet  Google Scholar 

  33. Bravyi, S., Hastings, M. B. & Verstraete, F. Lieb-Robinson bounds and the generation of correlations and topological quantum order. Phys. Rev. Lett. 97, 050401 (2006).

    Article  ADS  Google Scholar 

  34. Eldar, L. & Harrow, A. W. Local Hamiltonians whose ground states are hard to approximate. In Proc. 58th IEEE Annual Symposium on Foundations of Computer Science (FOCS’17) 427–438 (IEEE, 2017).

  35. Aharonov, D. & Touati, Y. Quantum circuit depth lower bounds for homological codes. Preprint at https://arxiv.org/abs/1810.03912 (2018).

  36. Raussendorf, R., Bravyi, S. & Harrington, J. Long-range quantum entanglement in noisy cluster states. Phys. Rev. A 71, 062313 (2005).

    Article  ADS  Google Scholar 

  37. Aaronson, S. & Chen, L. Complexity-theoretic foundations of quantum supremacy experiments. In Proc. 32nd Computational Complexity Conference (CCC ’17) (Ed. O’Donnell, R.) 22:1–22:67 (Dagstuhl Publishing, 2017).

  38. Bouland, A., Fefferman, B., Nirkhe, C. & Vazirani, U. Quantum supremacy and the complexity of random circuit sampling. In Proc. 10th Innovations in Theoretical Computer Science (ITCS’19) 15:1–15:2 (2019).

  39. Movassagh, R. Cayley path and quantum computational supremacy: a proof of average-case #P-hardness of Random Circuit Sampling with quantified robustness. Preprint at https://arxiv.org/abs/1909.06210 (2019).

  40. Aaronson, S. & Gunn, S. On the classical hardness of spoofing linear cross-entropy benchmarking. Preprint at https://arxiv.org/abs/1910.12085 (2019).

  41. Bravyi, S. & Kitaev, A. Universal quantum computation with ideal Clifford gates and noisy ancillas. Phys. Rev. A 71, 022316 (2005).

    Article  ADS  MathSciNet  Google Scholar 

  42. O’Gorman, J. & Campbell, E. T. Quantum computation with realistic magic-state factories. Phys. Rev. A 95, 032338 (2017).

    Article  ADS  Google Scholar 

  43. Litinski, D. A game of surface codes: large-scale quantum computing with lattice surgery. Quantum 3, 128 (2019).

    Article  Google Scholar 

  44. Napp, J. et al. Efficient classical simulation of random shallow 2D quantum circuits. Preprint at https://arxiv.org/abs/2001.00021 (2019).

  45. Kahanamoku-Meyer, G. D. Forging quantum data: classically defeating an IQP-based quantum test. Preprint at https://arxiv.org/abs/1912.05547 (2019).

Download references

Acknowledgements

S.B. acknowledges support from the IBM Research Frontiers Institute and funding from the MIT-IBM Watson AI Lab under the project Machine Learning in Hilbert Space. R.K. acknowledges support by the Technical University of Munich—Institute of Advanced Study, funded by the German Excellence Initiative and the European Union Seventh Framework Programme under grant agreement no. 291763, by the DFG Cluster of Excellence 2111 (Munich Center for Quantum Science and Technology) and by the German Federal Ministry of Education through the funding programme Photonics Research Germany, contract no. 13N14776 (QCDA-QuantERA). D.G. acknowledges the support of the Natural Sciences and Engineering Research Council of Canada (NSERC) under Discovery grant no. RGPIN-2019-04198. D.G. is a CIFAR fellow in the Quantum Information Science Program. D.G. acknowledges research funding from IBM Research. M.T. thanks the Stellenbosch Institute of Advanced Study for hosting him while part of this work was completed.

Author information

Authors and Affiliations

  1. IBM T. J. Watson Research Center, Yorktown Heights, NY, USA

    Sergey Bravyi

  2. Department of Combinatorics and Optimization & Institute for Quantum Computing, University of Waterloo, Waterloo, Ontario, Canada

    David Gosset

  3. Institute for Advanced Study & Zentrum Mathematik, Technical University of Munich, Munich, Germany

    Robert König

  4. School of Computer Science & Centre for Quantum Software and Information, University of Technology Sydney, Sydney, New South Wales, Australia

    Marco Tomamichel

  5. Department of Electrical and Computer Engineering & Centre for Quantum Technologies, National University of Singapore, Singapore, Singapore

    Marco Tomamichel

Authors
  1. Sergey Bravyi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. David Gosset
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Robert König
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Marco Tomamichel
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

All authors contributed important ideas during initial discussions and contributed equally to deriving the technical proofs and writing the paper.

Corresponding author

Correspondence to Marco Tomamichel.

Ethics declarations

Competing interests

The authors declare no competing interests.

Additional information

Peer review information Nature Physics thanks Stephen Bartlett, Sergio Boixo and Bill Fefferman for their contribution to the peer review of this work.

Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Supplementary information

Supplementary Information

Proofs of all the results presented in the main text.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Bravyi, S., Gosset, D., König, R. et al. Quantum advantage with noisy shallow circuits. Nat. Phys. 16, 1040–1045 (2020). https://doi.org/10.1038/s41567-020-0948-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/s41567-020-0948-z

This article is cited by

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing