Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

Origin of strong-field-induced low-order harmonic generation in amorphous quartz

Nature Physics volume 16, pages 1035–1039 (2020)Cite this article

Subjects

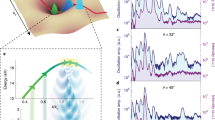
Abstract

Kerr-type nonlinearities form the basis for our physical understanding of nonlinear optical phenomena in condensed matter, such as self-focusing, solitary waves and wave mixing1,2,3. In strong fields, they are complemented by higher-order nonlinearities that enable high-harmonic generation, which is currently understood as the interplay of light-driven intraband charge dynamics and interband recombination4,5,6. Remarkably, the nonlinear response emerging from the subcycle injection dynamics of electrons into the conduction band, that is from ionization, has been almost completely overlooked in solids and only partially considered in the gas phase7,8,9,10. Here, we reveal this strong-field-induced nonlinearity in a-SiO2 as a typical wide-bandgap dielectric by means of time-resolved, low-order wave-mixing experiments, and show that, close to the material damage threshold, the so far unexplored injection current provides the leading contribution. The sensitivity of the harmonic emission to the subcycle ionization dynamics offers an original approach to characterize the evolution of laser-induced plasma formation in optical microprocessing.

This is a preview of subscription content, access via your institution

Relevant articles

Open Access articles citing this article.

Access options

Rent or buy this article

Get just this article for as long as you need it

$39.95

Learn more

Prices may be subject to local taxes which are calculated during checkout

Fig. 1: Schematic description of harmonic generation in solids.
Fig. 2: Time-resolved measurement of low-order two-colour harmonics.
Fig. 3: Experiments in c-SiO2.
Fig. 4: Characterization of m underlying the harmonic emission (for n = 1) as a function of the pump intensity in a-SiO2.

Data availability

Source data are provided with this paper. All other data that support the plots within this paper and other findings of this study are available from the corresponding author upon reasonable request.

References

  1. Boyd, R. W. Nonlinear Optics (Academic, 2002).

  2. Shen, Y.-R. The Principles of Nonlinear Optics (Wiley-Interscience, 1984, 2002).

  3. Agrawal, G. (ed.) Nonlinear Fiber Optics (Academic, 2013).

  4. Vampa, G. et al. All-optical reconstruction of crystal band structure. Phys. Rev. Lett. 115, 193603 (2015).

    Article  ADS  Google Scholar 

  5. Schubert, O. et al. Sub-cycle control of terahertz high-harmonic generation by dynamical Bloch oscillations. Nat. Photon. 8, 119–123 (2014).

    Article  ADS  Google Scholar 

  6. Ghimire, S. et al. Observation of high-order harmonic generation in a bulk crystal. Nat. Phys. 7, 138–141 (2011).

    Article  Google Scholar 

  7. Mitrofanov, A. V. et al. Optical detection of attosecond ionization induced by a few-cycle laser field in a transparent dielectric material. Phys. Rev. Lett. 106, 147401 (2011).

    Article  ADS  Google Scholar 

  8. Brunel, F. Harmonic generation due to plasma effects in a gas undergoing multiphoton ionization in the high-intensity limit. J. Opt. Soc. Am. B 7, 521–526 (1990).

    Article  ADS  Google Scholar 

  9. Siders, C. W., Rodriguez, G., Siders, J. L. W., Omenetto, F. G. & Taylor, A. J. Measurement of ultrafast ionization dynamics of gases by multipulse interferometric frequency-resolved optical gating. Phys. Rev. Lett. 87, 263002 (2001).

    Article  ADS  Google Scholar 

  10. Verhoef, A. J. et al. Optical detection of tunneling ionization. Phys. Rev. Lett. 104, 163904 (2010).

    Article  ADS  Google Scholar 

  11. Sommer, A. et al. Attosecond nonlinear polarization and light-matter energy transfer in solids. Nature 534, 86–90 (2016).

    Article  ADS  Google Scholar 

  12. Krausz, F. & Ivanov, M. Attosecond physics. Rev. Mod. Phys. 81, 163–234 (2009).

    Article  ADS  Google Scholar 

  13. Vampa, G. et al. Linking high harmonics from gases and solids. Nature 522, 462–464 (2015).

    Article  ADS  Google Scholar 

  14. Wang, Z. et al. The roles of photo-carrier doping and driving wavelength in high harmonic generation from a semiconductor. Nat. Commun. 8, 1686 (2017).

    Article  ADS  Google Scholar 

  15. Corkum, P. B. Plasma perspective on strong field multiphoton ionization. Phys. Rev. Lett. 71, 1994–1997 (1993).

    Article  ADS  Google Scholar 

  16. Lewenstein, M., Balcou, P., Ivanov, M. Y., L’Huillier, A. & Corkum, P. B. Theory of high-harmonic generation by low-frequency laser fields. Phys. Rev. A 49, 2117–2132 (1994).

    Article  ADS  Google Scholar 

  17. Luu, T. T. et al. Extreme ultraviolet high-harmonic spectroscopy of solids. Nature 521, 498–502 (2015).

    Article  ADS  Google Scholar 

  18. Vampa, G. & Brabec, T. Merge of high harmonic generation from gases and solids and its implications for attosecond science. J. Phys. B 50, 083001 (2017).

    Article  ADS  Google Scholar 

  19. Lanin, A. A., Stepanov, E. A., Fedotov, A. B. & Zheltikov, A. M. Mapping the electron band structure by intraband high-harmonic generation in solids. Optica 4, 516–519 (2017).

    Article  ADS  Google Scholar 

  20. Jiang, S. et al. Crystal symmetry and polarization of high-order harmonics in ZnO. J. Phys. B 52, 225601 (2019).

    Article  ADS  Google Scholar 

  21. Vampa, G. et al. Theoretical analysis of high-harmonic generation in solids. Phys. Rev. Lett. 113, 073901 (2014).

    Article  ADS  Google Scholar 

  22. Otobe, T. Analytical formulation for modulation of time-resolved dynamical Franz-Keldysh effect by electron excitation in dielectrics. Phys. Rev. B 96, 235115 (2017).

    Article  ADS  Google Scholar 

  23. Geissler, M. et al. Light propagation in field-ionizing media: extreme nonlinear optics. Phys. Rev. Lett. 83, 2930–2933 (1999).

    Article  ADS  Google Scholar 

  24. Yabana, K., Sugiyama, T., Shinohara, Y., Otobe, T. & Bertsch, G. F. Time-dependent density functional theory for strong electromagnetic fields in crystalline solids. Phys. Rev. B 85, 045134 (2012).

    Article  ADS  Google Scholar 

  25. Sudrie, L. et al. Femtosecond laser-induced damage and filamentary propagation in fused silica. Phys. Rev. Lett. 89, 186601 (2002).

    Article  ADS  Google Scholar 

  26. Quéré, F. et al. Coherent wake emission of high-order harmonics from overdense plasmas. Phys. Rev. Lett. 96, 125004 (2006).

    Article  ADS  Google Scholar 

  27. You, Y. S. et al. High-harmonic generation in amorphous solids. Nat. Commun. 8, 724 (2017).

    Article  ADS  Google Scholar 

  28. You, Y. S., Reis, D. A. & Ghimire, S. Anisotropic high-harmonic generation in bulk crystals. Nat. Phys. 13, 345–349 (2016).

    Article  Google Scholar 

  29. Ammosov, M. V., Delone, N. B. & Krainov, V. P. Tunnel ionization of complex atoms and of atomic ions in an alternating electromagnetic field. Sov. Phys. JETP 64, 1191–1194 (1986).

    Google Scholar 

  30. McDonald, C. R., Vampa, G., Corkum, P. B. & Brabec, T. Intense-laser solid state physics: unraveling the difference between semiconductors and dielectrics. Phys. Rev. Lett. 118, 173601 (2017).

    Article  ADS  Google Scholar 

  31. Audebert, P. et al. Space-time observation of an electron gas in SiO2. Phys. Rev. Lett. 73, 1990–1993 (1994).

    Article  ADS  Google Scholar 

Download references

Acknowledgements

We wish to thank M. Jupé (Laser Zentrum Hannover) for measuring the bandgap of the samples used in this study. A.M.-B. acknowledges financial support from the Deutsche Forschungsgemeinschaft (IDs DFG ME4427/1-1 and DFG ME4427/1-2). T.F. acknowledges financial support from the Deutsche Forschungsgemeinschaft via a Heisenberg Grant (ID 398382624) and via SPP1840 (ID 281272685), from the Bundesministerium für Bildung und Forschung (BMBF, ID 05K16HRB) and from the European Social Fund (ID ESF/14-BM-A55-0007/19) and the Ministry of Education, Science and Culture of Mecklenburg-Vorpommern, Germany, via the project ‘NEISS’. Computing time was provided by the North German Supercomputing Alliance (HLRN, ID mvp00013).

Author information

Author notes

  1. These authors contributed equally: P. Jürgens, B. Liewehr, B. Kruse.

Authors and Affiliations

  1. Max-Born-Institute for Nonlinear Optics and Short Pulse Spectroscopy, Berlin, Germany

    P. Jürgens, D. Engel, A. Husakou, T. Witting, M. Ivanov, M. J. J. Vrakking, T. Fennel & A. Mermillod-Blondin

  2. Institute for Physics, University of Rostock, Rostock, Germany

    B. Liewehr, B. Kruse, C. Peltz & T. Fennel

Authors
  1. P. Jürgens
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. B. Liewehr
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. B. Kruse
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. C. Peltz
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. D. Engel
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. A. Husakou
    View author publications

    You can also search for this author in PubMed Google Scholar

  7. T. Witting
    View author publications

    You can also search for this author in PubMed Google Scholar

  8. M. Ivanov
    View author publications

    You can also search for this author in PubMed Google Scholar

  9. M. J. J. Vrakking
    View author publications

    You can also search for this author in PubMed Google Scholar

  10. T. Fennel
    View author publications

    You can also search for this author in PubMed Google Scholar

  11. A. Mermillod-Blondin
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

A.M.-B., P.J. and M.J.J.V. conceived, performed and analysed the experiment, with the help of D.E., T.W. and B.L. B.K., C.P., A.H., M.I. and T.F. developed the theoretical model. A.M.-B. and T.F. wrote the manuscript, with input from all authors.

Corresponding authors

Correspondence to T. Fennel or A. Mermillod-Blondin.

Ethics declarations

Competing interests

The authors declare no competing interests.

Additional information

Peer review information Nature Physics thanks Ruifeng Lu, Alexander Pukhov and the other, anonymous, reviewer(s) for their contribution to the peer review of this work.

Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Supplementary information

Extended Data Fig. 1 Schematic experimental setup.

A strong mid-IR pump laser pulse is temporally and spatially overlapped with a NIR probe laser pulse in the volume of a SiO2 sample. After recollimation by a CaF2 lens (L1) the signals emerging from the sample are separately analyzed in the two parts of the detection unit. L1 - L4: Lenses, BS: Beam splitters, DM: Dielectric mirror (high reflectivity at 0.8 μm), PD: Photodiode, BD: Beam dump, P1, P2: prisms.

Extended Data Fig. 2 Probe intensity scans in a-SiO2.

Harmonic yield of the first four harmonic orders depending on the intensity of the probe laser pulse together with linear fits to the experimental results. The pump and probe beams have parallel polarizations. The linear relationship between harmonic yield and probe intensity indicates that only one probe photon is involved in the nonlinear wave mixing. The probe intensity used in the experiments is Iprobe = 0.015 TW/cm2.

Extended Data Fig. 3 Plasma diagnostics in a-SiO2.

Loss of probe transmission in the irradiated region for a pump intensity of ≈12 TW/cm2. The first maximum of the signal is attributed to probe depletion due to reversible two-beam coupling processes during the pump–probe overlap (symbolized as an orange region). These contributions can be safely excluded when the pump beam has propagated through the focal region, that is for τ > 200 fs. The remaining signal is then only due to absorption in the undercritical electron–hole plasma. The probe absorption decays with a characteristic time on the order of ≈150 fs, corresponding to the carrier trapping constant usually reported for fused silica31.

Extended Data Fig. 4 Determination of the pump–probe overlap.

a, Variation of the central probe wavelength as a function of the number of pump–probe delay increments (one increment represents a delay of ≈6.7 fs). The shift of the central wavelength is due to cross-phase modulation and shows a maximum when pump and probe overlap in the focal region. b, projection of the n = 1 harmonic on the delay axis. The grey dotted curve was obtained by direct integration of the data shown in a.

Extended Data Fig. 5 Effective non-linearity as function of the bandgap.

Orange dashed line: the sum of Kerr-type and Brunel nonlinearities are considered. Orange solid line: the contribution of the injection is also taken into account. The region shaded in violet shows the effective order of nonlinearity obtained experimentally for an intensity of 12 TW/cm2 when measurement uncertainties are included. The squares correspond to the bandgap values (that is 7.5, 7.7, and 8.0 eV) chosen to illustrate the robustness of our model versus bandgap variations in Fig. 4 of the main manuscript.

Supplementary information

Supplementary Information

Supplementary Figs. 1–4 and discussion.

Source data

Source Data Fig. 2

Raw experimental data for Fig. 2b.

Source Data Fig. 3

Raw experimental data for Fig. 3.

Source Data Fig. 4

Raw experimental data, statistical data (error bars) and numerical simulation results for Fig. 4.

Rights and permissions

Reprints and Permissions

About this article

Verify currency and authenticity via CrossMark

Cite this article

Jürgens, P., Liewehr, B., Kruse, B. et al. Origin of strong-field-induced low-order harmonic generation in amorphous quartz. Nat. Phys. 16, 1035–1039 (2020). https://doi.org/10.1038/s41567-020-0943-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/s41567-020-0943-4

This article is cited by

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing