Abstract
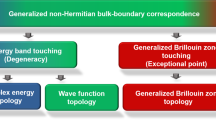
Bulk–boundary correspondence, a guiding principle in topological matter, relates robust edge states to bulk topological invariants. Its validity, however, has so far been established only in closed systems. Recent theoretical studies indicate that this principle requires fundamental revisions for a wide range of open systems with effective non-Hermitian Hamiltonians. Therein, the intriguing localization of nominal bulk states at boundaries, known as the non-Hermitian skin effect, suggests a non-Bloch band theory in which non-Bloch topological invariants are defined in generalized Brillouin zones, leading to a general bulk–boundary correspondence beyond the conventional framework. Here, we experimentally observe this fundamental non-Hermitian bulk–boundary correspondence in discrete-time non-unitary quantum-walk dynamics of single photons. We demonstrate pronounced photon localizations near boundaries even in the absence of topological edge states, thus confirming the non-Hermitian skin effect. Facilitated by our experimental scheme of edge-state reconstruction, we directly measure topological edge states, which are in excellent agreement with the non-Bloch topological invariants. Our work unequivocally establishes the non-Hermitian bulk–boundary correspondence as a general principle underlying non-Hermitian topological systems and paves the way for a complete understanding of topological matter in open systems.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout





Similar content being viewed by others
References
Hasan, M. Z. & Kane, C. L. Colloquium: topological insulators. Rev. Mod. Phys. 82, 3045–3067 (2010).
Qi, X. L. & Zhang, S. C. Topological insulators and superconductors. Rev. Mod. Phys. 83, 1057–1110 (2011).
Lee, T. E. Anomalous edge state in a non-Hermitian lattice. Phys. Rev. Lett. 116, 133903 (2016).
Yao, S. & Wang, Z. Edge states and topological invariants of non-Hermitian systems. Phys. Rev. Lett. 121, 086803 (2018).
Yao, S., Song, F. & Wang, Z. Non-Hermitian Chern bands. Phys. Rev. Lett. 121, 136802 (2018).
Kunst, F. K., Edvardsson, E., Budich, J. C. & Bergholtz, E. J. Biorthogonal bulk–boundary correspondence in non-Hermitian systems. Phys. Rev. Lett. 121, 026808 (2018).
Yokomizo, K. & Murakami, S. Non-Bloch band theory of non-Hermitian systems. Phys. Rev. Lett. 123, 066404 (2019).
Alvarez, V. M., Vargas, J. B., Berdakin, M. & Torres, L. F. Topological states of non-Hermitian systems. Eur. Phys. J. Spec. Top. 227, 1295–1308 (2018).
Lee, C. H. & Thomale, R. Anatomy of skin modes and topology in non-Hermitian systems. Phys. Rev. B 99, 201103(R) (2019).
Ghatak, A. & Das, T. New topological invariants in non-Hermitian systems. J. Phys. Condens. Matter 31, 263001 (2019).
Borgnia, D. S., Kruchkov, A. J. & Slager, R.-J. Non-Hermitian boundary modes. Phys. Rev. Lett. 124, 056802 (2020).
Martinez Alvarez, V. M., Barrios Vargas, J. E. & Foa Torres, L. E. F. Non-Hermitian robust edge states in one dimension: anomalous localization and eigenspace condensation at exceptional points. Phys. Rev. B 97, 121401(R) (2018).
Longhi, S. Probing non-Hermitian skin effect and non-Bloch phase transitions. Phys. Rev. Res. 1, 023013 (2019).
McDonald, A., Pereg-Barnea, T. & Clerk, A. A. Phase-dependent chiral transport and effective non-Hermitian dynamics in a bosonic Kitaev–Majorana chain. Phys. Rev. X 8, 041031 (2018).
Poli, C., Bellec, M., Kuhl, U., Mortessagne, F. & Schomerus, H. Selective enhancement of topologically induced interface states in a dielectric resonator chain. Nat. Commun. 6, 6710 (2015).
Zeuner, J. M. et al. Observation of a topological transition in the bulk of a non-Hermitian system. Phys. Rev. Lett. 115, 040402 (2015).
Xiao, L. et al. Observation of topological edge states in parity–time-symmetric quantum walks. Nat. Phys. 13, 1117–1123 (2017).
Zhan, X. et al. Detecting topological invariants in nonunitary discrete-time quantum walks. Phys. Rev. Lett. 119, 130501 (2017).
Xiao, L. et al. Higher winding number in a nonunitary photonic quantum walk. Phys. Rev. A 98, 063847 (2018).
Wang, K. et al. Simulating dynamic quantum phase transitions in photonic quantum walks. Phys. Rev. Lett. 122, 020501 (2019).
Wang, K. et al. Observation of emergent momentum–time skyrmions in parity–time-symmetric non-unitary quench dynamics. Nat. Commun. 10, 2293 (2019).
Xiao, L. et al. Observation of critical phenomena in parity–time-symmetric quantum dynamics. Phys. Rev. Lett. 123, 230401 (2019).
Weimann, S. et al. Topologically protected bound states in photonic parity–time-symmetric crystals. Nat. Mater. 16, 433–438 (2017).
Parto, M. et al. Edge-mode lasing in 1D topological active arrays. Phys. Rev. Lett. 120, 113901 (2018).
Zhou, H. et al. Observation of bulk Fermi arc and polarization half charge from paired exceptional points. Science 359, 1009–1012 (2018).
Ozawa, T. et al. Topological photonics. Rev. Mod. Phys. 91, 015006 (2019).
Bandres, M. A. et al. Topological insulator laser: experiments. Science 359, eaar4005 (2018).
Zhu, W. et al. Simultaneous observation of a topological edge state and exceptional point in an open and non-Hermitian acoustic system. Phys. Rev. Lett. 121, 124501 (2018).
Wu, Y. et al. Observation of parity–time symmetry breaking in a single-spin system. Science 364, 878–880 (2019).
Li, J. et al. Observation of parity–time symmetry breaking transitions in a dissipative Floquet system of ultracold atoms. Nat. Commun. 10, 855 (2019).
Shen, H., Zhen, B. & Fu, L. Topological band theory for non-Hermitian Hamiltonians. Phys. Rev. Lett. 120, 146402 (2018).
Leykam, D., Bliokh, K. Y., Huang, C., Chong, Y. D. & Nori, F. Edge modes, degeneracies, and topological numbers in non-Hermitian systems. Phys. Rev. Lett. 118, 040401 (2017).
Gong, Z. et al. Topological phases of non-Hermitian systems. Phys. Rev. X 8, 031079 (2018).
El-Ganainy, R. et al. Non-Hermitian physics and PT symmetry. Nat. Phys. 14, 11–19 (2018).
Kawabata, K., Shiozaki, K., Ueda, M. & Sato, M. Symmetry and topology in non-Hermitian physics. Phys. Rev. X 9, 041015 (2019).
Zhou, H. & Lee, J. Y. Periodic table for topological bands with non-Hermitian symmetries. Phys. Rev. B 99, 235112 (2019).
Rudner, M. S. & Levitov, L. S. Topological transition in a non-Hermitian quantum walk. Phys. Rev. Lett. 102, 065703 (2009).
Esaki, K., Sato, M., Hasebe, K. & Kohmoto, M. Edge states and topological phases in non-Hermitian systems. Phys. Rev. B 84, 205128 (2011).
Zhu, B., Lü, R. & Chen, S. PT symmetry in the non-Hermitian Su–Schrieffer–Heeger model with complex boundary potentials. Phys. Rev. A 89, 062102 (2014).
Rudner, M. S., Lindner, N. H., Berg, E. & Levin, M. Anomalous edge states and the bulk–edge correspondence for periodically driven two-dimensional systems. Phys. Rev. X 3, 031005 (2013).
Asbóth, J. K. & Obuse, H. Bulk–boundary correspondence for chiral symmetric quantum walks. Phys. Rev. B 88, 121406(R) (2013).
Deng, T. & Yi, W. Non-Bloch topological invariants in a non-Hermitian domain-wall system. Phys. Rev. B 100, 035102 (2019).
Yao, S., Yan, Z. & Wang, Z. Topological invariants of Floquet systems: general formulation, special properties, and Floquet topological defects. Phys. Rev. B 96, 195303 (2017).
Fruchart, M. Complex classes of periodically driven topological lattice systems. Phys. Rev. B 93, 115429 (2016).
Longhi, S. Non-Bloch PT symmetry breaking in non-Hermitian photonics quantum walks. Opt. Lett. 44, 5804–5807 (2019).
Helbig, T. et al. Observation of bulk boundary correspondence breakdown in topolectrical circuits. Preprint at https://arxiv.org/abs/1907.11562 (2019).
Ghatak, A., Brandenbourger, M., van Wezel, J. & Coulais, C. Observation of non-Hermitian topology and its bulk–edge correspondence. Preprint at https://arxiv.org/abs/1907.11619 (2019).
Brody, D. C. Biorthogonal quantum mechanics. J. Phys. A Math. Theor. 47, 035305 (2014).
Acknowledgements
This work has been supported by the National Natural Science Foundation of China (grant nos. 11674056, 11674189, U1930402 and 11974331) and a start-up fund from the Beijing Computational Science Research Center. W.Y. acknowledges support from the National Key Research and Development Program of China (grant nos. 2016YFA0301700 and 2017YFA0304100).
Author information
Authors and Affiliations
Contributions
L.X. performed the experiments, with contributions from K.W. and G.Z.; W.Y., T.D. and Z.W. developed the theoretical aspects and performed the theoretical analysis; P.X. designed the experiments and analysed the results; P.X., W.Y. and Z.W. wrote the paper, with input from all authors.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Peer review information Nature Physics thanks Andrea Alu and the other, anonymous, reviewer(s) for their contribution to the peer review of this work.
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Supplementary Information
Supplementary Information and Figs. 1–5.
Source data
Source Data Fig. 3
Source data for Figure 3.
Source Data Fig. 4
Source data for Figure 4.
Source Data Fig. 5
Source data for Figure 5.
Rights and permissions
About this article
Cite this article
Xiao, L., Deng, T., Wang, K. et al. Non-Hermitian bulk–boundary correspondence in quantum dynamics. Nat. Phys. 16, 761–766 (2020). https://doi.org/10.1038/s41567-020-0836-6
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41567-020-0836-6
This article is cited by
-
Topological temporally mode-locked laser
Nature Physics (2024)
-
Non-Hermitian topological phase transitions controlled by nonlinearity
Nature Physics (2024)
-
Non-reciprocal topological solitons in active metamaterials
Nature (2024)
-
Optomechanical realization of the bosonic Kitaev chain
Nature (2024)
-
Non-Hermitian non-equipartition theory for trapped particles
Nature Communications (2024)