Abstract
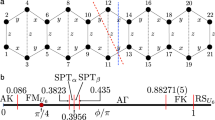
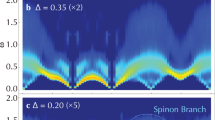
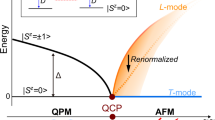
Complex bound states of magnetic excitations, known as Bethe strings, were predicted almost a century ago to exist in one-dimensional quantum magnets1. The dispersions of the string states have so far remained the subject of intense theoretical studies2,3,4,5,6,7. Here, by performing neutron scattering experiments on the one-dimensional Heisenberg–Ising antiferromagnet SrCo2V2O8 in high longitudinal magnetic fields, we reveal in detail the dispersion relations of the string states over the full Brillouin zone, as well as their magnetic field dependencies. Furthermore, the characteristic energy, the scattering intensity and linewidth of the observed string states exhibit excellent agreement with our precise Bethe–ansatz calculations. Our results establish the important role of string states in the quantum spin dynamics of one-dimensional systems, and will invoke studies of their dynamical properties in more general many-body systems.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout





Similar content being viewed by others
Data availability
The datasets for the inelastic neutron scattering experiment on the time-of-flight LET spectrometer are available from the ISIS facility, Rutherford Appleton Laboratory data portal (10.5286/ISIS.E.RB1510288). The datasets for the inelastic neutron scattering experiment on the cold triple-axis ThALES spectrometer are available from the Institute Laue–Langevin data portal (https://doi.ill.fr/10.5291/ILL-DATA.4-05-700). Additional INS data were taken on the FLEXX spectrometer at HZB, Berlin, Germany. High-field (above 15 T) INS data were taken on the HFM/EXED high magnetic field facility for neutron scattering at HZB, Berlin, Germany. The data represented in Figs. 3, 4 and 5 are available as Source Data Figs. 3, 4 and 5. All other raw and derived data used to support the findings of this study are available from the authors upon request.
Code availability
The code is available upon reasonable request from J.W.
References
Bethe, H. Zur Theorie der Metalle. I. Eigenwerte und Eigenfunktionen der linearen Atomkette. Z. Phys. 71, 205–226 (1931).
Gaudin, M. Thermodynamics of the Heisenberg–Ising ring for Δ > 1. Phys. Rev. Lett. 26, 1301–1304 (1971).
Karbach, M. & Muller, G. Introduction to the Bethe ansatz I. Comput. Phys. 11, 36 (1997).
Kitanine, N., Mailet, J. M. & Terras, V. Form factors of the XXZ Heisenberg spin-1/2 finite chain. Nucl. Phys. B 554, 647–678 (1999).
Kohno, M. Dynamically dominant excitations of string solutions in the spin-1/2 antiferromagnetic Heisenberg chain in a magnetic field. Phys. Rev. Lett. 102, 037203 (2009).
Pereira, R. G., White, S. R. & Affleck, I. Exact edge singularities and dynamical correlations in spin-1/2 chains. Phys. Rev. Lett. 100, 027206 (2008).
Takahashi, M. & Suzuki, M. One-dimensional anisotropic Heisenberg model at finite temperatures. Prog. Theor. Phys. 48, 2187–2209 (1972).
Batchelor, M. T. The Bethe ansatz after 75 years. Phys. Today 60, 36–40 (2007).
Faddeev, L. D. & Takhtajan, L. A. What is the spin of a spin wave? Phys. Lett. A 85, 375–377 (1981).
Haldane, F. D. M. General relation of correlation exponents and spectral properties of one-dimensional Fermi systems: application to the anisotropic S = 1/2 Heisenberg chain. Phys. Rev. Lett. 45, 1358–1362 (1980).
Ganahl, M., Rabel, E., Essler, F. H. L. & Evertz, H. G. Observation of complex bound states in the spin-1/2 Heisenberg XXZ chain using local quantum quenches. Phys. Rev. Lett. 108, 077206 (2012).
Caux, J. S. & Maillet, J. M. Computation of dynamical correlation functions of Heisenberg chains in a magnetic field. Phys. Rev. Lett. 95, 077201 (2005).
Wang, Z. et al. Experimental observation of Bethe strings. Nature 554, 219–223 (2018).
Bera, A. K., Lake, B., Stein, W. D. & Zander, S. Magnetic correlations of the quasi-one-dimensional half-integer spin-chain antiferromagnets Sr M 2V2O8 (M =Co, Mn). Phys. Rev. B 89, 094402 (2014).
Grenier, B. et al. Neutron diffraction investigation of the H-T phase diagram above the longitudinal incommensurate phase of BaCo2V2O8. Phys. Rev. B 92, 134416 (2015).
Faure, Q. et al. Tomonaga–Luttinger liquid spin dynamics in the quasi-one-dimensional Ising-like antiferromagnet BaCo2V2O8. Phys. Rev. Lett. 123, 027204 (2019).
Bera, A. K. et al. Spinon confinement in a quasi-one dimensional anisotropic Heisenberg magnet. Phys. Rev. B 96, 054423 (2017).
Lake, B., Tennant, D. A., Frost, C. D. & Nagler, S. E. Quantum criticality and universal scaling of a quantum antiferromagnet. Nat. Mater. 4, 329–334 (2005).
Wu, L. S. Orbital-exchange and fractional quantum number excitations in an f-electron metal, Yb2Pt2Pb. Science 352, 1206–1210 (2016).
Stone, M. B. Extended quantum critical phase in a magnetized spin-1/2 antiferromagnetic chain. Phys. Rev. Lett. 91, 037205 (2003).
Grenier, B. Longitudinal and transverse Zeeman ladders in the Ising-like chain antiferromagnet BaCo2V2O8. Phys. Rev. Lett. 114, 017201 (2015).
Coldea, R. et al. Quantum criticality in an Ising chain: experimental evidence for emergent E8 symmetry. Science 327, 177–180 (2010).
Wang, Z. Spinon confinement in the one-dimensional Ising-like antiferromagnet SrCo2V2O8. Phys. Rev. B 91, 140404 (R) (2015).
Polyakov, A. M. Quark confinement and topology of gauge theories. Nucl. Phys. B 120, 429–458 (1977).
Shelton, D. G., Nersesyan, A. A. & Tsvelik, A. M. Antiferromagnetic spin ladders: crossover between spin S = 1/2 and S = 1 chains. Phys. Rev. B 53, 8521–8532 (1996).
Lake, B. Confinement of fractional quantum number particles in a condensed-matter system. Nat. Phys. 6, 50–55 (2010).
Yang, W., Wu, J., Xu, S., Wang, Z. & Wu, C. One-dimensional quantum spin dynamics of Bethe string states. Phys. Rev. B 100, 184406 (2019).
Korepin, V. E., Bogoliubov, N. M. & Izergin, A. G. Quantum Inverse Scattering Method and Correlation Functions (Cambridge Univ. Press, 1997).
Yang, C. N. & Yang, C. P. One-dimensional chain of anisotropic spin–spin interactions. I. Proof of Bethe’s hypothesis for ground state in a finite system. Phys. Rev. 150, 321–327 (1966).
Lejay, P. et al. Crystal growth and magnetic property of MCo2V2O8 (M = Sr and Ba). J. Cryst. Growth 317, 128–131 (2011).
Skourski, Y., Kuz’min, M. D., Skokov, K. P., Andreev, A. V. & Wosnitza, J. High-field magnetization of Ho2Fe17. Phys. Rev. B 83, 214420 (2011).
Russina, M. & Mezei, F. First implementation of repetition rate multiplication in neutron spectroscopy. Nucl. Instrum. Meth. A 604, 624–631 (2009).
Nakamura, M. et al. First demonstration of novel method for inelastic neutron scattering measurement utilizing multiple incident energies. J. Phys. Soc. Jpn 78, 093002 (2009).
Arnold, O. et al. Mantid—Data analysis and visualization package for neutron scattering and μ-SR experiments. Nucl. Instrum. Meth. A 764, 156–166 (2014).
Le, M. D. et al. Gains from the upgrade of the cold neutron triple-axis spectrometer FLEXX at the BER-II reactor. Nucl. Instrum. Meth. A 729, 220–226 (2013).
Prokhnenko, O., Smeibidl, P., Stein, W.-D., Bartkowiak, M. & Stüsser, N. HFM/EXED: the high magnetic field facility for neutron scattering at BER II. J. Large Scale Res. Facilities 3, A115 (2017).
Bartkowiak, M., Stuesser, N. & Prokhnenko, O. The design of the inelastic neutron scattering mode for the extreme environment diffractometer with the 26 T high field magnet. Nucl. Instrum. Meth. A 797, 121–129 (2015).
Korepin, V. E. Calculation of norms of Bethe wave functions. Commun. Math. Phys. 86, 391–418 (1982).
Slavnov, N. A. Calculation of scalar products of wave functions and form factors in the framework of the algebraic Bethe ansatz. Theor. Math. Phys. 79, 502–508 (1989).
Caux, J. S., Hagemans, R. & Maillet, J. M. Computation of dynamical correlation functions of Heisenberg chains: the gapless anisotropic regime. J. Stat. Mech. 2005, P09003 (2005).
Acknowledgements
We thank the HFM/EXED team and P. Smeibidl, R. Wahle and S. Gerischer for their technical support during the measurements. J.W. acknowledges additional support from a Shanghai talent programme. The high-field experiments at Dresden were supported by Hochfeld Magnetlabor Dresden at HZDR, a member of the European Magnetic Field Laboratory (EMFL).
Author information
Authors and Affiliations
Contributions
A.K.B. and B.L. conceived the experiments. A.K.B., J.W. and B.L. coordinated the project. A.K.B. and A.T.M.N.I. prepared and characterized the high-quality single crystals. A.K.B., B.K. and J.M.L. performed the bulk measurements. A.K.B., B.L. and R.B. performed the LET experiments. B.L. and M.Boehm. performed ThALES experiments. B.L., M.Bartkowiak. and O.P. performed the HFM/EXED measurements. B.L. and J.X. performed the FLEXX measurements. J.W. and W.Y. carried out the Bethe–ansatz calculations. A.K.B. and B.L. analysed the experimental data. J.W. and W.Y. analysed the Bethe–ansatz calculations. The comparison between experimental and theoretical results was made by A.K.B., J.W., W.Y. and B.L. A.K.B. wrote the manuscript, with contributions from B.L., J.W., W.Y. and Z.W. All authors discussed the data and its interpretation.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Supplementary Information
Supplementary Figs. 1–6, Discussion and Table 1.
Source data
Source Data Fig. 3
Source Data for Fig. 3.
Source Data Fig. 4
Source Data for Fig. 4.
Source Data Fig. 5
Source Data for Fig. 5.
Rights and permissions
About this article
Cite this article
Bera, A.K., Wu, J., Yang, W. et al. Dispersions of many-body Bethe strings. Nat. Phys. 16, 625–630 (2020). https://doi.org/10.1038/s41567-020-0835-7
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41567-020-0835-7
This article is cited by
-
Emergent many-body composite excitations of interacting spin-1/2 trimers
Nature Communications (2022)
-
Driven quantum bits push computational limit
Nature (2022)
-
Long-lived phantom helix states in Heisenberg quantum magnets
Nature Physics (2022)
-
Multiple fermion scattering in the weakly coupled spin-chain compound YbAlO3
Nature Communications (2021)