Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

  • Article
  • Published:

Resonant phase-matching between a light wave and a free-electron wavefunction

Nature Physics volume 16, pages 1123–1131 (2020)Cite this article

Subjects

A Publisher Correction to this article was published on 22 January 2021

This article has been updated

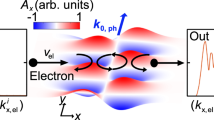
Abstract

Quantum light–matter interactions of bound electron systems have been studied extensively. By contrast, quantum interactions of free electrons with light have only become accessible in recent years, following the discovery of photon-induced near-field electron microscopy (PINEM). So far, the fundamental free electron–light interaction in all PINEM experiments has remained weak due to its localized near-field nature, which imposes an energy–momentum mismatch between electrons and light. Here, we demonstrate a strong interaction between free-electron waves and light waves, resulting from precise energy–momentum phase-matching with the extended propagating light field. By exchanging hundreds of photons with the field, each electron simultaneously accelerates and decelerates in a coherent manner. Consequently, each electron’s quantum wavefunction evolves into a quantized energy comb, spanning a bandwidth of over 1,700 eV, requiring us to extend the PINEM theory. Our observation of coherent electron phase-matching with a propagating wave is a type of inverse-Cherenkov interaction that occurs with a quantum electron wavefunction, demonstrating how the extended nature of the electron wavefunction can alter stimulated electron–light interactions.

This is a preview of subscription content, access via your institution

Access options

Buy this article

  • Purchase on Springer Link
  • Instant access to full article PDF
Buy now

Prices may be subject to local taxes which are calculated during checkout

Fig. 1: Quantum versus classical phase-matching of an electron and light.
Fig. 2: Experimental set-up.
Fig. 3: Comparison of the experimental data with both conventional PINEM theory and our extended theory.
Fig. 4: Conditions for phase-matching and theoretical analysis.
Fig. 5: Experimental results showing the coherent electron energy comb forming a plateau or energy peaks.

Similar content being viewed by others

Data availability

All data that support the plots and other findings of this study are available from the corresponding author upon reasonable request. Source data are provided with this paper.

Change history

  • 22 January 2021

    A Correction to this paper has been published: https://doi.org/10.1038/s41567-021-01178-3.

References

  1. Barwick, B., Flannigan, D. J. & Zewail, A. H. Photon-induced near-field electron microscopy. Nature 462, 902–906 (2009).

    ADS  Google Scholar 

  2. García de Abajo, F. J., Asenjo-Garcia, A. & Kociak, M. Multiphoton absorption and emission by interaction of swift electrons with evanescent light fields. Nano Lett. 10, 1859–1863 (2010).

    ADS  Google Scholar 

  3. Park, S. T., Lin, M. & Zewail, A. H. Photon-induced near-field electron microscopy (PINEM): theoretical and experimental. New J. Phys. 12, 123028 (2010).

    ADS  Google Scholar 

  4. Feist, A. et al. Quantum coherent optical phase modulation in an ultrafast transmission electron microscope. Nature 521, 200–203 (2015).

    ADS  Google Scholar 

  5. Piazza, L. et al. Simultaneous observation of the quantization and the interference pattern of a plasmonic near-field. Nat. Commun. 6, 6407 (2015).

    ADS  Google Scholar 

  6. Vanacore, G. M. et al. Ultrafast generation and control of an electron vortex beam via chiral plasmonic near fields. Nat. Mater. 18, 573–579 (2019).

    ADS  Google Scholar 

  7. Wang, K. et al. Coherent interaction between free electrons and a photonic cavity. Nature 582, 50–54 (2020).

    ADS  Google Scholar 

  8. Kfir, O. et al. Controlling free electrons with optical whispering-gallery modes. Nature 582, 46–49 (2020).

    ADS  Google Scholar 

  9. Vanacore, G. M. et al. Attosecond coherent control of free-electron wave functions using semi-infinite light fields. Nat. Commun. 9, 2694 (2018).

    ADS  Google Scholar 

  10. Cherenkov, P. A. Visible emission of clean liquids by action of γ radiation. Dokl. Akad. Nauk SSSR 2, 451 (1934).

    Google Scholar 

  11. Smith, S. J. & Purcell, E. M. Visible light from localized surface charges moving across a grating. Phys. Rev. 92, 1069 (1953).

    ADS  Google Scholar 

  12. Ginzburg, V. L. & Frank, I. M. Radiation of electrons and atoms moving along the axis of a tube in a dense medium. in. Dokl. Akad. Nauk SSSR 56, 699–702 (1947).

    Google Scholar 

  13. Danos, M., Geschwind, S., Lashinsky, H. & Van Trier, A. Čerenkov effect at microwave frequencies. Phys. Rev. 92, 828–829 (1953).

    ADS  Google Scholar 

  14. Fontana, J. R. & Pantell, R. H. A high‐energy, laser accelerator for electrons using the inverse Cherenkov effect. J. Appl. Phys. 54, 4285–4288 (1983).

    ADS  Google Scholar 

  15. Piestrup, M. A. An analysis of oblique angle stimulated Cherenkov radiation with some experimental results. IEEE J. Quantum Electron. 19, 1827–1834 (1983).

    ADS  Google Scholar 

  16. Mizuno, K., Pae, J., Nozokido, T. & Furuya, K. Experimental evidence of the inverse Smith–Purcell effect. Nature 328, 45–47 (1987).

    ADS  Google Scholar 

  17. Kimura, W. D. et al. Laser acceleration of relativistic electrons using the inverse Cherenkov effect. Phys. Rev. Lett. 74, 546–549 (1995).

    ADS  Google Scholar 

  18. García De Abajo, F. J., Rivacoba, A., Zabala, N. & Yamamoto, N. Boundary effects in Cherenkov radiation. Phys. Rev. B 69, 155420 (2004).

    ADS  Google Scholar 

  19. Schächter, L. Beam-Wave Interaction in Periodic and Quasi-Periodic Structures (Springer, 1997).

  20. Friedman, A., Gover, A., Kurizki, G., Ruschin, S. & Yariv, A. Spontaneous and stimulated emission from quasifree electrons. Rev. Mod. Phys. 60, 471–535 (1988).

    ADS  Google Scholar 

  21. Mizrahi, A. & Schächter, L. Optical Bragg accelerators. Phys. Rev. E 70, 016505 (2004).

    ADS  Google Scholar 

  22. Kaminer, I. et al. Spectrally and spatially resolved Smith–Purcell radiation in plasmonic crystals with short-range disorder. Phys. Rev. X 7, 011003 (2017).

    Google Scholar 

  23. Shaffer, T. M., Pratt, E. C. & Grimm, J. Utilizing the power of Cerenkov light with nanotechnology. Nat. Nanotechnol. 12, 106–117 (2017).

    ADS  Google Scholar 

  24. Galbraith, W. & Jelley, J. V. Light pulses from the night sky associated with cosmic rays. Nature 171, 349–350 (1953).

    ADS  Google Scholar 

  25. Ypsilantis, T. & Seguinot, J. Theory of ring imaging Cherenkov counters. Nucl. Inst. Methods Phys. Res. A 343, 30–51 (1994).

    ADS  Google Scholar 

  26. Lin, X. et al. Controlling Cherenkov angles with resonance transition radiation. Nat. Phys. 14, 816–821 (2018).

    Google Scholar 

  27. Ruggiero, A., Holland, J. P., Lewis, J. S. & Grimm, J. Cerenkov luminescence imaging of medical isotopes. J. Nucl. Med. 51, 1123–1130 (2010).

    Google Scholar 

  28. Vijayraghavan, K. et al. Broadly tunable terahertz generation in mid-infrared quantum cascade lasers. Nat. Commun. 4, 2021 (2013).

    ADS  Google Scholar 

  29. Brasch, V. et al. Photonic chip-based optical frequency comb using soliton Cherenkov radiation. Science 351, 357–360 (2016).

    ADS  MathSciNet  MATH  Google Scholar 

  30. Kozák, M. et al. Acceleration of sub-relativistic electrons with an evanescent optical wave at a planar interface. Opt. Express 25, 19195–19204 (2017).

    ADS  Google Scholar 

  31. Gover, A. et al. Superradiant and stimulated-superradiant emission of bunched electron beams. Rev. Mod. Phys. 91, 035003 (2019).

    ADS  MathSciNet  Google Scholar 

  32. Edighoffer, J. A., Kimura, W. D., Pantell, R. H., Piestrup, M. A. & Wang, D. Y. Observation of inverse Cherenkov interaction between free electrons and laser light. Phys. Rev. A 23, 1848–1854 (1981).

    ADS  Google Scholar 

  33. Zembrod, A., Puell, H. & Giordmaine, J. A. Surface radiation from non-linear optical polarisation. Opto-Electron. 1, 64–66 (1969).

    Google Scholar 

  34. Luo, C., Ibanescu, M., Johnson, S. G. & Joannopoulos, J. D. Cerenkov radiation in photonic crystals. Science 299, 368–371 (2003).

    ADS  Google Scholar 

  35. García de Abajo, F. J. et al. Cherenkov effect as a probe of photonic nanostructures. Phys. Rev. Lett. 91, 143902 (2003).

    ADS  Google Scholar 

  36. Adamo, G. et al. Light well: a tunable free-electron light source on a chip. Phys. Rev. Lett. 103, 113901 (2009).

    ADS  Google Scholar 

  37. Genevet, P. et al. Controlled steering of Cherenkov surface plasmon wakes with a one-dimensional metamaterial. Nat. Nanotechnol. 10, 804–809 (2015).

    ADS  Google Scholar 

  38. Andersen, T. I. et al. Electron–phonon instability in graphene revealed by global and local noise probes. Science 364, 154–157 (2019).

    ADS  Google Scholar 

  39. Ginzburg, V. L. Quantum theory of radiation of electron uniformly moving in medium. Zh. Eksp. Teor. Fiz. 10, 589–600 (1940).

    Google Scholar 

  40. Sokolov, A. Quantum theory of Cherenkov effect. Dokl. Akad. Nauk SSSR 28, 415–417 (1940).

    Google Scholar 

  41. Kaminer, I. et al. Quantum Čerenkov radiation: spectral cutoffs and the role of spin and orbital angular momentum. Phys. Rev. X 6, 011006 (2016).

    Google Scholar 

  42. Tsesses, S., Bartal, G. & Kaminer, I. Light generation via quantum interaction of electrons with periodic nanostructures. Phys. Rev. A 95, 013832 (2017).

    ADS  Google Scholar 

  43. Roques-Carmes, C., Rivera, N., Joannopoulos, J. D., Soljačić, M. & Kaminer, I. Nonperturbative quantum electrodynamics in the Cherenkov effect. Phys. Rev. X 8, 041013 (2018).

    Google Scholar 

  44. Talebi, N. Schrödinger electrons interacting with optical gratings: quantum mechanical study of the inverse Smith–Purcell effect. New J. Phys. 18, 123006 (2016).

    ADS  Google Scholar 

  45. Gover, A. & Pan, Y. Dimension-dependent stimulated radiative interaction of a single electron quantum wavepacket. Phys. Lett. A 382, 1550–1555 (2018).

    ADS  MathSciNet  MATH  Google Scholar 

  46. Nehemia, S. et al. Observation of the stimulated quantum Cherenkov effect. Preprint at https://arxiv.org/pdf/1909.00757.pdf (2019).

  47. Talebi, N. Interaction of electron beams with optical nanostructures and metamaterials: from coherent photon sources towards shaping the wave function. J. Opt. 19, 103001 (2017).

    ADS  Google Scholar 

  48. Rivera, N., Wong, L. J., Joannopoulos, J. D., Soljačić, M. & Kaminer, I. Light emission based on nanophotonic vacuum forces. Nat. Phys. 15, 1284–1289 (2019).

    Google Scholar 

  49. Polman, A., Kociak, M. & García de Abajo, F. J. Electron-beam spectroscopy for nanophotonics. Nat. Mater. 18, 1158–1171 (2019).

    ADS  Google Scholar 

  50. Tsarev, M. V. & Baum, P. Characterization of non-relativistic attosecond electron pulses by transition radiation from tilted surfaces. New J. Phys. 20, 033002 (2018).

    ADS  Google Scholar 

  51. Baum, P. Quantum dynamics of attosecond electron pulse compression. J. Appl. Phys. 122, 223105 (2017).

    ADS  Google Scholar 

  52. Pan, Y., Zhang, B. & Gover, A. Anomalous photon-induced near-field electron microscopy. Phys. Rev. Lett. 122, 183204 (2019).

    ADS  Google Scholar 

  53. Peralta, E. A. et al. Demonstration of electron acceleration in a laser-driven dielectric microstructure. Nature 503, 91–94 (2013).

    ADS  Google Scholar 

  54. England, R. J. et al. Dielectric laser accelerators. Rev. Mod. Phys. 86, 1337–1389 (2014).

    ADS  Google Scholar 

  55. Keller, O. Quantum Theory of Near-Field Electrodynamics (Springer, 2011).

  56. Linhart, J. G. Čerenkov radiation of electrons moving parallel to a dielectric boundary. J. Appl. Phys. 26, 527–533 (1955).

    ADS  MATH  Google Scholar 

  57. Liu, H., Baskin, J. S. & Zewail, A. H. Infrared PINEM developed by diffraction in 4D UEM. Proc. Natl Acad. Sci. USA 113, 2041–2046 (2016).

    ADS  Google Scholar 

  58. Morimoto, Y. & Baum, P. Diffraction and microscopy with attosecond electron pulse trains. Nat. Phys. 14, 252–256 (2018).

    Google Scholar 

  59. Das, P. et al. Stimulated electron energy loss and gain in an electron microscope without a pulsed electron gun. Ultramicroscopy 203, 44–51 (2019).

    Google Scholar 

  60. Madan, I. et al. Holographic imaging of electromagnetic fields via electron-light quantum interference. Sci. Adv. 5, 8358 (2019).

    ADS  Google Scholar 

  61. Agostini, P., Fabre, F., Mainfray, G., Petite, G. & Rahman, N. K. Free-free transitions following six-photon ionization of xenon atoms. Phys. Rev. Lett. 42, 1127–1130 (1979).

    ADS  Google Scholar 

  62. Corkum, P. B. & Krausz, F. Attosecond science. Nat. Phys. 3, 381–387 (2007).

    Google Scholar 

  63. Murdia, C. et al. Controlling light emission with electron wave interference. Preprint at https://arxiv.org/pdf/1712.04529.pdf (2017).

  64. Remez, R. et al. Observing the quantum wave nature of free electrons through spontaneous emission. Phys. Rev. Lett. 123, 060401 (2019).

    ADS  Google Scholar 

  65. Guzzinati, G. et al. Probing the symmetry of the potential of localized surface plasmon resonances with phase-shaped electron beams. Nat. Commun. 8, 14999 (2017).

    ADS  Google Scholar 

  66. Yang, Y. et al. Maximal spontaneous photon emission and energy loss from free electrons. Nat. Phys. 14, 894–899 (2018).

    Google Scholar 

  67. Baum, P. & Zewail, A. H. Attosecond electron pulses for 4D diffraction and microscopy. Proc. Natl Acad. Sci. USA 104, 18409 (2007).

    ADS  Google Scholar 

  68. Priebe, K. E. et al. Attosecond electron pulse trains and quantum state reconstruction in ultrafast transmission electron microscopy. Nat. Photon. 11, 793–797 (2017).

    ADS  Google Scholar 

  69. Kozák, M., Schönenberger, N. & Hommelhoff, P. Ponderomotive generation and detection of attosecond free-electron pulse trains. Phys. Rev. Lett. 120, 103203 (2018).

    ADS  Google Scholar 

  70. Kfir, O. Entanglements of electrons and cavity photons in the strong-coupling regime. Phys. Rev. Lett. 123, 103602 (2019).

    ADS  Google Scholar 

  71. Di Giulio, V., Kociak, M. & de Abajo, F. J. G. Probing quantum optical excitations with fast electrons. Optica 6, 1524–1534 (2019).

    ADS  Google Scholar 

Download references

Acknowledgements

We thank the IDES Company and especially S.T. Park for illuminating discussions and advice. We are also grateful to I. Goykhman for fruitful discussions. The experiments were performed on the UTEM of the I. K. AdQuanta group installed in the Electron Microscopy Center (MIKA) in the Department of Material Science and Engineering at the Technion. The research was supported by ERC starting grant NanoEP 851780 and the Israel Science Foundation grant 831/19. K.W. is partially supported by a fellowship from the Lady Davis Foundation. I.K. acknowledges the support of the Azrieli Faculty Fellowship.

Author information

Author notes

  1. These authors contributed equally: Raphael Dahan, Saar Nehemia.

Authors and Affiliations

  1. Department of Electrical Engineering, Russell Berrie Nanotechnology Institute and Solid-State Institute, Technion, Israel Institute of Technology, Haifa, Israel

    Raphael Dahan, Saar Nehemia, Michael Shentcis, Ori Reinhardt, Yuval Adiv, Xihang Shi, Orr Be’er, Morgan H. Lynch, Yaniv Kurman, Kangpeng Wang & Ido Kaminer

Authors
  1. Raphael Dahan
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Saar Nehemia
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Michael Shentcis
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Ori Reinhardt
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Yuval Adiv
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Xihang Shi
    View author publications

    You can also search for this author in PubMed Google Scholar

  7. Orr Be’er
    View author publications

    You can also search for this author in PubMed Google Scholar

  8. Morgan H. Lynch
    View author publications

    You can also search for this author in PubMed Google Scholar

  9. Yaniv Kurman
    View author publications

    You can also search for this author in PubMed Google Scholar

  10. Kangpeng Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  11. Ido Kaminer
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

R.D. achieved the grazing-angle condition in the transmission electron microscope and led the experimental work including sample preparation. S.N. and R.D. worked on the design before the experiment began. R.D., K.W., M.S., O.B., Y.A. and S.N. carried out the experiments. K.W., S.N., Y.A., R.D., O.R. and Y.K. developed the theory and analysed the results. M.H.L. and X.S. contributed to the discussion of the results. I.K. conceived the research. All authors provided substantial input to all aspects of the project and to the writing of the manuscript.

Corresponding author

Correspondence to Ido Kaminer.

Ethics declarations

Competing interests

The authors declare no competing interests.

Additional information

Peer review information Nature Physics thanks Albert Polman and the other, anonymous, reviewer(s) for their contribution to the peer review of this work.

Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Supplementary information

Supplementary Information

Supplementary Discussion and Supplementary Figs. 1–6.

Supplementary Video 1

Finite-difference time-domain simulation of the setup. The simulation assumes a right-angle glass prism with refractive index 1.513 at 700 nm, 15 μm leg size, and base angle of 45°. The laser undergoes total internal reflection inside the prism and generates an evanescent field that interacts with the electron that passes nearby.

Source data

Source Data Fig. 1

Data used to plot Fig. 1b–d. Experimental data points and theoretical data from the classical and quantum mechanical models.

Source Data Fig. 3

Data used to plot Fig. 3. Experimental data points and data from conventional as well as extended PINEM theory.

Source Data Fig. 4

Data used to plot Fig. 4b–e. Experimental and theoretical data points.

Source Data Fig 5

Data used to plot Fig. 5a,b. Experimental data points and theoretical data from the classical and quantum mechanical models.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Dahan, R., Nehemia, S., Shentcis, M. et al. Resonant phase-matching between a light wave and a free-electron wavefunction. Nat. Phys. 16, 1123–1131 (2020). https://doi.org/10.1038/s41567-020-01042-w

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/s41567-020-01042-w

This article is cited by

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing