Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

  • Letter
  • Published:

Non-Gaussian quantum states of a multimode light field

Nature Physics volume 16, pages 144–147 (2020)Cite this article

Subjects

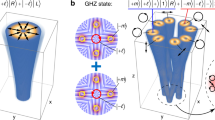
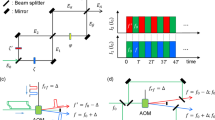
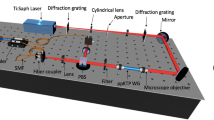
Abstract

Advanced quantum technologies require scalable and controllable quantum resources1,2. Gaussian states of multimode light, such as squeezed states and cluster states, are scalable quantum systems3,4,5, which can be generated on demand. However, non-Gaussian features are indispensable in many quantum protocols, especially to reach a quantum computational advantage6. Embodying non-Gaussianity in a multimode quantum state remains a challenge as non-Gaussian operations generally cannot maintain coherence among multiple modes. Here, we generate non-Gaussian quantum states of a multimode light field by removing a single photon in a mode-selective manner from a Gaussian state7. To highlight the potential for continuous-variable quantum technologies, we first demonstrated the capability to generate negativity of the Wigner function in a controlled mode. Subsequently, we explored the interplay between non-Gaussianity and quantum entanglement and verify a theoretical prediction8 about the propagation of non-Gaussianity along the nodes of photon-subtracted cluster states. Our results demonstrate large-scale non-Gaussianity with great flexibility along with an ensured compatibility with quantum information protocols. This range of features makes our approach ideal to explore the physics of non-Gaussian entanglement9,10 and to develop quantum protocols, which range across quantum computing11,12, entanglement distillation13 and quantum simulations14.

This is a preview of subscription content, access via your institution

Access options

Buy this article

  • Purchase on Springer Link
  • Instant access to full article PDF
Buy now

Prices may be subject to local taxes which are calculated during checkout

Fig. 1: Mode-selective photon subtraction from a multimode quantum state.
Fig. 2: Wigner function reconstructed from experimental data.
Fig. 3: Effect of photon subtraction on cluster states.

Similar content being viewed by others

Data availability

The data that support the plots within this paper and other findings of this study are available from the corresponding author upon reasonable request.

References

  1. Andersen, U. L., Neergaard-Nielsen, J. S., van Loock, P. & Furusawa, A. Hybrid discrete- and continuous-variable quantum information. Nat. Phys. 11, 713–719 (2015).

    Article  Google Scholar 

  2. Biamonte, J. et al. Quantum machine learning. Nature 549, 195–202 (2017).

    Article  ADS  Google Scholar 

  3. Yokoyama, S. et al. Ultra-large-scale continuous-variable cluster states multiplexed in the time domain. Nat. Photonics 7, 982–986 (2013).

    Article  ADS  Google Scholar 

  4. Roslund, J., Medeiros de Araújo, R., Jiang, S., Fabre, C. & Treps, N. Wavelength-multiplexed quantum networks with ultrafast frequency combs. Nat. Photonics 8, 109–112 (2014).

    Article  ADS  Google Scholar 

  5. Chen, M., Menicucci, N. C. & Pfister, O. Experimental realization of multipartite entanglement of 60 modes of a quantum optical frequency comb. Phys. Rev. Lett. 112, 120505 (2014).

    Article  ADS  Google Scholar 

  6. Mari, A. & Eisert, J. Positive Wigner functions render classical simulation of quantum computation efficient. Phys. Rev. Lett. 109, 230503 (2012).

    Article  ADS  Google Scholar 

  7. Averchenko, V., Jacquard, C., Thiel, V., Fabre, C. & Treps, N. Multimode theory of single-photon subtraction. New J. Phys. 18, 083042 (2016).

    Article  ADS  Google Scholar 

  8. Walschaers, M., Sarkar, S., Parigi, V. & Treps, N. Tailoring non-Gaussian continuous-variable graph states. Phys. Rev. Lett. 121, 220501 (2018).

    Article  ADS  Google Scholar 

  9. Valido, A. A., Levi, F. & Mintert, F. Hierarchies of multipartite entanglement for continuous-variable states. Phys. Rev. A 90, 052321 (2014).

    Article  ADS  Google Scholar 

  10. Park, K., Marek, P. & Filip, R. Conditional superpositions of Gaussian operations on different modes of light. Phys. Rev. A 91, 033814 (2015).

    Article  ADS  Google Scholar 

  11. Lloyd, S. & Braunstein, S. L. Quantum computation over continuous variables. Phys. Rev. Lett. 82, 1784–1787 (1999).

    Article  ADS  MathSciNet  Google Scholar 

  12. Menicucci, N. C. et al. Universal quantum computation with continuous-variable cluster states. Phys. Rev. Lett. 97, 110501 (2006).

    Article  ADS  Google Scholar 

  13. Eisert, J., Scheel, S. & Plenio, M. B. Distilling Gaussian states with Gaussian operations is impossible. Phys. Rev. Lett. 89, 137903 (2002).

    Article  ADS  MathSciNet  Google Scholar 

  14. Banchi, L., Fingerhuth, M., Babej, T., Ing, C. and Arrazola, J.M. Molecular docking with Gaussian boson sampling. Preprint at https://arxiv.org/abs/1902.00462 (2019).

  15. Aasi, J. et al. Enhanced sensitivity of the LIGO gravitational wave detector by using squeezed states of light. Nat. Photonics 7, 613–619 (2013).

    Article  ADS  Google Scholar 

  16. Takeda, S., Mizuta, T., Fuwa, M., Van Loock, P. & Furusawa, A. Deterministic quantum teleportation of photonic quantum bits by a hybrid technique. Nature 500, 315–318 (2013).

    Article  ADS  Google Scholar 

  17. Spekkens, R. W. Negativity and contextuality are equivalent notions of nonclassicality. Phys. Rev. Lett. 101, 020401 (2008).

    Article  ADS  MathSciNet  Google Scholar 

  18. Wenger, J., Tualle-Brouri, R. & Grangier, P. Non-Gaussian statistics from individual pulses of squeezed light. Phys. Rev. Lett. 92, 153601 (2004).

    Article  ADS  Google Scholar 

  19. Ourjoumtsev, A., Tualle-Brouri, R., Laurat, J. & Grangier, P. Generating optical Schrödinger kittens for quantum information processing. Science 312, 83–86 (2006).

    Article  ADS  Google Scholar 

  20. Ourjoumtsev, A., Ferreyrol, F., Tualle-Brouri, R. & Grangier, P. Preparation of non-local superpositions of quasi-classical light states. Nat. Phys. 5, 189–192 (2009).

    Article  Google Scholar 

  21. Sychev, D. V. et al. Enlargement of optical Schrödinger’s cat states. Nat. Photonics 11, 379–382 (2017).

    Article  ADS  Google Scholar 

  22. Serikawa, T. et al. Generation of a cat state in an optical sideband. Phys. Rev. Lett. 121, 143602 (2018).

    Article  ADS  Google Scholar 

  23. Biagi, N., Costanzo, L. S., Bellini, M. and Zavatta, A. Entangling macroscopic light states by delocalized photon addition. Preprint at https://arxiv.org/abs/1811.10466 (2018).

  24. Jeong, H. et al. Generation of hybrid entanglement of light. Nat. Photonics 8, 564–569 (2014).

    Article  ADS  Google Scholar 

  25. Morin, O. et al. Remote creation of hybrid entanglement between particle-like and wave-like optical qubits. Nat. Photonics 8, 570–574 (2014).

    Article  ADS  Google Scholar 

  26. Das, T., Prabhu, R., Sen De, A. & Sen, U. Superiority of photon subtraction to addition for entanglement in a multimode squeezed vacuum. Phys. Rev. A 93, 052313 (2016).

    Article  ADS  Google Scholar 

  27. Walschaers, M., Fabre, C., Parigi, V. & Treps, N. Entanglement and Wigner function negativity of multimode non-Gaussian states. Phys. Rev. Lett. 119, 183601 (2017).

    Article  ADS  Google Scholar 

  28. Ansari, V., Donohue, J. M., Brecht, B. & Silberhorn, C. Tailoring nonlinear processes for quantum optics with pulsed temporal-mode encodings. Optica 5, 534–550 (2018).

    Article  ADS  Google Scholar 

  29. Cai, Y. et al. Multimode entanglement in reconfigurable graph states using optical frequency combs. Nat. Commun. 8, 15645 (2017).

    Article  ADS  Google Scholar 

  30. Ra, Y.-S., Jacquard, C., Dufour, A., Fabre, C. & Treps, N. Tomography of a mode-tunable coherent single-photon subtractor. Phys. Rev. X 7, 031012 (2017).

    Google Scholar 

  31. Bogdanov, Y. I. et al. Multiphoton subtracted thermal states: description, preparation, and reconstruction. Phys. Rev. A 96, 063803 (2017).

    Article  ADS  Google Scholar 

  32. Marek, P., Provazník, J. & Filip, R. Loop-based subtraction of a single photon from a traveling beam of light. Opt. Express 26, 29837–29847 (2018).

    Article  ADS  Google Scholar 

  33. Katamadze, K. G., Avosopiants, G. V., Bogdanov, Y. I. & Kulik, S. P. How quantum is the ‘quantum vampire’ effect?: testing with thermal light. Optica 5, 723–726 (2018).

    Article  ADS  Google Scholar 

  34. Ježek, M. et al. Experimental test of the strongly nonclassical character of a noisy squeezed single-photon state. Phys. Rev. A 86, 043813 (2012).

    Article  ADS  Google Scholar 

  35. Albarelli, F., Genoni, M. G., Paris, M. G. A. & Ferraro, A. Resource theory of quantum non-Gaussianity and Wigner negativity. Phys. Rev. A 98, 052350 (2018).

    Article  ADS  Google Scholar 

  36. Plick, W. N., Arzani, F., Treps, N., Diamanti, E. & Markham, D. Violating bell inequalities with entangled optical frequency combs and multipixel homodyne detection. Phys. Rev. A 98, 062101 (2018).

    Article  ADS  Google Scholar 

  37. Neergaard-Nielsen, J., Nielsen, B., Hettich, C., Molmer, K. & Polzik, E. Generation of a superposition of odd photon number states for quantum information networks. Phys. Rev. Lett. 97, 083604 (2006).

    Article  ADS  Google Scholar 

  38. Duan, L.-M., Giedke, G., Cirac, J. I. & Zoller, P. Inseparability criterion for continuous variable systems. Phys. Rev. Lett. 84, 2722–2725 (2000).

    Article  ADS  Google Scholar 

  39. Simon, R. Peres–Horodecki separability criterion for continuous variable systems. Phys. Rev. Lett. 84, 2726–2729 (2000).

    Article  ADS  Google Scholar 

  40. Bowen, W. P., Schnabel, R., Lam, P. K. & Ralph, T. C. Experimental investigation of criteria for continuous variable entanglement. Phys. Rev. Lett. 90, 043601 (2003).

    Article  ADS  Google Scholar 

  41. van Loock, P., Weedbrook, C. & Gu, M. Building Gaussian cluster states by linear optics. Phys. Rev. A 76, 032321 (2007).

    Article  ADS  Google Scholar 

Download references

Acknowledgments

We thank Valentina Parigi for fruitful discussions. This work is supported by the French National Research Agency project COMB and the European Union Grant QCUMbER (no. 665148). N.T. is a member of the Institut Universitaire de France. Y.-S.R. acknowledges support from the European Commission through Marie Skłodowska-Curie actions (no. 708201) and the National Research Foundation of Korea funded by the Ministry of Education (NRF-2018R1A6A3A03012129) and the Ministry of Science and ICT (NRF-2019R1C1C1005196). M.W. acknowledges funding through research fellowship WA 3969/2-1 from the German Research Foundation.

Author information

Authors and Affiliations

  1. Laboratoire Kastler Brossel, Sorbonne Université, CNRS, ENS-PSL Research University, Collège de France, Paris, France

    Young-Sik Ra, Adrien Dufour, Mattia Walschaers, Clément Jacquard, Thibault Michel, Claude Fabre & Nicolas Treps

  2. Department of Physics, Korea Advanced Institute of Science and Technology (KAIST), Daejeon, Korea

    Young-Sik Ra

  3. Center for Quantum Computation and Communication Technology, Department of Quantum Science, The Australian National University, Canberra, Australian Capital Territory, Australia

    Thibault Michel

Authors
  1. Young-Sik Ra
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Adrien Dufour
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Mattia Walschaers
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Clément Jacquard
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Thibault Michel
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Claude Fabre
    View author publications

    You can also search for this author in PubMed Google Scholar

  7. Nicolas Treps
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

Y.-S.R. and A.D. conducted the experiments with help from C.J. and T.M. Y.-S.R. and M.W. analysed the data. M.W. developed the theoretical model. Y.-S.R., M.W. and N.T. wrote the manuscript with input from all the authors. C.F. and N.T. supervised the project. All the authors contributed to scientific discussions.

Corresponding author

Correspondence to Young-Sik Ra.

Ethics declarations

Competing interests

The authors declare no competing interests.

Additional information

Peer Review Information Nature Physics thanks Marco Bellini and the other, anonymous, reviewer(s) for their contribution to the peer review of this work.

Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Extended data

Extended Data Fig. 1 Wigner function reconstructed with optical loss correction.

Optical loss only by the homodyne detection (\(12.5 \%\)) has been corrected. Comparing with Fig. 2, non-Gaussian Wigner functions show reduced \({W}_{0}\). Errors noted in parentheses are one standard deviations calculated by bootstrapping.

Source data

Extended Data Fig. 2 Purities of the Wigner functions in Fig. 2(a).

For comparison, the purity of a Wigner function by the ideal photon subtraction is provided in square brackets, which agrees well with the experimental result. Low purity in a photon-subtracted mode is attributed to a non-ideal input state19. No optical loss is corrected in the calculation. Errors noted in parentheses are one standard deviations calculated by bootstrapping.

Extended Data Fig. 3 Effect of mode mismatch between photon subtraction and measurement.

When a single photon is subtracted in \({{\rm{HG}}}_{0}-i{{\rm{HG}}}_{1}\), a Wigner function (without optical loss correction) is obtained in a measurement mode having (a) full match (\({{\rm{HG}}}_{0}-i{{\rm{HG}}}_{1}\)), (b) partial match (\(i{{\rm{HG}}}_{1}\)), and (c) no match (\({{\rm{HG}}}_{0}+i{{\rm{HG}}}_{1}\)). Errors noted in parentheses are one standard deviations calculated by bootstrapping.

Source data

Extended Data Fig. 4 Experimental covariance matrix.

(a) is for \(x\) quadratures, seen from above, and (b) is for \(p\) quadratures, seen from below. Mode indexes are \({{\rm{HG}}}_{0}\), \(i{{\rm{HG}}}_{1}\), \({{\rm{HG}}}_{2}\), and \(i{{\rm{HG}}}_{3}\), where \(i\) is added for the odd-index HG modes to have \(p\)-squeezed vacua in all modes. For clarity, the vacuum noise (corresponding to the identity matrix) is subtracted from the covariance matrix. In the covariance matrix, variances of (\(x\), \(p\)) quadratures are (\(2.8{\rm{dB}}\), \(-1.8{\rm{dB}}\)) in mode 0, (\(2.1{\rm{dB}}\), \(-1.6{\rm{dB}}\)) in mode 1, (\(1.6{\rm{dB}}\), \(-1.0{\rm{dB}}\)) in mode 2, and (\(1.4{\rm{dB}}\), \(-0.7{\rm{dB}}\)) in mode 3.

Source data

Source Data Fig. 2

Data of W\({}_{0}\) and F shown in Fig. 2.

Source Data Fig. 3

Data used to plot Fig. 3.

Source Data Extended Data Fig. 1

Data of W\({}_{0}\) and F shown in Extended Data Fig. 1.

Source Data Extended Data Fig. 3

Data of W\({}_{0}\) shown in Extended Data Fig. 3.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ra, YS., Dufour, A., Walschaers, M. et al. Non-Gaussian quantum states of a multimode light field. Nat. Phys. 16, 144–147 (2020). https://doi.org/10.1038/s41567-019-0726-y

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/s41567-019-0726-y

This article is cited by

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing