Abstract
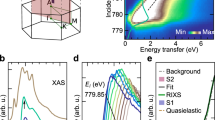
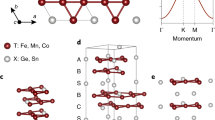
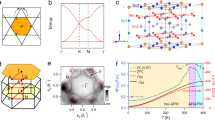
Electronic systems with flat bands are predicted to be a fertile ground for hosting emergent phenomena including unconventional magnetism and superconductivity1,2,3,4,5,6,7,8,9,10,11,12,13,14,15, but materials that manifest this feature are rare. Here, we use scanning tunnelling microscopy to elucidate the atomically resolved electronic states and their magnetic response in the kagome magnet Co3Sn2S2 (refs. 16,17,18,19,20). We observe a pronounced peak at the Fermi level, which we identify as arising from the kinetically frustrated kagome flat band. On increasing the magnetic field up to ±8 T, this state exhibits an anomalous magnetization-polarized many-body Zeeman shift, dominated by an orbital moment that is opposite to the field direction. Such negative magnetism is induced by spin–orbit-coupling quantum phase effects21,22,23,24,25 tied to non-trivial flat band systems. We image the flat band peak, resolve the associated negative magnetism and provide its connection to the Berry curvature field, showing that Co3Sn2S2 is a rare example of a kagome magnet where the low-energy physics can be dominated by the spin–orbit-coupled flat band.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
Data availability
The data that support the plots within this paper and other findings of this study are available from the corresponding author upon reasonable request.
References
Soumyanarayanan, A., Reyren, N., Fert, A. & Panagopoulos, C. Emergent phenomena induced by spin–orbit coupling at surfaces and interfaces. Nature 539, 509–517 (2016).
Wang, J. & Zhang, S.-C. Topological states of condensed matter. Nat. Mater. 16, 1062–1067 (2017).
Hasan, M. Z., Xu, S.-Y. & Bian, G. Topological insulators, topological superconductors and Weyl fermion semimetals. Phys. Scr. T164, 014001 (2015).
Hasan, M. Z., Xu, S.-Y., Belopolski, I. & Huang, S.-M. Discovery of Weyl fermion semimetals and topological Fermi arc states. Annu. Rev. Condens. Matter Phys. 8, 289–309 (2017).
Sachdev, S. Topological order, emergent gauge fields, and Fermi surface reconstruction. Rep. Prog. Phys. 82, 014001 (2019).
Cao, Y. et al. Unconventional superconductivity in magic-angle graphene superlattices. Nature 556, 43–50 (2018).
Yin, J.-X. et al. Giant and anisotropic spin–orbit tunability in a strongly correlated kagome magnet. Nature 562, 91–95 (2018).
Belopolski, I. et al. Topological Weyl lines and drumhead surface states in a room-temperature magnet. Preprint at https://arxiv.org/abs/1712.09992 (2017).
Si, Q. & Steglich, F. Heavy fermions and quantum phase transitions. Science 329, 1161–1166 (2010).
Mielke, A. Exact ground states for the Hubbard model on the Kagome lattice. J. Phys. Rev. Lett. 69, 1608 (1992).
Wu, C., Bergan, D., Balents, L. & Sarma, S. D. Flat bands and Wigner crystallization in the honeycomb optical lattice. Phys. Rev. Lett. 99, 070401 (2007).
Leykam, D., Andreanov, A. & Flach, S. Artificial flat band systems: from lattice models to experiments. Adv. Phys. X 3, 1473052 (2018).
Tang, E., Mei, J. W. & Wen, X. G. High-temperature fractional quantum Hall states. Phys. Rev. Lett. 106, 236802 (2011).
Sun, K. et al. Nearly flat bands with nontrivial topology. Phys. Rev. Lett. 106, 236803 (2011).
Neupert, T. et al. Fractional quantum Hall states at zero magnetic field. Phys. Rev. Lett. 106, 236804 (2011).
Vaqueiro, P. & Sobany, G. G. A powder neutron diffraction study of the metallic ferromagnet Co3Sn2S2. Solid State Sci. 11, 513–518 (2009).
Dedkov, Y. S., Holder, M., Molodtsov, S. L. & Rosner, H. Electronic structure of shandite Co3Sn2S2. J. Phys. Conf. Ser. 100, 072011 (2008).
Schnelle, W. et al. Ferromagnetic ordering and half-metallic state of Sn2Co3S2 with the Shandite-type structure. Phys. Rev. B 88, 144404 (2013).
Wang, Qi et al. Large intrinsic anomalous Hall effect in half-metallic ferromagnet Co3Sn2S2 with magnetic Weyl fermions. Nat. Commun. 9, 3681 (2018).
Liu, E. et al. Giant anomalous Hall angle in a half-metallic magnetic Weyl semimetal. Nat. Phys. 14, 1125–1131 (2018).
Karplus, R. & Luttinger, J. M. Hall effect in ferromagnetics. Phys. Rev. 95, 1154–1160 (1954).
Xiao, D., Chang, M. C. & Niu, Q. Berry phase effects on electronic properties. Rev. Mod. Phys. 82, 1959–2007 (2010).
Resta, R. Electrical polarization and orbital magnetization: the modern theories. J. Phys. Condens. Matter 22, 123201 (2010).
Thonhauser, T. Theory of orbital magnetization in solids. Int. J. Mod. Phys. B 25, 1429 (2011).
Vanderbilt, D. Berry Phases in Electronic Structure Theory: Electric Polarization, Orbital Magnetization and Topological Insulators (Cambridge University Press, Cambridge, 2018).
Fischer, Ø. et al. Scanning tunneling spectroscopy of high-temperature superconductors. Rev. Mod. Phys. 79, 353 (2007).
Ternes, M., Heinrich, Andreas., J. & Schneider, W.-D. Spectroscopic manifestations of the Kondo effect on single adatoms. J. Phys. Condens. Matter 21, 053001 (2009).
Nayak, A. K. et al. Large anomalous Hall effect driven by a nonvanishing Berry curvature in the noncolinear antiferromagnet Mn3Ge. Sci. Adv. 2, e1501870 (2016).
Kuroda, K. et al. Evidence for magnetic Weyl fermions in a correlated metal. Nat. Mater. 16, 1090–1095 (2017).
Aivazian, G. et al. Magnetic control of valley pseudospin in monolayer WSe2. Nat. Phys. 11, 148–152 (2015).
Acknowledgements
STM experimental and theoretical work at Princeton University was supported by the Gordon and Betty Moore Foundation (GBMF4547/M.Z.H.). ARPES characterization of the sample is supported by the United States Department of Energy (US DOE) under the Basic Energy Sciences programme (grant number DOE/BES DE-FG-02–05ER46200). Work at Renmin University was supported by the Ministry of Science and Technology of China (2016YFA0300504), the National Natural Science Foundation of China (nos. 11574394, 11774423 and 11822412), the Fundamental Research Funds for the Central Universities, and the Research Funds of Renmin University of China (15XNLF06, 15XNLQ07 and 18XNLG14). S.S.T. and T.N. acknowledge support from the European Union’s Horizon 2020 research and innovation programme (ERC-StG-Neupert-757867-PARATOP). Z.W. and K.J. acknowledge US DOE grant DE-FG02–99ER45747. We also acknowledge the Natural Science Foundation of China (grant nos. 11790313 and 11774007), the Key Research Program of the Chinese Academy of Sciences (grant no. XDPB08-1), the National Key R&D Program of China (grant nos. 2016YFA0300403 and 2018YFA035601), the Princeton Center for Theoretical Science and the Princeton Institute for the Science and Technology of Materials Imaging and Analysis Center at Princeton University. Muon spin rotation (µSR) experiments were performed at the πE3 beamline of the Paul Scherrer Institute, using the HAL-9500 µSR spectrometer. Z.G. acknowledges R. Scheuermann for support in the µSR experiments. M.Z.H. acknowledges support from Lawrence Berkeley National Laboratory and the Miller Institute of Basic Research in Science at the University of California, Berkeley in the form of a Visiting Miller Professorship.
Author information
Authors and Affiliations
Contributions
J.-X.Y. and S.S.Z. conducted the STM and STS experiments in consultation with M.Z.H.; Q.W., H.Lei, H.Z. and S.J. synthesized and characterized the sequence of samples; Z.G. performed µSR measurements in consultation with M.Z.H.; G.C., T.N., S.S.T., B.L., K.J., H.Lin and Z.W. carried out theoretical analysis in consultation with J.-X.Y. and M.Z.H.; I.B., N.S., D.M., M.L. and T.A.C. contributed to sample characterization and instrument calibration; J.-X.Y., S.S.Z. and M.Z.H. performed the data analysis and figure development and wrote the paper with contributions from all authors; M.Z.H. supervised the project. All authors discussed the results, interpretation and conclusion.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Journal peer review information: Nature Physics thanks Oleg Yazyev and other anonymous reviewer(s) for their contribution to the peer review of this work.
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Supplementary Information
Supplementary Figures 1–7, additional theoretical details and Supplementary References 31–35.
Rights and permissions
About this article
Cite this article
Yin, JX., Zhang, S.S., Chang, G. et al. Negative flat band magnetism in a spin–orbit-coupled correlated kagome magnet. Nat. Phys. 15, 443–448 (2019). https://doi.org/10.1038/s41567-019-0426-7
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41567-019-0426-7
This article is cited by
-
The discovery of three-dimensional Van Hove singularity
Nature Communications (2024)
-
Ultrafast magnetization enhancement via the dynamic spin-filter effect of type-II Weyl nodes in a kagome ferromagnet
Nature Communications (2024)
-
Magnetism and fermiology of kagome magnet YMn6Sn4Ge2
npj Quantum Materials (2024)
-
Atomically precise engineering of spin–orbit polarons in a kagome magnetic Weyl semimetal
Nature Communications (2024)
-
Flat-band hybridization between f and d states near the Fermi energy of SmCoIn5
npj Quantum Materials (2024)