Abstract
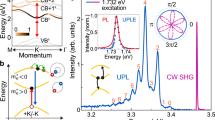
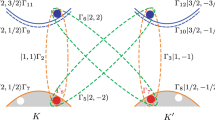
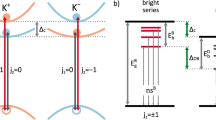
Monolayer transition metal dichalcogenides (TMDCs) are promising two-dimensional (2D) semiconductors for application in optoelectronics. Their optical properties are dominated by two series of photo-excited exciton states—A (XA) and B (XB)1,2—that are derived from direct interband transitions near the band extrema. These exciton states have large binding energies and strong optical absorption3,4,5,6, and form an ideal system to investigate many-body effects in low dimensions. Because spin–orbit coupling causes a large splitting between bands of opposite spins, XA and XB are usually treated as spin-polarized Ising excitons, each arising from interactions within a specific set of states induced by interband transitions between pairs of either spin-up or spin-down bands (TA or TB). Here, by using monolayer MoS2 as a prototypical system and solving the first-principles Bethe–Salpeter equations, we demonstrate a strong intravalley exchange interaction between TA and TB, indicating that XA and XB are mixed states instead of pure Ising excitons. Using 2D electronic spectroscopy, we observe that an optical excitation of the lower-energy TA induces a population of the higher-energy TB, manifesting the intravalley exchange interaction. This work elucidates the dynamics of exciton formation in monolayer TMDCs, and sheds light on many-body effects in 2D materials.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout



Similar content being viewed by others
Data availability
The data that support the findings of this study are available from the corresponding authors on reasonable request.
References
Mak, K. F., Lee, C., Hone, J., Shan, J. & Heinz, T. F. Atomically thin MoS2: a new direct-gap semiconductor. Phys. Rev. Lett. 105, 136805 (2010).
Splendiani, A. et al. Emerging photoluminescence in monolayer MoS2. Nano Lett. 10, 1271–1275 (2010).
Qiu, D. Y., da Jornada, F. H. & Louie, S. G. Optical spectrum of MoS2: many-body effects and diversity of exciton states. Phys. Rev. Lett. 111, 216805 (2013).
Ugeda, M. M. et al. Giant bandgap renormalization and excitonic effects in a monolayer transition metal dichalcogenide semiconductor. Nat. Mater. 13, 1091–1095 (2014).
Ye, Z. et al. Probing excitonic dark states in single-layer tungsten disulphide. Nature 513, 214–218 (2014).
Chernikov, A. et al. Exciton binding energy and nonhydrogenic Rydberg series in monolayer WS2. Phys. Rev. Lett. 113, 076802 (2014).
Rossler, U., Jorda, S. & Broido, D. Fine structure of quantum well excitons. Solid State Commun. 73, 209–214 (1990).
Andreani, L. C. & Bassani, F. Exchange interaction and polariton effects in quantum-well excitons. Phys. Rev. B 41, 7536–7544 (1990).
Damen, T. C., Via, L., Cunningham, J. E., Shah, J. & Sham, L. J. Subpicosecond spin relaxation dynamics of excitons and free carriers in GaAs quantum wells. Phys. Rev. Lett. 67, 3432–3435 (1991).
Maialle, M. Z., de Andrada e Silva, E. A. & Sham, L. J. Exciton spin dynamics in quantum wells. Phys. Rev. B 47, 15776–15788 (1993).
Vinattieri, A. et al. Exciton dynamics in GaAs quantum wells under resonant excitation. Phys. Rev. B 50, 10868–10879 (1994).
Yu, T. & Wu, M. W. Valley depolarization due to intervalley and intravalley electron–hole exchange interactions in monolayer MoS2. Phys. Rev. B 89, 205303 (2014).
Jones, A. M. et al. Optical generation of excitonic valley coherence in monolayer WSe2. Nat. Nanotech. 8, 634–638 (2013).
Hao, K. et al. Direct measurement of exciton valley coherence in monolayer WSe2. Nat. Phys. 12, 677–682 (2016).
Yu, H., Liu, G.-B., Gong, P., Xu, X. & Yao, W. Dirac cones and Dirac saddle points of bright excitons in monolayer transition metal dichalcogenides. Nat. Commun. 5, 3876 (2014).
Qiu, D. Y., Cao, T. & Louie, S. G. Nonanalyticity, valley quantum phases, and lightlike exciton dispersion in monolayer transition metal dichalcogenides: theory and first-principles calculations. Phys. Rev. Lett. 115, 176801 (2015).
Wu, F., Qu, F. & MacDonald, A. H. Exciton band structure of monolayer MoS2. Phys. Rev. B 91, 075310 (2015).
Mai, C. et al. Many-body effects in valleytronics: direct measurement of valley lifetimes in single-layer MoS2. Nano Lett. 14, 202–206 (2014).
Singh, A. et al. Coherent electronic coupling in atomically thin MoSe2. Phys. Rev. Lett. 112, 216804 (2014).
Hao, K. et al. Coherent and incoherent coupling dynamics between neutral and charged excitons in monolayer MoSe2. Nano Lett. 16, 5109–5113 (2016).
Mak, K. F. et al. Tightly bound trions in monolayer MoS2. Nat. Mater. 12, 207–211 (2013).
Brixner, T. et al. Two-dimensional spectroscopy of electronic couplings in photosynthesis. Nature 434, 625–628 (2005).
Panitchayangkoon, G. et al. Long-lived quantum coherence in photosynthetic complexes at physiological temperature. Proc. Natl Acad. Sci. USA 107, 12766–12770 (2010).
Li, X., Zhang, T., Borca, C. N. & Cundiff, S. T. Many-body interactions in semiconductors probed by optical two-dimensional Fourier transform spectroscopy. Phys. Rev. Lett. 96, 057406 (2006).
Graham, M. W., Calhoun, T. R., Green, A. A., Hersam, M. C. & Fleming, G. R. Two-dimensional electronic spectroscopy reveals the dynamics of phonon-mediated excitation pathways in semiconducting single-walled carbon nanotubes. Nano Lett. 12, 813–819 (2012).
Moody, G. et al. Intrinsic homogeneous linewidth and broadening mechanisms of excitons in monolayer transition metal dichalcogenides. Nat. Commun. 6, 8315 (2015).
Czech, K. J. et al. Measurement of ultrafast excitonic dynamics of few-layer MoS2 using state-selective coherent multidimensional spectroscopy. ACS Nano 9, 12146–12157 (2015).
Jones, A. M. et al. Excitonic luminescence upconversion in a two-dimensional semiconductor. Nat. Phys. 12, 323–327 (2016).
Yang, L. et al. Long-lived nanosecond spin relaxation and spin coherence of electrons in monolayer MoS2 and WS2. Nat. Phys. 11, 830–834 (2015).
Deslippe, J. et al. BerkeleyGW: a massively parallel computer package for the calculation of the quasiparticle and optical properties of materials and nanostructures. Comput. Phys. Commun. 183, 1269–1289 (2012).
Giannozzi, P. et al. Quantum ESPRESSO: a modular and open-source software project for quantum simulations of materials. J. Phys. Condens. Matter 21, 395502 (2009).
Hybertsen, M. S. & Louie, S. G. Electron correlation in semiconductors and insulators: band gaps and quasiparticle energies. Phys. Rev. B 34, 5390–5413 (1986).
da Jornada, F. H., Qiu, D. Y. & Louie, S. G. Nonuniform sampling schemes of the Brillouin zone for many-electron perturbation-theory calculations in reduced dimensionality. Phys. Rev. B 95, 035109 (2017).
Deslippe, J., Samsonidze, G., Jain, M., Cohen, M. L. & Louie, S. G. Coulomb-hole summations and energies for GW calculations with limited number of empty orbitals: a modified static remainder approach. Phys. Rev. B 87, 165124 (2013).
Mostofi, A. A. et al. Wannier90: a tool for obtaining maximally-localised Wannier functions. Comput. Phys. Commun. 178, 685–699 (2008).
Rohlfing, M. & Louie, S. G. Electron–hole excitations in semiconductors and insulators. Phys. Rev. Lett. 81, 2312–2315 (1998).
Rohlfing, M. & Louie, S. G. Electron–hole excitations and optical spectra from first principles. Phys. Rev. B 62, 4927–4944 (2000).
Yu, L. et al. Design, modeling, and fabrication of chemical vapor deposition grown MoS2 circuits with E-mode FETs for large-area electronics. Nano Lett. 16, 6349–6356 (2016).
Li, H. et al. From bulk to monolayer MoS2: evolution of Raman scattering. Adv. Funct. Mater. 22, 1385–1390 (2012).
Mak, K. F., Lee, C., Hone, J., Shan, J. & Heinz, T. F. Atomically thin MoS2: a new direct-gap semiconductor. Phys. Rev. Lett. 105, 136805 (2010).
Cerullo, G., Nisoli, M., Stagira, S. & De Silvestri, S. Sub-8-fs pulses from an ultrabroadband optical parametric amplifier in the visible. Opt. Lett. 23, 1283–1285 (1998).
Shirakawa, A., Sakane, I. & Kobayashi, T. Pulse-front-matched optical parametric amplification for sub-10-fs pulse generation tunable in the visible and near infrared. Opt. Lett. 23, 1292–1294 (1998).
Isaienko, O. & Borguet, E. Pulse-front matching of ultrabroadband near-infrared noncollinear optical parametric amplified pulses. J. Opt. Soc. Am. B 26, 965–972 (2009).
Brixner, T., Mancal, T., Stiopkin, I. V. & Fleming, G. R. Phase-stabilized two-dimensional electronic spectroscopy. J. Chem. Phys. 121, 4221–4236 (2004).
Guo, L., Monahan, D. M. & Fleming, G. R. Rapid and economical data acquisition in ultrafast frequency-resolved spectroscopy using choppers and a microcontroller. Opt. Express 26, 18126–18132 (2016).
Monahan, D. M. et al. Room-temperature coherent optical phonon in 2D electronic spectra of CH3NH3PbI3 perovskite as a possible cooling bottleneck. J. Phys. Chem. Lett. 8, 3211–3215 (2017).
Acknowledgements
We thank G. Moody and K. Hao for helpful discussion. This material is based on work supported by the National Science Foundation under grant no. CHE-1362830, grant no. DMR-1508412 and grant no. EFMA-1542741. D.M.M. received a National Science Foundation Graduate Research Fellowship under grant no. DGE-1106400. Advanced codes were provided by the Center for Computational Study of Excited-State Phenomena in Energy Materials (C2SEPEM) at LBNL, which is funded by the US Department of Energy, Office of Science, Basic Energy Sciences, Materials Sciences and Engineering Division under contract no. DE-AC02-05CH11231, as part of the Computational Materials Sciences Program. Computational resources were provided by the DOE at Lawrence Berkeley National Laboratory’s NERSC facility and the NSF through XSEDE resources at NICS. Y.-H. L. acknowledges support from the Ministry of Science and Technology (MoST-106-2119-M-007-023-MY3; MoST-105-2112-M-007-032-MY3), the Frontier Research Center on Fundamental and Applied Sciences of Matters, and the Center for Quantum Technology of National Tsing Hua University.
Author information
Authors and Affiliations
Contributions
L.G., M.W., T.C. and D.M.M. contributed equally to this work. L.G., M.W. and T.C. conceived the concept. Supervised by G.R.F., L.G. and D.M.M. led the experiments by designing the optical system and acquiring the data. M.W. and T.C. performed the theoretical studies under the supervision of S.G.L. Y.-H.L. provided the samples. L.G., M.W. and T.C. wrote the manuscript. All authors discussed the results and commented on the manuscript.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Supplementary Information
Supplementary Figures 1–11; Supplementary Tables 1–4; Supplementary References 1–24
Rights and permissions
About this article
Cite this article
Guo, L., Wu, M., Cao, T. et al. Exchange-driven intravalley mixing of excitons in monolayer transition metal dichalcogenides. Nat. Phys. 15, 228–232 (2019). https://doi.org/10.1038/s41567-018-0362-y
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41567-018-0362-y
This article is cited by
-
Optical two-dimensional coherent spectroscopy of excitons in transition-metal dichalcogenides
Frontiers of Physics (2024)
-
Two-dimensional electronic spectroscopy
Nature Reviews Methods Primers (2023)
-
Time-domain observation of interlayer exciton formation and thermalization in a MoSe2/WSe2 heterostructure
Nature Communications (2023)
-
Opto-valleytronics in the 2D van der Waals heterostructure
Nano Research (2021)
-
Dynamics of exciton energy renormalization in monolayer transition metal disulfides
Nano Research (2020)