Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

  • Article
  • Published:

Multiple Coulomb phase in the fluoride pyrochlore CsNiCrF6

Nature Physics volume 15, pages 60–66 (2019)Cite this article

Subjects

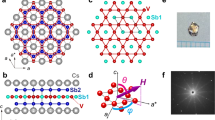
Abstract

The Coulomb phase is an idealized state of matter whose properties are determined by factors beyond conventional considerations of symmetry, including global topology, conservation laws and emergent order. Theoretically, Coulomb phases occur in ice-type systems such as water ice and spin ice; in dimer models; and in certain spin liquids. However, apart from ice-type systems, more general experimental examples are very scarce. Here we study the partly disordered material CsNiCrF6 and show that this material is a multiple Coulomb phase with signature correlations in three degrees of freedom: charge configurations, atom displacements and spin configurations. We use neutron and X-ray scattering to separate these correlations and to determine the magnetic excitation spectrum. Our results show how the structural and magnetic properties of apparently disordered materials may inherit, and be dictated by, a hidden symmetry—the local gauge symmetry of an underlying Coulomb phase.

This is a preview of subscription content, access via your institution

Access options

Buy this article

  • Purchase on Springer Link
  • Instant access to full article PDF
Buy now

Prices may be subject to local taxes which are calculated during checkout

Fig. 1: Illustration of Coulomb-phase construction/mappings and structural features of CsNiCrF6.
Fig. 2: Illustration of theoretical predictions of the dynamical response of the pyrochlore Heisenberg antiferromagnet.
Fig. 3: Structural diffuse scattering in CsNiCrF6.
Fig. 4: Magnetic diffuse scattering in CsNiCrF6.
Fig. 5: Wavevector and temperature dependence of magnetic dynamics in CsNiCrF6.

Similar content being viewed by others

Data availability

The experimental data and their supplementary information, analyses and computer codes that support the plots within this paper and the findings of this study are available from the corresponding author upon reasonable request.

References

  1. Landau, L. D. & Lifshitz, E. M. Statistical Physics (Pergamon, New York, 1980).

    MATH  Google Scholar 

  2. Pekker, D. & Varma, C. M. Amplitude/Higgs modes in condensed matter physics. Annu. Rev. Condens. Matter Phys. 6, 269–297 (2015).

    Article  ADS  Google Scholar 

  3. Henley, C. L. The “Coulomb phase” in frustrated systems. Annu. Rev. Condens. Matter Phys. 1, 179–210 (2010).

    Article  ADS  Google Scholar 

  4. Jaubert, L. D. C. et al. Topological-sector fluctuations and Curie-law crossover in spin ice. Phys. Rev. X 3, 011014 (2013).

    Google Scholar 

  5. Huse, D., Krauth, W., Moessner, R. & Sondhi, S. L. Coulomb and liquid dimer models in three dimensions. Phys. Rev. Lett. 91, 167004 (2003).

    Article  ADS  Google Scholar 

  6. Hermele, M., Fisher, M. P. A. & Balents, L. Pyrochlore photons: the U(1) spin liquid in a S = 1/2 three-dimensional frustrated magnet. Phys. Rev. B 69, 064404 (2004).

    Article  ADS  Google Scholar 

  7. Benton, O., Sikora, O. & Shannon, N. Seeing the light: experimental signatures of emergent electromagnetism in a quantum spin ice. Phys. Rev. B 86, 075154 (2012).

    Article  ADS  Google Scholar 

  8. Gingras, M. J. P. & McClarty, P. A. Quantum spin ice: a search for gapless quantum spin liquids in pyrochlore magnets. Rep. Prog. Phys. 77, 056501 (2014).

    Article  ADS  Google Scholar 

  9. Anderson, P. W. Ordering and antiferromagnetism in ferrites. Phys. Rev. 102, 1008–1013 (1956).

    Article  ADS  Google Scholar 

  10. McClarty, P. A., O’Brien, A. & Pollmann, F. Coulombic charge ice. Phys. Rev. B 89, 195123 (2014).

    Article  ADS  Google Scholar 

  11. Moessner, R. & Chalker, J. T. Properties of a classical spin liquid: the Heisenberg pyrochlore antiferromagnet. Phys. Rev. Lett. 80, 2929–2932 (1998).

    Article  ADS  Google Scholar 

  12. Isakov, S. V., Gregor, K., Moessner, R. & Sondhi, S. L. Dipolar spin correlations in classical pyrochlore magnets. Phys. Rev. Lett. 93, 167204 (2004).

    Article  ADS  Google Scholar 

  13. Moessner, R. & Chalker, J. T. Low-temperature properties of classical geometrically frustrated antiferromagnets. Phys. Rev. B 58, 12049–12062 (1998).

    Article  ADS  Google Scholar 

  14. Conlon, P. H. & Chalker, J. T. Spin dynamics in pyrochlore Heisenberg antiferromagnets. Phys. Rev. Lett. 102, 237206 (2009).

    Article  ADS  Google Scholar 

  15. Conlon, P. H. Aspects of Frustrated Magnetism. PhD thesis, Univ. Oxford (2010).

  16. Henley, C. L. Power-law spin correlations in pyrochlore antiferromagnets. Phys. Rev. B 71, 014424 (2005).

    Article  ADS  Google Scholar 

  17. Fennell, T. et al. Magnetic Coulomb phase in the spin ice Ho2Ti2O7. Science 326, 415–417 (2009).

    Article  ADS  Google Scholar 

  18. Harris, M. J., Bramwell, S. T., McMorrow, D. F., Zeiske, T. & Godfrey, K. W. Geometrical frustration in the ferromagnetic pyrochlore Ho2Ti2O7. Phys. Rev. Lett. 79, 2554–2557 (1997).

    Article  ADS  Google Scholar 

  19. Castelnovo, C., Moessner, R. & Sondhi, S. L. Magnetic monopoles in spin ice. Nature 451, 42–45 (2008).

    Article  ADS  Google Scholar 

  20. Ryzhkin, I. A. Magnetic relaxation in rare-earth oxide pyrochlores. J. Exp. Theor. Phys. 101, 481–486 (2005).

    Article  ADS  Google Scholar 

  21. Overy, A. R. et al. Design of crystal-like aperiodic solids with selective disorder–phonon coupling. Nat. Commun. 7, 10445 (2016).

    Article  ADS  Google Scholar 

  22. Shen, L., Greaves, C., Riyat, R., Hansen, T. C. & Blackburn, E. Absence of magnetic long-range order in Y2CrSbO7: bond-disorder-induced magnetic frustration in a ferromagnetic pyrochlore. Phys. Rev. B 96, 094438 (2017).

    Article  ADS  Google Scholar 

  23. Li, Y. et al. Crystalline electric-field randomness in the triangular lattice spin-liquid YbMgGaO4. Phys. Rev. Lett. 118, 107202 (2017).

    Article  ADS  Google Scholar 

  24. Zhu, Z., Maksimov, P. A., White, S. R. & Chernyshev, A. L. Disorder-induced mimicry of a spin liquid in YbMgGaO4. Phys. Rev. Lett. 119, 157201 (2017).

    Article  ADS  Google Scholar 

  25. Harris, M. J., Zinkin, M. P. & Zeiske, T. Magnetic excitations in a highly frustrated pyrochlore antiferromagnet. Phys. Rev. B 52, R707–R710 (1995).

    Article  ADS  Google Scholar 

  26. Zinkin, M. P., Harris, M. J. & Zeiske, T. Short-range magnetic order in the frustrated pyrochlore antiferromagnet CsNiCrF6. Phys. Rev. B 56, 11786–11790 (1997).

    Article  ADS  Google Scholar 

  27. Banks, S. T. & Bramwell, S. T. Magnetic frustration in the context of pseudo-dipolar ionic disorder. EPL 97, 27005 (2012).

    Article  Google Scholar 

  28. Keen, D. A. & Goodwin, A. L. The crystallography of correlated disorder. Nature 521, 303–309 (2015).

    Article  ADS  Google Scholar 

  29. Babel, D., Pausewang, G. & Viebahn, W. Die Struktur einiger Fluoride, Oxide und Oxidfluoride AMe2X6 der RbNiCrF6 -Typ. Zeitschrift für Naturforshung B 22, 1219–1220 (1967).

    Article  Google Scholar 

  30. Shoemaker, D. P. et al. Atomic displacements in the charge ice pyrochlore Bi2Ti2O6O’ studied by neutron total scattering. Physical Review B 81, 144113 (2010).

    Article  ADS  Google Scholar 

  31. Welberry, T. R. & Butler, B. D. Interpretation of diffuse X-ray scattering via models of disorder. J. Appl. Crystallogr. 27, 205–231 (1994).

    Article  Google Scholar 

  32. Neder, R. B. & Proffen, T. “Diffuse Scattering and Defect Structure Simulations: A cook book using the program DISCUS”. (Oxford University Press, Oxford, 2008).

    Book  Google Scholar 

  33. Paddison, J. A. M. & Goodwin, A. L. Empirical magnetic structure solution of frustrated spin systems. Phys. Rev. Lett. 108, 017204 (2012).

    Article  ADS  Google Scholar 

  34. Billinge, S. J. L. & Levin, I. The problem with determining atomic structure at the nanoscale. Science 316, 561–565 (2007).

    Article  ADS  Google Scholar 

  35. Brown, I. D. Recent developments in the methods and applications of the bond valence model. Chem. Rev. 109, 6858–6919 (2009).

    Article  Google Scholar 

  36. Chernyshev, V. V., Zhukov, S. G., Yatsenko, A. V., Aslanov, L. A. & Schenk, H. The use of continuous atomic distributions in structural investigations. Acta Cryst. A50, 601–605 (1994).

    Article  Google Scholar 

  37. Jaubert, L. D. C., Haque, M. & Moessner, R. Analysis of a fully packed loop model arising in a magnetic Coulomb phase. Phys. Rev. Lett. 107, 177202 (2011).

    Article  ADS  Google Scholar 

  38. Blunt, M. O. et al. Random tiling and topological defects in a two-dimensional molecular network. Science 322, 1077–1081 (2008).

    Article  ADS  Google Scholar 

  39. Jacobsen, J. L. & Alet, F. Semiflexible fully packed loop model and interacting rhombus tilings. Phys. Rev. Lett. 102, 145702 (2009).

    Article  ADS  Google Scholar 

  40. Savary, L. & Balents, L. Quantum spin liquids: a review. Rep. Prog. Phys. 80, 016502 (2017).

    Article  ADS  Google Scholar 

  41. Plumb, K. W. et al. Continuum of quantum fluctuations in a three-dimensional S = 1 Heisenberg magnet. Nat. Phys. https://doi.org/10.1038/s41567-018-0317-3 (2018).

  42. Koza, M. M. et al. Breakdown of phonon glass paradigm in La- and Ce-filled Fe4Sb12 skutterudites. Nat. Mater. 7, 805–810 (2008).

    Article  ADS  Google Scholar 

  43. Shimojima, T. et al. Interplay of superconductivity and rattling phenomena in β-pyrochlore KOs2O6 studied by photoemission spectroscopy. Phys. Rev. Lett. 99, 117003 (2007).

    Article  ADS  Google Scholar 

  44. De Pape, R. & Ferey, G. A new form of FeF3 with the pyrochlore structure: Soft chemistry synthesis, crystal structure, thermal transitions and structural correlations with the other forms of FeF3. Mater. Res. Bull. 21, 971–978 (1986).

    Article  Google Scholar 

  45. Kim, S. W. et al. RbFe2+Fe3+F6: Synthesis, structure, and characterization of a new charge-ordered magnetically frustrated pyrochlore-related mixed-metal fluoride. Chem. Sci. 3, 741–751 (2012).

    Article  Google Scholar 

  46. Songvilay, M. et al. Anharmonic magnon excitations in noncollinear and charge-ordered RbFe2+Fe3+F6. Phys. Rev. Lett. 121, 087201 (2018).

    Article  ADS  Google Scholar 

  47. Willmott, P. R. et al. The Materials Science beamline upgrade at the Swiss Light Source. J. Synchrotron. Radiat. 20, 667–682 (2013).

    Article  Google Scholar 

  48. Rodriguez-Carvajal, J. Recent advances in magnetic structure determination. Physica B 192, 55–69 (1993).

    Article  ADS  Google Scholar 

  49. Wilkinson, C., Cowan, J. A., Myles, D. A. A., Cipriani, F. & McIntyre, G. J. VIVALDI - a thermal-neutron Laue diffractometer for physics, chemistry and materials science. Neutron News 13, 37–41 (2002).

    Article  Google Scholar 

  50. Campbell, J. W., Hao, Q., Harding, M. M., Nguti, N. D. & Wilkinson, C. LAUEGEN version 6.0 and INTLDM. J. Appl. Crystallogr. 31, 496–502 (1998).

    Article  Google Scholar 

  51. Helliwell, J. R. et al. The recording and analysis of synchrotron X-radiation Laue diffraction photographs. J. Appl. Crystallogr. 22, 483–497 (1989).

    Article  Google Scholar 

  52. Sheldrick, G. M. A short history of SHELX. Acta Cryst A64, 112–122 (2008).

    Article  Google Scholar 

  53. Schefer, J. et al. Single-crystal diffraction instrument TriCS at SINQ. Physica B 276-278, 168–169 (2000).

    Article  ADS  Google Scholar 

  54. Petricek, V., Dusek, M. & Palatinus, L. Crystallographic Computing System JANA2006: General features. Zeitschrift für Kristallographie 229, 345–352 (2014).

    Google Scholar 

  55. Stewart, J. R. et al. Disordered materials studied using neutron polarization analysis on the multi-detector spectrometer. D7. J. Appl. Crystallogr. 42, 69–84 (2009).

    Article  Google Scholar 

  56. Lee, S.-H. et al. Emergent excitations in a geometrically frustrated magnet. Nature 418, 856–858 (2002).

    Article  ADS  Google Scholar 

  57. Melko, R. G., den Hertog, B. C. & Gingras, M. J. P. Long-range order at low temperatures in dipolar spin ice. Phys. Rev. Lett. 87, 067203 (2001).

    Article  ADS  Google Scholar 

Download references

Acknowledgements

We thank R. Stewart, M. Green and B. Fåk for discussions, J. Chalker for reading and commenting on the manuscript, and X. Thonon for support of cryogenics at the ILL. M.R. was supported by the Schweizerischer Nationalfonds zur Förderung der Wissenschaftlichen Forschung, grant no. 200021_140862. This work is based on experiments performed at the Institut Laue-Langevin, Grenoble, France; the Swiss spallation neutron source SINQ, Paul Scherrer Institut, Villigen, Switzerland; and the Swiss Light Source, Paul Scherrer Institut.

Author information

Authors and Affiliations

  1. Laboratory for Neutron Scattering and Imaging, Paul Scherrer Institut, Villigen PSI, Switzerland

    T. Fennell, M. Ruminy & O. Zaharko

  2. School of Divinity, University of Edinburgh, New College, Edinburgh, UK

    M. J. Harris

  3. Quantum Condensed Matter Division, Oak Ridge National Laboratory, Oak Ridge, TN, USA

    S. Calder

  4. Institut Laue-Langevin, Grenoble, France

    M. Boehm, P. Steffens & M.-H. Lemée-Cailleau

  5. Swiss Light Source, Paul Scherrer Institut, Villigen PSI, Switzerland

    A. Cervellino

  6. London Centre for Nanotechnology and Department of Physics and Astronomy, University College London, London, UK

    S. T. Bramwell

Authors
  1. T. Fennell
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. M. J. Harris
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. S. Calder
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. M. Ruminy
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. M. Boehm
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. P. Steffens
    View author publications

    You can also search for this author in PubMed Google Scholar

  7. M.-H. Lemée-Cailleau
    View author publications

    You can also search for this author in PubMed Google Scholar

  8. O. Zaharko
    View author publications

    You can also search for this author in PubMed Google Scholar

  9. A. Cervellino
    View author publications

    You can also search for this author in PubMed Google Scholar

  10. S. T. Bramwell
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

T.F., M.J.H., S.C., M.B., P.S. and S.T.B. carried out inelastic neutron scattering experiments. T.F., M.-H.L.-C. and O.Z. carried out neutron diffraction experiments. M.R. and A.C. carried out X-ray diffraction experiments. T.F. analysed all data and made calculations. T.F., M.J.H. and S.T.B. wrote the paper in collaboration with all other authors.

Corresponding author

Correspondence to T. Fennell.

Ethics declarations

Competing interests

The authors declare no competing interests.

Additional information

Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Supplementary information

Supplementary information

Supplementary Text, Figures 1–3, Table 1

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Fennell, T., Harris, M.J., Calder, S. et al. Multiple Coulomb phase in the fluoride pyrochlore CsNiCrF6. Nature Phys 15, 60–66 (2019). https://doi.org/10.1038/s41567-018-0309-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/s41567-018-0309-3

This article is cited by

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing