Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

  • Article
  • Published:

Bose–Einstein condensation in a plasmonic lattice

Nature Physics volume 14, pages 739–744 (2018)Cite this article

Subjects

Abstract

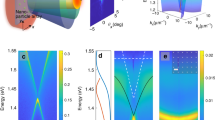
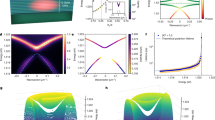
Bose–Einstein condensation is a remarkable manifestation of quantum statistics and macroscopic quantum coherence. Superconductivity and superfluidity have their origin in Bose–Einstein condensation. Ultracold quantum gases have provided condensates close to the original ideas of Bose and Einstein, while condensation of polaritons and magnons has introduced novel concepts of non-equilibrium condensation. Here, we demonstrate a Bose–Einstein condensate of surface plasmon polaritons in lattice modes of a metal nanoparticle array. Interaction of the nanoscale-confined surface plasmons with a room-temperature bath of dye molecules enables thermalization and condensation in picoseconds. The ultrafast thermalization and condensation dynamics are revealed by an experiment that exploits thermalization under propagation and the open-cavity character of the system. A crossover from a Bose–Einstein condensate to usual lasing is realized by tailoring the band structure. This new condensate of surface plasmon lattice excitations has promise for future technologies due to its ultrafast, room-temperature and on-chip nature.

This is a preview of subscription content, access via your institution

Access options

Buy this article

  • Purchase on Springer Link
  • Instant access to full article PDF
Buy now

Prices may be subject to local taxes which are calculated during checkout

Fig. 1: The system consists of a gold nanoparticle array with an energy dispersion, overlaid with dye molecules.
Fig. 2: Spectral and spatial evolution of the sample luminescence shows a crossover from thermalization to lasing to BEC.
Fig. 3: Momentum and energy distributions show accumulation of population to the band edge.
Fig. 4: Interference experiments show spatial coherence.

Similar content being viewed by others

References

  1. Griffin, A., Snoke, D. & Stringari, S. Bose–Einstein Condensation (Cambridge Univ. Press, Cambridge, 1995).

  2. Lee, P. A., Nagaosa, N. & Wen, X.-G. Doping a Mott insulator: Physics of high-temperature superconductivity. Rev. Mod. Phys. 78, 17–85 (2006).

    ADS  Google Scholar 

  3. Zwerger, W. The BCS–BEC Crossover and the Unitary Fermi Gas (Springer, Berlin, 2012).

  4. Volovik, G. The Universe in a Helium Droplet (Oxford Univ. Press, Oxford, 2003).

  5. Anderson, M. H., Ensher, J. R., Matthews, M. R., Wieman, C. E. & Cornell, E. Observation of Bose–Einstein condensation in a dilute atomic vapor. Science 269, 198–201 (1995).

    ADS  Google Scholar 

  6. Davis, K. B. et al. Bose–Einstein condensation in a gas of sodium atoms. Phys. Rev. Lett. 75, 3969–3973 (1995).

    ADS  Google Scholar 

  7. Bradley, C. C., Sackett, C. A., Tollett, J. J. & Hulet, R. G. Evidence of Bose–Einstein condensation in an atomic gas with attractive interactions. Phys. Rev. Lett. 75, 1687–1690 (1995).

    ADS  Google Scholar 

  8. Imamoglu, A., Ram, R. J., Pau, S. & Yamamoto, Y. Nonequilibrium condensates and lasers without inversion: Exciton-polariton lasers. Phys. Rev. A 53, 4250–4253 (1996).

    ADS  Google Scholar 

  9. Deng, H., Haug, H. & Yamamoto, Y. Exciton-polariton Bose–Einstein condensation. Rev. Mod. Phys. 82, 1489–1537 (2010).

    ADS  Google Scholar 

  10. Carusotto, I. & Ciuti, C. Quantum fluids of light. Rev. Mod. Phys. 85, 299–366 (2013).

    ADS  Google Scholar 

  11. Sieberer, L. M., Huber, S. D., Altman, E. & Diehl, S. Dynamical critical phenomena in driven-dissipative systems. Phys. Rev. Lett. 110, 195301 (2013).

    ADS  Google Scholar 

  12. Byrnes, T., Kim, N. Y. & Yamamoto, Y. Exciton-polariton condensates. Nat. Phys. 10, 803–813 (2014).

    Google Scholar 

  13. Deng, H., Weihs, G., Santori, C., Bloch, J. & Yamamoto, Y. Condensation of semiconductor microcavity exciton polaritons. Science 298, 199–202 (2002).

    ADS  Google Scholar 

  14. Kasprzak, J. et al. Bose–Einstein condensation of exciton polaritons. Nature 443, 409–414 (2006).

    ADS  Google Scholar 

  15. Balili, R., Hartwell, V., Snoke, D., Pfeiffer, L. & West, K. Bose–Einstein condensation of microcavity polaritons in a trap. Science 316, 1007–1010 (2007).

    ADS  Google Scholar 

  16. Baumberg, J. J. et al. Spontaneous polarization buildup in a room-temperature polariton laser. Phys. Rev. Lett. 101, 136409 (2008).

    ADS  Google Scholar 

  17. Amo, A. et al. Collective fluid dynamics of a polariton condensate in a semiconductor microcavity. Nature 457, 291–295 (2009).

    ADS  Google Scholar 

  18. Daskalakis, K. S., Maier, S. A., Murray, R. & Kéna-Cohen, S. Nonlinear interactions in an organic polariton condensate. Nat. Mater. 13, 271–278 (2014).

    ADS  Google Scholar 

  19. Plumhof, J. D., Stöferle, T., Mai, L., Scherf, U. & Mahrt, R. F. Room-temperature Bose–Einstein condensation of cavity exciton-polaritons in a polymer. Nat. Mater. 13, 247–252 (2014).

    ADS  Google Scholar 

  20. Lerario, G. et al. Room-temperature superfluidity in a polariton condensate. Nat. Phys. 13, 837–841 (2017).

    Google Scholar 

  21. Berloff, N. G. et al. Realizing the classical XY Hamiltonian in polariton simulators. Nat. Mater. 16, 1120–1126 (2017).

    ADS  Google Scholar 

  22. Caputo, D. et al. Topological order and thermal equilibrium in polariton condensates. Nat. Mater. 17, 145–151 (2017).

    ADS  Google Scholar 

  23. Demokritov, S. O. et al. Bose–Einstein condensation of quasi-equilibrium magnons at room temperature under pumping. Nature 443, 430–433 (2006).

    ADS  Google Scholar 

  24. Giamarchi, T., Rüegg, C. & Tchernyshyov, O. Bose–Einstein condensation in magnetic insulators. Nat. Phys. 4, 198–204 (2008).

    Google Scholar 

  25. Klaers, J., Schmitt, J., Vewinger, F. & Weitz, M. Bose–Einstein condensation of photons in an optical microcavity. Nature 468, 545–548 (2010).

    ADS  Google Scholar 

  26. Marelic, J. et al. Spatiotemporal coherence of non-equilibrium multimode photon condensates. New J. Phys. 18, 103012 (2016).

    ADS  Google Scholar 

  27. Zou, S., Janel, N. & Schatz, G. C. Silver nanoparticle array structures that produce remarkably narrow plasmon lineshapes. J. Chem. Phys. 120, 10871–10875 (2004).

    ADS  Google Scholar 

  28. García de Abajo, F. J. Colloquium: Light scattering by particle and hole arrays. Rev. Mod. Phys. 79, 1267–1290 (2007).

    ADS  Google Scholar 

  29. Auguié, B. & Barnes, W. L. Collective resonances in gold nanoparticle arrays. Phys. Rev. Lett. 101, 143902 (2008).

    ADS  Google Scholar 

  30. Rodriguez, S. R. K., Feist, J., Verschuuren, M. A., Garcia Vidal, F. J. & Gómez Rivas, J. Thermalization and cooling of plasmon-exciton polaritons: towards quantum condensation. Phys. Rev. Lett. 111, 166802 (2013).

    ADS  Google Scholar 

  31. Martikainen, J.-P., Heikkinen, M. O. J. & Törmä, P. Condensation phenomena in plasmonics. Phys. Rev. A 90, 053604 (2014).

    ADS  Google Scholar 

  32. Schmitt, J. et al. Thermalization kinetics of light: From laser dynamics to equilibrium condensation of photons. Phys. Rev. A 92, 011602(R) (2015).

    ADS  Google Scholar 

  33. Khurgin, J. B. Ultimate limit of field confinement by surface plasmon polaritons. Faraday Discuss. 178, 109–122 (2015).

    ADS  Google Scholar 

  34. Maier, S. A. et al. Plasmonics—a route to nanoscale optical devices. Adv. Mater. 13, 1501–1505 (2001).

    Google Scholar 

  35. Novotny, L. & van Hulst, N. Antennas for light. Nat. Photon. 5, 83–90 (2011).

    ADS  Google Scholar 

  36. Zhou, W. et al. Lasing action in strongly coupled plasmonic nanocavity arrays. Nat. Nanotechnol. 8, 506–511 (2013).

    ADS  Google Scholar 

  37. Hakala, T. K. et al. Lasing in dark and bright modes of a finite-sized plasmonic lattice. Nat. Commun. 8, 13687 (2017).

    ADS  Google Scholar 

  38. Ramezani, M. et al. Plasmon-exciton-polariton lasing. Optica 4, 31–37 (2017).

    ADS  Google Scholar 

  39. Törmä, P. & Barnes, W. L. Strong coupling between surface plasmon polaritons and emitters: a review. Rep. Progress Phys. 78, 013901 (2015).

    ADS  Google Scholar 

  40. Dridi, M. & Schatz, G. C. Model for describing plasmon-enhanced lasers that combines rate equations with finite-difference time-domain. J. Opt. Soc. Am. B 30, 2791–2797 (2013).

    ADS  Google Scholar 

  41. Kirton, P. & Keeling, J. Nonequilibrium model of photon condensation. Phys. Rev. Lett. 111, 100404 (2013).

    ADS  Google Scholar 

  42. Chiocchetta, A., Gambassi, A. & Carusotto, I. in Universal Themes of Bose–Einstein Condensation (eds Proukakis, N. P., Snoke D. W. & Littlewood, P. B.) (Cambridge Univ. Press, Cambridge, 2017).

  43. Bajoni, D., Senellart, P., Lematre, A. & Bloch, J. Photon lasing in GaAs microcavity: Similarities with a polariton condensate. Phys. Rev. B 76, 201305 (2007).

    ADS  Google Scholar 

  44. Ketterle, W. & van Druten, N. J. Bose–Einstein condensation of a finite number of particles trapped in one or three dimensions. Phys. Rev. A 54, 656–660 (1996).

    ADS  Google Scholar 

  45. Kosterlitz, J. M. Kosterlitz–Thouless physics: a review of key issues. Rep. Progress Phys. 79, 026001 (2016).

    ADS  Google Scholar 

  46. Altman, E., Sieberer, L. M., Chen, L., Diehl, S. & Toner, J. Two-dimensional superfluidity of exciton polaritons requires strong anisotropy. Phys. Rev. X 5, 011017 (2015).

    Google Scholar 

  47. Chikkaraddy, R. et al. Single-molecule strong coupling at room temperature in plasmonic nanocavities. Nature 535, 127–130 (2016).

    ADS  Google Scholar 

  48. Guo, R., Hakala, T. K. & Törmä, P. Geometry dependence of surface lattice resonances in plasmonic nanoparticle arrays. Phys. Rev. B 95, 155423 (2017).

    ADS  Google Scholar 

  49. Hasan, M. Z. & Kane, C. L. Colloquium: Topological insulators. Rev. Mod. Phys. 82, 3045–3067 (2010).

    ADS  Google Scholar 

  50. Kataja, M. et al. Surface lattice resonances and magneto-optical response in magnetic nanoparticle arrays. Nat. Commun. 6, 7072 (2015).

    ADS  Google Scholar 

  51. Julku, A. Condensation of Surface Lattice Resonance Excitations. MSc thesis, Aalto Univ. (2015).

  52. Moilanen, A. J. Dispersion Relation and Density of States for Surface Lattice Resonance Excitations. MSc thesis, Aalto Univ. (2016).

Download references

Acknowledgements

We thank M. Heikkinen, D.-H. Kim, R. Moerland and M. Nečada for useful discussions. This work is dedicated in memory of D. Jin and her inspiring example. This work was supported by the Academy of Finland through its Centres of Excellence Programme (2012–2017) and under project numbers 284621, 303351 and 307419, and by the European Research Council (ERC-2013-AdG-340748-CODE). This article is based on work from COST Action MP1403 Nanoscale Quantum Optics, supported by COST (European Cooperation in Science and Technology). K.S.D. acknowledges financial support by a Marie Skłodowska-Curie Action (H2020-MSCA-IF-2016, project id 745115). Part of the research was performed at the Micronova Nanofabrication Centre, supported by Aalto University. The Triton cluster at Aalto University was used for the computations.

Author information

Author notes

  1. These authors contributed equally: Tommi K. Hakala and Antti J. Moilanen.

Authors and Affiliations

  1. COMP Centre of Excellence, Department of Applied Physics, Aalto University School of Science, Aalto, Finland

    Tommi K. Hakala, Antti J. Moilanen, Aaro I. Väkeväinen, Rui Guo, Jani-Petri Martikainen, Konstantinos S. Daskalakis, Heikki T. Rekola, Aleksi Julku & Päivi Törmä

Authors
  1. Tommi K. Hakala
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Antti J. Moilanen
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Aaro I. Väkeväinen
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Rui Guo
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Jani-Petri Martikainen
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Konstantinos S. Daskalakis
    View author publications

    You can also search for this author in PubMed Google Scholar

  7. Heikki T. Rekola
    View author publications

    You can also search for this author in PubMed Google Scholar

  8. Aleksi Julku
    View author publications

    You can also search for this author in PubMed Google Scholar

  9. Päivi Törmä
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

P.T. initiated and supervised the project. T.K.H., A.J.M., R.G. and A.I.V. performed the experiments. A.J.M., T.K.H. and A.I.V. analysed the data. T.K.H., K.S.D. and H.T.R. built the experimental set-up. A.J.M., J.-P.M. and A.J. performed the theoretical modelling. R.G. fabricated the samples. All authors discussed the results. P.T., A.J.M. and T.K.H. wrote the manuscript together with all authors.

Corresponding author

Correspondence to Päivi Törmä.

Ethics declarations

Competing interests

The authors declare no competing interests.

Additional information

Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Supplementary information

Supplementary Information

Supplementary Figure 1–14, Supplementary References

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Hakala, T.K., Moilanen, A.J., Väkeväinen, A.I. et al. Bose–Einstein condensation in a plasmonic lattice. Nature Phys 14, 739–744 (2018). https://doi.org/10.1038/s41567-018-0109-9

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/s41567-018-0109-9

This article is cited by

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing