Abstract
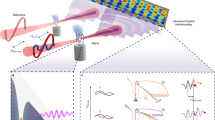
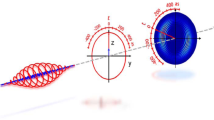
Quantum particles can penetrate potential barriers by tunnelling1. If that barrier is rotating, the tunnelling process is modified2,3. This is typical for electrons in atoms, molecules or solids exposed to strong circularly polarized laser pulses4,5,6. Here we measure how the transmission probability through a rotating tunnel depends on the sign of the magnetic quantum number m of the electron and thus on the initial direction of rotation of its quantum phase. We further show that our findings agree with a semiclassical picture, in which the electron keeps part of that rotary motion on its way through the tunnel by measuring m-dependent modification of the electron emission pattern. These findings are relevant for attosecond metrology as well as for interpretation of strong-field electron emission from atoms and molecules7,8,9,10,11,12,13,14 and directly demonstrate the creation of ring currents in bound states of ions with attosecond precision. In solids, this could open a way to inducing and controlling ring-current-related topological phenomena15.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout



Similar content being viewed by others
References
Keldysh, L. V. Ionization in the field of a strong electromagnetic wave. Sov. Phys. JETP 20, 1307–1314 (1965).
Ivanov, M., Spanner, M. & Smirnova, O. Anatomy of strong field ionization. J. Mod. Opt. 52, 165–184 (2005).
Yudin, G. & Ivanov, M. Nonadiabatic tunnel ionization: Looking inside a laser cycle. Phys. Rev. A 64, 13409 (2001).
Barth, I. & Smirnova, O. Nonadiabatic tunneling in circularly polarized laser fields: Physical picture and calculations. Phys. Rev. A 84, 63415 (2011).
Kaushal, J. & Smirnova, O. Nonadiabatic Coulomb effects in strong-field ionization in circularly polarized laser fields. Phys. Rev. A 88, 13421 (2013).
Zhu, X. et al. Helicity sensitive enhancement of strong-field ionization in circularly polarized laser fields. Opt. Express 24, 4196–4209 (2016).
Kaushal, J., Morales, F. & Smirnova, O. Opportunities for detecting ring currents using an attoclock setup. Phys. Rev. A 92, 63405 (2015).
Landsman, A. S. & Keller, U. Attosecond science and the tunnelling time problem. Phys. Rep. 547, 1–24 (2015).
Shafir, D. et al. Resolving the time when an electron exits a tunnelling barrier. Nature 485, 343–346 (2012).
Pedatzur, O. et al. Attosecond tunnelling interferometry. Nat. Phys. 11, 815–819 (2015).
Torlina, L. et al. Interpreting attoclock measurements of tunnelling times. Nat. Phys. 11, 503–508 (2015).
Garg, M. et al. Multi-petahertz electronic metrology. Nature 538, 359–363 (2016).
Eckle, P. et al. Attosecond ionization and tunneling delay time measurements in helium. Science 322, 1525–1529 (2008).
Hassan, M. T. et al. Optical attosecond pulses and tracking the nonlinear response of bound electrons. Nature 530, 66–70 (2016).
Rechtsman, M. et al. Photonic Floquet topological insulators. Nature 496, 196–200 (2013).
Barth, I. & Manz, J. Electric ring currents in atomic orbitals and magnetic fields induced by short intense circularly polarized π laser pulses. Phys. Rev. A 75, 12510 (2007).
Fleischer, A. et al. Probing angular correlations in sequential double ionization. Phys. Rev. Lett. 107, 113003 (2011).
Fechner, L., Camus, N., Ullrich, J., Pfeifer, T. & Moshammer, R. Strong-field tunneling from a coherent superposition of electronic states. Phys. Rev. Lett. 112, 213001 (2014).
Barth, I. & Smirnova, O. Hole dynamics and spin currents after ionization in strong circularly polarized laser fields. J. Phys. B 47, 204020 (2014).
Barth, I. & Smirnova, O. Spin-polarized electrons produced by strong-field ionization. Phys. Rev. A 88, 13401 (2013).
Li, Y. et al. Nonadiabatic tunnel ionization in strong circularly polarized laser fields: counterintuitive angular shifts in the photoelectron momentum distribution. Opt. Express 23, 28801–28807 (2015).
Hartung, A. et al. Electron spin polarization in strong-field ionization of xenon atoms. Nat. Photon. 10, 526–528 (2016).
Herath, T., Yan, L., Lee, S. K. & Li, W. Strong-field ionization rate depends on the sign of the magnetic quantum number. Phys. Rev. Lett. 109, 43004 (2012).
Torlina, L. & Smirnova, O. Time-dependent analytical R-matrix approach for strong-field dynamics. I. One-electron systems. Phys. Rev. A 86, 43408 (2012).
Torlina, L., Kaushal, J. & Smirnova, O. Time-resolving electron-core dynamics during strong-field ionization in circularly polarized fields. Phys. Rev. A 88, 53403 (2013).
Torlina, L., Morales, F., Muller, H. G. & Smirnova, O. Ab initio verification of the analytical R-matrix theory for strong field ionization. J. Phys. B 47, 204021 (2014).
Jagutzki, O. et al. Multiple hit readout of a microchannel plate detector with a three-layer delay-line anode. IEEE Trans. Nucl. Sci. 49, 2477–2483 (2002).
Ullrich, J. et al. Recoil-ion and electron momentum spectroscopy: reaction-microscopes. Rep. Prog. Phys. 66, 1463–1545 (2003).
Eckart, S. et al. Nonsequential double ionization by counterrotating circularly polarized two-color laser fields. Phys. Rev. Lett. 117, 133202 (2016).
Pfeiffer, A. N. et al. Calculation of valence electron motion induced by sequential strong-field ionisation. Mol. Phys. 111, 2283–2291 (2013).
Nandor, M. J., Walker, M. A., Van Woerkom, L. D. & Muller, H. G. Detailed comparison of above-threshold-ionization spectra from accurate numerical integrations and high-resolution measurements. Phys. Rev. A 60, R1771–R1774 (1999).
Muller, H. G. An efficient propagation scheme for the time-dependent Schrodinger equation in the velocity gauge. Laser Phys. 9, 138–148 (1999).
Shvetsov-Shilovski, N. I. et al. Semiclassical two-step model for strong-field ionization. Phys. Rev. A 94, 13415 (2016).
Delone, N. B. & Krainov, V. P. Energy and angular electron spectra for the tunnel ionization of atoms by strong low-frequency radiation. J. Opt. Soc. Am. B 8, 1207–1211 (1991).
Lemell, C. et al. Low-energy peak structure in strong-field ionization by midinfrared laser pulses: Two-dimensional focusing by the atomic potential. Phys. Rev. A 85, 11403 (2012).
Eckle, P. et al. Attosecond angular streaking. Nat. Phys. 4, 565–570 (2008).
Pfeiffer, A. N. et al. Attoclock reveals natural coordinates of the laser-induced tunnelling current flow in atoms. Nat. Phys. 8, 76–80 (2012).
Bruner, B. D. et al. Multidimensional high harmonic spectroscopy of polyatomic molecules: detecting sub-cycle laser-driven hole dynamics upon ionization in strong mid-IR laser fields. Faraday Discuss. 194, 369–405 (2016).
Torlina, L. & Smirnova, O. Coulomb time delays in high harmonic generation. New J. Phys. 19, 23012 (2017).
Acknowledgements
This work was supported by the DFG Priority Programme ‘Quantum Dynamics in Tailored Intense Fields’ (projects DO 604/29-1, BA 5623/1-1, IV 156/6-1, SM 292/5-1).
Author information
Authors and Affiliations
Contributions
S.E., M.K., M.R., A.H., J.R., F.T., K.F., N.S., K.H., L.Ph.H.S., T.J., M.S. and R.D. contributed to the experiment. S.E., M.K., M.R., K.L., I.B., J.K., F.M., M.I., O.S. and R.D. contributed to the theoretical results. S.E., M.K., T.J., M.S. and R.D. performed the analysis of the experimental data. All authors contributed to the manuscript.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Supplementary Figures
Supplementary Figures 1–4
Rights and permissions
About this article
Cite this article
Eckart, S., Kunitski, M., Richter, M. et al. Ultrafast preparation and detection of ring currents in single atoms. Nature Phys 14, 701–704 (2018). https://doi.org/10.1038/s41567-018-0080-5
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41567-018-0080-5
This article is cited by
-
Tunnelling of electrons via the neighboring atom
Light: Science & Applications (2024)
-
Spatiotemporal imaging and shaping of electron wave functions using novel attoclock interferometry
Nature Communications (2024)
-
Geometric magnetism and anomalous enantio-sensitive observables in photoionization of chiral molecules
Communications Physics (2023)
-
Attosecond circular-dichroism chronoscopy of electron vortices
Nature Physics (2022)
-
A look under the tunnelling barrier via attosecond-gated interferometry
Nature Photonics (2022)