Abstract
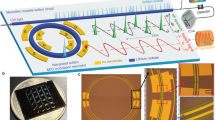
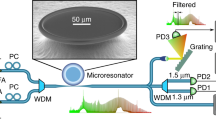
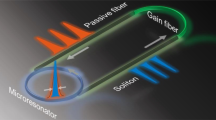
Microcavity-based frequency combs, or ‘microcombs’1,2, have enabled many fundamental breakthroughs3,4,5,6,7,8,9,10,11,12,13,14,15,16,17,18,19,20,21 through the discovery of temporal cavity-solitons. These self-localized waves, described by the Lugiato–Lefever equation22, are sustained by a background of radiation usually containing 95% of the total power23. Simple methods for their efficient generation and control are currently being investigated to finally establish microcombs as out-of-the-lab tools24. Here, we demonstrate microcomb laser cavity-solitons. Laser cavity-solitons are intrinsically background-free and have underpinned key breakthroughs in semiconductor lasers22,25,26,27,28. By merging their properties with the physics of multimode systems29, we provide a new paradigm for soliton generation and control in microcavities. We demonstrate 50-nm-wide bright soliton combs induced at average powers more than one order of magnitude lower than the Lugiato–Lefever soliton power threshold22, measuring a mode efficiency of 75% versus the theoretical limit of 5% for bright Lugiato–Lefever solitons23. Finally, we can tune the repetition rate by well over a megahertz without any active feedback.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout





Similar content being viewed by others
Data availability
The data that support the plots within this paper and other findings of this study are available from the corresponding author upon reasonable request.
References
Kippenberg, T. J., Holzwarth, R. & Diddams, S. A. Microresonator-based optical frequency combs. Science 332, 555–559 (2011).
Pasquazi, A. et al. Micro-combs: a novel generation of optical sources. Phys. Rep. 729, 1–81 (2017).
Suh, M.-G., Yang, Q.-F., Yang, K. Y., Yi, X. & Vahala, K. J. Microresonator soliton dual-comb spectroscopy. Science 354, 600–603 (2016).
Yu, M. et al. Silicon-chip-based mid-infrared dual-comb spectroscopy. Nat. Commun. 9, 1869 (2018).
Marin-Palomo, P. et al. Microresonator-based solitons for massively parallel coherent optical communications. Nature 546, 274–279 (2017).
Pfeifle, J. et al. Optimally coherent Kerr combs generated with crystalline whispering gallery mode resonators for ultrahigh capacity fiber communications. Phys. Rev. Lett. 114, 093902 (2015).
Liang, W. et al. High spectral purity Kerr frequency comb radio frequency photonic oscillator. Nat. Commun. 6, 7957 (2015).
Spencer, D. T. et al. An optical-frequency synthesizer using integrated photonics. Nature 557, 81–85 (2018).
Trocha, P. et al. Ultrafast optical ranging using microresonator soliton frequency combs. Science 359, 887–891 (2018).
Suh, M.-S. & Vahala, K. J. Soliton microcomb range measurement. Science 359, 884–887 (2018).
Kues, M. et al. On-chip generation of high-dimensional entangled quantum states and their coherent control. Nature 546, 622–626 (2017).
Reimer, C. et al. Generation of multiphoton entangled quantum states by means of integrated frequency combs. Science 351, 1176–1180 (2016).
Brasch, V. et al. Photonic chip–based optical frequency comb using soliton Cherenkov radiation. Science 351, 357–360 (2016).
Del’Haye, P. et al. Phase-coherent microwave-to-optical link with a self-referenced microcomb. Nat. Photon. 10, 516–520 (2016).
Suh, M.-G. et al. Searching for exoplanets using a microresonator astrocomb. Nat. Photon. 13, 25–30 (2019).
Obrzud, E. et al. A microphotonic astrocomb. Nat. Photon. 13, 31–35 (2019).
Haelterman, M., Trillo, S. & Wabnitz, S. Dissipative modulation instability in a nonlinear dispersive ring cavity. Opt. Commun. 91, 401–407 (1992).
Leo, F. et al. Temporal cavity-solitons in one-dimensional Kerr media as bits in an all-optical buffer. Nat. Photon. 4, 471–476 (2010).
Herr, T. et al. Temporal solitons in optical microresonators. Nat. Photon. 8, 145–152 (2013).
Xue, X. et al. Mode-locked dark pulse Kerr combs in normal-dispersion microresonators. Nat. Photon. 9, 594–600 (2015).
Cole, D. C., Lamb, E. S., Del’Haye, P., Diddams, S. A. & Papp, S. B. Soliton crystals in Kerr resonators. Nat. Photon. 11, 671–676 (2017).
Lugiato, L. A., Prati, F. & Brambilla, M. Nonlinear Optical Systems (Cambridge Univ. Press, Cambridge, 2015).
Xue, X., Wang, P. ‐H., Xuan, Y., Qi, M. & Weiner, A. M. Microresonator Kerr frequency combs with high conversion efficiency. Laser Photon. Rev. 11, 1600276 (2017).
Yao, B. et al. Gate-tunable frequency combs in graphene–nitride microresonators. Nature 558, 410–414 (2018).
Tanguy, Y., Ackemann, T., Firth, W. J. & Jäger, R. Realization of a semiconductor-based cavity soliton laser. Phys. Rev. Lett. 100, 013907 (2008).
Barland, S. et al. Cavity-solitons as pixels in semiconductor microcavities. Nature 419, 699–702 (2002).
Genevet, P., Barland, S., Giudici, M. & Tredicce, J. R. Cavity soliton laser based on mutually coupled semiconductor microresonators. Phys. Rev. Lett. 101, 123905 (2008).
Marconi, M., Javaloyes, J., Balle, S. & Giudici, M. How lasing localized structures evolve out of passive mode locking. Phys. Rev. Lett. 112, 223901 (2014).
Wright, L. G., Christodoulides, D. N. & Wise, F. W. Spatio-temporal mode-locking in multimode fiber lasers. Science 358, 94–97 (2017).
Grelu, P. & Akhmediev, N. Dissipative solitons for mode-locked lasers. Nat. Photon. 6, 84–92 (2012).
Stern, B., Ji, X., Okawachi, Y., Gaeta, A. L. & Lipson, M. Battery-operated integrated frequency comb generator. Nature 562, 401–405 (2018).
Obrzud, E., Lecomte, S. & Herr, T. Temporal solitons in microresonators driven by optical pulses. Nat. Photon. 11, 600–607 (2017).
Yang, Q.-F., Yi, X., Yang, K. Y. & Vahala, K. Counter-propagating solitons in microresonators. Nat. Photon. 11, 560–564 (2017).
Miller, S. A. et al. Tunable frequency combs based on dual microring resonators. Opt. Express 23, 21527–21540 (2015).
Gustave, F. et al. Observation of mode-locked spatial laser solitons. Phys. Rev. Lett. 118, 044102 (2017).
Krupa, K. et al. Spatial beam self-cleaning in multimode fibres. Nat. Photon. 11, 237–241 (2017).
Scroggie, A. J., Firth, W. J. & Oppo, G.-L. Cavity-soliton laser with frequency selective feedback. Phys. Rev. A 80, 013829 (2009).
Peccianti, M. et al. Demonstration of a stable ultrafast laser based on a nonlinear microcavity. Nat. Commun. 3, 765 (2012).
Wang, W. et al. Repetition rate multiplication pulsed laser source based on a microring resonator. ACS Photon. 4, 1677–1683 (2017).
Bao, H. et al. Type-II micro-comb generation in a filter-driven four wave mixing laser. Photon. Res. 6, B67–B73 (2018).
Andral, U. et al. Fiber laser mode locked through an evolutionary algorithm. Optica 2, 275–278 (2015).
Haboucha, A., Leblond, H., Salhi, M., Komarov, A. & Sanchez, F. Analysis of soliton pattern formation in passively mode-locked fiber lasers. Phys. Rev. A 78, 043806 (2008).
Del’Haye, P., Arcizet, O., Gorodetsky, M. L., Holzwarth, R. & Kippenberg, T. J. Frequency comb assisted diode laser spectroscopy for measurement of microcavity dispersion. Nat. Photon. 3, 529–533 (2009).
Acknowledgements
The authors acknowledge support from the UK Quantum Technology Hub for Sensors and Metrology, EPSRC, under grant no. EP/M013294/1 and from INNOVATE UK, project ‘IOTA’ grant agreement no. EP/R043566/1. This project received funding from the European Research Council (ERC) under the European Union’s Horizon 2020 Research and Innovation Programme (grant no. 725046). A.P. acknowledges support from the People Programme (Marie Curie Actions) of the European Union’s FP7 Programme under REA grant agreement CHRONOS (327627). B.W. acknowledges support from the People Programme (Marie Curie Actions) of the European Union’s FP7 Programme under REA grant agreement INCIPIT (PIOF-GA-2013-625466). S.T.C. acknowledges support from the Research Grant Council of Hong Kong (GRF# 9042663). B.E.L. acknowledges support from the Strategic Priority Research Program of the Chinese Academy of Sciences (grant no. XDB24030300). R.M. acknowledges funding from the Natural Sciences and Engineering Research Council of Canada (NSERC) through the Strategic, Discovery and Acceleration Grants Schemes, by the MESI PSR-SIIRI Initiative in Quebec, by the Canada Research Chair Program, as well as additional support by the Government of the Russian Federation through the ITMO Fellowship and Professorship Program (grant no. 074-U 01) and by the 1000 Talents Sichuan Program.
Author information
Authors and Affiliations
Contributions
A.P., H.B. and M.P. developed the original concept. B.E.L. and S.T.C. designed and fabricated the integrated devices. H.B performed the experiments. A.P. developed the theoretical model. A.C., M.R., L.D.L., J.S.T.G., G.-L.O., D.J.M., R.M. and B.W. contributed to the development of the experiment, the numerical model and the data analysis. A.P., B.W., G.-L.O., D.J.M., R.M., H.B. and M.P. contributed to the writing of the manuscript. B.W., M.P. and A.P. supervised the research.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Supplementary Information
This file contains more information about the work, Supplementary Figures 1–8 and Supplementary Tables 1–2.
Rights and permissions
About this article
Cite this article
Bao, H., Cooper, A., Rowley, M. et al. Laser cavity-soliton microcombs. Nat. Photonics 13, 384–389 (2019). https://doi.org/10.1038/s41566-019-0379-5
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41566-019-0379-5
This article is cited by
-
Turnkey photonic flywheel in a microresonator-filtered laser
Nature Communications (2024)
-
Genetic algorithm-enhanced microcomb state generation
Communications Physics (2024)
-
Ultrashort dissipative Raman solitons in Kerr resonators driven with phase-coherent optical pulses
Nature Photonics (2024)
-
High Q-factor reconfigurable microresonators induced in side-coupled optical fibres
Light: Science & Applications (2023)
-
Universality of light thermalization in multimoded nonlinear optical systems
Nature Communications (2023)