Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

  • Article
  • Published:

Synchronization of coupled optical microresonators

Nature Photonics volume 12, pages 688–693 (2018)Cite this article

Subjects

Abstract

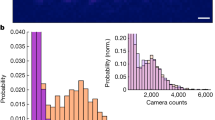
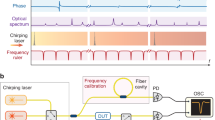
The phenomenon of synchronization occurs universally across the natural sciences and provides critical insight into the behaviour of coupled nonlinear dynamical systems. It also offers a powerful approach to robust frequency or temporal locking in diverse applications including communications, superconductors and photonics. Here, we report the experimental synchronization of two coupled soliton mode-locked chip-based frequency combs separated over distances of 20 m. We show that such a system obeys the universal Kuramoto model for synchronization and that the cavity solitons from the microresonators can be coherently combined, which overcomes the fundamental power limit of microresonator-based combs. This study could significantly expand the applications of microresonator combs, and with its capability for massive integration it offers a chip-based photonic platform for exploring complex nonlinear systems.

This is a preview of subscription content, access via your institution

Access options

Buy this article

  • Purchase on Springer Link
  • Instant access to full article PDF
Buy now

Prices may be subject to local taxes which are calculated during checkout

Fig. 1: Illustration of the synchronization of microresonator combs.
Fig. 2: Dynamics of synchronization.
Fig. 3: Characterization of synchronization behaviour.
Fig. 4: Demonstration of coherent comb combining.

Similar content being viewed by others

Data availability

The data that support the plots within this paper and other findings of this study are available from the corresponding author upon reasonable request.

References

  1. Strogatz, S. H. From Kuramoto to Crawford: exploring the onset of synchronization in populations of coupled oscillators. Physica D 143, 1–20 (2000).

    Article  ADS  MathSciNet  Google Scholar 

  2. Buck, J. Synchronous rhythmic flashing of fireflies. II. Q. Rev. Biol. 63, 265–289 (1988).

    Article  Google Scholar 

  3. Michaels, D. C., Matyas, E. P. & Jalife, J. Mechanisms of sinoatrial pacemaker synchronization: a new hypothesis. Circ. Res. 61, 704–714 (1987).

    Article  Google Scholar 

  4. Wiesenfeld, K., Colet, P. & Strogatz, S. H. Synchronization transitions in a disordered Josephson series array. Phys. Rev. Lett. 76, 404–407 (1996).

    Article  ADS  Google Scholar 

  5. York, R. A. & Compton, R. C. Quasi-optical power combining using mutually synchronized oscillator arrays. IEEE Trans. Microwave Theory Tech. 39, 1000–1009 (1991).

    Article  ADS  Google Scholar 

  6. Ramirez, J. P., Olvera, L. A., Nijmeijer, H. & Alvarez, J. The sympathy of two pendulum clocks: beyond Huygens observations. Sci. Rep. 6, 23580 (2016).

    Article  ADS  Google Scholar 

  7. Kuramoto, Y. Self-entrainment of a population of coupled non-linear oscillators. In Proc. Int. Symp. Mathematical Problems in Theoretical Physics (ed. Araki, H.) 420–422 (Springer, Berlin, 1975).

  8. Nixon, M. et al. Controlling synchronization in large laser networks. Phys. Rev. Lett. 108, 214101 (2012).

    Article  ADS  Google Scholar 

  9. Cheo, P. K., Liu, A. & King, G. G. A high-brightness laser beam from a phase-locked multicore Yb-doped fiber laser array. IEEE Photon. Technol. Lett. 13, 439–441 (2001).

    Article  ADS  Google Scholar 

  10. Udem, T., Holzwarth, R. & Hänsch, T. W. Optical frequency metrology. Nature 416, 233–237 (2002).

    Article  ADS  Google Scholar 

  11. Cundiff, S. T. & Ye, J. Colloquium: femtosecond optical frequency combs. Rev. Mod. Phys. 75, 325–342 (2003).

    Article  ADS  Google Scholar 

  12. Dudley, J. M., Genty, G. & Coen, S. Supercontinuum generation in photonic crystal fiber. Rev. Mod. Phys. 78, 1135 (2006).

    Article  ADS  Google Scholar 

  13. Newbury, N. R. Searching for applications with a fine-tooth comb.Nat. Photon. 5, 186–188 (2011).

    Article  ADS  Google Scholar 

  14. Del’Haye, P. et al. Optical frequency comb generation from a monolithic microresonator. Nature 450, 1214–1217 (2007).

    Article  ADS  Google Scholar 

  15. Savchenkov, A. A. et al. Tunable optical frequency comb with a crystalline whispering gallery mode resonator. Phys. Rev. Lett. 101, 093902 (2008).

    Article  ADS  Google Scholar 

  16. Levy, J. S. et al. CMOS-compatible multiple-wavelength oscillator for on-chip optical interconnects. Nat. Photon. 4, 37–40 (2010).

    Article  ADS  Google Scholar 

  17. Razzari, L. et al. CMOS-compatible integrated optical hyper-parametric oscillator. Nat. Photon. 4, 41–45 (2010).

    Article  ADS  Google Scholar 

  18. Herr, T. et al. Universal formation dynamics and noise of Kerr-frequency combs in microresonators. Nat. Photon. 6, 480–487 (2012).

    Article  ADS  Google Scholar 

  19. Leo, F., Gelens, L., Emplit, P., Haelterman, M. & Coen, S. Dynamics of one-dimensional Kerr cavity solitons. Opt. Express 21, 9180–9191 (2013).

    Article  ADS  Google Scholar 

  20. Parra-Rivas, P., Gomila, D., Matías, M. A., Coen, S. & Gelens, L. Dynamics of localized and patterned structures in the Lugiato–Lefever equation determine the stability and shape of optical frequency combs. Phys. Rev. A 89, 043813 (2014).

    Article  ADS  Google Scholar 

  21. Anderson, M., Leo, F., Coen, S., Erkintalo, M. & Murdoch, S. G. Observation of spatiotemporal instabilities of temporal cavity solitons. Optica 3, 1071–1074 (2016).

    Article  Google Scholar 

  22. Bao, C. et al. Observation of Fermi–Pasta–Ulam recurrence induced by breather solitons in an optical microresonator. Phys. Rev. Lett. 117, 163901 (2016).

    Article  ADS  Google Scholar 

  23. Yu, M. et al. Breather soliton dynamics in microresonators. Nat. Commun. 8, 14569 (2017).

    Article  ADS  Google Scholar 

  24. Lucas, E., Karpov, M., Guo, H., Gorodetsky, M. L. & Kippenberg, T. J. Breathing dissipative solitons in optical microresonators. Nat. Commun. 8, 736 (2017).

    Article  ADS  Google Scholar 

  25. Leo, F. et al. Temporal cavity solitons in one-dimensional Kerr media as bits in an all-optical buffer. Nat. Photon. 4, 471–476 (2010).

    Article  ADS  Google Scholar 

  26. Saha, K. et al. Modelocking and femtosecond pulse generation in chip-based frequency combs. Opt. Express 21, 1335–1343 (2013).

    Article  ADS  Google Scholar 

  27. Herr, T. et al. Temporal solitons in optical microresonators. Nat. Photon. 8, 145–152 (2014).

    Article  ADS  Google Scholar 

  28. Jang, J. K., Erkintalo, M., Coen, S. & Murdoch, S. G. Temporal tweezing of light through the trapping and manipulation of temporal cavity solitons. Nat. Commun. 6, 7370 (2015).

    Article  ADS  Google Scholar 

  29. Del’Haye, P. et al. Phase steps and resonator detuning measurements in microresonator frequency combs. Nat. Commun. 6, 5668 (2015).

    Article  Google Scholar 

  30. Liang, W. et al. High spectral purity Kerr frequency comb radio frequency photonic oscillator. Nat. Commun. 6, 7957 (2015).

    Article  Google Scholar 

  31. Yi, X., Yang, Q.-F., Yang, K. Y., Suh, M.-G. & Vahala, K. Soliton frequency comb at microwave rates in a high-Q silica microresonator. Optica 2, 1078–1085 (2015).

    Article  Google Scholar 

  32. Joshi, C. et al. Thermally controlled comb generation and soliton modelocking in microresonators. Opt. Lett. 41, 2565–2568 (2016).

    Article  ADS  Google Scholar 

  33. Wang, P.-H. et al. Intracavity characterization of micro-comb generation in the single-soliton regime. Opt. Express 24, 10890–10897 (2016).

    Article  ADS  Google Scholar 

  34. Webb, K. E., Erkintalo, M., Coen, S. & Murdoch, S. G. Experimental observation of coherent cavity soliton frequency combs in silica microspheres. Opt. Lett. 41, 4613–4616 (2016).

    Article  ADS  Google Scholar 

  35. Yang, Q.-F., Yi, X., Yang, K. Y. & Vahala, K. Counter-propagating solitons in microresonators. Nat. Photon. 11, 560–564 (2017).

    Article  Google Scholar 

  36. Joshi, C. et al. Counter-rotating cavity solitons in a silicon nitride microresonator. Opt. Lett. 43, 547–550 (2018).

    Article  ADS  Google Scholar 

  37. Papp, S. B., Del’Haye, P. & Diddams, S. A. Parametric seeding of a microresonator optical frequency comb. Opt. Express 21, 17615–17624 (2013).

    Article  ADS  Google Scholar 

  38. Obrzud, E., Lecomte, S. & Herr, T. Temporal solitons in microresonators driven by optical pulses. Nat. Photon. 11, 600–607 (2017).

    Article  Google Scholar 

  39. Wen, Y. H., Lamont, M. R. E. & Gaeta, A. L. Synchronization of multiple parametric frequency combs. OSA Technical Digest Paper FW1D.4 (2014).

  40. Munns, J. H. D., Walmsley, I. A. & Wen, Y. H. Novel interactions of dissipative Kerr solitons in nonlinear cavity networks. In Proc. European Conf. Lasers and Electro-Optics and European Quantum Electronics Conferences EF_1_1 (OSA, Washington DC, 2017).

  41. Lugiato, L. A. & Lefever, R. Spatial dissipative structures in passive optical systems. Phys. Rev. Lett. 58, 2209 (1987).

    Article  ADS  Google Scholar 

  42. Wabnitz, S. Suppression of interactions in a phase-locked soliton optical memory. Opt. Lett. 18, 601–603 (1993).

    Article  ADS  Google Scholar 

  43. Coen, S., Randle, H. G., Sylvestre, T. & Erkintalo, M. Modeling of octave-spanning Kerr frequency combs using a generalized mean-field Lugiato–Lefever model. Opt. Lett. 38, 37–39 (2013).

    Article  ADS  Google Scholar 

  44. Chembo, Y. K. & Menyuk, C. R. Spatiotemporal Lugiato–Lefever formalism for Kerr-comb generation in whispering-gallery-mode resonators. Phys. Rev. A 87, 053852 (2013).

    Article  ADS  Google Scholar 

  45. Levy, J. S. et al. High-performance silicon-nitride-based multiple-wavelength source. IEEE Photon. Technol. Lett. 24, 1375–1377 (2012).

    Article  ADS  Google Scholar 

  46. Marin-Palomo, P. et al. Microresonator-based solitons for massively parallel coherent optical communications. Nature 546, 274–279 (2017).

    Article  ADS  Google Scholar 

  47. Carmon, T., Yang, L. & Vahala, K. J. Dynamical thermal behavior and thermal self-stability of microcavities. Opt. Express 12, 4742–4750 (2004).

    Article  ADS  Google Scholar 

  48. Adler, R. A study of locking phenomena in oscillators. Proc. IRE 34, 351–357 (1946).

    Article  Google Scholar 

  49. Del’Haye, P., Beha, K., Papp, S. B. & Diddams, S. A. Self-injection locking and phase-locked states in microresonators-based optical frequency combs. Phys. Rev. Lett. 112, 043905 (2014).

    Article  ADS  Google Scholar 

  50. Taheri, H., Eftekhar, A. A., Wiesenfeld, K. & Adibi, A. Anatomy of phase locking in hyperparametric oscillations based on Kerr nonlinearity. IEEE Photon. J. 9, 6100911 (2017).

    Article  Google Scholar 

  51. Trebino, R. Frequency-Resolved Optical Gating: The Measurement of Ultrashort Laser Pulses (Springer, New York, 2000)..

    Book  Google Scholar 

  52. Xue, X. et al. Thermal tuning of Kerr frequency combs in silicon nitride microring resonators. Opt. Express 24, 687–698 (2016).

    Article  ADS  Google Scholar 

  53. Wen, Y. H., Lamont, M. R. E., Strogatz, S. H. & Gaeta, A. L. Self-organization in Kerr-cavity-soliton formation in parametric frequency combs. Phys. Rev. A 94, 063843 (2016).

    Article  ADS  Google Scholar 

  54. Taheri, H., Del’Haye, P., Eftekhar, A. A., Wiesenfeld, K. & Adibi, A. Self-synchronization phenomena in the Lugiato–Lefever equation. Phys. Rev. A 96, 013828 (2017).

    Article  ADS  Google Scholar 

  55. Ji, X. et al. Ultra-low-loss on-chip resonators with sub-milliwatt parametric oscillation threshold. Optica 4, 619–624 (2017).

    Article  Google Scholar 

Download references

Acknowledgements

This work was supported by the Air Force Office of Scientific Research (AFOSR) (grant no. FA9550-15-1-0303), the National Science Foundation (NSF) (grant no. CCF-1640108) and the Semiconductor Research Corporation (SRC) (grant no. SRS 2016-EP-2693-A). The authors also thank K. Bergman and R. Polster for lending the high-resolution optical spectrum analyser and autocorrelator.

Author information

Authors and Affiliations

  1. Department of Applied Physics and Applied Mathematics, Columbia University, New York, NY, USA

    Jae K. Jang, Alexander Klenner, Yoshitomo Okawachi & Alexander L. Gaeta

  2. School of Electrical and Computer Engineering, Cornell University, Ithaca, NY, USA

    Xingchen Ji

  3. Department of Electrical Engineering, Columbia University, New York, NY, USA

    Xingchen Ji & Michal Lipson

Authors
  1. Jae K. Jang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Alexander Klenner
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Xingchen Ji
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Yoshitomo Okawachi
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Michal Lipson
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Alexander L. Gaeta
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

J.K.J. and A.K. performed experiment. J.K.J carried out theoretical analysis and numerical simulation, and wrote the manuscript with inputs from all authors. J.K.J., A.K., Y.O. and A.L.G. contributed to the interpretation of data. X.J. fabricated the devices under the supervision of M.L. A.L.G. supervised the overall project.

Corresponding author

Correspondence to Alexander L. Gaeta.

Ethics declarations

Competing interests

The authors declare no competing interests.

Additional information

Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Supplementary information

Supplementary Information

This file contains supplementary figures and additional information about the work

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Jang, J.K., Klenner, A., Ji, X. et al. Synchronization of coupled optical microresonators. Nature Photon 12, 688–693 (2018). https://doi.org/10.1038/s41566-018-0261-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/s41566-018-0261-x

This article is cited by

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing