Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

  • Letter
  • Published:

Charge-order-enhanced capacitance in semiconductor moiré superlattices

Nature Nanotechnology volume 16, pages 1068–1072 (2021)Cite this article

Subjects

Abstract

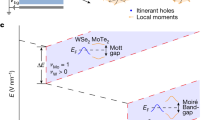
Van der Waals moiré materials have emerged as a highly controllable platform to study electronic correlation phenomena1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16,17. Robust correlated insulating states have recently been discovered at both integer and fractional filling factors of semiconductor moiré systems10,11,12,13,14,15,16,17. In this study we explored the thermodynamic properties of these states by measuring the gate capacitance of MoSe2/WS2 moiré superlattices. We observed a series of incompressible states for filling factors 0–8 and anomalously large capacitance in the intervening compressible regions. The anomalously large capacitance, which was nearly 60% above the device’s geometrical capacitance, was most pronounced at small filling factors, below the melting temperature of the charge-ordered states, and for small sample–gate separation. It is a manifestation of the device-geometry-dependent Coulomb interaction between electrons and phase mixing of the charge-ordered states. Based on these results, we were able to extract the thermodynamic gap of the correlated insulating states and the device’s electronic entropy and specific heat capacity. Our findings establish capacitance as a powerful probe of the correlated states in semiconductor moiré systems and demonstrate control of these states via sample–gate coupling.

This is a preview of subscription content, access via your institution

Access options

Buy this article

  • Purchase on Springer Link
  • Instant access to full article PDF
Buy now

Prices may be subject to local taxes which are calculated during checkout

Fig. 1: Charge-order-enhanced capacitance in MoSe2/WS2 moiré superlattices.
Fig. 2: Dependence of capacitance enhancement on sample–gate separation.
Fig. 3: Electronic entropy and specific heat capacity of device 4 (d/aM ≈ 0.6).

Similar content being viewed by others

Data availability

The data that support the findings of this study are available within the paper. Source data are provided with this paper.

References

  1. Cao, Y. et al. Correlated insulator behavior at half-filling in magic-angle graphene superlattices. Nature 556, 80–84 (2018).

    Article  CAS  Google Scholar 

  2. Cao, Y. et al. Unconventional superconductivity in magic-angle graphene superlattices. Nature 556, 43–50 (2018).

    Article  CAS  Google Scholar 

  3. Yankowitz, M. et al. Tuning superconductivity in twisted bilayer graphene. Science 363, 1059–1064 (2019).

    Article  CAS  Google Scholar 

  4. Tomarken, S. L. et al. Electronic compressibility of magic-angle graphene superlattices. Phys. Rev. Lett. 123, 046601 (2019).

    Article  CAS  Google Scholar 

  5. Chen, G. et al. Evidence of a gate-tunable Mott insulator in a trilayer graphene moiré superlattice. Nat. Phys. 15, 237–241 (2019).

    Article  CAS  Google Scholar 

  6. Lu, X. et al. Superconductors, orbital magnets and correlated states in magic-angle bilayer graphene. Nature 574, 653–657 (2019).

    Article  CAS  Google Scholar 

  7. Sharpe, A. L. et al. Emergent ferromagnetism near three-quarters filling in twisted bilayer graphene. Science 365, 605–608 (2019).

    Article  CAS  Google Scholar 

  8. Chen, G. et al. Tunable correlated Chern insulator and ferromagnetism in a moiré superlattice. Nature 579, 56–61 (2020).

    Article  CAS  Google Scholar 

  9. Serlin, M. et al. Intrinsic quantized anomalous Hall effect in a moiré heterostructure. Science 367, 900–903 (2020).

    Article  CAS  Google Scholar 

  10. Regan, E. C. et al. Mott and generalized Wigner crystal states in WSe2/WS2 moiré superlattices. Nature 579, 359–363 (2020).

    Article  CAS  Google Scholar 

  11. Tang, Y. et al. Simulation of Hubbard model physics in WSe2/WS2 moiré superlattices. Nature 579, 353–358 (2020).

    Article  CAS  Google Scholar 

  12. Shimazaki, Y. et al. Strong correlated electrons and hybrid excitons in a moiré heterostructure. Nature 580, 472–477 (2020).

    Article  CAS  Google Scholar 

  13. Wang, L. et al. Correlated electronic phases in twisted bilayer transition metal dichalcogenides. Nat. Mater. 19, 861–866 (2020).

    Article  CAS  Google Scholar 

  14. Chu, Z. et al. Nanoscale conductivity imaging of correlated electronic states in WSe2/WS2 moiré superlattices. Phys. Rev. Lett. 125, 186803 (2020).

    Article  CAS  Google Scholar 

  15. Xu, Y. et al. Correlated insulating states at fractional fillings of moiré superlattices. Nature 587, 214–218 (2020).

    Article  CAS  Google Scholar 

  16. Jin, C. et al. Stripe phases in WSe2/WS2 moiré superlattices. Nat. Mater. https://doi.org/10.1038/s41563-021-00959-8 (2021).

  17. Huang, X. et al. Correlated insulating states at fractional fillings of the WS2/WSe2 moiré lattices. Nat. Phys. https://doi.org/10.1038/s41567-021-01171-w (2021).

  18. Ando, T., Fowler, A. B. & Stern, F. Electronic properties of two-dimensional systems. Rev. Mod. Phys. 54, 437 (1982).

    Article  CAS  Google Scholar 

  19. Smith, T. P., Goldberg, B. B., Stiles, P. J. & Heiblum, M. Direct measurement of the density of states of a two-dimensional electron gas. Phys. Rev. B 32, 2696(R) (1985).

    Article  Google Scholar 

  20. Bello, M. S., Levin, E. I., Shklovskiĩ, B. I. & Éfros, A. L. Density of localized states in the surface impurity band of a metal-insulator-semiconductor structure. Sov. Phys. J. Exp. Theor. Phys. 53, 822 (1981).

    Google Scholar 

  21. Eisenstein, J. P., Pfeiffer, L. N. & West, K. W. Negative compressibility of interacting two-dimensional electron and quasiparticle gases. Phys. Rev. Lett. 68, 674 (1992).

    Article  CAS  Google Scholar 

  22. Li, L. et al. Very large capacitance enhancement in a two-dimensional electron system. Science 332, 825–828 (2011).

    Article  CAS  Google Scholar 

  23. Wu, Y. et al. Negative compressibility in graphene-terminated black phosphorus heterostructures. Phys. Rev. B 93, 035455 (2016).

    Article  Google Scholar 

  24. Zondiner, U. et al. Cascade of phase transition and Dirac revivals in magic-angle graphene. Nature 582, 203–208 (2020).

    Article  CAS  Google Scholar 

  25. Park, J. M., Cao, Y., Watanabe, K., Taniguchi, T. & Jarillo-Herrero, P. Flavour Hund’s coupling, Chern gaps, and charge diffusivity in moiré graphene. Nature 592, 43–48 (2021).

    Article  CAS  Google Scholar 

  26. Skinner, B. & Shklovskii, B. I. Anomalously large capacitance of a plane capacitor with a two-dimensional electron gas. Phys. Rev. B 82, 155111 (2010).

    Article  Google Scholar 

  27. Wu, F., Lovorn, T., Tutuc, E. & MacDonald, A. H. Hubbard model physics in transition metal dichalcogenide moiré bands. Phys. Rev. Lett. 121, 026402 (2018).

    Article  CAS  Google Scholar 

  28. Zhang, Y., Yuan, N. F. Q. & Fu, L. Moiré quantum chemistry: charge transfer in transition metal dichalcogenide superlattices. Phys. Rev. B 102, 201115(R) (2020).

    Article  Google Scholar 

  29. Pan, H., Wu, F. & Das Sarma, S. Quantum phase diagram of a Moiré-Hubbard model. Phys. Rev. B 102, 201104(R) (2020).

    Article  Google Scholar 

  30. Padhi, B., Chitra, R. & Phillips, P. W. Generalized Wigner crystallization in moiré materials. Phys. Rev. B 103, 125146 (2021).

    Article  CAS  Google Scholar 

  31. Meng, Y. et al. Electrical switching between exciton dissociation to exciton funneling in MoSe2/WS2 heterostructure. Nat. Commun. 11, 2640 (2020).

    Article  CAS  Google Scholar 

  32. Larentis, S. et al. Large effective mass and interaction-enhanced Zeeman splitting of K-valley electrons in MoSe2. Phys. Rev. B 97, 201407(R) (2018).

    Article  Google Scholar 

  33. Zhang, Z. et al. Flat bands in twisted bilayer transition metal dichalcogenides. Nat. Phys. 16, 1093–1096 (2020).

    Article  CAS  Google Scholar 

  34. Jin, C. et al. Observation of moiré excitons in WSe2/WS2 heterostructure superlattices. Nature 567, 76–80 (2019).

    Article  CAS  Google Scholar 

  35. Ruiz-Tijerina, D. A. & Fal’ko, V. I. Interlayer hybridization and moiré superlattice minibands for electrons and excitons in heterobilayers of transition-metal dichalcogenides. Phys. Rev. B 99, 125424 (2019).

    Article  CAS  Google Scholar 

  36. Li, H. et al. Imaging moiré flat bands in three-dimensional reconstructed WSe2/WS2 superlattices. Nat. Mater. https://doi.org/10.1038/s41563-021-00923-6 (2021).

  37. Wang, L. et al. One-dimensional electrical contact to a two-dimensional material. Science 342, 614–617 (2013).

    Article  CAS  Google Scholar 

  38. Ashoori, R. C. et al. Single-electron capacitance spectroscopy of discrete quantum levels. Phys. Rev. Lett. 68, 3088 (1992).

    Article  CAS  Google Scholar 

  39. Zibrov, A. A. et al. Tunable interacting composite fermion phases in a half-filled bilayer-graphene Landau level. Nature 549, 360–364 (2017).

    Article  CAS  Google Scholar 

  40. Shi, Q. et al. Odd- and even-denominator fractional quantum Hall states in monolayer WSe2. Nat. Nanotechnol. 15, 569–573 (2020).

    Article  CAS  Google Scholar 

  41. Saito, Y. et al. Isospin Pomeranchuk effect in twisted bilayer graphene. Nature 592, 220–224 (2021).

    Article  CAS  Google Scholar 

  42. Rozen, A. et al. Entropic evidence for a Pomeranchuk effect in magic-angle graphene. Nature 592, 214–219 (2021).

    Article  CAS  Google Scholar 

Download references

Acknowledgements

T.L. thanks Q. Shi and L. Ma for discussions on capacitance measurements. This work was supported by the US Department of Energy (DOE), Office of Science, Basic Energy Sciences (BES), under award number DE-SC0019481 (capacitance measurements), the National Science Foundation (Platform for the Accelerated Realization, Analysis, and Discovery of Interface Materials (PARADIM)) under cooperative agreement number DMR-1539918 (device fabrication) and the Air Force Office of Scientific Research Hybrid Materials MURI under award number FA9550-18-1-0480 (optical and device characterizations). This work was performed in part at Cornell NanoScale Facility, an NNCI member supported by NSF grant NNCI-1542081. Growth of the hBN crystals was supported by the Elemental Strategy Initiative of MEXT, Japan and CREST (JPMJCR15F3), JST. K.F.M. acknowledges support through a David and Lucille Packard Fellowship.

Author information

Authors and Affiliations

  1. School of Applied and Engineering Physics, Cornell University, Ithaca, NY, USA

    Tingxin Li, Jiacheng Zhu, Yanhao Tang, Jie Shan & Kin Fai Mak

  2. National Institute for Materials Science, Tsukuba, Japan

    Kenji Watanabe & Takashi Taniguchi

  3. Laboratory of Atomic and Solid State Physics, Cornell University, Ithaca, NY, USA

    Veit Elser, Jie Shan & Kin Fai Mak

  4. Kavli Institute at Cornell for Nanoscale Science, Ithaca, NY, USA

    Jie Shan & Kin Fai Mak

Authors
  1. Tingxin Li
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jiacheng Zhu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Yanhao Tang
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Kenji Watanabe
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Takashi Taniguchi
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Veit Elser
    View author publications

    You can also search for this author in PubMed Google Scholar

  7. Jie Shan
    View author publications

    You can also search for this author in PubMed Google Scholar

  8. Kin Fai Mak
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

T.L. performed the measurements. T.L. and J.Z. fabricated the devices and analysed the data. Y.T. provided assistance in the device fabrication. V.E. performed theoretical calculations. K.W. and T.T. grew the bulk hBN crystals. T.L., J.S. and K.F.M. designed the scientific objectives and oversaw the project. T.L., V.E., J.S. and K.F.M co-wrote the manuscript. All authors discussed the results and commented on the manuscript.

Corresponding authors

Correspondence to Jie Shan or Kin Fai Mak.

Ethics declarations

Competing interests

The authors declare no competing interests.

Additional information

Peer review information Nature Nanotechnology thanks Raymond Ashoori and the other, anonymous, reviewer(s) for their contribution to the peer review of this work.

Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Extended data

Extended Data Fig. 1 Penetration capacitance.

Penetration capacitance (a) and top gate capacitance \(C/C_g\) (b) as a function of top gate voltage at 20 K (d/aM ≈ 0.6). We also show the top gate capacitance (obtained from a) in b for comparison. Good agreement between the penetration and top gate capacitance measurements is observed.

Source data

Extended Data Fig. 2 Frequency dependent capacitance (d/aM ≈ 0.6).

Top gate capacitance as a function of top gate voltage at 10 K (a) and at 20 K (b) under different excitation frequencies (10 mV modulation amplitude). A decrease in capacitance with increasing frequency is observed at 10 K, signifying the growing importance of the resistive component in the AC measurement. Meanwhile, no obvious frequency dependence is seen at 20 K, a temperature at which the sample/contact resistance becomes substantially smaller. The capacitance measurement is therefore in the low-frequency limit and reliable for most of the filling factors except ν = 1 and ν = 2.

Source data

Extended Data Fig. 3 Capacitive and resistive channels.

a, Top gate (or filling) dependence of the capacitive channel output (top) and the resistive channel output (bottom) at different excitation frequencies (device 4 at 10 K). A constant background was subtracted from the capacitive channel for better comparison. The resistive signal gradually goes away with decreasing frequency for most of the filling factors except ν = 1 and ν = 2, where a small increase instead is observed. We conclude that the AC capacitance measurement at 10 K is reliable for most of the filling factors at the lowest frequency (137 Hz) for this device. However, for ν = 2 and ν = 1, the AC measurement is in the high-frequency limit so that the measured capacitance dips at ν = 2 and ν = 1 only reflect the large sample/contact resistance. b, Same as a measured at different temperatures (device 1 at 3137 Hz). As the resistive signal grows with decreasing temperature, the capacitive signal decreases accordingly at 10 K and above. The resistive signal is negligible compared to the capacitive signal. In addition, small resistive peaks at ν = 2 and ν = 1 grow with decreasing temperature. All of these observations show that the measurements are in the low-frequency limit for all filling factors in this device at 10 K and above.

Source data

Extended Data Fig. 4 Top gate capacitance of device 2.

The top gate capacitance shows incompressible states up to ν = 8.

Source data

Extended Data Fig. 5 Temperature dependence of device 1.

a, Top gate capacitance as a function of filling factor at different temperatures. The charge-ordered states melt and the large capacitance enhancement disappears at high temperatures. b, Top gate capacitance at selected filling factors as a function of temperature. The capacitance in the incompressible region (v = 1/3 and 1/2) decreases rapidly below their transition temperatures, whereas the capacitance in the compressible regions (v = 0.42) increases. c, Filling factor versus top gate voltage at different temperatures obtained from integrating the data in a. The inset shows the temperature dependence of the top gate voltage at two constant filling factors. d, Filling factor dependence of (S−νkBln2), where S is the electronic entropy per moiré unit cell of the device extracted from two temperature windows, 15-40 (black) and 40-80 K (red).

Source data

Extended Data Fig. 6 Temperature dependence of Δμ (device 1).

Thermodynamic gap size Δμ as a function of temperature for v = 1 to 4 extracted from the capacitance measurements. Linear extrapolation (dashed lines) was used to extract the zero-temperature gap size tabulated in Table 1.

Source data

Extended Data Fig. 7 Optical micrograph.

Optical image of a typical device.

Extended Data Fig. 8 Lumped circuit model.

Lumped circuit model for the top gate capacitance measurement (a) and penetration capacitance measurement (b).

Extended Data Fig. 9 Table for the calculated chemical potentials.

Calculated chemical potentials, μν, (in units of εa) at different filling factors ν and d/aM.

Source data

Source Data Fig. 1

Source data for Fig. 1d,e with each panel as a different tab.

Source Data Fig. 2

Source data for Fig. 2 with each panel given as a different tab.

Source Data Fig. 3

Source data for Fig. 3 with each panel given as a different tab.

Source Data Extended Data Fig. 1

Source data for Extended Data Fig. 1 with each panel given as a different tab.

Source Data Extended Data Fig. 2

Source data for Extended Data Fig. 2 with each panel given as a different tab.

Source Data Extended Data Fig. 3

Source data for Extended Data Fig. 3 with each panel given as a different tab.

Source Data Extended Data Fig. 4

Source data for Extended Data Fig. 4 with each panel given as a different tab.

Source Data Extended Data Fig. 5

Source data for Extended Data Fig. 5 with each panel given as a different tab.

Source Data Extended Data Fig. 6

Source data for Extended Data Fig. 6 with each panel given as a different tab.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Li, T., Zhu, J., Tang, Y. et al. Charge-order-enhanced capacitance in semiconductor moiré superlattices. Nat. Nanotechnol. 16, 1068–1072 (2021). https://doi.org/10.1038/s41565-021-00955-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/s41565-021-00955-8

This article is cited by

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing