Abstract
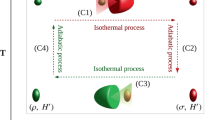
Cyclical heat engines are a paradigm of classical thermodynamics, but are impractical for miniaturization because they rely on moving parts. A more recent concept is particle-exchange (PE) heat engines, which uses energy filtering to control a thermally driven particle flow between two heat reservoirs1,2. As they do not require moving parts and can be realized in solid-state materials, they are suitable for low-power applications and miniaturization. It was predicted that PE engines could reach the same thermodynamically ideal efficiency limits as those accessible to cyclical engines3,4,5,6, but this prediction has not been verified experimentally. Here, we demonstrate a PE heat engine based on a quantum dot (QD) embedded into a semiconductor nanowire. We directly measure the engine’s steady-state electric power output and combine it with the calculated electronic heat flow to determine the electronic efficiency η. We find that at the maximum power conditions, η is in agreement with the Curzon–Ahlborn efficiency6,7,8,9 and that the overall maximum η is in excess of 70% of the Carnot efficiency while maintaining a finite power output. Our results demonstrate that thermoelectric power conversion can, in principle, be achieved close to the thermodynamic limits, with direct relevance for future hot-carrier photovoltaics10, on-chip coolers or energy harvesters for quantum technologies.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$259.00 per year
only $21.58 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Scovil, H. & Schulz-DuBois, E. Three-level masers as heat engines. Phys. Rev. Lett. 2, 262–263 (1959).
Humphrey, T. E. & Linke, H. Quantum, cyclic, and particle-exchange heat engines. Physica E 29, 390–398 (2005).
Mahan, G. & Sofo, J. The best thermoelectric. Proceed. Natl Acad. Sci. USA 93, 7436–7439 (1996).
Humphrey, T. E., Newbury, R., Taylor, R. P. & Linke, H. Reversible quantum Brownian heat engines for electrons. Phys. Rev. Lett. 89, 116801 (2002).
Humphrey, T. E. & Linke, H. Reversible thermoelectric nanomaterials. Phys. Rev. Lett. 94, 096601 (2005).
Van den Broeck, C. Thermodynamic efficiency at maximum power. Phys. Rev. Lett. 95, 190602 (2005).
Curzon, F. & Ahlborn, B. Efficiency of a Carnot engine at maximum power output. Am. J. Phys. 43, 22–24 (1975).
Esposito, M., Lindenberg, K. & Van den Broeck, C. Universality of efficiency at maximum power. Phys. Rev. Lett. 102, 130602 (2009).
Esposito, M., Kawai, R., Lindenberg, K. & Van den Broeck, C. Efficiency at maximum power of low-dissipation Carnot engines. Phys. Rev. Lett. 105, 150603 (2010).
Limpert, S., Bremner, S. & Linke, H. Reversible electron–hole separation in a hot carrier solar cell. New J. Phys. 17, 095004 (2015).
Callen, H. B. Thermodynamics and an Introduction to Thermostatistics 2nd edn, Ch. 4 (John Wiley & Sons, New York, 1985).
Wong, W. A., Wilson, S., Collins, J. & Wilson, K. Advanced Stirling Converter (ASC) Technology Maturation Report NASA/TM-2016-218908 (National Aeronautics and Space Agency, 2016).
Nakpathomkun, N., Xu, H. Q. & Linke, H. Thermoelectric efficiency at maximum power in low-dimensional systems. Phys. Rev. B 82, 235428 (2010).
O’Dwyer, M. F., Humphrey, T. E. & Linke, H. Concept study for a high-efficiency nanowire based thermoelectric. Nanotechnology 17, S338–S343 (2006).
Esposito, M., Lindenberg, K. & Van den Broeck, C. Thermoelectric efficiency at maximum power in a quantum dot. Europhys. Lett. 85, 60010 (2009).
Staring, A. A. M. et al. Coulomb-blockade oscillations in the thermopower of a quantum dot. Europhys. Lett. 22, 57 (1993).
Svilans, A., Leijnse, M. & Linke, H. Experiments on the thermoelectric properties of quantum dots. C. R. Phys. 17, 1096–1108 (2016).
Prance, J. R. et al. Electronic refrigeration of a two-dimensional electron gas. Phys. Rev. Lett. 102, 146602 (2009).
Björk, M. T. et al. Few-electron quantum dots in nanowires. Nano Lett. 4, 1621 (2004).
Turek, M. & Matveev, K. Cotunneling thermopower of single electron transistors. Phys. Rev. B 65, 115332 (2002).
Gluschke, J. G., Svensson, S. F., Thelander, C. & Linke, H. Fully tunable, non-invasive thermal biasing of gated nanostructures suitable for low-temperature studies. Nanotechnology 25, 385704 (2014).
König, J., Schoeller, H. & Schön, G. Cotunneling at resonance for the single-electron transistor. Phys. Rev. Lett. 78, 4482 (1997).
Leijnse, M. & Wegewijs, M. Kinetic equations for transport through single-molecule transistors. Phys. Rev. B 78, 235424 (2008).
Gergs, N. M., Hörig, C. B., Wegewijs, M. R. & Schuricht, D. Charge fluctuations in nonlinear heat transport. Phys. Rev. B 91, 201107 (2015).
Dresselhaus, M. S. et al. New directions for low-dimensional thermoelectric materials. Adv. Mat. 19, 1043–1053 (2007).
Conibeer, G., Jiang, C. W., Green, M., Harder, N. & Straub, A. Selective energy contacts for potential application to hot carrier PV cells. Proc. 3rd World Conf. Photovolt. En. Conv. 3, 2730–2733 (2003).
Persson, A. I., Fröberg, L. E., Jeppesen, S., Björk, M. T. & Samuelson, L. Surface diffusion effects on growth of nanowires by chemical beam epitaxy. J. Appl. Phys. 101, 034313 (2007).
Fröberg, L. E. et al. Transients in the formation of nanowire heterostructures. Nano Lett. 8, 3815–3818 (2008).
Acknowledgements
We thank S. Lehmann for the structural imaging of the nanowires used in this study. We acknowledge financial support by the People Programme (Marie Curie Actions) of the European Union’s Seventh Framework Programme (FP7-People-2013-ITN) under REA grant agreement no. 608153 (PhD4Energy), by the Swedish Energy Agency (project P38331-1), by the Swedish Research Council (projects 621-2012-5122, 2014-5490, 2015-00619 and 2016-03824), by the Knut and Alice Wallenberg Foundation (project 2016.0089), Marie Sklodowska Curie Actions, Cofund, Project INCA 600398 and by NanoLund. The computations were performed on resources provided by the Swedish National Infrastructure for Computing (SNIC) at LUNARC.
Author information
Authors and Affiliations
Contributions
H.L. and M.L. designed and guided the study. E.A.H. and S.F. performed preliminary experiments. S.F. grew the nanowires. A.S., A.M.B. and C.T. designed and fabricated the devices and carried out the experiments. M.J. and M.L. performed the theoretical calculations. M.J. and A.S. analysed the data. All the authors contributed to writing and editing the manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary Information
Supplementary Information
Supplementary Sections 1–5
Rights and permissions
About this article
Cite this article
Josefsson, M., Svilans, A., Burke, A.M. et al. A quantum-dot heat engine operating close to the thermodynamic efficiency limits. Nature Nanotech 13, 920–924 (2018). https://doi.org/10.1038/s41565-018-0200-5
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41565-018-0200-5
This article is cited by
-
Seebeck Power Generation and Peltier Cooling in a Normal Metal-Quantum Dot-Superconductor Nanodevice
Journal of Low Temperature Physics (2024)
-
Atom-level electronic physicists are needed to develop practical engines with a quantum advantage
npj Quantum Information (2023)
-
Filtering electrons by mode coupling in finite semiconductor superlattices
Scientific Reports (2022)
-
Spin-thermoelectric effects in a quantum dot hybrid system with magnetic insulator
Scientific Reports (2022)
-
Bipolar thermoelectric Josephson engine
Nature Nanotechnology (2022)