Abstract
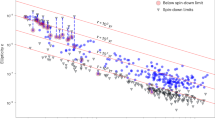
Gravitational waves at ultra-low frequencies (≲100 nHz) are key to understanding the assembly and evolution of astrophysical black hole binaries with masses ~106–109 M⊙ at low redshifts1,2,3. These gravitational waves also offer a unique window into a wide variety of cosmological processes4,5,6,7,8,9,10,11. Pulsar timing arrays12,13,14 are beginning to measure15 this stochastic signal at ~1–100 nHz and the combination of data from several arrays16,17,18,19 is expected to confirm a detection in the next few years20. The dominant physical processes generating gravitational radiation at nHz frequencies are still uncertain. Pulsar timing array observations alone are currently unable21 to distinguish a binary black hole astrophysical foreground22 from a cosmological background due to, say, a first-order phase transition at a temperature ~1–100 MeV in a weakly interacting dark sector8,9,10,11. This letter explores the extent to which incorporating integrated bounds on the ultra-low-frequency gravitational wave spectrum from any combination of cosmic microwave background23,24, big bang nucleosynethesis25,26 or astrometric27,28 observations can help to break this degeneracy.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 digital issues and online access to articles
$119.00 per year
only $9.92 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
Data availability
The data used for the analyses presented is publicly available from: https://data.nanograv.org.
Code availability
The code used for the analyses presented here, including that to make all figures, is available at https://github.com/cjm96/PTA_and_IntegratedConstraints, which uses code from https://github.com/AMitridate/bokeh/tree/master/bokeh-app.
References
Sesana, A., Vecchio, A. & Colacino, C. N. The stochastic gravitational-wave background from massive black hole binary systems: implications for observations with pulsar timing arrays. Mon. Not. R. Astron. Soc. 390, 192–209 (2008).
Sesana, A. Systematic investigation of the expected gravitational wave signal from supermassive black hole binaries in the pulsar timing band. Mon. Not. R. Astron. Soc. 433, L1–L5 (2013).
Kelley, L. Z., Blecha, L., Hernquist, L., Sesana, A. & Taylor, S. R. The gravitational wave background from massive black hole binaries in Illustris: spectral features and time to detection with pulsar timing arrays. Mon. Not. R. Astron. Soc. 471, 4508–4526 (2017).
De Luca, V., Franciolini, G. & Riotto, A. NANOGrav data hints at primordial black holes as dark matter. Phys. Rev. Lett. 126, 041303 (2021).
Vaskonen, V. & Veermäe, H. Did NANOGrav see a signal from primordial black hole formation? Phys. Rev. Lett. 126, 051303 (2021).
Ellis, J. & Lewicki, M. Cosmic string interpretation of NANOGrav pulsar timing data. Phys. Rev. Lett. 126, 041304 (2021).
Blasi, S., Brdar, V. & Schmitz, K. Has NANOGrav found first evidence for cosmic strings? Phys. Rev. Lett. 126, 041305 (2021).
Nakai, Y., Suzuki, M., Takahashi, F. & Yamada, M. Gravitational waves and dark radiation from dark phase transition: connecting NANOGrav pulsar timing data and Hubble tension. Phys. Lett. B 816, 136238 (2021).
Ratzinger, W. & Schwaller, P. Whispers from the dark side: confronting light new physics with NANOGrav data. SciPost Phys. 10, 047 (2021).
Addazi, A., Cai, Y.-F., Gan, Q., Marciano, A. & Zeng, K. NANOGrav results and dark first order phase transitions. Sci. China Phys. Mech. Astron. 64, 290411 (2021).
Schwaller, P. Gravitational waves from a dark phase transition. Phys. Rev. Lett. 115, 181101 (2015).
Sazhin, M. V. Opportunities for detecting ultralong gravitational waves. Sov. Astron. 22, 36–38 (1978).
Detweiler, S. Pulsar timing measurements and the search for gravitational waves. Astrophys. J. 234, 1100–1104 (1979).
Foster, R. S. & Backer, D. C. Constructing a pulsar timing array. Astrophys. J. 361, 300–308 (1990).
Arzoumanian, Z. et al. The NANOGrav 12.5 yr data set: search for an isotropic stochastic gravitational-wave background. Astrophys. J. Lett. 905, L34 (2020).
Shannon, R. M. et al. Gravitational waves from binary supermassive black holes missing in pulsar observations. Science 349, 1522–1525 (2015).
Lentati, L. et al. European Pulsar Timing Array limits on an isotropic stochastic gravitational-wave background. Mon. Not. R. Astron. Soc. 453, 2576–2598 (2015).
Arzoumanian, Z. et al. The NANOGrav 11 year data set: pulsar-timing constraints on the stochastic gravitational-wave background. Astrophys. J. 859, 47 (2018).
Verbiest, J. P. W. et al. The International Pulsar Timing Array: first data release. Mon. Not. R. Astron. Soc. 458, 1267–1288 (2016).
Pol, N. S. et al. Astrophysics milestones for pulsar timing array gravitational-wave detection. Astrophys. J. Lett. 911, L34 (2021).
Arzoumanian, Z. et al. Searching for gravitational waves from cosmological phase transitions with the NANOGrav 12.5-year dataset. Preprint at https://arxiv.org/abs/2104.13930 (2021).
Middleton, H. et al. Massive black hole binary systems and the NANOGrav 12.5 yr results. Mon. Not. R. Astron. Soc. 502, L99–L103 (2021).
Smith, T. L., Pierpaoli, E. & Kamionkowski, M. New cosmic microwave background constraint to primordial gravitational waves. Phys. Rev. Lett. 97, 021301 (2006).
Clarke, T. J., Copeland, E. J. & Moss, A. Constraints on primordial gravitational waves from the cosmic microwave background. J. Cosmol. Astropart. Phys. 2020, 002 (2020).
Cooke, R. J., Pettini, M., Jorgenson, R. A., Murphy, M. T. & Steidel, C. C. Precision measures of the primordial abundance of deuterium. Astrophys. J. 781, 31 (2014).
Maggiore, M. Gravitational Waves Vol. 2 (Oxford Univ. Press, 2018).
Darling, J., Truebenbach, A. E. & Paine, J. Astrometric limits on the stochastic gravitational wave background. Astrophys. J. 861, 113 (2018).
Book, L. G. & Flanagan, É. É. Astrometric effects of a stochastic gravitational wave background. Phys. Rev. D 83, 024024 (2011).
Planck Collaboration Planck 2018 results. VI. Cosmological parameters. Astron. Astrophys. 641, A6 (2020).
Mihaylov, D. P., Moore, C. J., Gair, J. R., Lasenby, A. & Gilmore, G. Astrometric effects of gravitational wave backgrounds with non-Einsteinian polarizations. Phys. Rev. D 97, 124058 (2018).
Caprini, C., Durrer, R. & Servant, G. The stochastic gravitational wave background from turbulence and magnetic fields generated by a first-order phase transition. J. Cosmol. Astropart. Phys. 2009, 024 (2009).
Jinno, R. & Takimoto, M. Gravitational waves from bubble collisions: an analytic derivation. Phys. Rev. D 95, 024009 (2017).
Hindmarsh, M., Huber, S. J., Rummukainen, K. & Weir, D. J. Shape of the acoustic gravitational wave power spectrum from a first order phase transition. Phys. Rev. D 96, 103520 (2017).
Perera, B. B. P. et al. The International Pulsar Timing Array: second data release. Mon. Not. R. Astron. Soc. 490, 4666–4687 (2019).
Janssen, G. et al. Gravitational wave astronomy with the SKA. In Proc. Advancing Astrophysics with the Square Kilometre Array (AASKA14) 37 (SKA, 2015).
Hellings, R. W. & Downs, G. S. Upper limits on the isotropic gravitational radiation background from pulsar timing analysis. Astrophys. J. Lett. 265, L39–L42 (1983).
Brandenburg, A., Clarke, E., He, Y. & Kahniashvili, T. Can we observe the QCD phase transition-generated gravitational waves through pulsar timing arrays? Phys. Rev. D 104, 043513 (2021).
Neronov, A., Pol, A. R., Caprini, C. & Semikoz, D. NANOGrav signal from magnetohydrodynamic turbulence at the QCD phase transition in the early universe. Phys. Rev. D 103, L041302 (2021).
Li, S.-L., Shao, L., Wu, P. & Yu, H. NANOGrav signal from first-order confinement-deconfinement phase transition in different QCD-matter scenarios. Phys. Rev. D 104, 043510 (2021).
Abe, K. T., Tada, Y. & Ueda, I. Induced gravitational waves as a cosmological probe of the sound speed during the QCD phase transition. J. Cosmol. Astropart. Phys. 2021, 048 (2021).
Vagnozzi, S. Implications of the NANOGrav results for inflation. Mon. Not. R. Astron. Soc. 502, L11–L15 (2021).
Braginsky, V. B., Kardashev, N. S., Polnarev, A. G. & Novikov, I. D. Propagation of electromagnetic radiation in a random field of gravitational waves and space radio interferometry. Nuovo Cimento B 105, 1141–1158 (1990).
Kaiser, N. & Jaffe, A. Bending of light by gravity waves. Astrophys. J. 484, 545–554 (1997).
Schutz, B. F. Astrometric and timing effects of gravitational waves. Proc. Int. Astron. Union 261, 234–239 (2010).
Pyne, T., Gwinn, C. R., Birkinshaw, M., Eubanks, T. M. & Matsakis, D. N. Gravitational radiation and very long baseline interferometry. Astrophys. J. 465, 566–577 (1996).
Shapiro, I. I. in Methods in Experimental Physics Vol. 12, 261–276 (Academic Press, 1976).
Gaia Collaboration et al. The Gaia mission. Astron. Astrophys. 595, A1 (2016).
Gaia Collaboration Gaia early data release 3. Summary of the contents and survey properties. Astron. Astrophys. 649, A1 (2021).
Moore, C. J., Mihaylov, D. P., Lasenby, A. & Gilmore, G. Astrometric search method for individually resolvable gravitational wave sources with Gaia. Phys. Rev. Lett. 119, 261102 (2017).
Klioner, S. A. Gaia-like astrometry and gravitational waves. Class. Quantum Gravity 35, 045005 (2018).
Mignard, F. & Klioner, S. Analysis of astrometric catalogues with vector spherical harmonics. Astron. Astrophys. 547, A59 (2012).
Qin, W., Boddy, K. K., Kamionkowski, M. & Dai, L. Pulsar-timing arrays, astrometry, and gravitational waves. Phys. Rev. D 99, 063002 (2019).
O’Beirne, L. & Cornish, N. J. Constraining the polarization content of gravitational waves with astrometry. Phys. Rev. D 98, 024020 (2018).
Mihaylov, D. P., Moore, C. J., Gair, J. R., Lasenby, A. & Gilmore, G. Astrometric effects of gravitational wave backgrounds with nonluminal propagation speeds. Phys. Rev. D 101, 024038 (2020).
Gwinn, C. R., Eubanks, T. M., Pyne, T., Birkinshaw, M. & Matsakis, D. N. Quasar proper motions and low-frequency gravitational waves. Astrophys. J. 485, 87–91 (1997).
Titov, O., Lambert, S. B. & Gontier, A. M. VLBI measurement of the secular aberration drift. Astron. Astrophys. 529, A91 (2011).
Paine, J., Darling, J., Graziani, R. & Courtois, H. M. Secular extragalactic parallax: measurement methods and predictions for Gaia. Astrophys. J. 890, 146 (2020).
Hobbs, D. et al. GaiaNIR: Combining optical and near-infra-red (NIR) capabilities with time-delay-integration (TDI) sensors for a future Gaia-like mission. Preprint at https://arxiv.org/abs/1609.07325 (2016).
Theia Collaboration et al. Theia: faint objects in motion or the new astrometry frontier. Preprint at https://arxiv.org/abs/1707.01348 (2017).
Speagle, J. S. DYNESTY: a dynamic nested sampling package for estimating Bayesian posteriors and evidences. Mon. Not. R. Astron. Soc. 493, 3132–3158 (2020).
Skilling, J. Nested sampling. AIP Conf. Proc. 735, 395–405 (2004).
Middleton, H., Del Pozzo, W., Farr, W. M., Sesana, A. & Vecchio, A. Astrophysical constraints on massive black hole binary evolution from pulsar timing arrays. Mon. Not. R. Astron. Soc. 455, L72–L76 (2016).
Phinney, E. S. A practical theorem on gravitational wave backgrounds. Preprint at https://arxiv.org/abs/astro-ph/0108028 (2001).
Acknowledgements
A.V. acknowledges the support of the Royal Society and Wolfson Foundation. We thank W. Ratzinger and K. Schmitz for useful discussions on the analysis.
Author information
Authors and Affiliations
Contributions
Both C.J.M. and A.V. jointly conceived this study. C.J.M. performed the numerical calculations. Both C.J.M. and A.V. jointly analysed and interpreted the results and wrote the manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Peer review information Nature Astronomy thanks Lijing Shao and the other, anonymous, reviewer(s) for their contribution to the peer review of this work.
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Supplementary Information
Supplementary Fig. 1.
Rights and permissions
About this article
Cite this article
Moore, C.J., Vecchio, A. Ultra-low-frequency gravitational waves from cosmological and astrophysical processes. Nat Astron 5, 1268–1274 (2021). https://doi.org/10.1038/s41550-021-01489-8
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41550-021-01489-8
This article is cited by
-
Cosmological interpretation for the stochastic signal in pulsar timing arrays
Science China Physics, Mechanics & Astronomy (2024)
-
Implications for the supermassive black hole binaries from the NANOGrav 15-year data set
Science China Physics, Mechanics & Astronomy (2023)
-
Scalar induced gravitational waves in light of Pulsar Timing Array data
Science China Physics, Mechanics & Astronomy (2023)