Introduction

The spin Hall effect (SHE) is a phenomenon that a transverse pure spin current appears if a longitudinal electric field is applied1,2,3,4. Topological insulators (TIs) and quantum SHE systems were considered as ideal materials for producing pure spin current5,6. However, the experiments have shown that the bulk carrier problem prevents the widespread applications of TIs in spintronic devices because of the hybridization between surface states and bulk states in Bi2Se3 class materials7,8. Generally speaking, there are two kinds of mechanisms for the SHE, which are the extrinsic mechanism and intrinsic mechanism. For the former, impurities and defects with strong SOC are the main scattering resources. Both the side-jump and skew scattering are specifically identified and similar to those in the study of anomalous Hall effect. The intrinsic mechanism roots in the topologically nontrivial energy bands, which can be accurately calculated based on ab initio calculations and Kubo formula. Recently, heavy metals and compounds including heavy elements have attracted growing attention for the possibility to realize large SHE9,10,11,12,13. In particular, a lot of interests focus on topological semimetals (TSMs), which exhibit similar spin-momentum locking in both bulk and topological surface states. To date, the SHC of some TSMs, like TaAs, NbAs, and rutile oxides9,14, is still far below that of pure heavy metal Pt and W. Therefore, the TSMs with larger SHC are still highly desirable both for the purposes of fundamental research and technical applications.

According to the distribution and degeneracy of crossing nodal points, TSMs can usually be divided into three categories: Dirac semimetals, Weyl semimetals, and nodal line semimetals (NLSMs)15,16,17,18,19,20,21,22,23. The Weyl points are discrete points in momentum space where the conduction bands and valence bands cross each other. However, in the NLSMs, these crossing points are not discrete but form a continuous path. Compared to the Fermi arc surface states existing in Dirac and Weyl semimetals, NLSMs show drumhead surface states, which are regarded as excellent platforms hosting many interaction-induced nontrivial states, such as superconductivity and fractional topological insulator21. It is known that the nodal line can be protected by appropriate symmetries, such as mirror symmetry, time-reversal symmetry, and inversion symmetry24,25,26. Interestingly, the nodal line states have been classified into various types, e.g., isolated closed nodal ring, nodal chain, nodal link, nodal knots, nodal straight line, and even crossing nodal-line (CNL)27,28. Until now, the real NLSMs that can host CNL are very rare28. It is curious to find these CNL semimetals (CNLSMs) and explore their possibility on exotic SHC applications.

In this article, using first-principles calculations, we propose that the X3Y (X = Ta, Nb; Y = As, Sb, Bi) compounds are a family of CNLSMs. Interestingly, without taking into account the effects of SOC, X3Y compounds host two sets of complex CNL around Fermi level. One is located around the corners, while others are distributed in the bulk of momentum space. When the SOC is present, Dirac points are emergent along the R–M path due to the C4 rotational symmetry and are absent along other directions with a very tiny gap. Importantly, it is found that Ta3Y compounds exhibit large intrinsic SHC. For example, the SHC is around 1500 \((\hbar /e)\left( {{\mathrm{{\Omega}}} \cdot {\mathrm{cm}}} \right)^{ - 1}\) in stable Ta3As compound, which is attributed to its large SOC band splitting. Therefore, the prediction of Dirac CNLSMs in X3Y family not only extends our current knowledge on NLSMs, but also suggests a routine of realizing large SHC to replace noble metals. Significantly, X3Y family materials, in particular the stable Ta3As compound, are promising candidates for the application in spintronic devices29,30,31,32.

Results and discussion

Crystal structures and stability of X3Y compounds

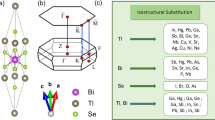
The crystal structure of the X3Y (X = Ta, Nb; Y = As, Sb, Bi) compounds is the same as the ordered L12 phase Cu3Au16,33. We adopt the cubic structure with the space group \({\mathrm{Pm}}\bar 3m\) (No. 221), as shown in Fig. 1a. A primitive cell contains three X atoms at the plane center and one Y atom at the vertex. Figure 1b shows the bulk Brillouin zone (BZ) of the X3Y and the projected surface BZ of the (010) surface. Previous studies mainly focused on the Ta3Y compounds with the Ti3P-type (A15 phase, space group 223) structure34,35, instead of the \({\mathrm{Pm}}\bar 3m\) phase. To illustrate the thermodynamic and lattice stability of \({\mathrm{Pm}}\bar 3m\)-phase X3Y compounds, we calculate their formation enthalpies (ΔH) and the phonon spectra. The negative ΔH values of Ta3As, Nb3As, and Nb3Sb indicate that they are thermodynamically stable against phase decomposition, as shown in Fig. 1c. In addition, the absence of imaginary modes in the entire BZ further confirms their lattice stability, as shown in Supplementary Fig. 1. The calculated lattice constant of Ta3As, Nb3As, and Nb3Sb are 4.102, 4.105, and 4.226 Å, respectively.

Fig. 1: Crystal structure.
figure 1

a The atomic structure of the L12 phase X3Y (X = Ta, Nb; Y = As, Sb, Bi) family compounds. Blue and yellow spheres denote the X and Y atoms, respectively. b The bulk Brillouin zone (BZ) and the projected BZ on the (010) surface. c The formation enthalpies (ΔH) of different X3Y compounds. The unit is eV per unit-cell.

Full size image

Electronic structures and effective Hamiltonian model

The calculated electronic structures of Ta3As, Nb3As, and Nb3Sb present similar characters, with fourfold degeneracy around a crossing point because of the time-reversal and inversion symmetry. Without the SOC effects, Dirac points exist along high-symmetry paths in the BZ, e.g., M–X and M–Γ paths, as shown in Fig. 2. Similar to the case of Cu3PdN and Mackay–Terrones crystal, the band crossings can be understood from the argument of codimension16,17,18,19,20,21. In general, the four bands without SOC effects near the Dirac points can be described by two identical 2 × 2 Hamiltonians, which are written as

$${\it{H}}\left( {\it{k}} \right) = {\it{d}}_{\it{x}}\left( {\it{k}} \right)\sigma _{\it{x}} + {\it{d}}_{\it{y}}\left( {\it{k}} \right)\sigma _{\it{y}}{\it{ + d}}_{\it{z}}\left( {\it{k}} \right)\sigma _{\it{z}}$$
(1)

Where σx,y,z and dx,y,z(k) are Pauli matrices and corresponding coefficients, respectively. At Γ and R point with little group Oh, the time-reversal, inversion symmetry as well as rotation symmetry restrict the coefficients, with dy(k) = 0, dx(k), and dz(k) being odd and even functions correspondingly. We can write dx,z(k) as follow,

$$d_x\left( k \right) = \gamma k_xk_yk_z + \lambda _zk_x^2k_y^2k_z + \lambda _yk_x^2k_yk_z^2 + \lambda _xk_xk_y^2k_z^2 + \ldots$$
(2)
$$d_z\left( k \right) = M - B(k_x^2 + k_y^2 + k_z^2)$$
(3)
Fig. 2: Calculated band structure of X3Y family materials without and with spin-orbit coupling (SOC).
figure 2

a, d Band structure of Ta3As without and with SOC (the left column); b, e That of Nb3As (the middle column); c and f That of Nb3Sb (the right column). Here, the position of point ∆ (panel a) related to band inversion contributes to the large SHC.

Full size image

Here γ, λx,y,z, M, and B are the corresponding coefficients. The eigenvalue of Eq. (1) is \(E\left( k \right) = \pm \sqrt {d_x^2\left( k \right) + d_z^2(k)}\). The band crossing points appear only for the case of E(k) = 0, i.e., both dx(k) = 0 and dz(k) = 0. Here, the condition dz(k) = 0 is satisfied only when energy bands inversion occurs. As shown in Supplementary Fig. 2, it is clear that the p-d bands inversion happens along the R–Γ, R–X, and R–M paths. Furthermore, if we ignore the higher-order terms in Eq. (2) with dx(k) = γkxkykz = 0, Eq. (2) and Eq. (3) induce a nodal line topological state16.

However, the crossing points are gapped along M–X, M–Γ, R–Γ, and R–X paths but preserved along R–M path when taking into account the SOC in Fig. 2. The band structures are similar for Ta3As, Nb3As, and Nb3Sb. Clearly, both the R and Γ points belong to the Oh double point group, and the irreducible representation of bands near the Dirac points is marked with the red symbol Γi (i = 4, 5, 6, 7). (i) The R–X path: Moving from R to X, the symmetry is lowered to C2v double point group, where only the Γ5 irreducible representation exists36. (ii) The R-Γ path: Characterized by C3v double group, both the occupied band and non-occupied one along R-Γ path are the Γ4 irreducible representation in C3v double point group. As we know, if the two bands at the crossing points belong to the same irreducible representation, the crossing points will not be protected by the symmetry37. In Fig. 2a (without SOC) and Fig. 2d (with SOC), it is observed that the crossing points are gapped with a tiny value along R–X and R-Γ path due to the effect of SOC. (iii) The R–M path: The crossing points still exist, which results from the non-occupied and occupied bands belonging to different irreducible representations Γ6 and Γ7 in the C4v double point group, respectively. The existing crossing points are protected by crystal symmetry, thus no gap appears along the R–M path. To express the influence of SOC strength on Dirac points, we take Ta3As as an example to show the evolution of energy bands at different SOC strengths along the R–X and R–M path in Supplementary Fig. 4. When the SOC strength is artificially increased to three times larger than the intrinsic value, the Dirac points disappear obviously along the R–Γ path but still exist along the R–M path.

Crossing nodal-lines (CNL) and drumhead surface states

In previous research for Dirac nodal line and nodal sphere, the energy gap is Eg < 2 meV28. This energy scale is rough and it is desirable to explore the behavior of Dirac nodal points more precisely. Therefore, we explore extremely tiny energy gaps which are identified at 0.1 meV level, and present their distributions in 3D momentum space as well as the topological surface states in Fig. 3. It is clearly seen that continuous Dirac nodal points are merging into nodal lines, and they cross each other forming the crossing nodal-lines. Moreover, three nodal lines intersect at the R point, while two lines form a nodal net centered on the Γ point. And because of the same Oh double group at both points, the crossing nodal-lines appear at the position of the R point and Γ point. As we know, the NLSMs should present drumhead surface states, which can be observed in Fig. 3b with the connection between conduction and valence bands on the (010) surface21. Besides, the surface states on other oriented surfaces, like (100) and (001) surface, are also calculated, as shown in Supplementary Fig. 7. It is observed that the drumhead surface states on the above three surfaces are almost the same, which is similar to the case in YH3 materials28.

Fig. 3: The crossing nodal-lines and topological surface states.
figure 3

a The distribution of the Dirac points without spin-orbit coupling in the first Brillouin zone. Crossing nodal-lines are observed. b The drumhead surface state on the (010) surface at the R point around the Fermi level. The spin-orbit coupling is included.

Full size image

Spin Hall conductivity

As a measurable and calculated physical quantity, the intrinsic spin Hall conductivity (SHC) is usually used to describe the strength of the SHE. The SHC and SHE in TSMs have been explored in a large number of previous works. It is indicated that band crossings may lead to large SHC9,14. In view of band characters, we also find and analyze large SHC around the Fermi energy in the Ta3Y (Y = As, Sb, Bi) compounds. At the zero-temperature limit, the intrinsic SHC tensor can be expressed using the Kubo formula38,39,

$$\sigma _{xy} = \frac{\hbar }{{VN_k^3}}\mathop {\sum}\limits_k {\mathop {\sum}\limits_n {f_{nk} \times \mathop {\sum}\limits_{m \ne n} {\frac{{2Im[{\langle{nk}}{\mathrm{|}}\hat J_x{\mathrm{|}}mk{\rangle{}}{\langle{mk}}{\mathrm{|}} - e\hat v_y{\mathrm{|}}nk{\rangle{}}]}}{{({\it{\epsilon }}_{nk} - {\it{\epsilon }}_{mk})^2 - (\hbar \omega + i\eta )^2}}} } }$$
(4)

Here, \(\hat j_x = \frac{1}{2}[\hat s_z,\hat v_x]\) is the spin current operator, V is the primitive cell volume, \(N_k^3\) is the number of k points in the BZ, n (m), \(\epsilon_n\) and |mk〉 are band indexes, eigenvalues and the Bloch wave function, respectively, fnk is the Fermi distribution function, and \(\hat v_y = \frac{1}{\hbar }\frac{{\partial H(k)}}{{\partial k_y}}\) is the velocity operator. Besides, we multiply a factor \(2e/\hbar\) to the SHC in the unit of charge conductivity (Ω·cm)−1, similar to anomalous Hall conductivity40.

The SHC with the chemical position for the X3Y family is displayed in Fig. 4a. Obviously, the SHC reaches its peak value of about 1300–1700 \((\hbar /e)({\mathrm{{\Omega}}} \cdot {\mathrm{cm}})^{ - 1}\) just near the Fermi energy. In comparison, the SHC of Ta3As is approximate 1500 \((\hbar /e)({\mathrm{{\Omega}}} \cdot {\mathrm{cm}})^{ - 1}\), which is much larger than that of GaAs compounds about 100 \((\hbar /e)({\mathrm{{\Omega}}} \cdot {\mathrm{cm}})^{ - 1}\), WTe2 <400 \((\hbar /e)({\mathrm{{\Omega}}} \cdot {\mathrm{cm}})^{ - 1}\), OsO2 ~541 \((\hbar /e)({\mathrm{{\Omega}}} \cdot {\mathrm{cm}})^{ - 1}\), and TaAs ~781 \((\hbar /e)({\mathrm{{\Omega}}} \cdot {\mathrm{cm}})^{ - 1}\), as well as 4d and 5d transition metals <1000 \((\hbar /e)({\mathrm{{\Omega}}} \cdot {\mathrm{cm}})^{ - 1}\) and β−W ~ 1255 \((\hbar /e)({\mathrm{{\Omega}}} \cdot {\mathrm{cm}})^{ - 1}\)[ 9,10,11,12,14,39,41, even comparable to that of Pt ~2200 \((\hbar /e)({\mathrm{{\Omega}}} \cdot {\mathrm{cm}})^{ - 1}\)[ 10. Thus, Ta3Y compounds belong to a kind of Dirac CNLSMs with large SHC, which could be applied to spintronic devices such as the magnetic tunnel junction based on spin-orbit torque29.

Fig. 4: The intrinsic spin Hall conductivity (SHC).
figure 4

a The SHC \(\sigma _{xy}^z\) as a function of the chemical position. The gray vertical line corresponding to the Fermi energy. Lines in different colors represent the SHC of different CNLSMs X3Y compounds. b The band-resolved SHC of Ta3As. c The momentum-resolved SHC of Ta3As along the high-symmetry momentum paths.

Full size image

Further, we analyze the mechanism by calculating the band-resolved and momentum-resolved SHC of the stable Ta3As compound, corresponding to Fig. 4b, c. Here, the contribution to SHC is noted with red color dots in Fig. 4b, and the momentum-resolved SHC after taking the logarithm is shown in Fig. 4c. From Fig. 4 and Fig. 2 as well as Supplementary Fig. 5, we observe that the states around Δ point (along X–M path) mostly contribute to the large SHC with strong SOC effect. Note that the SHC of CNLSMs Ta3Y compounds is much larger than that of Nb3Y. Basically, the element Ta is heavier than Nb. Thus, the SOC in Ta3Y compounds may be stronger than those in Nb3Y, leading to larger SHC in CNLSMs Ta3As family. Moreover, the SHC of Ta3Bi compound almost arrives to 1700 \((\hbar /e)({\mathrm{{\Omega}}} \cdot {\mathrm{cm}})^{ - 1}\) and becomes the largest one in the Dirac CNLSMs X3Y compounds, thanks to the larger atomic mass of element Bi than other members in the same group. In brief, the stronger SHE and larger SHC in X3Y family materials stem from larger atomic mass and stronger atomic SOC in these compounds. In addition, we show the same character of band structures and the effect of SOC on band inversion in different X3Y materials in Supplementary Fig. 8.

In summary, based on the first-principles calculations, we have predicted the X3Y (X = Ta, Nb; Y = As, Sb, Bi) family compounds as a kind of Dirac crossing nodal-line semimetal (CNLSMs) with large spin Hall conductivity (SHC), which can serve as an ideal platform to research rich topological quantum states and explore the application in spintronics. In the absence of SOC, three nodal lines converge at the R point and two lines cross with forming a nodal net around the Γ point. After SOC is included, Dirac points are protected along the R–M path by the C4 rotational symmetry and open a very tiny gap along other directions. The drumhead surface states conform to the feature of Dirac CNLSMs. At the same time, by calculating the momentum-resolved and band-resolved spin Hall conductivity, the large SHC in Ta3As is attributed to the SOC along X–M path, instead of the nodal points. This mechanism differs from both the Weyl point leading to SHC for TaAs and nodal line inducing SHC for IrO29,14. The prediction in this work should promote further research on spin Hall effect, the topological and superconductivity properties, as well as quantum phase transitions. On the other hand, the fascinating crossing nodal-lines and the remarkable SHC at Fermi energy will contribute to their practical applications in assembling spintronic devices such as magnetic spin-orbit torque random access memory.

Methods

DFT calculations

The first-principles calculations are performed within the framework of density functional theory (DFT), as implemented in QUANTUM ESPRESSO package42. The generalized gradient approximation in the parametrization of Perdew–Burke–Ernzerhof is used for the exchange-correlation potential43. The kinetic energy cut-off and the charge density cut-off of the plane wave basis are chosen with 70 Ry and 850 Ry, respectively. All atoms are relaxed until the forces are smaller than 0.001 eV/Å. An 41 × 41 × 41 k-point mesh in the electronic self-consistent calculations is used for the Brillouin zone (BZ) integration. To evaluate the thermodynamic stabilities of X3Y compounds, the formation enthalpies (ΔH) were calculated as: ΔH = E(X3Y)-3E(X)-E(Y). The phonon spectra were calculated using the PHONOPY codes based on the finite displacement method within a 4 × 4 × 4 supercell with 3 × 3 × 3 k-point mesh44. The spin Berry curvature, spin Hall conductivity, and topological edge states are calculated by using a tight-binding Hamiltonian constructed on a basis of maximally localized Wannier functions implemented in Wannier90 and WannierTools codes45,46. The SHC is obtained by summing the spin Berry curvatures over all the occupied bands in a dense k-point grid of 200 × 200 × 200.