Introduction

Nuclear astrophysics, the study of nuclear processes in stars and in the cosmos, is a mature science that, among other topics, led to the theory of stellar evolution. In this theory, the uncertainty of the C/O ratio at the end of helium burning still remains significant. In his Nobel speech in 1984, W.A. Fowler stated "the ratio of 12C to 16O in helium burning is of paramount importance in nuclear astrophysics"1, as it still is today. For example, it determines the fate of Type II supernovae (black hole or neutron star) as well as the light curves of Type Ia supernovae, which are used to measure cosmological distances, leading to the recent discovery of the accelerated expansion and Dark Energy.

In stellar helium burning, carbon and oxygen are formed. Since the formation of carbon is rather well understood, the C/O ratio is determined largely by the single remaining uncertain process: the fusion of 12C with an alpha-particle to form 16O, denoted as the 12C(α, γ)16O reaction. This reaction was measured in terrestrial laboratories to energies below 2 MeV, but it needs to be extrapolated to stellar conditions of a plasma with a temperature of kT ~ 20 keV, and the most efficient burning energy of 300 keV (the Gamow window1). In stellar conditions, two partial waves, ℓ = 1 and 2, contribute, and they are denoted by the spectroscopic E1 and E2 amplitudes in reaction and scattering theory1. The challenges in this field are measurements of angular distributions of the 12C(α, γ) reaction from which the E1 and E2 cross sections are extracted, for accurate extrapolations to stellar conditions.

Progress was achieved in measuring angular distributions of the 12C(α, γ) reaction by directly measuring the emitted gamma-rays2,3,4,5,6,7,8,9. However, large uncertainties remain in the measured E1 and E2 cross sections and their extrapolations to stellar conditions at the Gamow window, SE1(300) and SE2(300), respectively1. In the latest extrapolation to stellar conditions using R-matrix analysis, deBoer et al.10 examined the current world data and concluded that a "level of uncertainty ~10% may be in sight".

Previously measured angular distributions2,3,4,5,6,7,8,9 were fitted with the three parameters: E1 and E2 amplitudes and their mixing phase angle (ϕ12), as outlined by Dyer and Barnes2,11. The extracted ϕ12 values at energies of Ecm < 2.0 MeV8,9 were found in agreement with the theoretical prediction2,11: ϕ12 = \({\delta }_{2}\,-\,{\delta }_{1}\,+\,{\tan }^{-1}\,\eta /2\), where δ1 and δ2 are the measured α + 12C elastic scattering phase shifts, and for this system, η = 12 × α/β, where α is the fine structure constant and β = v/c. In the energy region immediately below 2.0 MeV, the ratio E1/E2 and ϕ12 are almost constant. In contrast, in the energy region of 2.0 < Ecm < 2.6 MeV, both E1/E2 and ϕ12 vary rapidly as a consequence of the broad 1− resonance at 9.58 MeV in 16O. As shown by Brune and Sayre (Fig. 12 of ref. 12), in this energy region, the variation of ϕ12 leads to subtle changes in the measured angular distributions. Therefore, the region of Ecm = 2.0−2.6 MeV, is ideally suited for testing the accuracy of measured angular distributions.

However, Assunção et al.6 observed substantial disagreement with the theoretical prediction for energies down to 1.31 MeV, including the region of interest Ecm = 2.0−2.6 MeV2 (cos ϕ12 differs by up to a factor 2). Ouellet et al.4 noted in Table II (footnote b) that they were unable to measure ϕ12 from 1.9−2.4 MeV. The data of Redder et al.3 are measured mostly with 100% error bars in this region, as are the data of Dyer and Barnes2. Thus, so far, no available data at Ecm = 2.0−2.6 MeV exhibit the predicted2 strong variation of ϕ12 over the 1− resonance region.

The theoretical values for ϕ12 were originally considered to be a prediction of R-Matrix theory11. However, more recently, it was shown that the theoretical prediction for ϕ122 is a consequence of the Watson theorem, which is derived assuming the unitarity of the scattering matrix13,14. This theoretical prediction is valid in general when the capture cross-section is small, and it is the only open reaction channel, as is the case here. Hence, the observed discrepancy between the world data and theory from 2.0 < Ecm < 2.6 MeV, is a disagreement with a fundamental prediction of quantum theory, and it cannot be overlooked.

Furthermore, the modern measurements of gamma-ray data of the 12C(α, γ) reaction6 were analysed12,13, and large uncertainties were concluded. Large backgrounds in the measured gamma-ray spectra, induced, for example, by in-beam neutrons (e.g. see Fig. 6 of ref. 6), leads to large uncertainties in the measured angular distributions, and the extracted E1/E2 cross sections13. The large uncertainties deduced for the modern data6 and similar gamma-ray data15 lead to uncertainties in the R-Matrix analyses and extrapolation to stellar conditions10. We conclude that measurements of data with low backgrounds are needed for accurate extrapolation to stellar energies.

In this work, cross sections and angular distributions of the 12C(α, γ)16O reaction are obtained by measuring the inverse 16O(γ, α)12C reaction with gamma-beams and a Time Projection Chamber (TPC) detector. In sharp contrast to measurements with gamma-ray detectors, our data are measured with a vanishingly small intrinsic background. Furthermore, when measuring with a traditional array of gamma-ray detectors, an accurate knowledge of the relative efficiencies of the different detectors is crucial. Using our method, angular distributions are measured in one detector and the angular efficiencies are easily simulated. We obtain cross sections in agreement with current world data and further evidence the strength of our method with accurately measured angular distributions, leading to extracted ϕ12 values in general agreement with the trend predicted by unitarity. This serves as strong motivation to extend the initial measurements reported here to lower energies, where measurements are even more demanding.

Results

Detailed angular distributions of the reaction were measured with an unprecedented event-by-event resolution of ~2∘, and over almost a full range of polar angles, using a TPC detector with an optical readout (O-TPC). We note from the outset that our measurements agree with current world data for the total reaction cross-section. We also observe similarly shaped angular distributions, and, in contrast to some previous work, an agreement with the predicted variation of ϕ12. Our initial success in measuring angular distributions, reported here, serves as a “proof of principle” for our method and it encourages us to extend our measurements to lower energies, using a TPC detector16 and gamma-ray beams from the HIγS facility17,18 in the USA and the newly constructed ELI-NP facility19,20 of the EU.

Inverse process (detailed balance)

In our experiment, instead of measuring the fusion of α + 12C to form 16O, we use gamma-ray beams to measure the time reversed process: the 16O(γ, α)12C photo-dissociation reaction. The photo-dissociation cross-section is directly related to the capture cross-section via the principle of detailed balance: \(\sigma (\gamma ,\alpha )={k}_{\alpha }^{2}/2{k}_{\gamma }^{2}\times \sigma (\alpha ,\gamma )\) where \(\hslash {k}_{\alpha }=\sqrt{2\mu {E}_{{{{{{{{\rm{cm}}}}}}}}}}\), and ℏkγ = ℏω/c = Eγ/c, and is larger by a factor of ~50. We measure the tracks of the emanating α and 12C in a TPC detector operating with CO2 gas21. The 16O photo-dissociation events are unambiguously identified and measured in a ~ 4π geometry with high efficiency and a vanishingly small intrinsic background, as shown in Fig. 1 and in the “Methods” section (see below). Since the angular distributions are measured in a single detector, the relative corrections between different angles (which are essential for measurements with a gamma detector array) are not necessary. These factors allow us to measure accurate angular distributions of the 12C(α, γ) reaction, as we report here.

Fig. 1: Schematic diagram of the experimental setup used at the HIγS facility at Duke University.
figure 1

Not drawn to scale. The distance between the two mirrors is 53.73 m, between the FEL-electron collision point and the collimator is 60 m, and between the collimator and the O-TPC is 20 m. We show the FEL-electron ring, the Compton Backscattered gamma-ray, the O-TPC with the PMTs and the opto-electronic chain, discussed in the text. In the upper left corner we show a typical CCD image of a track and a PMT signal with the fitted lineshape of tracks from an α and 12C. The three-dimensional track is reconstructed with the (y, z) coordinate measured by the CCD image and the x coordinate calculated using the time projection signal measured by the PMT, as discussed in the text.

Full size image

Gamma beam

Intense, quasi mono-energetic photon beams were produced at the High Intensity γamma Source (HIγS) of the Triangle Universities Nuclear Laboratory, as discussed in ref. 17. Briefly, as depicted in Fig. 1, high-energy gamma beams were generated through the inverse-Compton scattering of an electron bunch with the free-electron-laser (FEL) photons, produced by the previous electron bunch17. The gamma-beam energy is determined by the FEL wavelength and the electron energy. A circularly polarised gamma beam was used in order to limit the wear of optical components. Data were taken using beam intensities of ~108γ/s with an energy spread of ~3% (~300 keV FWHM), at the following nominal beam energies: Eγ = 9.08, 9.38, 9.58, 9.78, 10.1, and 10.4 MeV.

The gamma beam was defined by a 15 cm long lead collimator with an 11mm-diameter aperture. It then passed successively through five thin scintillating paddles that were used for online measurements of the beam flux. The gamma beam then passed through air to reach a 5 μm kapton window, which isolated the gas in the O-TPC from atmosphere. This was followed by a strong permanent magnet, which deflected electrons produced in the window away from the gaseous target.

Downstream of the O-TPC, the flux was also measured by detecting neutrons from the d(γ, n)p reaction using an in-beam D2O target. Prior to each measurement, copper of various thicknesses was inserted to attenuate the beam by up to a factor of 105. The remaining photons were incident on an HPGe or NaI(Tl) detector, to measure the photon energy and flux, respectively. The 10-inch NaI(Tl) detector provided the absolute number of photons in the attenuated beam, which was used to cross-calibrate the relative flux paddle detectors. The energy of beam was obtained using a large, high-efficiency HPGe detector. The measured spectra were unfolded using a Monte Carlo technique22,23,24 to obtain the energy profile of the beam. The O-TPC detector was aligned with respect to the beam using a gamma camera and lead absorbers placed in the front and back of the detector as discussed in refs. 22,23,24.

The O-TPC detector

The detector operation is discussed in ref. 21. Briefly, it was operated with a gas mixture of CO2 (80%) and N2 (20%), at 100 Torr pressure. The active gas volume was 30 × 30 × 21 cm3. The 12 mm wide gamma beam entered the active volume through a 15mm opening. The N2 gas was used to produce the near-UV (338 nm) light detected by the PMTs and the opto-electronic chain. The resulting α and 12C from the photo-dissociation of 16O propagated through the gas and ionised atoms along their tracks, with an energy loss profile that was calculated using SRIM25, giving the lineshape shown in Fig. 1. The electrons drift upwards in the O-TPC under the influence of a ~200 V/cm electric field (2 V/cmTorr reduced electric field). The drift velocity of the electrons was measured to be 11.1 mm/μs, in agreement with calculations using Magboltz26.

The drift electrons were then multiplied by a stronger electric field of 20 V/cmTorr, giving rise to an avalanche and producing light. The light was detected by four photomultiplier tubes (PMTs) that surrounded the top of the O-TPC, as depicted in Fig. 1. The PMT signals were digitised with a 100 MHz 12-bit flash ADC. The PMT signals measure the arrival times of the drift electrons, recording the time projection of the track, which allows us to measure the x-coordinate, as shown in Fig. 1. At the same time, optical photons propagate through the opto-electronic chain and are focussed onto a CCD camera, which photographs the track. An image of a typical track from the 16O(γ, α)12C reaction is shown as the left figure in Fig. 1. The photograph allows us to measure the (in-plane) y–z coordinates of the track. The combination of the time projection (x) and CCD image (y,z) provides the three-dimensional coordinates of the track, from which the scattering angles were determined.

The anode signal, track length, and the total light signal were used to measure the total energy deposited, event-by-event, in the TPC. Tracks in the O-TPC were calibrated using the 3.183 MeV α particles from a standard 148Gd radioactive source. The energy resolution was measured to be 4% (FWHM). The particle identification was achieved by measuring dE/dx of the particles along the track. These lineshapes were used to determine the photo-dissociation events, as shown in Fig. 1.

Background

Events recorded by the O-TPC include Compton electrons, cosmic rays, 14N(γ, p), 16,18O(γ, α), and 12C(γ, α)8Be reactions. All background events from Compton electrons, cosmic rays, 14N(γ, p) and 18O(γ, α) were easily removed. Compton electrons deposit up to 100 keV total energy in the O-TPC, and were removed by an 800 keV electronics threshold on the anode signal. The majority of the cosmic events were removed by inspecting the track image and requiring an interaction point which is within ± 6 mm of the centre of the gamma-beam position. Events from the 14N(γ, p) reaction were removed by identifying the dE/dx lineshape of the proton, which differs significantly from the α particles. The 18O(γ, α) reaction events deposit 934.95 keV more energy than the 16O(γ, α) events, and were easily removed by measuring the total energy deposited in the O-TPC.

However, the energy deposited by the 12C dissociation events is only 112.85 keV lower than for 16O dissociation events. Hence, the 12C dissociation events could not be removed, since the beam resolution is ~300 keV. However, it is worth noting that when the 12C and 16O dissociation events are identified, as seen below, we could still measure the reaction with the detector resolution (~100 keV), since the beam energy could be evaluated event-by-event, by adding the known Q-value to the energy deposited in the detector by the reaction products. We did not carry out this procedure here, due to the current low statistics.

Lineshape analysis

The analysis of the 12C(γ, α) events was already reported27,28. Namely, the measured PMT lineshape was fitted with the lineshapes predicted for 12C and 16O dissociation events, and the resulting reduced χ2/ν of each best fit was derived. In Fig. 2, we show a two-dimensional lego plot of the obtained reduced χ2/ν, demonstrating a clear separation of the 12C and 16O dissociation events with vanishingly small intrinsic background . This was followed by a visual inspection of the remaining events to remove the small number of background events.

Fig. 2: Two-dimensional Lego plot for separation of 16O and 12C events.
figure 2

Here we plot the smallest deduced χ2/ν of fitting the PMT lineshape with the predicted lineshapes of 16O and 12C dissociation events.

Full size image

The reconstructed three-dimensional track allowed us to measure the scattering angle and azimuthal angle of each event. The CCD image measured the in-plane angle (α), and the PMT signal the out-of-plane angle (β), from which the scattering angle (θ) and azimuthal angle (ϕ) were calculated21: tanϕ = tanβ/sinα and cosθ = cosβ × cosα.

The efficiency for detecting 16O(γ, α) events was calculated using Monte Carlo simulations. We define the fiducial volume over ∣β∣ < 55∘ to exclude the region where the scattering angle carries the largest uncertainty. Over the selected fiducial volume, we obtained an event-by-event angular resolution better than 2∘. The fiducial volume was further restricted to ∣β∣ > 20∘ in order to provide the cleanest separation of 16O and 12C dissociation events. We note that the fiducial cuts lead to a vanishing (event-by-event) efficiency at the extremes of our angular distributions. This reduced efficiency is the small price paid for achieving cleanest data, in a region of angles where the cross-section is anyhow predicted to be small. The Monte Carlo simulation also accounted for edge effects, where one of the particles escapes the detector, meaning that only a section of the track is contained inside the fiducial volume. The efficiency rises from 0% to 60% for scattering angles, θ, between 20∘ and 55∘. The efficiency varies from 60% to 40% between 55∘ and 90∘. Uncertainties in the fiducial volume cuts, including the range straggle of the α and 12C, give rise to a <1% uncertainty in the overall efficiency correction. The event-by-event efficiency curves, and their small systematic uncertainties, are included in the electronic supplement.

Measured Cross Sections

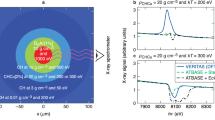
For each nominal beam energy Eγ = 9.38, 9.58, and 9.78 MeV, we accumulated ~500 16O photo-dissociation events. For Eγ = 9.08, 10.1, and 10.4 MeV, we accumulated ~100 events. The actual gamma-beam energies were measured using the attenuated beam and HPGe detector. The measured beam energies, with 30 keV uncertainties, were: Eγ(HPGe) = 9.01, 9.41, 9.61, 9.78, 10.10, and 10.43 MeV. The average of the previous world data (plotted in Fig. 3) was used to calculate the "effective energies"12, defined as the beam energy averaged over the FWHM of the broad gamma-beam, weighted by the global cross-section data. These are: \({E}_{\gamma }^{{{{{{{{\rm{eff}}}}}}}}}\) = 9.18, 9.45, 9.63, 9.80, 9.98, and 10.44 MeV corresponding to effective centre-of-mass energies \({E}_{{{{{{{{\rm{cm}}}}}}}}}^{{{{{{{{\rm{eff}}}}}}}}}\) = 2.02, 2.29, 2.47, 2.64, 2.82, and 3.28 MeV.

Fig. 3: The total reaction cross sections for the 12C(α, γ)16O reaction.
figure 3

We present the cross sections measured in this work, with a gamma-beam resolution of FWHM ~300 keV, compared with previous measurements using particle beams, with considerably better energy resolutions. The average of the shown previous world data was used to calculate the "effective energies" of our measurements with a broad gamma-beam. The error bars include 1σ SD statistical uncertainties due to the number of events measured at each energy, and uncertainties due to the beam energy spread.

Full size image

The integrated total beam intensity was measured using plastic paddle detectors, which were calibrated to absolute flux using the attenuated beam and 10" NaI(Tl) detector. There is an estimated 11% uncertainty in the beam flux, due to uncertainties in the copper attenuators. The counts at each beam energy, along with the beam flux and the efficiency, were used to calculate the total cross-section of the 16O(γ, α) reaction, from which we derived the cross-section of the 12C(α, γ) reaction, as shown in Fig. 3, using detailed balance. Using the method described in ref. 12, a correction factor was applied to the cross sections to account for the energy spread of the gamma beam. Systematic errors in the cross-section due to the beam energy uncertainty were estimated by examining the variation of the world data cross-section ±30 keV about each \({E}_{{{{{{{{\rm{cm}}}}}}}}}^{{{{{{{{\rm{eff}}}}}}}}}\). In Fig. 3, the error bars contain statistical uncertainties (4−15%) and uncertainties due to the beam energy, but exclude the global 11% uncertainty on the beam intensity.

An overall agreement with the previously measured total cross-section allows us to benchmark our results against previous measurements. It is essential to benchmark our data at energies above 2.0 MeV where the total cross-section (over the 1− resonance) is large and determined with high accuracy, with an agreement among measurements, as we show in Fig. 3. This benchmarking is required at this stage to further demonstrate the validity and strength of our method. It further motivates extending our measurements to lower energies, which is made possible by major investments in developing new initiatives18 and new facilities19,20.

Fitted angular distributions

The scattering angle in the laboratory frame was converted to the centre-of-mass scattering angle (shift < 2∘) and saved for each event. Data were then fitted with the partial wave decomposition2

$$W(\theta )= \, (3| {A}_{E1}{| }^{2}+5| {A}_{E2}{| }^{2}){P}_{0}(\cos \,\theta )\\ +\left(\frac{25}{7}| {A}_{E2}{| }^{2}-3| {A}_{E1}{| }^{2}\right){P}_{2}(\cos \,\theta )\\ -\frac{60}{7}| {A}_{E2}{| }^{2}{P}_{4}(\cos \,\theta )\\ +6\sqrt{3}| {A}_{E1}| | {A}_{E2}| \cos \,{\phi }_{12}\left[{P}_{1}(\cos \,\theta )-{P}_{3}(\cos \,\theta )\right],$$

by varying all three fit parameters: AE1, AE2, and ϕ12. The theoretical angular distributions were convolved with a Gaussian to account for a 2∘ angular uncertainty. All fits utilised the unbinned maximum likelihood method in order to negate the effects of angle binning and preserve our excellent angular resolution. The solid fit lines in Fig. 4 show the optimised partial wave decompositions convolved with the angular resolution, and include angular distribution attenuation factors (Qi) to account for angle binning2. For 20∘ bins, the obtained χ2/ν fit values are 0.17, 1.62, 1.51, 4.79, 5.89, and 0.85 at \({E}_{{{{{{{{\rm{cm}}}}}}}}}^{{{{{{{{\rm{eff}}}}}}}}}\) = 2.02, 2.29, 2.47, 2.64, 2.82, and 3.28 MeV, respectively. We note that the χ2 values are quite sensitive to the choice of angle binning. Due to the 2.64 MeV point’s proximity to the very narrow 2+ resonance at 2.68 MeV, this angular distribution was fit as a sum of a pure E2 angular distribution (40%) and the partial wave decomposition (60%)2. The relative contribution of the narrow E2 resonance was determined by examining the total energy deposited in the detector for all identified 16O events at this beam energy, which was fit with a sum of two Gaussians.

Fig. 4: Measured angular distributions of the 12C(α, γ)16O reaction.
figure 4

Plotted data include efficiency corrections and are presented at the shown "effective" centre-of-mass energies, with the three parameter fit (∣AE1∣, ∣AE2∣, and ϕ12) of the partial wave decomposition. The 1σ SD error bars show statistical uncertainties due to the number of events measured in each angle bin.

Full size image

Discussion

The obtained E1−E2 mixing phase angles (ϕ12) are shown in Fig. 5. The theoretically predicted ϕ122, discussed above, averaged over the beam energy resolution (FWHM ~300 keV), is also plotted in Fig. 5. Our measured ϕ12 values are in general agreement with the trend predicted by unitarity, exhibiting a strong variation across the 1− resonance region.

Fig. 5: The measured E1-E2 mixing phase angles.
figure 5

The measurements of ϕ12 are compared to the phase angles predicted by unitarity13, 14 (green line), and the prediction convolved with the gamma-beam energy resolution of FWHM ~300 keV (black line). The error bars show 1σ SD statistical uncertainties extracted from the fits to the angular distributions.

Full size image

In conclusion, we have presented a measurement of the 12C(α, γ)16O reaction, using an entirely different approach to previous experimental efforts, with different systematic errors. Our method permitted us to measure angular distributions spanning the energy region Ecm = 2.0−2.6 MeV, with improved precision, as for example in comparison with Assunção et al.6. This demonstrates the power of our experimental approach as a promising tool to investigate the cross-section of the 12C(α, γ)16O reaction at gamma-beam facilities.

Methods

The O-TPC allows us to reconstruct, in three dimensions, the events corresponding to the 16O(γ, α)12C reaction, by measuring all information pertaining to the reaction21. It measures the total energy deposited, the particle type, momentum, and angular distributions of the emitted particles. Thus, it allowed us to differentiate different reactions using three main tools: the photograph taken by the CCD camera, the total energy deposited by each event, and the time projection of each track. Further details of the analysis methods are provided below.

Image processing

At the beginning of each run, a photograph of the TPC was taken without the incident beam, which was subtracted from each image of a track. An example 12C + α track (zoomed), after this correction, is shown in Fig. 6a). The average background pixel value, \(\bar{p}\), and its standard deviation, σp, were quantified, and a threshold of \(\bar{p}+5{\sigma }_{p}\) applied. All pixel values below this threshold were set to zero. This results in Fig. 6a1). The resolution of the image was then degraded by a factor of 4 in each direction, resulting in Fig. 6a2). The hot pixels surrounding the main track correspond to partial tracks of electrons that scatter during the ionisation. These are removed by zeroing the pixel values in the compressed image that do not have 5 or more non-zero neighbouring pixels. This gives Fig. 6a3). Finally, with a mask provided by the remaining non-zero pixels, the original resolution of the image was restored to give Fig. 6a4).

Fig. 6: Image processing steps for the photo-dissociation and cosmic events.
figure 6

a 16O(γ, α)12C, b cosmic ray, c 14N(γ, p)13C.

Full size image

For highly ionising 12C + α and 14C + α tracks, corresponding to photo-dissociation of 16O and 18O, respectively, the final image consists of a single cluster of non-zero pixels on a blank background. Sparks in the TPC generate large numbers of clusters and are readily removed. Proton tracks induced by cosmic rays and from the photo-dissociation of 14N, shown in Fig. 6b, c, respectively, result in two or more clusters of non-zero pixels. This is because the stopping power of the proton is much lower than the 12/14C + α. This leads to low pixel values, closer to the background level. Only tracks with ≤2 clusters of pixels were taken for further analysis. A second data reduction cut was placed, demanding that the origin of each track lay within ±6 mm of the beam position. These two data analysis cuts removed ~70% of raw events.

From each image, the angle of the track in the y–z plane, relative to the beam direction, α, was extracted. The y–z coordinates of each pixel in a track were plotted and a linear fit was performed. The error on the gradient was extracted using standard techniques, which led to a typical uncertainty on the extracted α angle of ~2∘.

Track length and energy deposition

For each event, the two pixels with the largest separation were used to deduce the length of the track in the y-z plane of the coordinate system shown in Fig. 1. The x length was extracted from the time projection. These were combined to obtain the total track length. Additionally, the energy deposited by each event in the TPC was measured using the total pulse height. As expected, the track length and energy were correlated. A 2D plot of track length vs. energy is shown in Fig. 7. Regions of high intensity in this plot correspond to different reactions. With similar Q-values, the 12C and 16O photo-dissociation events appear within the same peak. The 18O photo-dissociation Q-value differs sufficiently (935 keV) that these events appear as a separate peak. A 2D software cut was placed around the 12C/16O peak to remove all other types of events.

Fig. 7: Histogram of track length vs. deposited energy.
figure 7

A clear separation of 16O/12C and 18O can be made.

Full size image

Lineshape analysis

In order to differentiate between the remaining 12C/16O events, and to extract the out-of-plane angle, β, a lineshape analysis was performed. The shape of the time projection depends on the stopping powers of the reaction products along with the β angle of the track. Tracks with large β result in long time projections, and those with small β give short time projections, corresponding to the track lengths in the x-direction. The SRIM software25 was used to determine lineshapes for each type of photo-dissociation event: 16O → 12C + α and 12C → 3α. These were then projected for β angles ranging between ±90∘. Each of the 180 β projections for both 12C and 16O were fitted to the time projection of each event. The β angle of the event was obtained through a χ2 minimisation. Differentiating between 12C and 16O events was achieved by examining the relative χ2/ν of the 16O → 12C + α and 12C → 3α best fits, as shown in Fig. 8. A histogram of the relative χ2/ν values was shown earlier in Fig. 2. A diagonal software cut through the centre of Fig. 2 removed the majority of 12C events from the data, preserving, on average, 95% of 16O events.

Fig. 8: Example lineshape fits used to distinguish 12C/16O events.
figure 8

a A typical 12C photo-dissociation time projection. b A typical 16O photo-dissociation time projection. The upper and lower plots in each panel show the best fits of 16O and 12C photo-dissociation theoretical lineshapes, respectively.

Full size image

Visual inspection

All remaining events underwent a visual inspection, to remove the small number of 12C events that escaped earlier software cuts. Along with the time projection, the longitudinal projection of the image of each track was also fitted with the 16O → 12C + α lineshape projected into the y–z plane, which provided a further dimension for event rejection.