Introduction

The study of quantum phase transitions is a rich topic of research in condensed matter physics. Quantum phase transitions, such as the plateau-to-plateau transition in the quantum Hall (QH) effect at high magnetic field, can be accessed by varying only one physical parameter (e.g., magnetic field) near absolute zero temperature1. The fundamental physics of quantum phase transition can be revealed by investigating its scaling behaviors at the crossing point of magnetoresistance at different temperatures2,3,4,5. The universal scaling behavior associated with such a phase transition is usually studied by characterizing a temperature-dependent resistivity/conductivity that is governed by a single exponent κ2,3,4,5. A similar feature of crossing magnetoresistance at different temperatures has also been observed in the QH to Anderson or Hall insulator transition6,7,8,9,10.

The quantum anomalous Hall (QAH) state, a zero magnetic field manifestation of the integer QH state, is also a topological phase of quantum matter11,12,13. Similar to the QH effect14, the QAH effect harbors dissipationless chiral edge states with quantized Hall resistance and vanishing longitudinal resistance15. These edge states are spin-polarized and their chirality is determined by the internal magnetization of the sample. The QAH effect was envisioned by Duncan Haldane11 and was first realized in magnetically doped topological insulator (TI) thin films13,16. To date, the QAH effect at zero magnetic field has been realized in the Cr- and/or V-doped TI systems13,16,17. An axion insulator state was recently realized in V-doped TI/TI/Cr-doped TI sandwich structures18,19. When the external magnetic field is swept between the coercive fields of the two magnetic TI films, i.e., the magnetizations of the two surfaces are antiparallel, the axion insulator state appears. The axion insulator is characterized by zero Hall resistance Rxy and a very large longitudinal resistance Rxx18,19. When the magnetizations of the two magnetic TI layers are driven by an external magnetic field from the antiparallel to parallel alignment, a quantum phase transition from an axion insulator to a QAH insulator has been demonstrated18,19.

In this study, we studied the scaling behavior of the quantum phase transition from the QAH insulator to the axion insulator in magnetic TI sandwich samples in the temperature range between 45 and 100 mK. We found the temperature dependence of the derivative of the longitudinal resistance Rxx on the magnetic field B evaluated at the critical field Bc follows a characteristic power-law behavior, i.e. \((\frac{{dR_{xx}}}{{dB}})_{B = B_{\mathrm{{c}}}} \propto T^{ - \kappa }\). The exponent κ is found to be 0.38 ± 0.02, smaller than the previously believed value κ ∼ 0.43 for the QH plateau-to-plateau transition4,20,21, but in good agreement with the recent high-precision numerical results on the Chalker–Coddington network model, which yields a correlation length exponent ν ~ 2.6 if the dynamic exponent p = 222,23,24,25. The experimental results are supported by theoretical arguments that the QAH to axion insulator phase transition, just like the QH plateau-to-plateau transition, can also be described using the Chalker–Coddington network model. In addition, by using a quasi-DC measurement circuit, we measured the two-terminal resistance R12 in the axion insulator regime and found it to be as high as 5.0 × 104 h/e2 (~1.3 GΩ) (Supplementary Note 1). This value suggests the highly insulating property of the topology-induced axion insulator state. By analyzing the temperature dependence of R12, we probed two different insulating behaviors in two adjacent temperature ranges, validating the reliability of our scaling study for the QAH to axion insulator transition.

Results

Sample structures and sample characterizations

The heterostructure samples used in this work are sandwiches with an undoped TI layer (5QL (Bi, Sb)2Te3 layer) inserted between two magnetic TI layers with a 3QL Cr-doped (Bi, Sb)2Te3 layer in the bottom and a 3QL V-doped (Bi, Sb)2Te3 layer on top (Fig. 1a). Specifically, the samples are 3QL (Bi, Sb)1.89V0.11Te3/5QL (Bi, Sb)2Te3/3QL (Bi, Sb)1.85Cr0.15Te3 sandwich heterostructures. These samples were grown on 0.5 mm-thick heat-treated SrTiO3 (111) substrate in a molecular beam epitaxy (MBE) chamber with a base vacuum of 2 × 10−10 mbar. The Bi/Sb ratio in each layer was optimized to tune the chemical potential near the charge neutral point. The transport studies were performed in two dilution refrigerators (Leiden 6 mK/14 T and 8 mK/9 T) with the magnetic field B applied perpendicular to the film plane. We used an excitation current of no more than 0.3 nA with a lowest trusted electron temperature ~45 mK (Supplementary Note 2). Six-terminal Hall bars with a bottom-gate electrode (Fig. 1b) were used for electrical transport studies.

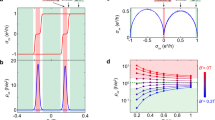
Fig. 1: QAH insulator to axion insulator transition in magnetic TI sandwich heterostructures.
figure 1

a Schematic of the magnetic/nonmagnetic/magnetic TI sandwich heterostructure. b Schematic of the Hall bar device used in electrical transport measurements. c Magnetic field B dependence of Rxy (blue) at Vg = Vg0 = +21.5 V. d B dependence of Rxx (red) and R12 (green) at Vg = Vg0 = +21.5 V. The four-terminal longitudinal resistance Rxx data are shown in red, whereas the two-terminal resistance R12 data are shown in green. When the R12 value is comparable with or higher than the impedance of parasitic capacitance of the measurement circuit, the R12 values measured with AC two-terminal method are not reliable and are represented by the green dashed lines. Rxy values in the highly insulating regime due to the inaccuracy caused by mutual mixing from huge Rxx are not shown. Measurements in c and d were taken when the environmental temperature of the dilution fridge is 15 mK.

Full size image

Figures 1c, d show the magnetic field B dependence of the Hall resistance Rxy (blue) and the longitudinal resistance Rxx (red) of the magnetic TI sandwich sample at T = 15 mK and Vg = Vg0 = +21.5 V. Here, Vg0 is the charge neutral point, determined by achieving the largest two-terminal resistance in the axion insulator regime. When the magnetization of the bottom Cr-doped TI layer is aligned in parallel with that of the top V-doped TI layer, the sample displays a perfect QAH state: at B = 0 T, Rxy(0) = ± h/e2 (within 0.07% of quantization) and Rxx(0) < 17 Ω. When the magnetization of the two magnetic TI layers is antiparallel, i.e., Bc1 < B < Bc2, where Bc1 and Bc2  are the coercive fields of Cr-doped and V-doped TI layers, the sample shows an axion insulator state19. As the axion insulator is a topology-induced insulating state, its Rxx value diverges. The traditional four-terminal measurement method is not suitable to measure such a large resistance. The two-terminal method with an AC signal source could deal with larger resistance but the largest resistance is still limited due to the unavoidable parasitic capacitance in the measurement circuit. Neither the traditional four-terminal measurement method nor the two-terminal method with an AC signal source provides reliable resistance values for such an insulating state. Therefore, we show four-terminal data only between 0 and ~10 h/e2, and AC two-terminal data from ~10 h/e2 to ~1000 h/e2 in Fig. 1c. At higher resistance range, we used a quasi-DC measurement scheme. This quasi-DC measurement method can yield reliable two-terminal resistance up to ~2 GΩ (~7.7 × 104 h/e2) (Supplementary Note 1). This method, however, is inconvenient for measurement when sweeping the magnetic field, because it requires very long measurement time and does not provide a “continuous” field-dependent resistance value as the AC lock-in technique.

QAH insulator to axion insulator quantum phase transition

When B > Bc2 > Bc1, the sample shows the perfect QAH state (Fig. 1c), so there must be a magnetic-field-driven quantum phase transition from an axion insulator to a QAH insulator near Bc2. To study the scaling behavior of this quantum phase transition, we measured the magnetic field B dependence of Rxx around Bc2 at different temperatures, as shown in Fig. 2a. All Rxx − B curves in the temperature range 45 mK ≤ T ≤ 80 mK cross each other at one point, specifically at the critical magnetic field B = Bc ~ 0.979 T. Bc separates the Rxx − B curves into two regions. For B < Bc, Rxx increases with lowering temperature, showing an “insulating” behavior. This “insulating” state corresponds to the axion insulator state with a large Rxx and zero Rxy near-zero temperature18,19. For B > Bc, Rxx decreases with lowering temperature, exhibiting a “metallic” behavior. This “metallic” state corresponds to the chiral edge channel of the QAH insulator state with zero Rxx and quantized Rxy at zero temperature13,16. This “metal” to insulator quantum phase transition is further demonstrated from the temperature dependence of Rxx measured at various magnetic fields (Fig. 2b). Figure 2c shows the value of Rxx at B = Bc for different temperatures. The Rxx value at T = 100 mK shows a clear deviation from the values measured at lower temperatures, which implies that we should confine our investigation of the scaling behavior of the QAH to axion insulator transition in the temperature range of 45 ≤ T ≤ 80 mK. It is noteworthy that one more transition from the QAH insulator to the axion insulator occurs at Bc1 ~ 0.20 T. We experienced instability problem of our magnet at low magnetic field region, so the data acquired near Bc1 cannot be used for scaling analysis.

Fig. 2: Temperature evolution of the QAH insulator to axion insulator transition.
figure 2

a Magnetic field dependence of Rxx at various temperatures. All curves cross at one critical point at Bc ~ 0.979 T. b Temperature dependence of Rxx at different magnetic fields. The sample shows “insulating” behavior for B < Bc and “metallic” behavior for B > Bc. At B = 0.980 T, Rxx is nearly independent of temperature. c Rxx value at the crossing point as a function of temperature.

Full size image

Scaling analysis of the QAH insulator to axion insulator transition

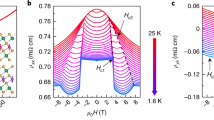
Next, we analyze the scaling behavior of the QAH to axion insulator phase transition. We follow the scaling analysis of the QH plateau-to-plateau transition2,3 and scale the B dependence of Rxx at different temperatures to a single parameter relation: \((\frac{{dR_{xx}}}{{dB}})_{B = B_{\mathrm{{c}}}} \propto T^{ - \kappa }\). Figure 3a shows the \((\frac{{dR_{xx}}}{{dB}})_{B = B_{\mathrm{{c}}}}\) at different temperatures plotted on log–log scale. The perfect linear fit in the temperature range 45 mK ≤ T ≤ 80 mK demonstrates the QAH to axion insulator quantum phase transition does show the universal scaling behavior. For T < 45 mK, \((\frac{{dR_{xx}}}{{dB}})_{B = B_{\mathrm{{c}}}}\) starts to deviate from the linear dependence, leading us to suspect that the real electron temperature of the sample is higher than the value registered by the thermometer on the mixing chamber in this temperature range (Supplementary Note 2). We note that κ can also be acquired in the Rxy analysis over the same temperature range, but it has a lower accuracy due to mutual mixing from huge Rxx (Supplementary Note 3).

Fig. 3: Scaling behavior of the QAH insulator to axion insulator transition.
figure 3

a Temperature dependence of \((\frac{{dR_{xx}}}{{dB}})_{B = B_{\mathrm{{c}}}}\) on log–log scale. The black dashed line is the linear fit. The inset shows Rxx as a function of the scaled magnetic field |B − Bc|·T−κ at different temperatures. b The two-terminal DC resistance R12 as a function of 1/T (bottom axis) or T (top axis) when the sample shows the most insulating state (i.e., B = 0.40 T). The vertical axis is on a log scale. The inset shows the measurement circuit we used to accurately measure the huge R12 of the axion insulator state.

Full size image

From the linear fit in Fig. 3a, we extracted a critical exponent κ ~ 0.38 ± 0.02. To further demonstrate the universal scaling behavior of the QAH to axion insulator transition, we used the one-parameter scaling relation \(R_{xx} = f[\left| {B - B_{\mathrm{c}}} \right| \cdot T^{ - \kappa }]\) to scale the B dependence of Rxx at different temperatures as a function of scaled magnetic field \(\left| {B - B_{\mathrm{c}}} \right| \cdot T^{ - \kappa }\)26,27. All curves except the curve at T = 100 mK are well-scaled to a single curve around the critical point. Figure 3a shows that including the 100 mK data for the scaling fit does not change κ, but if more high-temperature data points are included, a larger κ is found but not reliable (Supplementary Note 4). Below, we propose a theoretical picture to explain why the QAH to the axion insulator transition shares the same universality class of QH plateau-to-plateau transition and also demonstrate that κ ~ 0.38 ± 0.02 is in agreement with the latest high-precision numerical results of the correlation length exponent ν ~ 2.622,23,24,25.

Theoretical analysis of the QAH insulator to axion insulator transition

The top and bottom surface states in V-doped TI/TI/Cr-doped TI sandwich heterostructures can be described by the effective Hamiltonian:

$$H_{{\mathrm{t}},{\mathrm{b}}} = \pm \hbar v_{\mathrm{F}}\left( {s_xk_y\: - \:s_yk_x} \right) + [M_{{\mathrm{t}}.{\mathrm{b}}} + {\Delta}_{{\mathrm{t}},{\mathrm{b}}}\left( {\mathrm{r}} \right)]s_z + V_{{\mathrm{t}},{\mathrm{b}}}(r).$$
(1)

Here, sx,y,z are Pauli matrices acting on spin space, kx,y are wave vectors in x and y directions, and vF is the Fermi velocity. The Dirac mass term Mt,b and Δt,b(r) represent spatial-averaged and random parts of the exchange field in the z-direction due to the magnetization on top and bottom surfaces, respectively, where “t” and “b” denote the top and bottom surfaces. The random scalar potential Vt,b(r) denotes an inhomogeneous onsite potential inside the sandwich sample. In the clean limit, Vt,b(r) = 0 and Δt,b(r) = 0, so the Hall conductance of the top and bottom surfaces \({\upsigma}_{xy}^{{\mathrm{t}},{\mathrm{b}}} = {\mathrm{sign}}(M_{{\mathrm{t}},{\mathrm{b}}})e^2/2{\mathrm{h}}\) is determined by the sign of magnetization sign(Mt,b). The QAH phase with \({\upsigma}_{xy}^{\mathrm{t}} = {\upsigma}_{xy}^{\mathrm{b}}\) and the axion insulator phase with \({\upsigma}_{xy}^{\mathrm{t}} = - {\upsigma}_{xy}^{\mathrm{b}}\) require parallel and antiparallel magnetizations on the top and bottom surfaces, respectively. During the magnetization reversal process, the magnetization of one surface switches direction and a magnetic-field-driven quantum phase transition from the QAH insulator to the axion insulator occurs. Therefore, we consider only one surface in the following and denote it as top surface for concreteness. At the critical point of the QAH insulator to the axion insulator transition, the spatial-averaged magnetization of the top surface Mt = 0, whereas \({\Delta}_{\mathrm{t}}\left( {{r}} \right) \ne 0\) with an equal population of upward and downward magnetic domains (Fig. 4a). Now, the top surface state can be described by the random Dirac Hamiltonian with randomness in the mass Δt(r) and the scalar potential Vt(r):

$$H_{\mathrm{t}} = \hbar v_{\mathrm{F}}\left( {s_xk_y\: - \:s_yk_x} \right) + {\Delta}_{\mathrm{t}}\left( {\mathrm{r}} \right)s_z + V_t(r)$$
(2)

There exists a chiral edge mode between two regions with opposite masses for the Dirac Hamiltonian28. Due to a spatially varying random Dirac mass Δt(r), the random Dirac Hamiltonian Ht exhibits chiral edge modes confined to the zero-mass contours Δt(r) = 0 (Fig. 4a)28.

Fig. 4: Chiral edge modes along magnetic domain walls at the transition regime from the QAH insulator to the axion insulator and the Chalker–Coddington network model.
figure 4

a The chiral zero-energy edge states along contours of zero-mass domain walls of Dirac fermions. “+” and “−” denote domains of positive and negative masses of Dirac fermions, respectively. b The Chalker–Coddington network model on a square lattice. c At each node (red dashed square in b), a scattering matrix S describes the scattering from incoming (labeled by Zi = 1,3) to outgoing modes (labeled by Zi = 2,4). The chiral edge modes can be created by Dirac Hamiltonian with four domains of opposite Dirac masses labeled by ±Δ.

Full size image

Moreover, it is known that the Chalker–Coddington network model can be mapped to the random Dirac Hamiltonian28,29,30. Figure 4b shows the Chalker–Coddington network model on a square lattice structure. At each node (red dashed square in Fig. 4b), a scattering matrix S describes the scattering from incoming (labeled by Zi = 1,3) to outgoing channels (labeled by Zi = 2,4) (Fig. 4c). Here, the incoming and outgoing channels in the network model correspond to the chiral edge modes at zero-mass domain walls of the random Dirac Hamiltonian (Fig. 4a). Figure 4c shows the chiral edge channels at the four domains of opposite masses (labeled by ±Δt). All chiral edge channels in the network model can be created similarly and the Chalker–Coddington model is thus equivalent to the random Dirac Hamiltonian28,30. As the QH plateau-to-plateau transition (without electron–electron interactions) can also be described by the Chalker–Coddington model29, the quantum phase transition from the QAH insulator to the axion insulator must share the same universality class of QH plateau-to-plateau transition. Indeed, the critical exponent κ ~ 0.38 ± 0.02 revealed in our experiment is close to the generally accepted value κ ∼ 0.43 in the QH plateau-to-plateau transition4,20,21. The slight discrepancy of κ = p/2ν may result from a decreased dynamic exponent p or an increased correlation exponent ν. However, the theoretical problem of the QH plateau-to-plateau transition with strong electron–electron interactions remains open and a reliable method for calculating these exponents is yet to be established31,32,33,34,35,36. Recently, the high-precision numerical calculations show that the correlation length exponent ν of the Chalker–Coddington model is ~2.622,23,24 instead of ~2.33. It is noteworthy that the larger ν-value is also supported by the recent field theory analysis25. As a consequence, the critical exponent κ = p/2ν ∼ 0.38, if we assume p = 2 is in good agreement with the κ-value obtained in our experiment. Here, the exponent p = 2, exceeding the non-interacting value p = 1, may be induced by the appearance of the Coulomb interaction, similar to the discussion for the QH plateau-to-plateau transition31,32,33,34,35,36. In our samples, as the density of states of Dirac fermions is low, the long-range Coulomb interaction is unscreened and the triplet interaction channel is eliminated due to the strong spin-orbital coupling of TI materials32. We note that the QAH to axion insulator transition in magnetic TI sandwiches belongs to the QH-type instead of the Berezinskii–Kosterlitz–Thouless-type transition as we predicted in an individual magnetic TI thin film with random domains37. In such an individual magnetic TI thin film, the top and bottom surfaces are coupled and play a joint role, so this case is described by the Chalker–Coddington model with two channels37,38. In other words, the magnetization reversal during the QAH plateau-to-plateau transition occurs on both the top and bottom surfaces. This may be the reason why a different κ ~ 0.6 was found for the QAH to Anderson insulator transition39 and the QAH plateau-to-plateau transition40. However, the magnetization reversal in magnetic TI sandwich heterostructures during the QAH insulator to the axion insulator transition occurs on only one surface.

Discussion

A recent study on the quantum phase transition between a Chern insulator to an axion insulator driven by the external magnetic field in even number layers of topological antiferromagnet MnBi2Te4 reports a critical exponent κ ~ 0.4741. In the QH plateau-to-plateau transition, values of κ ranging from 0.15 to ~0.8 have been reported in the two-dimensional electron/hole gas samples2,5,42,43,44,45,46,47,48,49. Several factors can cause larger κ, e.g., the high concentration of ionized impurities or clustered impurities4. If the quantum percolation is turning into a classical percolation, κ is expected to eventually increase to 0.7531,50. It is noteworthy that the determination of κ is also sensitive to experimental issues, e.g., the small temperature regime where all curves crossing each other as shown in Fig. 2c. It is not appropriate to include the high-temperature data in our scaling analysis, which can cause a larger and nonreliable κ (Supplementary Note 4). We speculate that the appropriate amount of disorder in our sample might make κ as close as to the expected value based on the recent high-precision numerical results of the correlation length exponent ν ~ 2.622,23,24,25.

As noted above, the AC two-terminal measurement is not reliable when R12 > 103 h/e2. To measure R12 accurately in the most insulating regime, we employed the quasi-DC measurement method. The quasi-DC method uses a low frequency (f = 25.3 mHz) square wave as output, which can eliminate the influence of parasitic capacitance. The thermal voltage can be removed by taking the difference of the square wave generated current. Therefore, the quasi-DC method can provide more accurate resistance values when R12 is between ~25.8 MΩ (~ 103 h/e2) and 2 GΩ. By varying the magnetic field B, the most insulating state R12,max ~ 1.3 GΩ (~5.0 × 104 h/e2) was found at B = 0.40 T at T = 50 mK (Supplementary Note 5). The lowest Rxx in QAH state measured at 50 mK is ~20 Ω, so this suggests an order of ~108 ON/OFF resistance ratio in our magnetic TI sandwich samples.

Figure 3b shows the maximum two-terminal resistance R12,max of the axion insulator state measured at different temperatures between 50 mK and 220 mK. The logarithmic R12,max shows a linear dependence on T−1 with a slope ~333 mK below 95 mK and a steeper slope ~478 mK above 95 mK (Supplementary Note 5). We note that for T ≥ 100 mK, the scaling behavior starts to disappear (inset of Fig. 3a). Therefore, the insulating behavior at the high-temperature regime might involve the contribution from the classical thermal activation, whereas the low-temperature regime reflects solely the property of an unconventional insulating quantum state (i.e., axion insulator) favoring zero-temperature limit. This further validates our choice to carry out scaling behavior study between 45 mK and 80 mK.

To summarize, we analyzed the critical scaling behavior of the quantum phase transition from a QAH insulator to an axion insulator state in magnetic TI sandwich samples. We found that the derivative of the longitudinal resistance Rxx at the critical magnetic field follows a power-law dependence on temperature. Our experimental results combined with theoretical studies on the Chalker–Coddington model demonstrated that the QAH to axion insulator phase transition shares the same universality class with the QH plateau-to-plateau transition instead of the QAH plateau-to-plateau transition. Our work opens the door for further explorations of the critical behaviors of quantum phase transitions in topological materials.

Methods

Fabrications of magnetic TI sandwich Hall bar device

The magnetic TI sandwich heterostructure growth was carried out using a commercial MBE system with a vacuum ~2 × 10−10 mbar. The heat-treated insulating SrTiO3 (111) substrates were outgassed at ~530 °C for 1 h before the growth of the TI sandwich heterostructures. High-purity Bi (99.999%), Sb (99.9999%), Cr (99.999%), and Te (99.9999%) were evaporated from Knudsen effusion cells. During the growth of the TI, the substrate was maintained at ~240 °C. The flux ratio of Te per (Bi + Sb) was set to be >10 to prevent Te deficiency in the samples. To avoid possible contamination, a 10 nm-thick Te layer is deposited at room temperature on top of the magnetic TI sandwich heterostructures prior to their removal from the MBE chamber. Our prior study has demonstrated that the Te capping layer is much more insulating than the axion insulator in the low-temperature regime and plays a negligible effect in our electrical transport measurements51. We next scratched these magnetic TI heterostructure samples into a Hall bar geometry (Supplementary Note 6) using a computer-controlled probe station. The effective area of the Hall bar devices is ~1 mm × 0.5 mm. The electrical ohmic-contacts for dilution measurements were made by pressing indium spheres on the Hall bar. The bottom-gate electrode was prepared through an indium foil on the back side of the SrTiO3 substrate.

Transport measurements

Transport measurements were performed using two dilution refrigerators (Leiden 6 mK/14 T and 8 mK/9 T) with the magnetic field applied perpendicular to the film plane. The bottom-gate voltage was applied using a Keithley 2400. The four-terminal measurements are obtained by applying current from 1 to 2 (Fig. 1b), and Rxx = V34/I12 while Rxy = V35/I12. The excitation current in the dilution refrigerator measurements is ≤0.3 nA to suppress the heating effect. The lowest trusted electron temperature in our dilution transport measurements is ~45 mK (Supplementary Note 2). More details about the dilution measurements can be found in the Supplementary Information.

Effective model for theoretical analysis

In the Chalker–Coddington network model, we consider a square lattice of nodes and there are two incoming and two outgoing channels at each node. Here, the wave function for a particle on each link is represented by the probability amplitudes Zi = 1,…,4, where a random phase φi ∈ [0, 2π] is accumulated along with the link. Because of current conservation, \(|Z_1|^2 + |Z_3|^2 = |Z_2|^2 + |Z_4|^2\). Therefore, the two incoming channels (labeled by Zi = 1,3) and two outgoing channels (labeled by Zi = 2,4) at the node can be related by a 2 × 2 scattering matrix S

$$\left( {\begin{array}{*{20}{c}} {Z_2} \\ {Z_4} \end{array}} \right) = \left( {\begin{array}{*{20}{c}} {\cos \alpha } & {\sin \alpha } \\ { -\! \sin \alpha } & {\cos \alpha } \end{array}} \right)\left( {\begin{array}{*{20}{c}} {Z_1} \\ {Z_3} \end{array}} \right),$$

where α is a real parameter characterizing the tunneling. If α is identical for all nodes, the network model is critical at α = αc = π/4. In the experiment, the critical states are described by a random Dirac Hamiltonian with randomness in the mass Δt(r) and the scalar potential Vt(r): \(H_{\mathrm{t}} = \hbar v_{\mathrm{F}}( {s_xk_y\: - \:s_yk_x} ) + {\Delta}_{\mathrm{t}}\left( {\mathrm{r}} \right)s_z + V_t(r)\). Here, the links in the network model are related to the chiral edge modes along contours of zero-mass domain walls, whereas the scattering parameter α at the nodes can be obtained by solving the eigenvalue equation of Ht. In this Hamiltonian, sx,y,z are Pauli matrices acting on spin space, kx,y are wave vectors along x and y directions, and vF is the Fermi velocity.