Introduction

Bell’s theorem1, i.e., the incompatibility of quantum predictions with local hidden variable (LHV) models, is among the most influential results in quantum foundations. Despite being almost 60 years old, only recently the phenomenon of Bell nonlocality has been proven in a loophole-free manner in a series of independent experiments2,3,4,5,6. Apart from its fundamental importance, witnessing Bell nonlocality is at core of many applications in quantum information processing, ranging from quantum communication7 and distributed computing8, to quantum cryptography9, quantum key distribution10,11, and randomness generation12,13.

Indeed, the violation of a Bell inequality allows to bound the secure key rate that can be exchanged among distant nodes in a device-independent (DI) manner14,15,16, that is, with minimal assumptions on measurement apparatuses and relying exclusively on observed data. Most of these findings, however, only hold for the paradigmatic bipartite Bell scenario. Generalizations to the multipartite case have been proposed, for instance, based on multipartite entangled states, such as the Greenberger-Horne-Zeilinger states (GHZ)17. Notwithstanding, generation of such states on a photonic platform is still highly demanding18, affecting the near-term experimental relevance of device-independent communication protocols based on it. Accordingly, quantum networks of growing size and complexity aiming at the so-called quantum internet19,20 are much more likely to be composed of independent sources, each one generating small size entangled states but at much higher quality and rate.

The independence of the sources in a quantum network gives rise to a much richer set of correlations21,22,23,24,25,26,27,28,29,30 as compared with the standard Bell nonlocality. Not only the network scenario allows for nonlocality activation31 and less stringent detection efficiencies32, but also the emergence of truly new kinds of non-classical behaviors30. In spite of much recent theoretical advance, experimental implementations6,33,34,35 have been so far limited to the simplest possible quantum network, the bilocality model21,22. Such scenario is akin to the entanglement swapping experiment, involving two independent sources of entangled states, and only very recently was implemented while closing the locality and independence loopholes at the same time36.

In this work, we provide a proof of principle demonstration of the scalability of such networks, moving beyond the bilocality scenario, by considering up to four independent sources distributing entanglement among five nodes in a star-network topology. To ensure the independence of the sources, we employ four photonic setups located in four different laboratories, each one consisting of a source of entangled photons, pumped by a different laser, and a measurement station. Via this platform, we report the violation of polynomial chained Bell inequalities with an increasing number of parties and measurement settings, hence detecting the presence of nonlocal correlations among the nodes of multipartite networks. Our scalable approach could be useful both for demonstrating the nonlocality of topologically different scenarios30, as well as a testbed for device-independent protocols of information processing in quantum networks37.

Results

Causal modeling approach

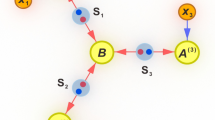
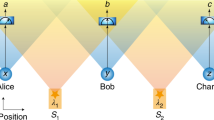
Topologically complex networks, composed of several sources and parties, are notoriously hard to characterize, especially if the independence of the sources is taken into consideration. A new approach has been introduced in the last few years to properly analyze these networks, which leverages on the theory of causal inference38. Indeed, the causal modeling approach39 has become a customary and powerful tool to represent classical and quantum networks27,40,41,42,43. In this framework, directed acyclic graphs (DAGs) provide both a graphical and a mathematical way to visualize causal structures (see Fig. 1). Each of the nodes stands for a random variable involved in the process, whose cause and effect relations are encoded by directed edges (arrows).

Fig. 1: Directed acyclic graph (DAG) representation for the star scenario with different number of sources.
figure 1

a Causal structure of the standard Bell scenario in which a single source Λ1 mediates the correlations between the two measurement stations with outcomes A and B and measurement choices X and Y, respectively. b DAG for the bilocal scenario where two independent hidden variables Λ1 and Λ2 distribute the correlations to three measurement stations with outcomes A, B, and C and choice of measurements X, Y, and Z, respectively. c, d DAG for the star-network with three and four independent sources Λ1, Λ2, Λ3, and Λ1\(,\ldots,\) Λ4, respectively. Each non-central measurement station has outcomes Ai (measurement choices Xi) and the central has outcome B (measurement choices Y).

Full size image

Bilocality scenario

The simplest quantum network beyond the paradigmatic Bell scenario (Fig. 1a) is the so-called bilocality scenario21,22 depicted in Fig. 1b, that is, a network with two independent sources Λ1 and Λ2. The three nodes, Alice, Bob, and Charlie, can perform measurements of different observables denoted by x1, x2, and y, chosen independently, with outcomes labeled as a1, a2, and b, respectively. Note that such independence in the choice of the measurements is also crucial in standard Bell tests6, in particular for the derivation of Bell inequalities22,27,41.

The aforementioned experiment is described by a probability distribution that, under the bilocality assumption, should be compatible with a bilocal LHV model, given by refs. 21,22

$$ p({a}_{1},{a}_{2},b| {x}_{1},{x}_{2},y)\\ \quad\,=\sum _{{\lambda }_{1},{\lambda }_{2}}p({\lambda }_{1})p({\lambda }_{2})p({a}_{1}| {x}_{1},{\lambda }_{1})p({a}_{2}| {x}_{2},{\lambda }_{2})p(b| y,{\lambda }_{1},{\lambda }_{2}).$$
(1)

Assuming that all these variables are dichotomous (with values 0 or 1), any correlation compatible with the bilocal model (1) should fulfill the following nonlinear Bell inequality:

$$S=\sqrt{| {I}_{1}| }+\sqrt{| {I}_{2}| }\le 1,$$
(2)

with

$${I}_{1}=\frac{1}{4}\sum _{{x}_{1},{x}_{2} = 0,1}\langle {A}_{1}^{{x}_{1}}{A}_{2}^{{x}_{2}}{B}^{0}\rangle ,$$
(3)
$${I}_{2}=\frac{1}{4}\sum _{{x}_{1},{x}_{2} = 0,1}{(-1)}^{{x}_{1}+{x}_{2}}\langle {A}_{1}^{{x}_{1}}{A}_{2}^{{x}_{2}}{B}^{1}\rangle ,$$
(4)

and \(\langle {A}_{1}^{{x}_{1}}{A}_{2}^{{x}_{2}}{B}^{y}\rangle\) being the expectation value of the measurements outcomes of the three nodes:

$$\langle {A}_{1}^{{x}_{1}}{A}_{2}^{{x}_{2}}{B}^{y}\rangle =\sum _{{a}_{1},{a}_{2},b = 0,1}{(-1)}^{{a}_{1}+{a}_{2}+b}p({a}_{1},{a}_{2},b| {x}_{1},{x}_{2},y).$$
(5)

In the last 2 years, inequality (2) has been experimentally violated in a number of photonic setups6,33,34,35,36. The first violation33 exploited polarization-entangled photons and the measurements performed in the central node B relied on a complete Bell-state measurement. Notably, bilocality violation can also be achieved with separable measurements22,23, as experimentally realized in refs. 6,35. The violation of bilocality is relevant, in particular, in the intermediate situation where local variable models can reproduce the observed correlations but bilocal models cannot33, namely one can violate a bilocal causality inequality even if the data admits a LHV model, where the independence of the sources is not taken into account. Accordingly, quantum states generating classical correlations in conventional scenarios can become powerful resources in a network, thus enlarging the capabilities to process information in a non-classical way.

n-locality in a star-network

The bilocality scenario can be extended to more complex networks, considering an increasing number of independent sources and involved nodes, as well as different topologies. In particular, a n-locality scenario involves n independent sources distributing correlations among the nodes. A topology that has received much theoretical attention is the star-network21,22,23,24,25, with n + 1 nodes interconnected by n independent sources, which contains the bilocality scenario as a particular case (see Figs. 1b–d). Each of the n peripheral nodes (called Ai) is connected through the source Λi to the central node of the network (called B). Labeling the measurements for B and external nodes Ai by y and xi and their outcomes by b and ai, respectively, a classical n-local hidden variable model implies that any classical observed distribution should be decomposable as

$$ p({a}_{1}\ldots {a}_{n}b| {x}_{1}\ldots {x}_{n}y) \\ \quad = \sum _{{\lambda }_{1},\ldots {\lambda }_{n}}p({a}_{1}| {x}_{1}{\lambda }_{1})\ldots p({a}_{n}| {x}_{n}{\lambda }_{n})p(b| y{\lambda }_{1}\ldots {\lambda }_{n})p({\lambda }_{1})\ldots p({\lambda }_{n}).$$
(6)

Considering that each of the nodes can perform k measurements described by dichotomous observables By (central node) and \({A}_{i}^{{x}_{i}}\) (external nodes), the n-local model implies the following nonlinear Bell inequality23:

$${S}_{n}^{k}=\mathop{\sum }\limits_{i = 1}^{k}| {I}_{i}{| }^{1/n}\le k-1$$
(7)
$$\,{\text{where}}\,\ {I}_{i}=\frac{1}{{2}^{n}}\mathop{\sum }\limits_{{x}_{1},...,{x}_{n} = i-1}^{i}\langle {A}_{1}^{{x}_{1}}\cdots {A}_{n}^{{x}_{n}}{B}^{i-1}\rangle$$
(8)

with \({A}_{i}^{k}=-{A}_{i}^{0}\). This is a generalization both of bilocality inequality (2), as well as of the so-called chained Bell inequality44, which are recovered in the case of n = 2 and n = 1, respectively. For this reason, we call inequality (7) the chained n-locality inequality. A larger number of measurement settings k for the chained form considered here provides advantages in device-independent protocols, either by reducing the experimental constraints for their violation45 or by leading to better security tests46. The set of classical correlations allowed by n-local models is shown in Fig. 3.

Let us now focus on the quantum violation of these inequalities, by highlighting a few important points. First, inequality (7) makes the explicit assumption that the sources of correlations are independent, thus any implementation of the corresponding causal structure should take that into account. For instance, the first experimental implementations33,34 adopted a single laser in order to generate the two pairs of entangled states, opening a loophole in the quantum violation of inequality (2). To avoid this loophole, as detailed below, in our experimental implementation, we used independent entanglement sources in separated laboratories. The second important point is that, in the star-network, Bob (the central node) has access to n independent physical systems, thus the most general measurement he can perform is a measurement in an entangled basis. In fact, theoretical results showing the activation of nonlocality in such networks31 rely on measurements on a GHZ basis. From the experimental perspective, however, such entangled measurements represent an extremely demanding task, as even measurements on a complete Bell basis cannot be implemented using linear optics, without resorting to hybrid or nonlinear approaches47,48. Nicely, however, it has been shown that the optimal quantum violation of the n-locality inequality can already be achieved if all measurements, including those of the central node in the networks, are made in separable bases22,23,24, thus avoiding the request to synchronize photons from different pairs as needed for projection on Bell basis. In summary, not only one can rely on independent sources but also make the simplest possible measurements to detect nonlocality in complex networks, paving the way of an experimentally scalable approach.

Let us now quantify the quantum violation of the n-locality inequality. By generating singlet entangled states and by performing the measurements reported in the Methods section, the upper bound of inequality (7) is the following:

$${S}_{n}^{k}=k\cos (\pi /2k),$$
(9)

that can be shown to be the optimal quantum violation24. We note that such bound does not depend on the number of nodes, n, but only on the number of measurements settings, k.

To experimentally implement the star-network causal structure depicted in Fig. 1d, we exploited the photonic platform of Fig. 2 with four independent polarization-entangled photon pair sources (a detailed description of the sources can be found in Supplementary Note 1). As shown in Fig. 2, three of the sources, that are pumped by different pulsed lasers located in the laboratories 1, 3, and 4, rely on spontaneous parametric down-conversion (SPDC) of type II, achieved through Beta-Barium Borate crystals, which emits degenerate photon pairs at 785 nm. Instead, the fourth source, located in laboratory 2, is pumped in the continuous-wave regime and generates photon pairs at 808 nm. This source scheme exploits a SPDC process of type II in a periodically poled KTP nonlinear crystal, placed into a Sagnac interferometer49. Thus, in our experimental implementation of the star-network (Fig. 1d), we strongly enforce the independence of the sources for the implemented causal structure.

Fig. 2: Experimental apparatus.
figure 2

a Four independent polarization-entangled photon pair sources and five measurement stations are available for the experimental realization of violation of the chained n-locality inequality (7) in a star-network configuration. b Physical location of the laboratories and scheme of the experimental apparatus. Distinct laboratories contain one source and one measurement station each, with the exception of Lab. 1, which also contains the central node B of the star-network on a separate optical table. c The entangled photon sources Λ1, Λ3, and  Λ4 are realized using a Beta-Barium Borate (BBO) crystal, pumped in a pulsed regime, which emits photon pairs at 785 nm using spontaneous parametric down-conversion (SPDC) of type II. The source Λ2 instead is pumped in continuous-wave regime and employs a periodically poled KTP crystal placed inside a Sagnac interferometer to generate entangled photon pairs at 808 nm via a type II SPDC process. Single photons are then measured in polarization using a half-wave plate (HWP) followed by a polarizing beam splitter (PBS).

Full size image

When a pair of photons is generated in each crystal, it is split: one photon is sent to the central node B, while the other remains within the laboratory Ai where it was generated, to be measured locally. The distribution of the shared photons among the nodes was accomplished by exploiting different optical fiber links. The maximum length of the fibers used was 25 m. At the output of each fiber, the polarization of the photon was properly compensated, to counteract unavoidable rotations along the path. In each laboratory, there is a measurement station, where polarization analysis can be performed by rotating a half-wave plate (HWP) placed right before a polarizing beam splitter (PBS). This apparatus allows each party to perform a number k of different projective measurements on their subsystem, since they are all represented by linear combinations of the Pauli matrices σx and σz. Accordingly, to perform the k measurement settings required to test inequality (7), each HWP was rotated to switch up to four different settings, in order to perform all the possible measurement combinations, which, in our star-shaped network, amount to 1024 combinations.

Photons arrival events were registered by single photons detectors (SPD) and a different time-tagger in each laboratory, and immediately sent to a central station where the synchronization and the coincidence detection took place. In particular, two detected photons were considered as simultaneous, provided that they were registered within a time, shorter than a given window. The results presented here were obtained using a coincidence time window of 80 μs, but a quantum violation was also observed for narrower values, up to 0.49 μs (see Supplementary Note 3).

We carried out the experiments for causal structures of star networks scenarios with n = 2, 3, 4 sources, whose independence is enforced by exploiting different independent pump lasers for SPDC processes occurring in dislocated laboratories, as mentioned above. Let us note that our setup relies, inevitably, on the measurement independence assumption, i.e., free choice of inputs measurements, as well as on the lack of correlations among the sources. Both such assumptions, indeed, cannot be enforced by any physical principle, but only be made less plausible as possible. For this reason, as already mentioned, we enforced the independence of the sources by adopting different laser devices, which, furthermore, were not relying on the same electric power source. Moreover, it requires the fair-sampling assumption, analogously to other most relevant experiments dealing with quantum networks6,33,34,35,36. In the end, given that the measurements were not performed providing space-like separation, we are assuming the lack of uncontrolled communication channels among the measurements stages, located in the separated laboratories.

In this framework, we managed to distinguish synchronous events by designing a sophisticated software, keeping track of the generation times of all of the four sources, as detailed in the Supplementary Note 3. On the other hand, by not requiring photons of different sources to interfere, we offered the possibility of using different types of sources within the same network and to have significantly higher generation rates.

Violations of n-locality with k = 2 measurement settings

The scheme previously described allows for the violation of n-locality with k different measurement settings. When considering the particular case of k = 2, the optimal measurement settings to perform at the external nodes and summarized in Eq. (10), are performed by the HWPs at measurement stages before PBS and with angles \({\theta }_{0}^{{\rm{A}}}={0}^{\circ }\) for choice xi = 0 and \({\theta }_{1}^{{\rm{A}}}=22.{5}^{\circ }\) for choice xi = 1. For the central node B, the HWP’s angles that project along states in Eq. (13) are \({\theta }_{0}^{{\rm{B}}}=11.2{5}^{\circ }\) for choice y = 0 and \({\theta }_{1}^{{\rm{B}}}=33.7{5}^{\circ }\) for choice y = 1. The corresponding results for this case are presented in Fig. 3 and can be extended by increasing the number of measurements k. The data in Fig. 3 show that the observed values are incompatible with the classical models for n-locality. Nonetheless, the obtained correlations are still compatible with a LHV model with a single shared source among all parties. To witness the non-classicality of the network correlations, the source independence has to be taken into account. In particular, for n = 2, we measured such parameters exploiting all of the six combinations of pairs among the four sources. The maximum observed value is \({S}_{\max }^{{\rm{obs}}}=1.218\pm 0.002\), violating the classical bound by 109 standard deviations. For n = 3, our setup allows for four triples of sources, achieving \({S}_{\max }^{{\rm{obs}}}=1.199\pm 0.004\), a violation of 50 standard deviations with respect to the classical bound. Finally, for n = 4 we obtained a value \({S}_{\max }^{{\rm{obs}}}=1.192\pm 0.005\), corresponding to a violation of 38 standard deviations. All the results for k = 2 are reported in Table 1.

Fig. 3: Experimental correlations represented in the I1, I2 plane (k = 2 settings).
figure 3

The purple, blue, red, and green regions represent the classical correlations for 1-, 2-, 3-, and 4-local scenarios, respectively. The 2-local case corresponds to the bilocal inequality while the 1-local reduces to the paradigmatic CHSH inequality. For n ≥ 2, the gray line bounds the correlations allowed by a local model with no assumption on sources independence. The green point represents the I1, I2 values measured in the case of n = 4 sources. The red, blue, and purple points represent the experimental values for all the possible combination of laboratories which are 4, 6, and 4 in the 3-, 2-, and 1-star cases, respectively. Error bars represent the standard error of the mean taken over different sequential acquisitions.

Full size image
Table 1 Experimental results for different number of sources n and k = 2 measurement settings.
Full size table

Violations of n-locality with k > 2 measurement settings

An increasing number of settings provides advantages in DI protocols based on the violation of Bell inequalities37. For that reason, we also consider the violation n-locality chained inequality (7) with an increasing number k of settings. In particular, we violated the 4-locality (n = 4) with up to four different measurement settings corresponding to a total 1024 combinations. Using this apparatus, we were able to violate the n-locality chained inequality (7) for the 2-, 3-, and 4-star-shaped networks scenarios, counting the 4-, 6-, and 8-fold coincidences events, in the case of k = 2, 3, 4 measurement settings. For the case of n = 2 and n = 3, each of these measurements was performed on every pair and triplet of the different four parties, thus allowing for six and four different combinations, respectively. The results for the different cases are presented in Fig. 4 as well as Table 2. For the particular case of n = 4 with k = 4, our results yields S = 3.157 ± 0.002, surpassing the classical limit by 71 standard deviations. The experimental values are fully compatible with the theoretical predictions where the noise of the whole system is taken into account (see Supplementary Note 2). Albeit with our apparatus we cannot close the locality loophole, because we would require an increase of the spatial distance among the parties, we approached such condition as much as possible through a reduction of the time window for the detection of coincidence events. Indeed, we present results also for narrower coincidence windows, up to 0.49 μs, which still allow for significant quantum violations (see Supplementary Note 3). Such reduction of the coincidence window lowers the probability of mutual causal influences among the parties in realistic experimental conditions.

Fig. 4: Experimental violation of the chained Bell inequality (7) for a 2, 3, and 4 sources n, depicted in different colors, and 2, 3, and 4 measurement settings k.
figure 4

Solid and dashed lines represent the classical and quantum bounds in (7), respectively, while dotted lines represent the expected value of the violation for noisy states. Measurement errors are not visible in the plot, numerical values are summarized in Table 2.

Full size image
Table 2 Experimental results for different number of sources n and measurement settings k.
Full size table

Discussion

Bell’s theorem is a conceptual cornerstone in our understanding of quantum theory that, with the rise of quantum information science, has also been turned into a novel tool for information processing. In spite of the key developments over the years in tasks such as quantum cryptography, self-testing, and entanglement certification, generalizations of Bell’s theorem beyond the simple single source scenario, in particular from the experimental point of view, are still an almost uncharted territory. That is precisely the aim of this work, to provide the first experimental implementation of a scalable quantum network consisting of an increasing number of independent entanglement sources, separated laboratories and measurement settings.

Here, we chose to investigate a topology that has received considerable theoretical attention lately, the star-network21,22,23,24,25, with a central node sharing an entangled state with n other peripheral nodes. In particular, we focused on the crucial task of certifying, in a device-independent manner, the presence of non-classical correlations among the nodes of the network. To this aim, we violated the chained n-locality inequality, an extension of the famous chained inequalities44 to the network case. The main strengths of this inequality are its adaptability to consider an increasing numbers of nodes in the network and of measurement settings for each party and also the possibility of achieving maximum quantum violation with separable measurements, a significant experimental advantage. We violated the chained n-locality inequality, thus ensuring the presence of truly non-classical correlations, by considering networks up to five nodes (four independent sources) and that each of the nodes could measure up to four different measurement settings.

In particular, networks of this kind might prove relevant, in the future, for the execution of multipartite protocols such as secret sharing50,51,52. Such task consists in a central node aiming to securely share a message with all the other parties, even if some of the receivers are untrusted. The crucial point, in this work, lies in the experimental realization of a quantum network of five stations, within which, the simultaneous presence of nonlocal correlations is certified. In this context, even though the ideal condition of space-like separated parties is not reached, we approximate such condition, by taking coincidence counts within a small time interval. Let us note that the limits on such interval are given by the rates of the sources. This approximate simultaneity, together with the independent sources, can certainly be considered as a very versatile tool for the future implementation of quantum networks and makes our platform easily adaptable to scenarios like the ones proposed by Lee and Hoban37, that could be applied to cryptographic tasks. Furthermore, for practical purposes and applications, the short time interval, that we adopt to detect simultaneous events, makes eventual communications among the parties less plausible in a plethora of realistic scenarios. To conclude, the novelty of our work stems from the presentation of the prototype of a scalable quantum network, going beyond the bilocality scenario, which allowed, for the first time, the violation of the chained n-locality inequalities. Moreover, the versatility of our platform can be exploited to study networks that are attracting growing attention, like the triangle network30 and the linear chain topology underlying quantum repeaters53.

Methods

Measurements performed

All of the four sources generate polarization-entangled pairs of photons in the Bell state \(\left|{\psi }^{-}\right\rangle =(\left|01\right\rangle -\left|10\right\rangle )/\sqrt{2}\). To obtain the maximum quantum violation of inequality (7), \(k\cos (\pi /2k)\), all the peripheral parties Ai must perform the following projective measurements on their subsystem:

$$\left|{\Psi }_{k,x}^{0}\right\rangle =\cos (x\pi /2k)\left|0\right\rangle +\sin (x\pi /2k)\left|1\right\rangle ,$$
(10)
$$\left|{\Psi }_{k,x}^{1}\right\rangle =\cos (x\pi /2k)\left|1\right\rangle -\sin (x\pi /2k)\left|0\right\rangle ,$$
(11)

for each setting xi = x. In turn, the central node B measures each of its n subsystems in the local basis:

$$\left|{\Phi }_{k,y}^{0}\right\rangle =\cos \frac{(2y+1)\pi }{4k}\left|0\right\rangle +\sin \frac{(2y+1)\pi }{4k}\left|1\right\rangle ,$$
(12)
$$\left|{\Phi }_{k,y}^{1}\right\rangle =\cos \frac{(2y+1)\pi }{4k}\left|1\right\rangle -\sin \frac{(2y+1)\pi }{4k}\left|0\right\rangle ,$$
(13)

for each setting yi = y, where the index i refers the system Bob shares with the i-th non-central part. The resulting measurement corresponds to \({B}_{y}={B}_{y}^{1}\otimes \cdots \otimes {B}_{y}^{n}\), where \({B}_{y}^{j}\) represent the measurement performed on each subsystem. Hence, to evaluate the quantum violation in (7), we need to perform k2n combinations of measurement settings, 2n for each term Ii appearing in (7).

Experimental details

For the experimental setups of Fig. 2, the three different pump lasers for sources 1, 3, and 4, with λ = 397.5 nm are produced by a second harmonic generation (SHG) process from a Ti:Sapphire mode-locked laser with repetition rate of 76 MHz. Photon pairs entangled in the polarization degree of freedom are generated exploiting type-II SPDC in 2-mm-thick beta-barium borate (BBO) crystals. Source 2, instead, employs a continuous-wave diode laser with wavelength of λ = 404 nm, which pumps a 20-mm-thick periodically poled KTP crystal inside a Sagnac interferometer, to generate photon pairs using a type-II degenerate SPDC process. The photons generated in all the sources are filtered in wavelength and spatial mode by using narrow band interference filters and single-mode fibers, respectively.

Coincidence counting

The photon detection events were collected and timed by a different time-tagger device for each party, located in the corresponding laboratory (see Fig. 2b). For each 1 s of data acquisition the events were sent to a central server, along with a random clock signal shared between all the time-taggers, which was used to synchronize the timestamps of events relative to different devices. To filter out part of the noise the raw data was first pre-processed by keeping only double coincidence events for each photon source, using a narrow coincidence window of 3.24 ns. Then coincidence events between multiple sources were counted every time one of such double coincidence event was recorded for each source in a window of 80 μs.