Introduction

As the counterparts of elementary particles of the standard model, the Dirac1,2,3,4 and Weyl5,6,7,8,9,10 fermions are found to exist in the form of quasiparticle excitations near Dirac and Weyl points, respectively. In Dirac semimetals, the Dirac points are fourfold degenerate with linear energy dispersion near the points11,12,13. By breaking either time-reversal or spatial inversion symmetry, one Dirac point splits into two Weyl points with twofold degeneracy14,15,16,17. Recently, several new fermions, which have no elementary particle counterparts, have been theoretically predicted as quasiparticle excitations near certain band-crossing points that are protected by several space-group symmetries18,19. In particular, triply degenerate point (TP) fermions have been predicted to exist in the electronic structure of a series of materials with tungsten–carbide (WC-) type crystal structure20,21,22,23. TPs can be viewed as an intermediate state between fourfold degenerate Dirac points and twofold degenerate Weyl points. Recent angle-resolved photoemission spectroscopy (ARPES) measurements have demonstrated the presence of TPs in MoP24 and WC25. Several magnetotransport studies on the WC-type materials, i.e., MoP26, WC27, and ZrTe28 have also been reported. However, the TP fermions are thought to make little contribution to the low-energy quasiparticle excitations because the TPs in MoP are far below the Fermi level (~1.5 eV). As a consequence, the exotic transport phenomena ascribed to TP fermions are difficult to test. Therefore, searching for new materials with TPs close to the Fermi level is essential to explore the exotic physics.

In this work, we theoretically predict that the trigonal layered PtBi2 hosts TP fermions that are close to the Fermi level. We performed angle-dependent magnetoresistance (MR) measurements under magnetic fields up to 40 T, and observed pronounced the Shubnikov–de Haas (SdH) quantum oscillations with a gigantic MR greater than 1.3 × 105% without any sign of saturation at 32 T and 1.8 K when the magnetic field is tilted approximately 30° from the c-axis to the a-axis in the ac plane. Similar oscillations in magnetization are also observed clearly due to the de Haas–van Alphen (dHvA) effect. Analyses of both SdH and dHvA oscillations reveal the existence of six principal Fermi pockets, in which three present signatures of relativistic fermion behavior with light effective masses. Combining the results of ab initio calculations and theoretical analyses, we demonstrate that the exotic experimental results can be reasonably understood within the framework of unique electronic structures around the TPs.

Results

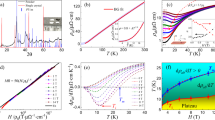
Crystal structure and schematic electronic structures

Trigonal layered PtBi2 can be also denoted Bi2Pt (hP9), which was experimentally reported in ref. 29. Our ab initio calculations using the crystal structure analysis by particle swarm optimization algorithm have identified that trigonal layered PtBi2 with space-group P31m (No. 157) has the lowest-energy structure among a series of PtBi2 compounds (See Supplementary Figures 1 and 12 and Supplementary Table 1). The crystal structure lacks mirror symmetry mz and inversion symmetry i due to the shrinking distortion of the top Bi layer and extension distortion of the bottom Bi layer, as shown in Fig. 1a. It also possesses rotational symmetry C3z and mirror symmetry my [see Fig. 1b, c]. The main features of the electronic structures from the ab initio calculations are schematically illustrated in Fig. 1d–f. There exists a pair of TPs along the C3z rotational axis H–K and (−H)–(−K) as shown in Fig. 1f, and they connect with each other by time-reversal symmetry, i.e., the two red dots connected by the red-dashed line in Fig. 1d. The band structures near one TP are shown in Fig. 1e. Apart from the H–K line in the my mirror plane, i.e., the H–K–Γ–A plane, the twofold degenerate bands split into two separate bands, which cross with the onefold band to form the linearly dispersive cross-points indicated by the colored spots in Fig. 1e, f. The cross-points form a track with different energy in the mirror plane in Fig. 1f. The features of the band structures can be roughly understood from symmetry arguments. For any point along the H–K line, the point group is C3v, which includes one unit operation, two threefold rotations, and three mirrors, i.e., {E, 2C2z, 3m}. The irreducible representations include one twofold E band and two onefold A1 and A2 bands. The ab initio calculations show that the twofold and onefold bands along the H–K line in Fig. 1e belong to the E and A1 irreducible representations, respectively. This is the reason they cross without opening a band gap, and the TPs are robust only if the symmetries in the C3v point group are not broken. Apart from the H–K line, for example, along the H–D line as shown in Fig. 1e, the C3z symmetry is broken, and the twofold bands have split into two bands with each band crossing with the onefold band to form doubly degenerate points. The doubly degenerate point is also robust and protected by mirror symmetry. More detailed analyses are shown below. The most remarkable feature of the electronic structure is that the energy window of the all cross-points including doubly and triply degenerate points lies in the range 0–0.3 eV when the Fermi level is set to 0 eV.

Fig. 1
figure 1

Crystal structure and schematic electronic structure. a Three-dimensional crystal structure of trigonal layered PtBi2. Top and bottom layers of Bi are labeled with different colors. b Side view of the lattice showing C3z rotation symmetry. Shrinking of top Bi layer and extension of bottom Bi layer result in a lack of an inverse center of trigonal layered PtBi2. c Top view of lattice showing my mirror symmetry; other two mirror reflection symmetries are obtained by rotating the top Bi atom. d Three-dimensional bulk Brillouin zone (BZ) and (001) surface BZ with high-symmetry points are indicated. Red spots indicate TPs. Red-dashed line connects a pair of TPs. e Schematic band structures along three different high-symmetry lines. Red spots at the crossing points along line H–K indicate the TPs. The light blue and green spots along H–Г and H–D are doubly degenerate points. Curves of mixed red and green color represent doubly degenerate bands (twofold) and those of single color represent nondegenerate bands (onefold). f Tracks of degenerate point around the Fermi level in H–K–Γ–A plane. Colors of the spots represent the energy relative to the Fermi energy EF. The point at line H–K is a TP, and the points off the H–K line are doubly degenerate

Full size image

Effect of magnetic field on resistivity

To experimentally characterize the topological properties related with the TP fermions in this compound, we synthesized high-quality single crystals of trigonal layered PtBi2 [see inset of Fig. 2a] by the metallic flux method. The lattice parameters are experimentally determined as a = b = 6.571 Å, and c = 6.159 Å. Figure 2a shows the temperature dependence of resistivity of PtBi2 at various magnetic fields applied along the c-axis from 2 to 300 K. The zero-field resistivity exhibits highly metallic behavior with ρxx(300 K) = 76.8 μΩ cm and ρxx(2 K) = 0.12 μΩ cm; thus, the residual resistance ratio (RRR) reaches R(300 K)/R(2 K) = 640, which is significantly higher than that previously reported in the literature30,31, revealing the high quality of our samples. When a magnetic field is applied along the c-axis, the resistivity increases and shows a plateau at low temperature. Similar behaviors have been observed in other semimetals, such as Bi32, WTe233, NbP34, and LaSb35.

Fig. 2
figure 2

Temperature-dependent resistivity and SdH oscillations in magnetic fields up to 16 T. a Temperature dependence of the resistivity ρxx measured under different magnetic fields. Inset: Optical image of a typical single crystal. b Magnetic field dependence of MR under different angles θ at 2 K. θ changes from 0° to 90°, which corresponds to the magnetic field tilting from the [001] to [100] direction. c Magnetic field dependence of resistivity at different temperatures when the field is applied along θ = 30°. Inset: Magnetic field dependence of MR measured at 32 T and 2 K. d SdH oscillatory component as a function of 1/B after subtracting a smooth background. Inset: Two frequency components Fα = 40 T and Fβ = 350 T extracted from oscillation patterns in the main panel. e Corresponding FFT spectra of the oscillations in the magnetic field range 8–16 T. Inset: Temperature dependence of the FFT amplitude. The solid curves are the fits to the LK formula. f Landau-level (LL) indices extracted from SdH oscillation plotted as a function of 1/B

Full size image

Figure 2(b) shows the MR properties measured at 2 K with magnetic fields up to 16 T using a physical property measurement system (PPMS, Quantum Design, Inc.) oriented at different angles, θ, tilted from the c-axis ([001]) to the [100] direction. It was seen that the MR presents strong angle-dependent behavior. When the applied magnetic field is oriented along the direction at approximately θ = 30°, the unsaturated MR, [(ρ(H)−ρ(0))/ρ(0)] × 100% is as large as 4.0 × 104 % at 16 T and ~1.3 × 105 % at 32 T [see the inset of Fig. 2c], and exhibits quasilinear field dependence, accompanied with significant the SdH quantum oscillations. These properties have recently been observed in topological semimetals with linear Dirac dispersion36,37. When the field orientation is away from θ = 30°, the sample shows a smaller MR with a quadratic behavior and weak quantum oscillations. The angle-dependent MR, as shown in Supplementary Fig. 2b, exhibits an unusual butterfly-shaped pattern, indicating the strong anisotropy of the electronic band structures. Detailed discussions about such a unique angle dependence of MR and SdH oscillations are presented in the Supplementary information.

Magnetoresistance oscillations

The strong SdH oscillations and giant linear MR around θ = 30° allow us to determine the nature of the band structures. Figure 2c shows the field dependence of resistivity measured at different temperatures with magnetic field B fixed along the direction θ = 30°. After subtracting a three-order polynomial background, the relative oscillatory component Δρxx versus 1/B is displayed in Fig. 2d. The Fast Fourier Transform (FFT) spectra of the oscillations in the magnetic field range 8–16 T are shown in Fig. 2e. The spectra reveal two principal frequencies, Fα = 40 T and Fβ = 350 T,with a harmonic frequency F2β = 700 T. According to the Onsager relation F = (ℏ/2πe)AF, where F is the frequency of oscillations, the cross-section of the Fermi surface, AF, is determined to be ~0.0038 and ~0.0331 Å−2 for α and β bands, respectively. The corresponding Fermi wave vector values kF are ~0.035 and ~0.103 Å−1, respectively.

Generally, the effective mass m* can be obtained from the fit of the temperature dependence of the FFT amplitude of the SdH oscillations. For a system with arbitrary band dispersions, the amplitude of the SdH oscillations can be described by the Lifshitz–Kosevich (LK) formula38,39:

$$\frac{{{\mathrm{\Delta }}\rho (T,B)}}{{\rho (B = 0)}} \propto f_m\sqrt B \frac{{2\pi ^2k_BT/\hbar \omega _c}}{{{\mathrm{sinh}}\left[ {2\pi ^2k_BT/\hbar \omega _c} \right]}}{\mathrm{exp}}\left( { - 2\pi ^2k_BT_{\mathrm{D}}/\hbar \omega _c} \right)\\ {\mathrm{cos}}\left[ {2\pi \left( {\frac{F}{B} + \gamma - \delta } \right)} \right]$$
(1)

Here, \(f_m = \left| {\frac{{\partial ^2A_F}}{{\partial k_H^2}}} \right|_m^{ - 1/2}\) is an extremal curvature factor that determines the strength of the angle-dependent MR about the direction of magnetic field, kH is the momentum along the magnetic field direction, kB is the Boltzmann constant, ωc = eB/m* is the cyclotron frequency with m*the effective cyclotron mass at the Fermi energy, and TD is the Dingle temperature. We fitted the data by the thermal damping term in the LK formula, i.e., \(\frac{{2\pi ^2k_BTm^ \ast /\hbar eB}}{{{\mathrm{sinh}}\left( {2\pi ^2k_BTm^ \ast /\hbar eB} \right)}}\), with 1/B being the average inverse field, as shown in the inset of Fig. 2e. The determined effective masses of the two bands are \(m_\alpha ^ \ast \approx 0.44 \pm 0.005\,m_0\) and \(m_\beta ^ \ast \approx 0.31 \pm 0.015\,m_0\) (m0 is the free electron mass), respectively.

We extracted the oscillation components of the α and β bands as shown in the inset of Fig. 2d based on the LK formula and plotted the Landau index n as function of 1/B in Fig. 2f. For a system of ρxx ≫ ρxy as trigonal layered PtBi2, in presence of multibands or large MR, the maxima of resistivity should be defined as integer generally40. According to the phase factor of the LK formula, i.e., \({\mathrm{cos}}\left[ {2\pi \left( {\frac{F}{B} + \gamma - \delta } \right)} \right]\), where γ is the Onsager phase factor that is related to the Berry phase through γ = 1/2 – ϕB/2π. In a conventional electron system, the Berry phase ϕB = 0, hence γ = 1/2. While for those massless Dirac materials with linear band dispersion, the nontrivial Berry phase is ϕB = π, which makes γ = 041. δ is the phase shift determined by the dimensionality of the Fermi surface with a value equal to 0 in 2D system and ±1/8 in the 3D case. The best linear fitting of n vs. 1/B gives the intercept value of nα = 0.23 ± 0.02 and nβ = −0.012 ± 0.05 for α and β band, respectively. The nβ locates between 0 ± 1/8 while nα is larger than 1/8. The deviation of the intercept from the ideal value n = ±1/8 for both bands should be due to the reason that the Fermi level does not cross the degenerated points. However, we also note that the phase shift can be affected by spin splitting and g-factor, the topological nature of these two bands can not be identified just by magnetotransport measurements at present.

To verify such an unsaturated MR being validated at even higher fields, we performed MR measurements on another sample S2 using a hybrid magnet with magnetic fields up to 40 T at θ ~ 30°, as shown in Fig. 3a. The SdH oscillations superimposed on the MR curves show more hyperfine structures than sample S1. After subtracting a three-order polynomial background, the relative oscillatory component Δρxx versus 1/B is displayed in Fig. 3(b). The FFT spectra of the oscillations as shown in Fig. 3(c) reveal five principal frequencies: Fα = 40 T and Fβ = 350 T with their harmonic frequencies F2β = 700 T and F3β = 1050 T, Fγ = 1240 T, Fη = 3030 T, and Fλ = 5110 T. The lower frequencies Fα = 40 T and Fβ = 350 T are the same as those of sample S1 measured with the PPMS. We determined the values of AF, the effective masses, and Fermi energy for the α, β, γ, η, and λ bands by fitting the amplitude of the SdH oscillations with the LK formula; the results are summarized in Supplementary Table 2. We have mapped the topology of the Fermi surface for the bands α, β, and γ through the data obtained from angle-dependent MR using a dc-resistive magnet up to 32 T on sample S1. The results suggest that the three bands show an anisotropic 3D Fermi surface, as shown in Supplementary Figs. 5 and 6.

Fig. 3
figure 3

SdH oscillations in magnetic fields up to 40 T. a Magnetic field dependence of resistivity at different temperatures when the field is applied along θ = 30°. b SdH oscillatory components as a function of 1/B after subtracting a smooth background. c Corresponding FFT spectra of oscillations

Full size image

Magnetization oscillations

Figure 4a shows the magnetization versus magnetic field of sample S3 measured at T = 2 K by a superconducting quantum interference device (SQUID) magnetometer with a magnetic field applied along the c-axis. Significant dHvA oscillations are observed and can be recognized from a field as low as 1 T. Figure 4b shows the FFT spectra of the dHvA oscillations. It can be seen that, in addition to the frequencies Fα = 41 T, F2α = 82 T, Fβ = 510 T, Fγ = 1220 T, and Fη = 3000 T being those obtained from the SdH oscillations, an additional extreme low frequency, Fδ = 3.8 T, is also found. (Here, the value of band β is larger than that obtained from the SdH oscillations, because the field is applied along the c-axis, while the SdH oscillations were obtained with a field applied at θ = 30°.) From the temperature-dependent dHvA oscillations, the effective mass of this δ band was extracted to be 0.21 m0 (see Supplementary Fig. 8). Following the customary practice of defining the LL index, the n-versus-1/B curve shows a linear dependence and gives an intercept nδ ~−0.48 ± 0.05. The physical parameters for the δ band are also included in Supplementary Table 2. Clearly, six main pockets in layered PtBi2 were recognized and three of them show light effective masses. In Fig. 4c, we summarize all the angle dependencies of the frequencies extracted from the SdH and dHvA oscillations of different samples. These frequencies are consistent between different samples and measurement techniques.

Fig. 4
figure 4

Magnetization oscillations and angle-dependent oscillation frequencies. a Magnetization curve measured at T = 2 K on sample S3 with magnetic field applied along the c-axis. b FFT spectra of dHvA oscillations. Inset: enlargement of FFT spectra. c Angle dependences of the frequencies extracted from the SdH and dHvA oscillations of different samples are labeled with different symbols. Theoretical calculated results are plotted with solid red lines

Full size image

Fermi surface topology

To fully understand the transport features ascribed to unique electronic structures near the cross-points, including TPs in layered PtBi2, we now analyze the calculated results in detail. Figure 5a shows six bands from the ab initio calculations. It is noted that both γ and λ have several satellite pockets. The satellite pockets from the γ band are too tiny to be experimentally identified, while the satellite pockets from the λ band, i.e., λ′ pockets, have been identified by the transport data, as shown in the Supplementary Information. Meanwhile, the calculation result shows that the γ pocket has a “dumbbell” shape (see Supplementary Fig. 6). Such anisotropy can be confirmed by the angle-dependent oscillation frequency shown in Fig. 4c, in which there are two branches of the γ pocket. The theoretically calculated frequencies are plotted for comparison with the full angular dependence of experimental frequencies in Fig. 4c. Consistency can be observed between the two results.

Fig. 5
figure 5

3D Fermi surface pockets and band structures. a Fermi surface pockets from ab initio calculations. Band structure along the direction of high-symmetry lines in the BZ (b) without and (c) spin–orbit coupling. Red circle along the H–K line labels the TP. The light blue circle along the H–Г line and the green circle along the H–D line labels the doubly degenerate points. Inset: Enlargement of the red rectangular boxes. The pink solid triangles (▲) and blue open squares (□) indicate the high-symmetry lines penetrating through the α and β pockets, respectively. d and e are magnified views of the blue-dashed rectangles in b and c, respectively. Bands are indicated by the relevant irreducible representations. f Spinless band along the H–K–Г–(−K)–(−H) line. g |A1〉 state split into two different substates by taking into account the spin degree of freedom due to the breaking of spatial inversion symmetry

Full size image

Band structures

Figure 5b, c shows the energy bands along high-symmetry lines in the BZ without and with spin–orbit coupling, respectively. The α and β pockets can be explicitly identified through the pink solid triangles (▲) and blue open squares (□), as shown in Fig. 5c. Both α and β pockets are hole type and they are linked by the TPs. The linear dispersion of the αpocket [near the red circles in Fig. 5c, e] is relevant to the TP along the H–K line, while the linear dispersion of the β pocket [near the light blue and green circles in Fig. 5c] is relevant to the doubly degenerate point in the mirror plane, such as the point along the H–Γ and H–D lines. Similar analyses can be applied to the γ, η, and λ pockets. It can be seen that theγ pocket is hole type, while the η and λ pockets are electron type, and all three pockets have quadratic dispersions with zero Berry phase. The tiny δ pocket also has a topologically trivial Berry phase, which is addressed in Supplementary Note 9.

Discussion

Now, we further consider the formation of the TPs. Focusing on the band along the H–K line indicated by the blue-dashed rectangles in Fig. 5b, c, their magnified counterparts are shown in Fig. 5d, e, respectively. In the absence of spin–orbit coupling, three bands exist. Among them, two are doubly degenerate and labeled with a red-dashed line and a green dashed-dotted line, while one is onefold and labeled with a solid blue line in Fig. 5d. To avoid the complexities induced by considering the specific sublattice and hybridization between s, p, and d orbitals, here we use the irreducible representations of the C3v point group to label the bands, i.e., |E±〉 and |A1〉. In the presence of spin–orbit coupling in Fig. 5e, the two degenerate |E±〉 states split into two doublets, i.e., (|E+,↑〉, |E−,↓〉) and (|E+,↓〉, |E−,↑〉). Such splitting is induced by the spin–orbit coupling. The |A1 state also splits into two states, i.e., |A1,↓〉 and |A1,↓〉 [as shown in Fig. 5g] when spin degrees of freedom are taken into account. Note that this splitting is induced by the absence of spatial inversion symmetry, and such splitting is similar to the splitting from Rashba spin–orbit coupling. Figure 5f, g clearly illustrates the splitting of the |A1〉 state induced by the absence of spatial inversion symmetry. The TP along the H–K lines is formed by the crossings between the doublets (|E+,↑〉, |E−,↓〉) and the singlet |A1,↑〉, while the TP along the (−H)–(−K) lines is formed by the crossings between the doublets (|E+,↓〉, |E−,↑〉), and singlet |A1,↓〉. The two TPs can transform into each other via the time-reversal-symmetry.

Finally, we point out the unique features of the TPs in trigonal layered PtBi2 in comparison with the TPs in WC-type materials. In the (001) surface BZ in Fig. 1d, we find that a pair of TPs is projected into two \(\bar K_1\) and \(\bar K_2\) points. The large separation of the two \(\bar K_1\) and \(\bar K_2\) points leads to the Fermi arcs that link the individual TPs on the surface and make them easily observable. In MoP, two TPs at the Γ-A line are projected onto the same Γ point, resulting in the disappearance of the Fermi arcs. Thus, the trigonal layered PtBi2 may be a candidate for the direct ARPES verification of TP fermions. Another remarkable feature is that the TPs in trigonal layered PtBi2 are quite close to the Fermi level, which provides possibilities to explore the properties of TP fermions.

In conclusion, we theoretically predict the existence of TP fermions in trigonal layered PtBi2. To verify their existence and explore their properties, we studied the quantum transport behaviors of high-quality trigonal layered PtBi2 single crystals systematically. When the magnetic field is tilted from the [001] to [100] direction, a giant linear magnetoresistance (1.3 × 105% at 32 T and 2 K) without any sign of saturation at approximately θ ~ 30°, accompanied by SdH oscillations, is observed. Our analyses of the SdH and dHvA oscillations revealed the existence of six principal Fermi pockets with three pockets showing light effective masses. Further analyses demonstrate that all of the main experimental results can be well understood in the context of the electronic structures around the TP fermions. The consistencies between the theoretical and experimental results support the emergence of TP fermions in trigonal layered PtBi2.

Methods

Single-crystal preparation

The single crystals of PtBi2 were synthesized via the self-flux method. The raw materials with molar ratio Pt:Bi = 1:8 were put into an alumina crucible, and then the crucible was sealed into a quartz tube under vacuum. The tubes were heated up to 800 °C, dwelled for 24 h, and then slowly cooled to 430 °C a rate of 2 °C/h. At this temperature, the flux was separated by a centrifuge.

Magnetotransport and magnetization measurements

The low-field MR was measured with standard four-probe using a 16 T PPMS (Quantum Design, Inc.). The magnetization measurements were carried out using a SQUID magnetometer. The MR at high magnetic fields (~32 and 40 T) were measured using standard a.c. lock-in techniques in either a He-3 cryostat on a dc-resistive magnet or a hybrid magnet at the high magnetic field laboratory (HMFL), Hefei, China.

Band structure calculations

The density functional theory42,43 calculations were performed by using the Vienna ab initio simulation package44, with Perdew–Burke–Ernzerhof45 type generalized gradient approximation in the exchange-correlation functional. The spin–orbit coupling was taken into account. The Brillouin zone is sampled by 12 × 12 × 13 k-point mesh. The Fermi surface is obtained by using Wannier 90 package46.

Data availability

The data that support the finding of this study are available from the corresponding authors upon reasonable request.