Introduction

The global internet traffic is constantly growing at a very high pace, sparking the general fear of a looming “capacity crunch”1,2 in the future. Silica-based solid-core fibres have limitations in high-capacity data transmission due to intrinsic material imperfections, i.e. Rayleigh scattering loss (RSL), chromatic dispersion and Kerr nonlinearity. While an optical loss level of 0.14 dB km−1 remains sustainable in present long-haul transmission systems and chromatic dispersion can be compensated by dispersion mapping, bulk material nonlinearity represents a more fundamental obstacle for capacity scaling, known as the nonlinear Shannon limit3,4. The contemporary scenario of space-division multiplexing (SDM)5 in association with multicore or multimode fibres cannot fully eliminate the capacity crunch concern, which encourages radical fibre innovations to coordinate with the ever-increasing data transmission demand.

Since its invention6, hollow-core photonic crystal fibres (HC-PCFs) have been considered as a potentially ideal channel for optical communications. The quasi-vacuum (i.e. no bulk core material) optical environment in HC-PCFs allow light to travel at its fastest speed without experiencing nonlinear effects and dispersion. Over the last two decades, HC-PCFs have developed into a versatile platform for a range of interdisciplinary applications in nonlinear optics7,8, ultrafast optics9, high power laser10, biophotonics11 and quantum optics12. However, the future of HC-PCFs in optical communications is still ambiguous due to several barriers, including their relatively high loss, limited bandwidth and their complex fibre structure. The current transmission loss record for hollow-core bandgap fibres (HC-PBGFs), whose light guidance relies on opening of the out-of-plane photonic bandgap underneath the light line13, is 1.7 dB km−1 at 1620 nm within a bandwidth of only 20 nm14 or a loss level of 5 dB km−1 at 1550 nm for a larger 200 nm bandwidth15. These marginal attenuations are intrinsically limited by the surface scattering loss (SSL)16, while the maximum achieved bandwidth is restrained by the upper limit of air-filling fraction17 and the surface mode anti-crossing18, indicating that significant improvement is difficult. Even with the state-of-the-art fabrication, the combination of the minimum loss and the maximum bandwidth in a single fibre is yet to be realized.

Hope has recently resurged with the emergence of hollow-core negative-curvature fibres (HC-NCFs)19, which originate from the Kagome-type broadband HCF in 200220. After the discovery of the hypocycloid-shape in the core-surround 21,22 and the simplified structure in the cladding23,24,25, HC-NCFs attracted massive interest in fibre optics community because: (i) SSL16 and surface mode effects26, the two main issues limiting performances of HC-PBGFs, can easily be suppressed in these fibres and, (ii) they offer extra degrees of freedom (e.g. core-surround shape) to control light leakage27. Typically, the inhibited coupling8 or anti-resonant reflecting optical waveguide (ARROW)28,29 guidance features broadband light transmission, sometimes with octave spanning bandwidth8, and lower spatial overlap of core mode with silica membrane, which results in a SSL level over one order of magnitude lower than in HC-PBGFs30,31. Hence, the main efforts in designing and fabricating HC-NCFs are diverted to the reduction of the confinement loss (CL) and the bending loss (BL). Current record is transmission loss of 7.7 dB km−1 at 750 nm and BL of 0.03 dB/turn at bend radius of 15 cm, obtained in a single-ring tube structure32. This level of transmission loss and BL are still far from telecom standard but some advanced NCF designs31,33, e.g. adding nested elements into the cladding tubes, optimistically indicate both ultralow CL and BL. However, with the current pressurized fibre drawing technique and with the consideration of fluid dynamics inside the furnace34, realization of such delicate structures with predicted optical performance brings substantial challenges due to the difficulty of precisely controlling the size and position of each tube element35,36,37. How to remarkably bring down CL and BL by introducing minimum complexity in both fibre design and fabrication becomes a crucial issue for the advancement of HC-NCF in optical communication technology.

In this letter, we report the design, fabrication and characterization of a new HCF structure referred to as hollow-core conjoined-tube negative-curvature fibre (HC-CTNCF or CTF for short). The design concept of this CTF is to conjoin twin (or triplet) anti-resonant (AR) tubes in the radial direction to efficiently confine light by multiple interfaces with sufficient consideration of fabrication simplicity. This new CTF combines almost all the qualities required for future optical communication systems and industrial up-scaling: ultralow transmission loss (2 dB km−1), wide bandwidth, low BL, single modedness and simple geometry.

Results

Fabricated fibre

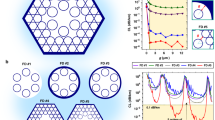
Figure 1a shows the fabricated CTF (see Methods). It consists of six untouched conjoined tubes encircling an air core with a diameter of D = 30.5 µm, each formed by conjoining two D-shaped air holes. As light attempts to leak out of the core, it successively encounters five different dielectric layers38 (or six glass:air interfaces), i.e. the negative-curvature glass layer with the thickness of t1 = 1.12 µm, the first layer of D-shaped air holes with the effective area of S1 = 270 µm2, the flat glass bar with the thickness of t2 = 1.06 µm, the second layer of D-shaped air holes with the effective area of S2 = 335 µm2, and the positive-curvature glass layer with the thickness of t3 = 1.16 µm (labelled in yellow in Fig. 1a). The three glass layers, having nearly the same thickness, operate in the second-order ARROW band28,29 at the wavelength centred around 1450 nm. The two layers of D-shaped air holes also stay in the first-order ARROW band thanks to careful control of sizes38. Accurate numerical simulation (the grey curve in Fig. 1c, see Methods) indicates that these five AR layers collectively bring down the CL by 62 dB from 1210 dB m−1 for a capillary bore (with the same core diameter) to 0.7 dB km−1 for the CTF at 1512 nm. Experimentally, a cut-back measurement (see Methods) from 330 to 5 m shows a transmission loss of 2 dB km−1 at 1512 nm, 3.7 dB km−1 at 1550 nm, and 13.4 dB km−1 at 1310 nm with the measurement uncertainty <0.1 dB km−1 (Supplementary Note 1). The experimentally measured threefold higher losses probably originates from structural non-uniformity in both the transverse and longitudinal dimensions. Additional effort in fabrication is still required to improve structural uniformity in order to achieve sub 1 dB km−1 loss level. The bandwidth with optical losses lower than 16 dB km−1 extends from 1302 to 1637 nm (covering half of the O band, and full E, S, C, L telecom bands), paving the way towards all the existing wavelength division multiplexing (WDM) transmission patterns (normal, coarse and dense WDM) in one HCF. In this measured spectrum, the loss peak at 1380 nm is due to OH− absorption which was introduced in the fibre drawing process and could be expelled by dehydroxylation in future trials39. Spectral oscillations at band edges, i.e. at 1281, 1295, 1318 and 1620 nm, probably stem from slight inconsistence of the AR conditions for different glass layers which could be optimized by more sophisticated control of the glass membrane thicknesses. Other spectral oscillations distributed over the whole transmission band are the result of Fano resonances40 introduced by the glass web connections. In this novel NCF design, instead of totally eliminating these connection points31, we choose to suitably arrange their positions to minimize the negative influences on both the CL and dispersion (Supplementary Note 2) and retain these connections to facilitate adding more AR layers in the cladding area.

Fig. 1
figure 1

Demonstration of an ultralow-loss broadband CTF. a Scanning electron microscope (SEM) image of the fabricated CTF with outer diameter of 295 µm. The yellow numbers label the five dielectric layers in the cladding. b Measured transmission spectra of the 330 m (black) and 5 m (blue) long CTF under the same launching condition. c Measured cut-back loss (black) and simulated loss (grey, including both CL and SSL). The spectral extension of the optical telecommunication O, E, S, C and L bands are also shown for comparison

Full size image

Bending loss

One fundamental characteristic of an optical fibre is that it allows flexible bending of the light. In a NCF consisting of one ring of ordinary tubes, there exists a tradeoff between the CL and the BL. Previously reported NCFs mostly pursue low CL by sacrificing BL with the adoption of a large core diameter (30–55 times the working wavelength)32,41,42, which represents a very small glancing angle at glass:air interfaces. Nevertheless, BL sensitivity is a crucial issue in practical applications and needs to be reduced. Special care was taken in this work to ensure a low BL by designing a core diameter only 20 times the central wavelength, comparable to that of 19 cell HC-PBGF43, while the ultralow CL is attained by the 5 AR layers in the CTF scheme (Fig. 1a). The increased number of AR layers combined with the reduced core diameter guarantee the simultaneous realization of ultralow CL and low BL. The measured BLs (see Methods) exhibit values as low as 0.7, 1.2 and 5 dB km−1 for bending radii R of 10, 7 and 5 cm at 1512 nm, respectively (Fig. 2). The simulated results (the black curves in Fig. 2, see Methods) follow a similar trend. For R = 5 cm, a loss peak at 1350 nm is predicted, coinciding with the critical bend-radius formula44. However, this peak was not experimentally observed probably due to the indeterministic bending orientations and the small variations of the bending radius along the total 390 loops. Under tighter bending (e.g. R < 5 cm), we found the fibre’s BL will be notably dependent on the bending direction45 which requires careful fibre handling. Since a bending radius of 7 cm is acceptable for most fibre applications, the BL-insensitive CTF represents a remarkable step toward practical applications of NCF technology.

Fig. 2
figure 2

Bending loss of the CTF. Measured and simulated bending loss spectra for a the bending radius R = 10 cm; b R = 7 cm and c R = 5 cm. Inset: Photo images of the fibre under test with the loop numbers of 200, 270 and 390, respectively

Full size image

Mode quality

The modal contents of the CTF have been characterized using spectral and spatial (S2) imaging techniques46 in the wavelength range 1510–1515 nm. For a fibre length L = 5 m and a bending radius R = 48 cm (nearly straight), the higher order modes (HOM) with group delays of 3.6, 9.2 and 10.8 ps m−1 can be discriminated with the multipath interference (MPI) values of −23, −26.5 and −21 dB, corresponding to LP11, LP21 and LP02 modes, respectively (Fig. 3a). When the fibre is bent with a smaller radius, these HOM peaks are suppressed. At R = 10 cm, only the peak of LP02 mode is visible with the MPI value of −25 dB. The HOM contents could be fully eliminated by increasing the fibre length to L = 15 m as shown in Fig. 3b, where no HOM is discernible for both the central launching condition and a deliberately 10 and 15 µm lateral offset launching condition, representing a HOM MPI value >27 dB (limited by the noise floor of the supercontinuum source). Simulation shows CL values of 0.7, 556, 58 and 150 dB km−1 for LP01, LP11, LP21, and LP02 modes at 1512 nm, respectively (in a straight state), with the lowest HOM extinction ratio of 79 (~19 dB) for the LP21 mode. In HC-PBGFs, intentionally designed shunt cores47 are needed for single modedness. In our CTF, the two D-shaped air holes, having the similar effective indices with the circular tubes of diameter d = 17.5 and 20 µm (hence equivalent d/D of 0.57 and 0.66, see Supplementary Note 3), naturally act as the shunt elements by resonantly out-coupling the core HOMs48. The CTF therefore could be regarded as a quasi-single-mode fibre for the length >15 m. With more subtle adjustment of the position of the glass bar inside conjoined tube, few mode guidance might be achieved in CTF, which would make them compactible with SDM transmission systems.

Fig. 3
figure 3

S2 analysis of the CTF. The typical multipath interference (MPI) vs. differential group delay (DGD) curve for a L = 5 m fibre with the bending radius of R = 48, 16 and 10 cm in central launch condition; b L = 15 m fibre with R = 48 cm in central, 10 µm offset and 15 µm offset launch conditions The insets show the reconstructed mode profiles

Full size image

Discussion

According to the multi-layered model38, in an annular/Bragg HCF, adding one glass layer gives rise to two extra glass:air interfaces. In optimal condition, this could drastically decrease the CL by 22.4 dB (Supplementary Note 4). In a NCF, additional influence from the shape of the glass wall should also be taken into account. In Fig. 4, simulation results indicate that, in comparison with the single-ring NCF, the added glass bar inside the conjoined tube reduces the CL by ~20 dB down to sub 1 dB km−1. More surprisingly, a two-bar version CTF with five conjoined tubes and the same core diameter could further decrease the CL to below 0.1 dB km−1. From a fabrication perspective, such a 2-bar CTF structure is much more feasible than previously proposed ultralow-loss NCF designs, e.g. nested tube structures31,33, where the Fano resonances caused by the glass connection points are deliberately avoided but the mechanical stability of the whole structure is less optimized. Our CTF architecture offers another probably more practical route. The simulated CL of the 2-bar CTF (the purple curve in Fig. 4) in the wavelength range of 1325–1585 nm exhibits Fano-induced oscillations varying from 0.005 to 0.09 dB km−1, well below the SSL level (0.11–0.24 dB km−1). The minor influence to the total loss spectrum (the pink curve in Fig. 4) verifies that the CTF has suppressed the detrimental effects of the glass connection points to a sufficient extent. The size/ellipticity of the conjoined tube and the positions of the glass bars could be regarded as new and unexplored engineering paradigm for future advanced NCF.

Fig. 4
figure 4

Comparison of simulated loss spectra of several designs. Schematic illustrations of the single-ring NCF, 1-bar CTF and 2-bar CTF are shown in the right column in black, blue and purple, respectively. They share the same inscribed core diameter of 30.5 µm. The physical dimensions for each dielectric layers from inward to outward are, t = 1.12 µm, d = 17.5 µm (same effective index with S1 in CTF, see Supplementary Note 3) for the single-ring NCF; t1 = 1.12 µm, S1 = 270 µm2, t2 = 1.06 µm, S2 = 335 µm2 for 1-bar CTF; t1 = 1.12 µm, S1 = 381 µm2, t2 = 1.06 µm, S2 = 564 µm2, t3 = 1.06 µm, S3 = 381 µm2 for 2-bar CTF. The CLs are plotted in solid curves. The SSL and the total loss are plotted in dashed curves. Average reductions of 20 dB from the single-ring NCF (~70 dB km−1) to 1-bar CTF (~0.7 dB km−1) and 15 dB from 1-bar CTF to 2-bar CTF (0.02 dB km−1) in CL are estimated from simulation

Full size image

In Table 1, a comprehensive comparison of CTF, single-ring NCF and PBGF is listed. As shown in this work, the first trial of the 1-bar CTF has already approached the optical loss level of PBGF with a 20-year history. The demonstrated fibre sample is far from the limit of CTF technique and can be further improved. Similar to the single-ring NCF, CTF could further expand the transmission bandwidth as long as thinner and more uniform glass membranes are realized. Other advantages of CTF over PBGF include lower modal spatial overlap with glass (thus higher laser damage threshold), lower dispersion, and higher group velocity, likening light propagation in vacuum. Although the BL of the CTF is still higher than the PBGF, it is already small enough for industrial application. The remaining issue lies on realization of polarization-maintaining fibres. Further investigation is needed but hybrid transmission bands49 could represent a potential solution.

Table 1 Optical performance comparison of PBGF, single-ring NCF and CTF
Full size table

To summarize, we believe the CTF demonstrated in this work, with a minimum loss of 2 dB km−1, transmission window spanning across O-L telecom bands, low bending sensitivity, high mode purity and simple structure, represents a new milestone towards the long-term goal of ultralow-loss HCF. Although the first trial of CTF has not achieved better optical losses than the more mature PBGF, it has, however, proved that neither CL, BL nor SSL are obstacle for further development of HCF. This makes HCF a strong contender to solid-core silica fibre for high-capacity data communication, where loss and nonlinear tolerance are of equal importance for enhancing spectral efficiency via higher order modulation formats. The next targets would be to upscale the fibre length to multi-kilometre and to optimize the loss level to sub −1 dB km−1, which both look viable in CTF and its variants.

Methods

Fibre fabrication

To make conjoined-tubes, a custom-made high purity thin slab is inserted into the centre of a thin glass tube and is drawn into capillaries. The latter is then drawn into fibres using the conventional two-stage stack-and-draw technique.

Simulation of CL

The CL is numerically simulated using a finite-element mode solver (COMSOL Multiphysics), with optimized mesh size and perfectly matched layer50. The geometrical parameters were determined from the SEM image of the fibre with small adjustment within the range of uncertainties. The SSL is calculated following the equations in ref. 31.

Transmission loss measurement

The loss spectrum is obtained by cut-back measurement from 330 to 5 m. A supercontinuum (SC) source is butt-coupled to the 330 m long CTF (inside a fibre splicer), which is looped on the drum during the measurement with R = 16 cm. The output end of the CTF is connected to an optical spectral analyzer (OSA) using a magnetic clamp bare fibre adaptor (OZ Optics). Multiple cleaves at the output end show little variation in the recorded spectra for both the 330 and 5 m long fibre samples. More details about the analysis of measurement error can be found in Supplementary Note 1.

BL measurement

The CTF’s bending loss is measured by comparing the transmission spectrum of a bent fibre with that of a quasi-straight fibre (with the bending radius R = 50 cm). The supercontinuum source is butt-coupled to a 130 m CTF, which is bent to R = 10, 7 and 5 cm with 200, 270 and 390 loops sequentially (shown in the insets of Fig. 2). The bending loss spectra are demonstrated in Fig. 2.

BL simulation

BL is calculated by substracting the loss of a bent fibre from that of a straight fibre. The conformal mapping technique is implemented for the simulation of a bent fibre51. Two bending orientation directions, θ = 0° and 30° (θ as defined in ref. 41), are taken into account and the two results are averaged.

S2 measurement

The CTF’s S2 measurement is performed by launching the SC light into a 5 m (15 m) CTF with different bending radii and the launching conditions are described in the caption of Fig. 3. At the output cross-sectional plane, a SMF28 fibre records the spectra at different positions. The spectra were acquired by an OSA with the resolution of 0.05 nm. The near filed image was also monitored by a camera.

Data availability

The data that support the findings of this study are available from the corresponding author upon reasonable request.