Introduction

The development of valleytronics offers great potential in providing an additional degree of freedom that may open a new field beyond that of electronics and spintronics1,2. Over the past four decades, the presence of valleytronics in cubic systems such as Si3,4, AlAs5,6, and even recently, diamond7 has been established, where strong biases have been utilized to distinguish three pairs of degenerate conduction band minima. More recently, developments in two-dimensional (2D) materials have sparked the revival of valleytronics with enlightening research in graphene and various transition metal dichalcogenides (TMDCs)8,9,10,11,12. By probing odd layers8,9 of such low dimensional materials, and/or the application of certain experimental conditions, such as cryogenic temperatures10,11 and/or strong electric12 or magnetic field13, valleytronic behavior can be detected. However, such requirements inevitably pose a plethora of practical challenges that create a high barrier in advancing the technology towards practical applications.

We report a model system in Tin(II) sulfide (SnS) that may present plausible solutions to the above-mentioned issues and provide a completely new platform with easy access to the valley degree of freedom. SnS has a Pmcn crystal structure with an orthorhombic unit cell comprising puckered layers analogous to black phosphorus14,15. This puckered-layered structure, gives rise to the high anisotropy between the armchair (y) and zigzag (x) directions, which then gives rise to directional behavior manifested in, for example, the Raman response16, in-plane electronic mobility17, and photoactivity18 of SnS. Such anisotropy can also be correlated to the reciprocal space. It has been demonstrated theoretically that, in monolayer SnS, there exist a pair of non-degenerate band gaps along the in-plane orthogonal high symmetry axes, which correspond to the y and x axes in real space19. These band gaps correspond to two sets of distinct valleys that can be directly accessed using light with different linear polarizations.

Here, we demonstrate the presence of two non-degenerate band gaps in bulk SnS that are, analogous to the monolayer case, effectively valleys with high polarization degrees. We follow the convention of labeling high symmetry k-points as previously reported in ref. 20, where the ΓY and ΓX axes in the reciprocal unit cell correspond to the y and x directions in real space. Density-functional theory (DFT) calculations yield a band structure with two local band gaps along the ΓY and ΓX axes, denoted by Eg,ΓY and Eg,ΓX, respectively. These valleys along the ΓY and ΓX axes have unique advantages over previously reported conventional and 2D valleytronic materials: (i) we can clearly differentiate two sets of valleys in multiple ways without the application of external bias fields as the valleys are inherently non-degenerate, enabling direct access to the valley degree of freedom, (ii) the valleys can be effectively polarized under ambient conditions without low temperature, and (iii) bulk SnS was utilized and can be prepared with relative ease, as it is not limited by the requirement of centrosymmetry breaking as in certain 2D materials.

Results

Material preparation and characterization

The crystal structure (left and right panels) and the corresponding band structure (middle panel) of SnS is presented in Fig. 1a, with the high armchair–zigzag anisotropy further emphasized in Fig. 1b. We synthesized bulk SnS microplates via physical vapor deposition (PVD) and observed their flat morphology and Sn:S ratio of 1:1 via SEM-EDX (Fig. 1c). Raman spectra (Fig. 1d) show four characteristic peaks of SnS (three Ag and one B3g). We utilized prior understanding of the polarization-dependent Raman responses16,21 of orthorhombic IV–VI monochalcogenides to determine the armchair (y) and zigzag (x) directions of the sample (Fig. 1d, Supplementary Fig. 1 and Supplementary Note 1). (The directions can also be directly observed from the optical microscopy image (Fig. 1c) due to the preferred growth facets in bulk crystals as demonstrated using surface energy arguments21.)

Fig. 1
figure 1

Structure and characterization of SnS. a (Left and right) Three-dimensional (3D) representation of the Brillouin zone of SnS superimposed on a Pmcn unit cell made up of puckered layers, showing the collinearity of the x(y) axis in real space and the ΓX(ΓY) axis in reciprocal space. (Middle) Quantitative view of the SnS band structure along X–Γ–Y, demonstrating the valley properties due to selectivity in linearly polarized light at non-degenerate band gaps. b Molecular structure of SnS visualized along the three main orthogonal axes projected from the unit cells presented in a, showing the correlation between the puckered arrangement of atoms and the high anisotropy along the y (armchair) and x (zigzag) directions. c Optical microscopy image of a SnS sample, showing a clear difference in angles made by the edges and ease of identifying the in-plane directions. Scale bar is 10 μm. Inset shows scanning electron microscopy (SEM) images and energy-dispersive X-ray spectroscopy (EDX) maps, demonstrating a flat surface and homogeneous distribution of Sn and S in equal ratio. d An example of Raman spectra obtained under parallel polarization, showing clear trends in intensities for the Ag and B3g modes, thus ascertaining the identity and orientation of the measured SnS sample

Full size image

Valley selectivity via absorption measurements

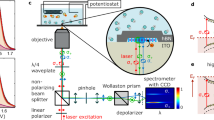
Figure 2 shows the optical setup configuration used for the reflection (R), transmission (T), and photoluminescence (PL) measurements, where the sample is rotated with respect to the polarization axis of the linearly polarized excitation light, giving an angle θ. (For simplicity, the armchair (y) direction, as previously determined, is set as θ = 0°.)

Fig. 2
figure 2

Schematic of the experimental setup for both PL and reflection measurements. Excitation was conducted using linearly polarized light on a sample that can be rotated to give an angle θ between the polarization axis and the armchair direction. A second polarizer was also placed either parallel or perpendicular to the excitation polarization for PL measurements

Full size image

We observe clear valley selectivity in the R and T spectra of SnS, shown in Fig. 3a, Supplementary Fig. 2, and Supplementary Note 2. The reflectivity of our sample remains stable at short wavelengths until the onset of fluctuations due to Fabry–Pérot interference oscillations22, which denotes the region where the absorption of SnS decreases rapidly. This agrees with the observation that the percentage of transmitted light is very small at wavelengths shorter than the oscillations. Distinct onsets are observed when the polarization of light is aligned to the armchair (zigzag) directions, respectively. From R and T, we obtained the corresponding wavelength-dependent absorption coefficients23,24, and hence the Tauc plots25 of SnS when aligned to the two axes directions. The Tauc plots (Fig. 3c) give band-gap values of 1.48 and 1.28 eV, clearly distinguishing the two sets of valleys.

Fig. 3
figure 3

Reflection and transmission measurements of SnS. White light with wavelengths ranging from 700 to 1050 nm illuminated the sample without a second polarizer. a Reflection spectra along armchair and zigzag directions, showing clear trends on the onset of Fabry–Pérot oscillations at 775 and 950 nm, respectively, demonstrating the presence of valley selectivity in absorption. b Band structure of bulk SnS. The directions y and x are the armchair and zigzag directions, respectively. The respective irreducible representations of the conduction band, valence band, and position vectors, as well as the point group and twofold rotation axis, are indicated for each direction. c (Left axis) Tauc plots of both directions, with fitting lines denoting band gaps at 1.28 and 1.48 eV for zigzag and armchair directions, respectively. (Right axis) Degree of optical dichroic anisotropy, αzigzag/αarmchair. d Absorption coefficients of both directions under different temperatures, showing a distinct increase in band gap with decreasing temperature. e Vashni plot extracted from the temperature varying absorption measurements

Full size image

The selectivity of the absorption to polarized light can be quantified by the degree of optical dichroic anisotropy, which is given by the ratio αzigzag/αarmchair in our system (Fig. 3c). We see that SnS is able to maintain a αzigzag/αarmchair > 200% for a range of more than 0.2 eV and also possesses a maximum αzigzag/αarmchair > 600%. This wide range and large anisotropy may potentially relax the strict requirements usually present in valleytronic systems.

Because the valley behavior in SnS is due to polarization-dependent band-gap transitions, we further investigated the temperature dependence of the absorption coefficients along the two axes directions. From the plots of α (Fig. 3d), we see that the x-axis intercept increases with decreasing temperatures, which agrees with the typical behavior of band gaps. The variations in band-gap values with temperature are then fitted with the Varshni equation26 (Fig. 3e), which shows that the valley behavior extends across the whole investigated temperature range.

Selection rules for valley selectivity

Our observation of polarization dependence of the valley peaks in bulk crystals of SnS has also been theoretically verified. Using an approach similar to the analysis of monolayer SnS19,27,28,29, we demonstrate, via DFT results, the application of light polarized along the y-axis (x-axis) in real space will predominantly allow excitation of electrons from the valence band to the conduction band of the band gap along ΓY (ΓX) in reciprocal space.

As shown in Fig. 3b, the band structure of bulk SnS has two nearly direct transitions T y and T x . There are no transitions with energies near band gap along the ΓZ and ZU directions.

In the electric dipole approximation, the transition rate between two states due to the electromagnetic perturbation is proportional to \(\left| {{\hat{\mathbf i}} \bullet {\langle} i{\mathrm{|}}{\mathbf{r}}{\mathrm{|}}f}{\rangle} \right|^2\), where |i,f〉 denote the initial and final states, \({\hat{\mathbf i}}\) is the direction of the polarization of the electromagnetic wave, and r is the position operator. Depending on the composition of the |i〉 and |f〉, the matrix element 〈i|r|f〉 can have non-vanishing components only in certain directions. If the component in the direction i vanishes, the transition is not allowed. Therefore, by calculating the dipole matrix elements 〈i|r|f〉, one can predict which light polarization is required for the state transition. The transition is allowed if the direct product of the irreducible representations that correspond to i, f, and r gives the fully symmetric representation A1.

With this in mind, we first turn to the transition T x . The wavevector (k) points along the ΓX direction (except for Γ and X) belong to the C2v point group, with the twofold rotation symmetry axis C2 along x. Looking at the composition of the valence and the conduction bands, we see that they are composed of orbitals, which are even under the reflection across the xy plane, but odd with respect to the xz plane, and therefore correspond to the B2 representation of the C2v point group. The character table for C2v (adapted from ref. 30) is presented in Table 1. The position vector x possesses an A1 representation. Thus, for light polarized along x, 〈i|x|f〉 transforms as B2⊗A1⊗B2 = A1 and the transition is allowed. However, for light polarized along y, the transition is forbidden because 〈i|y|f 〉 transforms as B2⊗B1⊗B2 = B1.

Table 1 Character table for C2v point group for k points along ΓX (top) and ΓY (bottom)
Full size table

Similarly, the k points along the ΓY direction (except for Γ and Y) belong to the C2v point group but in this case with C2 along the y direction. By analyzing the signs of the orbitals that make up both the conduction and valence bands under various symmetry operations of the C2v point group, both bands were determined to belong to the A1 representation. Therefore, the transition is allowed for light polarized along y (A1 representation), as 〈i|y|f〉 now transforms as A1⊗A1⊗A1 = A1 instead. The transition is now forbidden for light polarized along x (B2 representation) because 〈i|x|f〉 now transforms as A1⊗B2⊗A1 = B2.

Valley selectivity via photoluminescence

An important figure of merit for valleytronic systems is the polarization degrees of the valleys, P11, which can be obtained by comparing the PL intensities at each valley. For previously demonstrated 2D systems, P2D has been defined as11:

$$P_{2{\mathrm{D}}} = \frac{{I\left( {\sigma _ - } \right) - I(\sigma _ + )}}{{I\left( {\sigma _ - } \right) + I(\sigma _ + )}},$$

where \(I\left( {\sigma _{ - / + }} \right)\) represents the PL intensities that are left and right circularly polarized, respectively. As the transitions at each valley in SnS is purely symmetry-dependent, PL at each valley will be linearly polarized along the same direction as the incident excitation-allowed light; PL conducted under parallel polarization will thus demonstrate valley selectivity for both absorption and emission and can be used to calculate the polarization degrees between the non-degenerate valleys. We define these as the intervalley polarization degrees:

$$\begin{array}{ccccc}\\ P_{{\mathrm{intervalley}},\,\Gamma X} = \frac{{I_{\Gamma X\parallel }\left( {\theta = 90^\circ } \right) - I_{\Gamma Y\parallel }\left( {\theta = 90^\circ } \right)}}{{I_{\Gamma X\parallel }\left( {\theta = 90^\circ } \right) + I_{\Gamma Y\parallel }\left( {\theta = 90^\circ } \right)}}{\mathrm{and}}\,\\ P_{{\mathrm{intervalley}},\,\Gamma Y} = \frac{{I_{\Gamma Y\parallel }\left( {\theta = 0^\circ } \right) - I_{\Gamma X\parallel }\left( {\theta = 0^\circ } \right)}}{{I_{\Gamma Y\parallel }\left( {\theta = 0^\circ } \right) + I_{\Gamma X\parallel }\left( {\theta = 0^\circ } \right)}}\hskip2.1pc \end{array}$$

where Pintervalley,i and Ii||(θ) stands for the polarization degree and PL intensity under parallel polarization for the i valley, respectively. Pintervalley,i are lower-bound estimations of the polarization degrees at both sets of SnS valleys due to certain logical simplifications made because of the non-degeneracy of the valleys. Such estimations include (i) comparing the intensities at the two valleys at 0° and 90° only under parallel polarization, (ii) taking the intensity values from the fitted polar plots, and (iii) using normalized intensity at each valley to eliminate issues of differing emission and detection efficiencies at the two valleys.

For PL measurements, linearly polarized lasers at 532, 633, and 785 nm were used to excite the SnS plate, giving similar emission peaks, which confirm the PL nature of the peaks. A polarizer was placed before the detector parallel to the excitation polarization (Fig. 2) and the sample was rotated to give different θ values.

We observed two PL peaks at 817 and 995 nm (Fig. 4). The polarization dependence of both PL peaks is clearly observed. The 817 nm peak maximizes at the polarization that minimizes the 995 nm peak, and vice versa, demonstrating a 90° phase shift between the peaks and hence the orthogonality in polarization selectivity of the two sets of valleys responsible for such PL emissions. (Note the existence of a higher energy, broad peak centered at 760 nm that has similar polarization dependence as the 817 nm peak. We attribute the presence of the two PL peaks to the presence of two peaks in the valence band along the ΓY direction, which can potentially allow two transitions of different energy values from the conduction band to the valence band. As both peaks lie on the ΓY axis, they will obey the same selection rules. This observation may also be caused by other higher order transitions which is beyond the scope of discussion in this work.) For the peaks at 817 nm (ΓY) and 995 nm (ΓX), we calculated Pintervalley,ΓY = 92% and Pintervalley,ΓY = 62%, respectively.

Fig. 4
figure 4

Photoluminescence measurements of SnS. a Deconvolutions of PL peaks under parallel polarization at 817 and 995 nm give clear, opposite trends with respect to sample orientation, demonstrating the identity of the valley positions. b, c Polar plots of PL peak height intensities conducted under parallel polarization for the valleys at 817 and 995 nm, respectively (squares). The single circular data point in each polar plot represents the PL peak height intensities of the corresponding measurements conducted with the second polarizer perpendicular to the incident light when the respective axes are aligned to the excitation polarization. The black dashed arrows connect the two data points before and after the 90° rotation of the second polarizer, showing 95% and 96%  decrease in PL intensities at the ΓY and ΓX  valleys respectively. These show valley selectivity in the absorption and emission of light

Full size image

The uniqueness of SnS as a valleytronic system lies in the non-degeneracy of the valleys, which, unlike previous 2D materials, can be viewed as not only having an additional valley degree of freedom, but one that can be accessed via two routes; between valleys and within valleys, thereby allowing an additional valley selection pathway for applications. For anisotropy within each valley, we define the intravalley polarization degrees:

$$\begin{array}{ccccc}P_{{\mathrm{intravalley}},\,\Gamma X} =\frac{{I_{\Gamma X\parallel }\left( {\theta {\mathrm{ = }}90^\circ } \right) - I_{\Gamma X \bot }\left( {\theta {\mathrm{ = }}90^\circ } \right)}}{{I_{\Gamma X\parallel }\left( {\theta {\mathrm{ = }}90^\circ } \right) + I_{\Gamma X \bot }\left( {\theta {\mathrm{ = }}90^\circ } \right)}}{\mathrm{and}}\\ P_{{\mathrm{intravalley}},\,\Gamma Y} = \frac{{I_{\Gamma Y\parallel }\left( {\theta = 0^\circ } \right) - I_{\Gamma Y \bot }\left( {\theta = 0^\circ } \right)}}{{I_{\Gamma Y\parallel }\left( {\theta = 0^\circ } \right) + I_{\Gamma Y \bot }\left( {\theta = 0^\circ } \right)}}\hskip2pc \end{array}$$

We identified the maximum PL intensities for both valleys (θ = 90° for ΓX and θ = 0° for ΓY) and conducted PL measurements under cross polarization (second polarizer placed perpendicular to excitation polarization (Fig. 2)), obtaining near-zero Ii⊥(θ) for both valleys (Fig. 4b, c), giving Pintravalley,ΓY = 95% and Pintravalley,ΓX = 96%, respectively. The large difference between Ii⊥(θ) and Ii||(θ) at each valley further reinforces the notion of strong valley emission anisotropy at both valleys. Our P values are amongst the highest polarization degrees reported, even rivaling those of monolayer dichalcogenides obtained from experiments conducted at cryogenic temperatures8,11,31.

Our PL results are complemented by the absorption data, which provides a definite proof of the selectivity towards the absorption of polarized light, specifically backing the claim that the valleys indeed possess selectively for both absorption and emission of light.

Discussion

In summary, we have conducted reflection/ transmission and PL measurements that show the existence of two band gaps at 1.28 and 1.48 eV in bulk SnS under ambient conditions and without additional biases. These band gaps reside along the ΓY and ΓX axes in reciprocal space, are strongly and solely excited by y- and x-polarized light and also mostly emits y- and x-polarized light, respectively, effectively serving as non-degenerate valleys. Our system also has, nominally, the advantage of superior optical dichroic anisotropy of up to 600%, intravalley polarization degrees of 96 and 95%, respectively, and intervalley polarization degrees of 62% and 92%, respectively. Such attributes make SnS a model system and allow future work to be conducted with more controllability and potential for practical applications.

Methods

Sample preparation and characterization

SnS microplates were either mechanically exfoliated or synthesized using physical vapor deposition (PVD) on fused silica substrate (for PL measurements) and on Si/SiO2 substrates (for reflection measurements). 99.5% −4 mesh SnS powder from Alfa Aesar is used for both exfoliation and PVD synthesis. The PVD method used is adapted from ref. 16.

SEM was conducted using a FEI Quanta 3D FEG system.

Optical measurements

PL measurements were conducted using a Horiba Jobin Yvon LabRAM ARAMIS automated scanning confocal Raman microscope under 532, 633, or 785 nm excitation.

Reflection and transmission measurements were carried out using our in-house setup coupled to a Horiba iHR320 spectrometer and Horiba Synapse CCD detector. We used the reflection spectrum of a clean gold substrate (transmission spectrum of a clean fused SiO2 substrate) to normalize the reflection (transmission) spectra by considering the theoretical reflection (transmission) of gold (fused SiO2) obtained from reported literature values32,33. Absorption coefficients, α, were obtained from the normalized reflection, R, and transmission, T data via \(\alpha = - \frac{1}{d}{\mathrm{ln}}\left( {\frac{T}{{1 - R}}} \right)\)23,24 and plotted, disregarding the thickness, d, in a self-consistent manner without a loss of generality. Band-gap values were then obtained by determining the x-intercept of the corresponding direct Tauc plots25, plotted as (αhν)2 against hν, where E = hν is the incident photon energy.

Reflection, transmission and PL samples were rotated at 10° intervals in a counter-clockwise manner with respect to the excitation light to obtain an angle of θ between the y-axis and the axis of polarization.

DFT calculations

First-principles calculations based on density-functional theory were used to compute the electronic structure. The calculations were performed using the Quantum ESPRESSO code34.The core electrons were treated using a Troulier–Martins pseudopotential35. The Kohn–Sham orbitals were expanded in a plane-wave basis with a cutoff energy of 50 Ry. The exchange and correlation interaction were described using the PBE functional34. Dipole matrix elements were calculated with the epsilon code, which is part of the Quantum ESPRESSO package36.

Data availability

The data that support the findings of this study are available from the corresponding author upon request.