Abstract
Low-damping magnetic materials have been widely used in microwave and spintronic applications because of their low energy loss and high sensitivity. While the Gilbert damping constant can reach 10−4 to 10−5 in some insulating ferromagnets, metallic ferromagnets generally have larger damping due to magnon scattering by conduction electrons. Meanwhile, low-damping metallic ferromagnets are desired for charge-based spintronic devices. Here, we report the growth of Co25Fe75 epitaxial films with excellent crystalline quality evident by the clear Laue oscillations and exceptionally narrow rocking curve in the X-ray diffraction scans as well as from scanning transmission electron microscopy. Remarkably, the Co25Fe75 epitaxial films exhibit a damping constant <1.4 × 10−3, which is comparable to the values for some high-quality Y3Fe5O12 films. This record low damping for metallic ferromagnets offers new opportunities for charge-based applications such as spin-transfer-torque-induced switching and magnetic oscillations.
Similar content being viewed by others
Introduction
Insulating ferromagnets (FMs) with low Gilbert damping, such as Y3Fe5O12 (YIG), are advantageous for certain applications1,2,3,4,5,6,7,8,9,10,11; however, they are not suitable for spintronic devices based on charge currents, which require metallic FMs12,13,14,15,16,17. Ultralow-damping metallic FMs are desirable for spin-transfer-torque-induced magnetic switching and dynamics in magnetic multilayers18 and FM/heavy-metal structures19 because of the lower critical current needed. Previously, Gilbert damping constants (α) as low as 1.9 × 10−3 have been reported for epitaxial films of Fe and Fe alloys20,21,22. Recently, Schoen et al.23 studied magnetic damping in polycrystalline Co x Fe1−x films of various compositions and measured a minimum α = 2.1 × 10−3 for Co25Fe75 24,25,26. This ultralow damping is attributed to a minimum in the electronic density of states at the Fermi energy for x = 25%, since the intrinsic Gilbert damping has been shown to be proportional to the density of states at the Fermi energy in Co x Fe1−x .
These results from polycrystalline films motivated the studies reported here that were guided by the expectation that the lower defect density in high-quality epitaxial films of Co25Fe75 will lead to reduced damping22 as compared to their polycrystalline counterparts. In this study we grow Co25Fe75 epitaxial films using off-axis sputtering on two kinds of substrates with the goal of further reducing the magnetic damping of this promising metallic FM towards an unprecedented level. X-ray diffraction (XRD) and scanning transmission electron microscopy (STEM) verify that these films are single crystal with high crystalline quality. Variable frequency FM resonance (FMR) measurements confirm that these films exhibit significantly reduced Gilbert damping—<1.4 × 10−3—in contrast to polycrystalline films.
Results
Epitaxial growth of Co25Fe75
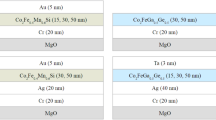
The growths were done using ultrahigh vacuum, off-axis sputtering with a base pressure lower than 2 × 10−10 Torr, which has previously been used to grow high-quality epitaxial films of various metals and oxides27,28,29,30,31,32. A Co25Fe75 sputter target 5 cm in diameter was prepared by annealing a pressed target of a stoichiometric Co and Fe powder mixture at 600 °C under H2 gas flow. The Co25Fe75 target was mounted on a horizontal sputtering source. The substrate was positioned at a horizontal distance of 5.4 cm from the target and 7.5 cm below the central axis of the target with the sample surface perpendicular to the target surface, which results in an average deposition angle of 54° relative to the target normal. We first grew 10 nm polycrystalline Co25Fe75 films on Si at room temperature with a 3 nm Cu seed layer and 5 nm Cr cap, which exhibited a low damping constant similar to that previously reported23. The Cr, Cu, and Co25Fe75 films were grown at Ar pressures of 10, 5, and 10 mTorr using DC sputtering with growth rates of 1.7, 3.6, and 2.4 nm/min, respectively. Co25Fe75 epitaxial films of 6.8 and 34 nm thicknesses were deposited at a substrate temperature of 300 °C directly on (001)-oriented MgO and MgAl2O4 (MAO) substrates (purchased from MTI), followed by a Cr capping layer grown at room temperature. MgO is a commonly used substrate with a lattice constant of a = 4.212 Å, which has a 3.9% lattice mismatch with Co25Fe75 when considering the 45° rotation between the body-centered-cubic (BCC) lattice of Co25Fe75 and the face-centered-cubic (FCC) lattice of MgO. MgAl2O4 (a = 8.083 Å) was used because it has a much better lattice match (within 0.4%) with Co25Fe75.
Characterization of crystalline quality
The crystalline quality of the epitaxial Co25Fe75 films was quantified by several methods. Figure 1a shows 2θ−ω scans obtained using triple-axis X-ray diffractometry of Cr(2.8 nm)/Co25Fe75(34 nm) and Cr(2.8 nm)/Co25Fe75(6.8 nm) films on MgO(001). The Co25Fe75(002) peaks at 65.139° and 64.844° for the 34 and 6.8 nm films correspond to out-of-plane BCC lattice constants of 2.862 and 2.873 Å, respectively. The XRD rocking curve (inset to Fig. 1a) of the Cr(2.8 nm)/Co25Fe75(34 nm)/MgO sample gives a full-width-at-half-maximum (FWHM) of 0.68°. Figure 1b shows a 2θ−ω scan of a Cr(2.8 nm)/Co25Fe75(34 nm)/MAO(001) sample, which exhibits clear Laue oscillations and a rocking curve FWHM of only 0.0057° (inset to Fig. 1b). The pronounced Laue oscillations and exceptionally narrow rocking curve are rarely seen in metallic epitaxial films, demonstrating very high crystalline quality enabled by the excellent lattice match between Co25Fe75 and MAO. Figure 1c, d show the X-ray reflectometry scans of a Cr(2.8 nm)/Co25Fe75(6.8 nm) bilayer on MgO and MAO, respectively, where the fitting gives a film roughness of 0.4 nm for both samples.
X-ray diffractometry and reflectometry of Co25Fe75 films on MgO and MgAl2O4 (MAO). a 2θ−ω X-ray diffraction (XRD) scans of Cr(2.8 nm)/Co25Fe75/MgO(001) films with the Co25Fe75 thickness of 6.8 and 34 nm. Inset: XRD rocking curve of the Co25Fe75(002) peak of the 34 nm film gives a full-width-half-maximum (FWHM) of 0.68°. b 2θ−ω scan of a Cr(2.8 nm)/Co25Fe75(34 nm)/MgAl2O4(001) sample. Inset: XRD rocking curve of the Co25Fe75(002) peak gives a FWHM of 0.0057°. X-ray reflectometry scans of c Cr(2.8 nm)/Co25Fe75(6.8 nm)/MgO(001) and d Cr(2.8 nm)/Co25Fe75(6.8 nm)/MAO(001) films, where a corresponding fit (black) gives a surface roughness of 0.4 nm for both samples
The Cr(2.8 nm)/Co25Fe75(6.8 nm) films on MgO and MAO were characterized by high-angle annular dark field scanning transmission electron microscopy (STEM) using an FEI probe-corrected Titan3 80–300 S/TEM. Figure 2 shows the STEM images viewed along the Co25Fe75 [010] direction (MgO and MAO [110] axis), which reveal the BCC ordering of Co25Fe75 and its epitaxy on the substrates. Electron energy loss spectroscopy was used to determine the Cr/Co25Fe75 boundary, which is unclear in the STEM images because of the small difference in their atomic numbers. The STEM images also show the epitaxial relationship between Co25Fe75 and the substrates as illustrated in Fig. 3a, where the BCC lattice of Co25Fe75 grows at a 45° in-plane rotation relative to the FCC lattice of MgO and the spinel lattice of MAO.
Atomic resolution images of Co25Fe75 films. Scanning transmission electron microscopy images of a Cr(2.8 nm)/Co25Fe75(6.8 nm)/MgO and b Cr(2.8 nm)/Co25Fe75(6.8 nm)/MAO films viewed along the Co25Fe75 [010] axis, where the boundary between Cr and Co25Fe75 as confirmed by electron energy loss spectroscopy scans is indicated by the dashed line. In a, the less clear Co25Fe75 atomic columns near the middle within 2 nm from the interface is due to strain relaxation via the disappearance of a Co25Fe75 column (MgO lattice constant is 3.9% larger than that of Co25Fe75). In b, the Co25Fe75 lattice matches perfectly with that of MAO without strain relaxation and its associated defects. Scale bars: 2 nm
Determination of in-plane magnetic hard axis. a Schematic illustration of the epitaxial relationship between the Co25Fe75 lattice and the MgO and MAO(001) substrates as well as the in-plane field angle ϕ H with respect to the Co25Fe75 [100] axis. In-plane magnetic hysteresis loops for the b Cr(2.8 nm)/Co25Fe75(6.8 nm)/MgO(001) and c Cr(2.8 nm)/Co25Fe75(6.8 nm)/MAO(001) sample for field applied along a Co25Fe75 [100] axis (ϕ H = 0°, easy axis) and a Co25Fe75 [110] axis (ϕ H = 45°, hard axis). A saturation magnetization of 2.46 and 2.34 T as well as an easy-axis coercive field of 20 and 2.5 Oe are obtained for the 6.8 nm Co25Fe75 film on MgO and MAO, respectively. The insets in b and c show a zoom-in view of the hard-axes hysteresis loops (ϕ H = 45°) to extract the in-plane magnetocrystalline anisotropy, H 4|| = 260 and 295 Oe, for the Co25Fe75 film on MgO and MAO, respectively
Characterization of in-plane magnetization
Magnetic hysteresis loops of Cr(2.8 nm)/Co25Fe75(6.8 nm) on MgO and MAO were measured at room temperature using a vibrating sample magnetometer at various in-plane orientations defined by ϕ H , the angle between the applied magnetic field (H) and the Co25Fe75 [100] axis (see Fig. 3a). Figure 3b shows two hysteresis loops for Cr(2.8 nm)/Co25Fe75(6.8 nm)/MgO measured at ϕ H = 0° and 45° where the small diamagnetic background from MgO has been subtracted. The ϕ H = 0° loop has a sharp magnetic reversal with a coercive field (H c) of 20 Oe while the ϕ H = 45° loop has a higher saturation field and H c = 23 Oe. By comparing the two hysteresis loops, we conclude that Co25Fe75 [100] (ϕ H = 0°) is the easy axis and Co25Fe75 [110] (ϕ H = 45°) is the in-plane hard axis within the framework of in-plane cubic anisotropy. The saturation magnetization, 4πM s = 2.46 ± 0.02 T, of the film on MgO is extracted from the hysteresis loop, which has been confirmed by a measurement using a SQUID magnetometer.
The inset to Fig. 3b shows a closer view of the hard-axis loop (ϕ H = 45°), which allows us to obtain the in-plane magnetocrystalline anisotropy. By applying separate linear fits to the saturated high field regime and the region between 200 and 0 Oe, we extract the in-plane magnetocrystalline anisotropy of 260 ± 10 Oe between the [100] and [110] axes33. This is in agreement with the in-plane cubic anisotropy H 4|| obtained from FMR measurements described below. Figure 3c shows the in-plane hysteresis loops for Cr(2.8 nm)/Co25Fe75(6.8 nm)/MAO, from which we obtain 4πM s = 2.34 ± 0.02 T and an in-plane magnetocrystalline anisotropy of 295 ± 10 Oe.
To probe the dynamic magnetic properties of the Co25Fe75 epitaxial films, we performed angular-dependent FMR measurements on our films. Figure 4a shows the derivative spectra of FMR absorption for the Cr(2.8 nm)/Co25Fe75(6.8 nm)/MgO sample for various ϕ H from −1° to 45° taken at a microwave frequency of f = 9.66 GHz in a cavity, from which the resonant field (H res) is obtained. From the in-plane angular dependence of H res, we can determine the magnetic anisotropy of the Co25Fe75 film. Magnetization subject to an energy landscape with cubic symmetry can be quantitatively described by the total free energy density (F) given by15, 34,
where θ is the out-of-plane angle and ϕ the in-plane angle for the orientation of the equilibrium magnetization (M) with respect to the easy axis, 4πM eff = 4πM s−H 2⊥ is the effective saturation magnetization, and H 2⊥ is the out-of-plane uniaxial anisotropy. H 4⊥, H 4∥, and H 2∥ are the out-of-plane cubic, in-plane cubic, and in-plane uniaxial anisotropy, respectively. By substituting Eq. (1) into the FMR resonance condition15, 34,
we can calculate H res for a given ϕ H , where γ is the gyromagnetic ratio and ω is the resonance angular frequency. For in-plane angular FMR, θ = 90° and the H 4⊥ term drops out; the in-plane equilibrium angle ϕ is determined by numerically minimizing the free energy density.
Angular dependence of ferromagnetic resonance. a Derivative ferromagnetic resonance (FMR) absorption spectra for a Cr(2.8 nm)/Co25Fe75(6.8 nm)/MgO(001) sample at in-plane angles ϕ H from −1° to 45°. b The resonance fields of the FMR spectra in a plotted against ϕ H. From the fit to the experimental data the values for H 4||, H 2||, and 4πM eff are extracted. c A similar H res vs. ϕ H plot for a Cr(2.8 nm)/Co25Fe75(6.8 nm)/MAO(001) sample
Figure 4b shows the in-plane angular dependence of H res for Cr(2.8 nm)/Co25Fe75(6.8 nm)/MgO and the fit using Eq. (2) and γ/2π = 29.5 ± 1.0 GHz T−1 35, which agrees well with the experimental data and gives H 2∥ = 0 ± 5 Oe, H 4∥ = 275 ± 5 Oe, and 4πM eff = 2.25 ± 0.02 T. The obtained H 4|| agrees with the value (260 Oe) determined from the magnetometry measurements in Fig 3b. Figure 4c shows the in-plane angular dependence of H res for Cr(2.8 nm)/Co25Fe75(6.8 nm)/MAO. From the fitting to the data, we obtain H 2∥ = −5 ± 5 Oe, H 4∥ = 325 ± 5 Oe, and 4πM eff = 1.96 ± 0.02 T, where the value of H 4|| is close to the anisotropy (295 Oe) obtained in Fig. 3c.
Measurement of Gilbert damping
Frequency-dependent FMR absorption was measured between 3 and 18 GHz using a microwave stripline by sweeping the magnetic field at various fixed frequencies. A small modulation of the magnetic field was applied to enable the measurement of differential absorbed power with a Schottky diode detector and a lock-in amplifier. Figure 5a, b show representative FMR spectra at f = 8 GHz with the magnetic field applied along the Co25Fe75 [110] axis for the Cr(2.8 nm)/Co25Fe75(6.8 nm)/MgO and Cr(2.8 nm)/Co25Fe75(6.8 nm)/MAO samples, which exhibit a peak-to-peak linewidth (∆H) of 12.5 and 5.4 Oe, respectively. The FMR linewidth of 5.4 Oe at 8 GHz rivals that of high-quality YIG films and is unprecedented for metallic FM films. We fit the f vs. H res plots in Fig. 5c, d using the same procedure for Fig. 4. The extracted values for in-plane anisotropies and effective saturation magnetization are: H 4∥ = 260 ± 5 Oe, H 2∥ = 0 ± 5 Oe, and 4πM eff = 2.19 ± 0.02 T for the film on MgO; H 4∥ = 315 ± 10 Oe, H 2∥ = −5 ± 5 Oe, and 4πM eff = 1.91 ± 0.02 T for the film on MAO, which agree with the results in Fig. 4.
Determination of Gilbert damping. Representative ferromagnetic resonance (FMR) absorption derivative spectra for the a Cr(2.8 nm)/Co25Fe75(6.8 nm)/MgO(001) and b Cr(2.8 nm)/Co25Fe75(6.8 nm)/MAO(001) sample at ϕ H = 45° measured at f = 8 GHz, which give a FMR linewidth of 12.5 and 5.4 Oe, respectively. Frequency vs. resonance field plots for the Co25Fe75 films on c MgO and d MAO measured at ϕ H = 45°, from which the anisotropy terms and effective saturation magnetization are extracted. e Frequency dependencies of the FMR linewidths for the Co25Fe75 films on MgO (red) and MAO (blue), where the behavior below 8 and 7 GHz, respectively, reflects incomplete saturation of the films leading to increases in the inhomogeneous broadening. The solid lines are linear fits to the frequency range above 8 (7) GHz for the film on MgO (MAO), from which the Gilbert damping and inhomogeneous broadening are extracted
Figure 5e shows the corresponding frequency dependencies of ∆H for the two samples. Below ~8 (7) GHz for the film on MgO (MAO), the data deviate from the linear dependence found above it. When comparing the hard-axis saturation fields of ~ 600 Oe to the H res of 581 Oe (592 Oe) at 8 (7) GHz for the film on MgO (MAO), we can understand these two regimes as follows. The films exhibit linear frequency dependence of ∆H above a threshold field where the magnetization is fully aligned. Below the threshold, the magnetization is not fully aligned, leading to non-uniform magnetization which inhomogeneously broadens the linewidth16. We first apply a linear fit in Fig. 5e in the frequency regime above 8 (7) GHz for the film on MgO (MAO), from which the damping constant can be determined using ΔH = ΔH inh + \(\frac{{4\pi \alpha f}}{{\sqrt 3 \gamma }}\), where ΔH inh is the inhomogeneous broadening36. For Co25Fe75(6.8 nm)/MgO, α = (7.1 ± 0.6) × 10−4 and ΔH inh = 9.2 ± 0.3 Oe; for Co25Fe75(6.8 nm)/MAO, α = (1.16 ± 0.02) × 10−3 and ΔH inh = 1.8 ± 0.1 Oe. If we choose a higher threshold field corresponding to 10 (9) GHz for the film on MgO (MAO), we obtain α = (8.5 ± 0.6) × 10−4 and ΔH inh = 8.4 ± 0.3 Oe for the film on MgO, and α = (1.21 ± 0.02) × 10−3 and ΔH inh = 1.5 ± 0.1 Oe for the film on MAO. The small ΔH inh in the Co25Fe75 film on MAO can be attributed to the high crystalline quality of the film. The damping constant in the film on MAO is slightly larger than that on MgO, for which the reason is unknown at this point. We note that FMR measurements at higher frequencies, e.g., 40 or 70 GHz, would further improve the accuracy for the extracted values of intrinsic damping in our films; however, we do not have this capability and will pursue such measurements through collaboration in the future. Based on our measurements, we are confident that the intrinsic damping constant in our epitaxial Co25Fe75 films is below 1.4 × 10−3, which is obtained when we assume ΔH inh = 0 for Co25Fe75/MAO.
Discussion
The measured Gilbert damping constant—<1 × 10−3—is extremely low for metallic FMs and, remarkably, is comparable to those reported for YIG films7. The lowest α (7.1 × 10−4) for our epitaxial Co25Fe75 films is considerably lower than the values reported for polycrystalline Co25Fe75 films23 (2.1 × 10−3) and epitaxial Fe films (1.9 × 10−3)22. Considering our measured damping constant also includes contributions from spin pumping into the Cr capping layer37 and radiative loss into the environment and stripline23, the intrinsic damping of our Co25Fe75 films should approach the calculated intrinsic value in the mid-low 10−4 regime23,24,25,26.
In summary, we observe extremely low magnetic damping in Co25Fe75 epitaxial thin films with excellent crystalline quality. This is the first direct measurement of a Gilbert damping constant in the 10−4 regime for metallic FM films, making it an ideal material for exploration of spintronic applications requiring metallic FMs.
Data availability
Data that support the findings of this report are available from the corresponding author upon request.
References
Sun, Y. Y. et al. Growth and ferromagnetic resonance properties of nanometer-thick yttrium iron garnet films. Appl. Phys. Lett. 101, 152405 (2012).
Sun, Y. Y. et al. Damping in yttrium iron garnet nanoscale films capped by platinum. Phys. Rev. Lett. 111, 106601 (2013).
Serga, A. A., Chumak, A. V. & Hillebrands, B. YIG magnonics. J. Phys. D 43, 264002 (2010).
Qu, D., Huang, S. Y., Hu, J., Wu, R. Q. & Chien, C. L. Intrinsic spin seebeck effect in Au/YIG. Phys. Rev. Lett. 110, 067206 (2013).
Lin, T., Tang, C. & Shi, J. Induced magneto-transport properties at palladium/yttrium iron garnet interface. Appl. Phys. Lett. 103, 132407 (2013).
d’Allivy Kelly, O. et al. Inverse spin Hall effect in nanometer-thick yttrium iron garnet/Pt system. Appl. Phys. Lett. 103, 082408 (2013).
Brangham, J. T. et al. Thickness dependence of spin Hall angle of Au grown on Y3Fe5O12 epitaxial films. Phys. Rev. B 94, 054418 (2016).
Wang, H. L. et al. Large spin pumping from epitaxial Y3Fe5O12 thin films to Pt and W layers. Phys. Rev. B. 88, 100406(R) (2013).
Heinrich, B. et al. Spin pumping at the magnetic insulator (YIG)/normal metal (Au) interfaces. Phys. Rev. Lett. 107, 066604 (2011).
Meier, D. et al. Longitudinal spin seebeck effect contribution in transverse spin Seebeck effect experiments in Pt/YIG and Pt/NFO. Nat. Commun. 6, 8211 (2015).
Prakash, A., Brangham, J., Yang, F. Y. & Heremans, J. P. Spin seebeck effect through antiferromagnetic NiO. Phys. Rev. B 94, 014427 (2016).
Costache, M. V., Sladkov, M., Watts, S. M., van der Wal, C. H. & van Wees, B. J. Electrical detection of spin pumping due to the precessing magnetization of a single ferromagnet. Phys. Rev. Lett. 97, 216603 (2006).
Saitoh, E., Ueda, M., Miyajima, H. & Tatara, G. Conversion of spin current into charge current at room temperature: inverse spin-Hall effect. Appl. Phys. Lett. 88, 182509 (2006).
Shikoh, E. et al. Spin-pump-induced spin transport in p-type Si at room temperature. Phys. Rev. Lett. 110, 127201 (2013).
Farle, M. Ferromagnetic resonance of ultrathin metallic layers. Rep. Prog. Phys. 61, 755–826 (1998).
Liu, X., Zhang, W., Carter, M. J. & Xiao, G. Ferromagnetic resonance and damping properties of CoFeB thin films as free layers in MgO-based magnetic tunnel junctions. J. Appl. Phys. 110, 033910 (2011).
Mosendz, O. et al. Detection and quantification of inverse spin hall effect from spin pumping in permalloy/normal metal bilayers. Phys. Rev. B 82, 214403 (2010).
Myers, E. B., Ralph, D. C., Katine, J. A., Louie, R. N. & Buhrman, R. A. Current-induced switching of domains in magnetic multilayer devices. Science 285, 867–870 (1999).
Liu, L. Q. et al. Spin-torque switching with the giant spin hall effect of tantalum. Science 336, 555–558 (2012).
Schreiber, F., Pflaum, J., Frait, Z., Muhge, T. & Pelzl, J. Gilbert damping and G-factor in Fe x Co1−x alloy-films. Solid State Commun. 93, 965 (1995).
Scheck, C., Cheng, L. & Bailey, W. E. Low damping in epitaxial sputtered iron films. Appl. Phys. Lett. 88, 252510 (2006).
Scheck, C., Cheng, L., Barsukov, I., Frait, Z. & Bailey, W. E. Low relaxation rate in epitaxial vanadium-doped ultrathin iron films. Phys. Rev. Lett. 98, 117601 (2007).
Schoen, M. A. W. et al. Ultra-low magnetic damping of a metallic ferromagnet. Nat. Phys. 12, 839 (2016).
Oogane, M. et al. Magnetic damping in ferromagnetic thin films. Jpn J. Appl. Phys. Part 1 45, 3889 (2006).
Mankovsky, S., Kodderitzsch, D., Woltersdorf, G. & Ebert, H. First-principles calculation of the Gilbert damping parameter via the linear response formalism with application to magnetic transition metals and alloys. Phys. Rev. B 87, 014430 (2013).
Lounis, S., Dias, M. D. & Schweflinghaus, B. Transverse dynamical magnetic susceptibilities from regular static density functional theory: evaluation of damping and g shifts of spin excitations. Phys. Rev. B 91, 104420 (2015).
Hauser, A. J. et al. Fully ordered Sr2CrReO6 epitaxial films: a high temperature ferrimagnetic semiconductor. Phys. Rev. B 85, 161201(R) (2012).
Du, C. H. et al. Control of magnetocrystalline anisotropy by epitaxial strain in double perovskite Sr2FeMoO6 films. Phys. Rev. Lett. 110, 147204 (2013).
Peters, B. et al. Epitaxial films of heusler compound Co2FeAl0.5Si0.5 with high crystalline quality grown by off-axis sputtering. Appl. Phys. Lett. 103, 162404 (2013).
Wang, H. L., Du, C. H., Hammel, P. C. & Yang, F. Y. Antiferromagnonic spin transport from Y3Fe5O12 into NiO. Phys. Rev. Lett. 113, 097202 (2014).
Du, C. H., Wang, H. L., Hammel, P. C. & Yang, F. Y. Y3Fe5O12 spin pumping for quantitative understanding of pure spin transport and spin hall effect in a broad range of materials (invited). J. Appl. Phys. 117, 172603 (2015).
Gallagher, J. C. et al. Robust zero-field skyrmion formation in FeGe epitaxial thin films. Phys. Rev. Lett. 118, 027201 (2017).
Kittel, C. Introduction to Solid State Physics 7th. edn (Wiley, 1996).
Liu, X. et al. Perpendicular magnetization reversal, magnetic anisotropy, multistep spin switching, and domain nucleation and expansion in Ga1-xMnxAs films. J. Appl. Phys. 98, 063904 (2005).
Taniguchi, T., Yakata, S., Imamura, H. & Ando, Y. Determination of penetration depth of transverse spin current in ferromagnetic metals by spin pumping. Appl. Phys. Express 1, 031302 (2008).
Kalarickal, S. S. et al. Ferromagnetic resonance linewidth in metallic thin films: comparison of measurement methods. J. Appl. Phys. 99, 093909 (2006).
Du, C. H., Wang, H. L., Yang, F. Y. & Hammel, P. C. Systematic variation of spin-orbit coupling with d-orbital filling: surprisingly large inverse spin hall effect in 3d transition metals. Phys. Rev. B 90, 140407(R) (2014).
Acknowledgements
This work was supported by National Science Foundation under Grant No. DMR-1507274 (sample growth and characterization, magnetization measurements, and data analysis) and by US Department of Energy, Office of Science, Basic Energy Sciences, under Award No. DE-FG02–03ER46054 (FMR measurements). Partial support for this work was provided by the Center for Emergent Materials, an NSF-funded MRSEC, under Grant No. DMR-1420451 (STEM characterization).
Author information
Authors and Affiliations
Contributions
P.C.H. and F.Y. designed this study; A.J.L., J.T.B., and Y.C. prepared the samples and performed vibrating sample magnetometer, XRD, and angular FMR measurements; B.D.E. and D.W.M. contributed the STEM images; S.P.W. and W.T.R. preformed the frequency-dependent FMR measurements. All authors wrote the manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Additional information
Publisher's note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Electronic supplementary material
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Lee, A.J., Brangham, J.T., Cheng, Y. et al. Metallic ferromagnetic films with magnetic damping under 1.4 × 10−3 . Nat Commun 8, 234 (2017). https://doi.org/10.1038/s41467-017-00332-x
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41467-017-00332-x
This article is cited by
-
Epitaxy enhancement in oxide/tungsten heterostructures by harnessing the interface adhesion
Applied Physics A (2024)
-
Regulation of Microstructure, Static, and Microwave Magnetic Performance of NiFe/FeMn/NiFe Heterogeneous Multilayer Films Based on Thickness of FeMn Films
Journal of Superconductivity and Novel Magnetism (2021)
-
Magnetic properties study of spin pinned NiFe/FeMn/NiFe heterogeneous multilayer films with different NiFe thicknesses
Applied Physics A (2020)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.