Introduction

Heat dissipation systems play an important role in preventing the thermal runaway of electronic devices with ever-increasing exhaust heat, ensuring normal operation and safety, and determining the design freedom of electronic devices. In particular, the next generation of thin and flexible electronics must provide smart applications, longevity, multifunctionality, flexibility, and wearability1, so it is essential to achieve these functions along with heat dissipation. Conventional cooling systems use convection to transfer the heat from a heat source to a convecting fluid. While this provides high cooling performance, it requires heat sinks with many metal/ceramic fins, as well as thermal interface materials2 to improve adhesion and thermal conduction with hard heat sources. Therefore, the components inevitably become bulky, making it difficult to fabricate thin and flexible electronic devices. Thermal design, especially for small heat-generating components, largely relies on thermal diffusion to the substrate3. While a system that diffuses heat throughout the substrate and housing by thermal conduction is expected to make the entire electronic to be thinner and more flexible than when using heat sinks, it requires a large area and a composite material with high thermal conductivity to provide sufficient cooling4,5,6,7, which ultimately leads to larger components and less flexibility. A heat dissipation mechanism that maintains flexibility is needed for the development of next-generation high-performance multifunctional devices.

Paper electronics have recently been developed as flexible and functional devices based on cellulose nanofiber (CNF) film substrates, such as flexible nonvolatile memory8,9, paper antennas10, transistors11, conductive paper12, and humidity sensors13. These applications originate from the high performance of CNF films, including toughness (mechanical strength of 100–500 MPa)14,15 and thermal stability (small coefficients of thermal expansion)16. In particular, for bottom-emission type (i.e., substrate transmission type) light-emitting devices, such as powder electroluminescent (EL) devices, CNF film is the most preferred substrate material because it has higher transparency and smoothness than plastic films or pulp papers, providing high luminescence17. As higher performance is achieved in these devices, the heat dissipation problem will become more significant. Therefore, it may be useful to use ascidian tunicate-derived CNF (T-CNF) films, which have recently been found to show in-plane thermal conductivities as high as 2.5 W/mK, which is 3–10 times higher than those of polymer films (usually <1 W/mK) often used as flexible substrates for electronics18,19. This is because even a small improvement in the thermal conductivity of the substrate material (in particular, polymeric materials with small thermal conductivity) has been estimated to significantly reduce the maximum temperature of a heat-generating light-emitting diode chip20. However, thermal diffusion cooling will not be able to keep up with the increase in heat generation.

To advance the further cooling of flexible two-dimensional (2D) films, it is essential to cut a slit in the film and allow fluid to flow through to induce convection cooling. The regular fin structure found in conventional heat sinks can be formed in the film. The closest structure to that structure is the periodic void structure created by kirigami. Kirigami is the traditional art of cutting paper into a wide range of three-dimensional (3D) structures with dimensional transformation over time (i.e., four-dimensional (4D) structures) by making a number of periodic incisions, and it is used in various engineering applications21. The simple incised design called “Amikazari” (net decoration) is highly versatile, and it is used not only in everyday decorations such as Tanabata (the Star Festival) paper ornaments but also in solar-cell substrates with optical-axis tracking22, diffraction gratings with variable spacing23, programmable multistable configurations of hydrogels24, graphene kirigami25, stretchable lithium-ion batteries26, stretchable bioprobes27, wearable sensors28, and strain sensors29. Unlike most of the above applications that focus on stretching dynamism and metamaterial designability for future 4D electronics, here, for the first time, we focus on the periodic void structure of kirigami that enhances interaction with air and forms an effective convection path. The kirigami structure allowing convection through the aperture is expected to be a novel smart heat dissipation platform. Elucidating the heat dissipation using kirigami-processed CNF film is thought to maximize the heat dissipation effect for general-purpose flexible film.

In this study, we verified the synergistic effect of convection and thermal conduction by kirigami. We clearly demonstrated the excellent cooling performance of a kirigami-processed CNF film through a heat dissipation test with a pseudo heat source using photothermal conversion by irradiating pseudo sunlight on a graphite-sprayed blackened area. The contributions of convection and thermal conduction to the heat dissipation of the kirigami specimen were characterized. We also demonstrated the heat-dissipation effect of powder EL devices, whose high heat generation has been a problem in their use, by forming them onto a CNF film and processing them into a kirigami EL device.

Results

Heat-dissipation test for kirigami

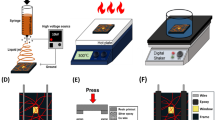
Amikazari-patterned kirigami specimens were prepared by laser processing of T-CNF films (Fig. 1a). Preliminary experiments showed that the T-CNF film with abundant carboxyl groups introduced on the CNF surface showed extended burning along the processed lines, suggesting that cellulose degradation was in progress even outside the pattern line. In addition, the extended burning made it difficult to process patterns with fine incision intervals (in particular, a = 1 mm) because the processed line was not precisely straight. Therefore, the introduction of carboxyl groups on the CNF surface by 2,2,6,6-tetramethylpiperidine-1-oxyl (TEMPO) oxidation was limited to a minimum amount to assist in fibrillation, resulting in the introduction of 0.204 mmol/g to prevent composition changes and improve the processability. As a result, kirigami specimens with incision intervals of 1–4 mm could be accurately cut without extended burning around the processed patterns, as shown in Fig. 1b.

Fig. 1: Kirigami specimen and heat-dissipation test.
figure 1

a Amikazari pattern definition with the incision interval a (mm) used in this study. b Appearance of the kirigami specimens composed of T-CNF film with various a values. c Setup of the heat dissipation test for the kirigami specimen retaining a certain stretch ratio and tilting angle. A pseudo heat source was created by photothermal conversion at the blackened area, and the temperature distribution was observed by a thermography camera while forced convection was generated by a circulator. The cooling effect was verified from the maximum temperature.

Full size image

A 1 cm × 1 cm blackened area was formed by spraying graphite in the center of only one side of the specimen as a pseudo heat source to test the heat dissipation performance of the kirigami specimen (Fig. 1a). The specimen was held with the blackened surface facing the ground and irradiated with intense white light (0.56 W/cm2) to generate heat by photothermal conversion30. The light irradiation intensity in this study was set based on the result that the powder EL device using a CNF film substrate showed a maximum current of 1.87 mA at 300 V17, which was calculated to be 0.56 W/cm2 of heat generated by assuming that all of the energy was converted to heat. The graphite-sprayed blackened area showed an absorption rate of more than 90% over a wide wavelength range and absorbed the incident light well (Fig. S1), while the unblackened CNF film did not effectively absorb light to generate heat30 (see Fig. S2). Because the film surface of the stretched kirigami specimen was inclined, a custom-made jig was prepared to hold the film surface horizontally (Fig. 1c). This jig allowed the film to be tilted and stretched around the center of the film where the blackened area formed. The temperature distribution was observed from the top surface of the film using a thermography camera, and the maximum temperature was determined. The heat dissipation test was conducted using a circulator to generate forced convection from the horizontal direction.

Convective heat dissipation by kirigami

The stretch ratio of the kirigami specimen was calculated by Dstretch/D0 (Fig. 2a). After determining the amount of stretching, the film surface was tilted so that it was horizontal, and forced convection was applied to the film surface. Preliminary experiments revealed that the inlet air velocity before passing through the kirigami specimen and the outlet air velocity after passing through the specimen were different even under the same test conditions. Therefore, we installed anemometer sensors at the inlet and outlet positions shown in Fig. 2b.

Fig. 2: Convective heat dissipation performance of the kirigami specimen.
figure 2

a Definition of the stretch ratio for the kirigami specimen (Dstretch/D0). b Setup for measurement of the inlet and outlet air velocities. Thermal images showing the temperature distributions of the pseudo heat source of the kirigami unprocessed T-CNF film during c natural convection and d forced convection. Thermal images showing the temperature distributions of the pseudo heat source of T-CNF kirigami specimens during e natural convection and f forced convection. g Relationship between the stretch ratio and the outlet air velocity. The insert shows the typical outlet air velocity profile for each stretch ratio. h Relationship between the vertical aperture distance b and the outlet air velocity. The insert shows the relationship between b and the thickness of the velocity boundary layer at kirigami surface δ. i Relationship between the stretch ratio and the maximum temperature. j Relationship between the outlet air velocity and the maximum temperature. The red dotted line is the best fit by the exponential decay function. The insert shows the relationship between the outlet air velocity and the calculated heat-transfer coefficient. The red line is the best fit by an allometric function with an exponent of 0.5.

Full size image

The maximum temperature of the T-CNF film before kirigami processing was 152 ± 2.3 °C during natural convection and 74.1 ± 1.5 °C during forced convection for an inlet air velocity of 3.0 m/s (Fig. 2c, d). The corresponding thermal resistance (Rth) values during natural and forced convection were calculated to be 230 and 91.3 K/W, respectively, using the equation Rth = ΔT/P, where ΔT and P are the temperature increment and heat input (0.56 W)31. The in-plane thermal conductivity of the T-CNF film was measured to be 2.2 ± 0.2 W/mK, which is reasonable compared with previously reported values18,32. The heat from the blackened area propagated to the surrounding area for several mm (Fig. 2c, d). When the a = 2 mm kirigami specimen was stretched 1.43 times, the maximum temperature was 124 ± 5.3 °C (Rth = 180 K/W) during natural convection, but the specimen promptly cooled to 50.3 ± 0.4 °C (Rth = 48.8 K/W) by convection at 3.0 m/s (Fig. 2e, f). The kirigami specimen reduced the thermal resistance to approximately one-fifth of that without kirigami and convection. The heat was efficiently transferred to the atmosphere in a steady manner, demonstrating the high convection-cooling performance of kirigami.

Interestingly, even though the inlet air velocity was always set to 3.0 m/s, the outlet air velocity was found to significantly vary depending on the stretch ratio of the kirigami specimen (Fig. 2g). The outlet air velocity at a stretch ratio of 1.43 asymptotically approached 3.0 m/s, which was almost the same as the inlet air velocity. The kirigami aperture seemed to show flow resistance, limiting the air velocity through the kirigami specimen. Therefore, the vertical aperture distance b, which is defined in Fig. 2b, was mathematically obtained for each stretch ratio. From the side view of the kirigami aperture shown in the upper left insert of Fig. 2h, the simple relationship

$$b = 2a\tan \theta$$
(1)

was established, where θ can be calculated from the basic relationship of \(D_{{{{\mathrm{stretch}}}}}/D_0 = \cos ^{ - 1}\theta\) for the Amikazari pattern. As b increased from 0 to ~2 mm, the outlet air velocity linearly increased, and it gradually approached the inlet air velocity of 3.0 m/s when b was greater than ~2 mm (Fig. 2h). We believe that this flow resistance is affected by the thickness of the velocity boundary layer at the kirigami surface δ. We thus estimated δ using the equation \(\delta \cong 5x/\sqrt {Re}\), where x is the distance from the tip of the flat plate (corresponding to 2a in the case of our kirigami specimen)33. Re is the Reynolds number: \(Re = \frac{{v_a \cdot L}}{\nu }\), with \(\nu = \frac{\mu }{\rho }\), \(\mu = \frac{{1.4592 \cdot T_{{{\mathrm{f}}}}^{\frac{3}{2}}}}{{109.10 + T_{{{\mathrm{f}}}}}} \times 10^{ - 6}\), and \(\rho = \frac{{351.99}}{{T_{{{\mathrm{f}}}}}} + \frac{{344.84}}{{T_{{{\mathrm{f}}}}^2}}\), where va is the air velocity, ν is the kinematic viscosity of air, μ is the viscosity of air, ρ is the density of air and Tf is the film temperature, which is the average temperature of the heat source and the air31,34. Because the velocity boundary layer occurs on both sides of the kirigami specimen, the relationship between the approximate δ and b/2 is plotted in the lower right insert of Fig. 2h. δ was then found to be larger than b/2 when b was 0 to ~2 mm. This result fully corresponds to the fact that the outlet air velocity is constrained by b when b is less than ~2 mm (Fig. 2h). The aperture distance owing to stretching the kirigami specimen limits the outlet air velocity if it is within two times the velocity boundary layer thickness.

For both natural and forced convection, the maximum temperature decreased as the stretch ratio increased (Fig. 2i). In particular, the maximum temperature of the unstretched kirigami specimen was comparable with that of the T-CNF specimen without kirigami processing (Fig. 2c and d), indicating that the 3D structural change caused by kirigami significantly contributed to convective heat dissipation. We demonstrated that the maximum temperature exponentially decayed with the outlet air velocity (Fig. 2j). This heat dissipation performance owing to convection can be expressed by the heat transfer coefficient, which represents the transferability of heat from the heat source to the convection air. The local heat transfer coefficient h (W/m2K) was calculated by

$$h = \frac{{\lambda \cdot Nu}}{L}$$
(2)

where λ, Nu, and L are the thermal conductivity of air, Nusselt number, and representative length, respectively31. L of the kirigami specimen was defined as the specimen length through which the convecting air passes, that is, the incision interval a. According to a previous study34, λ for air was calculated by \(\lambda = \frac{{2.3340 \times 10^{ - 3} \cdot T_{{{\mathrm{f}}}}^{\frac{3}{2}}}}{{164.54 + T_{{{\mathrm{f}}}}}}\). We used the maximum temperature of each specimen measured by thermography for the heat-source temperature. When forced convection was applied, laminar flow was assumed based on the small Re values of 143 to 360 for stretch ratios of 1 to 1.43 (well within the range of <5 × 105 for laminar flow)33. Nu was calculated by \(Nu = 0.664 \cdot Re^{\frac{1}{2}} \cdot Pr^{\frac{1}{3}}\). Pr is the Prandtl number: \(Pr = \frac{{\mu \cdot C}}{\lambda }\), where the specific heat of air \(C = 1030.5 - 0.19975 \cdot T_{{{\mathrm{f}}}} + 3.9734 \times 10^{ - 4} \cdot T_{{{\mathrm{f}}}}^2\)31,34.

In the case of natural convection, when assuming a downward heating plate, Nu was calculated by the equation \(Nu = 0.27 \cdot \left( {Gr \cdot Pr} \right)^{\frac{1}{4}}\), where Gr is the Grashof number31. However, in the case of the kirigami specimens with small L values (1–4 mm), the values of Gr Pr were found to be very small (~40), and they greatly deviated from the range in which natural convection can be assumed (105 < Gr Pr < 1010)31,33. This indicates that the buoyancy is not sufficient to be considered natural convection. When L is small, the area around the heating plate is not thermally stable, and the buoyancy caused by the temperature difference is unlikely to be a clear convection-driving source. Therefore, even at low air velocities with small Re values of 2–13 without applying any forced convection, it can be regarded as equivalent to laminar flow in forced convection because of its extremely low buoyancy owing to the small a value of the kirigami specimen. In this study, laminar flow, as in forced convection, was assumed for the natural-convection air velocity in the kirigami specimen to calculate the heat-transfer coefficient.

The heat-transfer coefficient of the kirigami specimen under natural convection at 0.12 m/s (Fig. 2e) was calculated to be 29.6 W/m2K, while that under forced convection at 3.0 m/s was 151.0 W/m2K, an increase of about five times. From the above equation, the heat-transfer coefficient is proportional to the 0.5th power of the air velocity, so fitting with an allometric function with an exponent of 0.5 gave a good approximation for the relationship between the heat-transfer coefficient and the outlet air velocity (insert of Fig. 2j). We clearly demonstrated that the convective heat-dissipation performance of kirigami specimens with small L values can be expressed using the outlet air velocity, which is the velocity of air passing through the kirigami specimen, and that thermal design can be achieved by the heat-transfer coefficient based on the forced-convection model, even in natural-convection mode.

Although the thermal resistance for convection heat transfer Rconv (\(= \left( {h \cdot A} \right)^{ - 1}\), where A is the heat-transfer area)31 is often used to describe the heat-dissipation performance of heat sinks and their surfaces35, the A value of the kirigami specimen (i.e., the blackened area) has a unique feature of almost constant A against the structural change by stretching, unlike heat sinks. We discussed the heat dissipation performance using only the heat-transfer coefficient, which more directly describes kirigami cooling. Similarly, the thermal resistance owing to thermal radiation was considered to be equal because the surface area and emissivity of the kirigami specimens made of T-CNF film were constant regardless of the stretch ratio.

When the kirigami specimens with a = 1, 2, 3, and 4 mm were stretched at a stretch ratio of 1.22, the heat dissipation performance was highest for a = 1 mm, and it was almost the same for a = 3 and 4 mm (Fig. S3a). For the same stretch ratio, a larger a makes b larger, which should be advantageous for heat dissipation. However, the outlet air velocity was almost the same at 2.6–2.8 m/s for all of the specimens with a = 1−4 mm (the insert in Fig. S3b). At a stretching ratio of 1.22, any a might have given b values that exceeded double δ. Therefore, the reason for the change in heat dissipation (different maximum temperatures) depending on a in Fig. S3a is thought to be that the longer a is, the less heat is transported to the film edge, and the amount of heat dissipated per unit area (i.e., the heat transfer coefficient) decreases. The calculated heat-transfer coefficient showed the same exponential decay trend (Fig. S3b) as in Fig. 2i. We speculate that the heat dissipation performance of kirigami film is determined by the balance between the outlet air velocity, which is defined by the a-value and the stretching ratio, and the thermal conductivity of the film.

In addition, there was no significant difference in heat dissipation when the film thickness was changed from 37 to 94 µm (Fig. S3c). Although the CNF film has a large anisotropy of thermal conductivity between the in-plane and thickness directions18, the heat would have been sufficiently transferred in the thickness direction during spreading in the plane direction because the heat propagates in the in-plane direction over a distance of a few mm, which is much larger than that of ~50 µm in the thickness direction. Considering that the heat dissipation is strongly influenced by the incision interval (Fig. S3a), we believe that the in-plane thermal conduction of the kirigami film mainly determined the heat dissipation in the steady state.

In the case of a conventional heat sink with a constant envelope volume, two factors largely compete to determine the heat dissipation performance: the fin spacing and the surface area. As the fin spacing increases, the air velocity between the fins increases and the heat dissipation performance improves, but as the surface area decreases, the heat dissipation performance reaches a head31. As a result, the optimal heat dissipation performance is determined by the balance between the air velocity between the fins and the surface area. Conversely, unlike heat sinks, the kirigami specimen can be assessed using a single factor, the outlet air velocity, which enables a simpler heat-dissipation design. In addition, our kirigami films can use various air directions to dissipate heat depending on their design. As shown in Fig. S4, if we design a propeller-shaped pattern by kirigami, we can use a perpendicular air direction for heat dissipation instead of a parallel air direction as in the Amikazari pattern.

Conductive heat dissipation by kirigami

Another factor that affects the heat dissipation of kirigami is the thermal conductivity of the film. We processed Amikazari patterns with a = 2 mm using film materials with thermal conductivities that greatly vary from ~1 to 400 W/mK (Fig. 3a) and conducted similar heat dissipation tests. An in-plane thermal conductivity of 0.9 W/mK has been reported for the polyimide film18, and literature values of 16, 90.5, 237, and 398 W/mK were used for stainless steel (SUS-304), Ni, Al, and Cu foils, respectively33. The differences among the thermal conductivities of the materials were particularly pronounced during natural convection, and the heat spread was significantly different, as shown in the thermal images in Fig. 3a. To accurately measure the temperature of the metallic materials, the entire surface of the thermographic side was blackened with graphite spray to reduce the infrared reflection and enhance the emissivity (see Fig. S2). We also analyzed the surface roughness of all the films using a scanning probe microscope (SPM), as shown in Fig. S5. The root-mean-square roughness (Rq) of the T-CNF film at the 20 µm square was slightly larger than that of PI and metal films due to fiber accumulation. However, the Rq of ~27 nm for the T-CNF film is almost equally small as that of ~26 nm for the Al film, and it can be concluded that the irregularities might not affect the thickness of the velocity boundary layer.

Fig. 3: Conductive heat dissipation performance of the kirigami specimens.
figure 3

a Various kirigami specimens with a = 2 mm composed of 50-µm-thick film materials with different thermal conductivities. The bottom row of figures shows the thermal images of the kirigami specimens at a stretch ratio of 1.43 corresponding to each material in natural convection. b Relationship between the stretch ratio and the outlet air velocity. The solid symbols represent the results with forced convection, and the open symbols represent those with natural convection. c Relationship between the stretch ratio and the maximum temperature. The symbol definitions are the same as those for (b). d Single logarithmic plot of the thermal conductivity of the kirigami material against the maximum temperature.

Full size image

The trend of the outlet air velocity was almost the same even when the film material was changed (Fig. 3b), and it reproduced a feature derived from the kirigami structure. This means that the heat-transfer coefficients determined by conditions such as the outlet air velocity and maximum temperature at each stretching ratio are equal regardless of the material. However, even though the air velocity was the same for the different materials, the maximum temperatures were significantly different, as shown in Fig. 3c. In both natural and forced convection, the maximum temperature was lower for higher thermal conductivity of the film material. At the same time, the temperatures of all of the materials decreased with increasing stretch ratio, which suggests that the effect of convection heat dissipation is similar to that in Fig. 2.

A clear trend was observed where the thermal conductivity of the film defined the maximum temperature for both natural and forced convection (Fig. 3d). T-CNF film, which has a relatively high thermal conductivity of 2.2 W/mK among polymeric films, also contributes to heat dissipation through thermal conduction. The result that films with higher thermal conductivity showed better cooling performance also occurs in conventional heat sinks. More importantly, polymeric films with low thermal conductivity, which could not previously be used as heat-dissipating components, also exhibited heat-dissipation performance at a practical level (~50 °C) for a generated heat of 0.56 W in the kirigami specimens. It is difficult to use electrically conductive and opaque film materials, such as metal foils, as electronics substrates without additional processing irrespective of their thermal conductivity, while insulating and translucent polymeric films cannot dissipate heat because of their low thermal conductivity. The kirigami heat dissipation framework overcomes this material dilemma, and we have demonstrated that even polymer films with low thermal conductivity can achieve heat dissipation that can withstand practical use.

Kirigami of EL devices

To highlight the functionality of kirigami in addition to its heat dissipation, we fabricated a traditional bottom-emission-type powder EL device on a T-CNF film (Fig. 4a). The powder EL device is a flexible flat light source that applies an alternating current (AC) voltage to zinc-sulfide-type phosphor particles printed on a film substrate36. This device enables the development of smart, energy-saving, and multifunctional light sources, such as large-area display textiles37, stretchable electronics38,39, and deformable displays40, owing to its high environmental resistance and lack of need for sealing. However, there are concerns that the inevitably generated heat will rapidly decrease the dielectric constant of barium titanate at above its Curie temperature41,42, causing a significant decrease in the luminance and luminous efficiency because the set electric field cannot be applied, thus shortening the lifetime by EL degradation43 and allowing glass transition for conventional polymeric packages. Therefore, the development of an effective cooling mechanism to enhance the luminous efficiency (i.e., promote energy savings), extend the lifetime, and improve the safety of use has remained an issue.

Fig. 4: Kirigami EL device.
figure 4

a Powder EL device formed on the T-CNF film and b its thermal image during emission. (c) Kirigami-processed EL device. Thermal images of the emitting kirigami EL device under d natural and e forced convection at a stretch ratio of ~1.3. f Demonstration of a wearable kirigami EL device. g Maximum emission intensity of each EL spectrum plotted against the tilting angle.

Full size image

Our EL device with an area of ~6 cm × 5 cm printed on the T-CNF film emitted light through the translucent T-CNF substrate at an AC effective voltage of 165 V at 1.2 kHz to show EL spectra comparable with those previously reported17 (Fig. S6). This powder EL device generated heat during the emission of light, reaching a maximum temperature of 73.5 °C (Fig. 4b). We then manually cut a double-column Amikazari pattern with a = 2.5 mm into the 3.5 cm × 5 cm area of this EL. After making the cut, the device continued to emit light while expanding and contracting because both of the electrodes still percolated (Fig. 4c). At a stretch ratio of ~1.3 times, the maximum temperature of the device was 68.6 °C under natural convection, but it rapidly cooled to 34.7 °C when exposed to convection at 3.0 m/s while emitting light (Fig. 4d, e). By lowering the temperature of the powder EL to the same level as body temperature, it is possible to make the device wearable (Fig. 4f). Convective heat dissipation by kirigami processing and the T-CNF film package realized a sufficiently cool powder EL device while maintaining flexibility for the first time.

Another functionality of kirigami is the controllability of the emission-angle range. Originally, the powder EL device showed almost no optical-axis directivity, but when it was stretched, the light-emitting surface was inclined, and thus, the emission angle could be dynamically modulated. By detecting the luminescence intensity at a tilt angle of ±60° to the stretching direction using a spectrometer, we showed an emission-angle range that clearly differed depending on the stretching magnification (Fig. 4g). The theoretically calculated tilting angles were 31.3° and 41.7° for stretch ratios of 1.17 and 1.34, respectively. This slight difference in the tilting angle was found to restrict the light emission angle. We demonstrated that kirigami provides good controllability of the emission-angle range with the stretch ratio for ELs without optical-axis directionality.

Discussion

We have demonstrated a kirigami heat-dissipation system that allows air convection through the periodic apertures of the Amikazari pattern. The kirigami aperture size determined by the stretch ratio defined the outlet air velocity and significantly enhanced the heat-transfer coefficient. Although the thermal conductivity of the film itself is as important as that of a conventional heat sink, we demonstrated that even a polymer-based film with low thermal conductivity can be made to function as a heat-dissipating component by using kirigami structures. Kirigami of the powder EL device cools it to approximately the same temperature as the human body, which is sufficiently cool to make it wearable, and allows the emission-angle range to be actively controlled by the stretch ratio. The variety of kirigami patterns, which are not limited to the Amikazari pattern, will make it possible to design dramatically more flexible heat-dissipation structures at will, as well as to improve the energy efficiency, extend the service life and improve the safety of electronics. Our heat dissipation concept based on kirigami processing of film materials will open up new pathways for the use of various conventional film materials as shape-variant cooling structures to freely design electronics.

Methods

Preparation of CNF film

The mantle of tunicate ascidian (Halocynthia roretzi) was cut into 1-cm squares with scissors and soaked overnight in 3 wt% NaOH solution for deproteinization. After washing, the product was bleached in 1 wt% NaClO2 solution at 80−90 °C under acidic conditions for 4 h until the product became white. The product was then washed with distilled water to obtain tunicate cellulose pulp. The tunicate cellulose pulp (1 g dry weight) was dispersed in 100 mL of distilled water with the addition of 0.016 g of TEMPO and 0.1 g of NaBr. Oxidation was initiated by adding 0.56 mL of 1.8 M NaClO aqueous solution, and the pH was kept higher than 10 by adding 0.5 M NaOH. After reacting for 1.5 h, the product was washed until the pH reached ~7 to obtain TEMPO-oxidized tunicate pulp. The pulp suspension at 0.2–0.5 wt% was then agitated by a high-speed blender for 20 min for fibrillation. After filtering the unfibrillated residual pulp using a metal mesh (50 mesh), tunicate cellulose nanofibers (T-CNFs) were obtained. The T-CNF suspension was filtered with a membrane filter (A010A090C, Advantec, Tokyo, Japan), and another membrane filter was used to cover the surface of the wet mat. The sandwiched mat was then hot-pressed at 110 °C and ~1 MPa for 10 min to dry the T-CNF film with a thickness of 46 µm. The in-plane thermal conductivity was calculated by the multiplication of the in-plane thermal diffusivity measured by a TA33 thermowave analyzer (Bethel Co., Ltd., Ibaraki, Japan), the heat capacity measured by a differential scanning calorimeter (Thermo Plus Evo2 8230, Rigaku, Tokyo, Japan) and the bulk density.

Kirigami specimen preparation

Amikazari patterns with incision intervals (a) of 1–4 mm were designed in a 5 cm × 2 cm rectangle. The T-CNF film was processed using a CO2 laser processing machine (HAJIME CL1 Plus, Oh-Laser Co., Ltd., Saitama, Japan) at a cutting speed of 20 mm/s and a laser intensity of 7.2 W. Polyimide film (Kapton 200H, Du Pont-Toray Co., Ltd., Tokyo, Japan) with a thickness of 50 µm was similarly processed. However, it was not cut under the same conditions of laser processing because it only burned, so the patterned film was manually cut after processing. Metal foils (stainless steel (SUS-304), Ni, Al, and Cu) with thicknesses of 50 µm were purchased from Nilaco Corp. (Tokyo, Japan). Kirigami processing of the metal foil was outsourced to Takumi Precise Metal Work Manufacturing Ltd. (Osaka, Japan), and it was performed by a laser micromachining system (Nissha HC600, Nippon Sharyo, Ltd., Nagoya, Japan). The SUS-304, Ni and Al foils were processed at laser-processing speeds of 3000, 3000, and 1500 mm/min with laser powers of 60, 60, and 530 W, respectively, using N2 assist gas. The Cu foil was processed at a speed of 3000 mm/min with a 400 W laser using O2 assist gas. The surface roughness (root-mean-square height, Rq) of each film was measured by a scanning probe microscope (SPM, AFM5000/AFM5300E, Hitachi High-Tech Corp., Tokyo, Japan) under cyclic contact mode using a cantilever (OMCL-AC160TS, Olympus Corp., Tokyo, Japan) with a spring constant of k = 26 N/m.

Heat-dissipation test

The heat dissipation test was conducted in a room environment with a temperature of 23 ± 1 °C and relative humidity of 30% ± 10%. The blackened area was formed on the kirigami-processed CNF specimen by masking a 1 cm × 1 cm area and coating it with graphite spray (FC-142, Fine Chemical Japan, Co., Ltd., Tokyo, Japan). Light absorption of the blackened area was measured by a UV-3600 Plus spectrophotometer (Shimadzu Corp., Kyoto, Japan) using an integrating sphere attachment (ISR-603, Shimadzu Corp.). A stretching and tilting jig was custom-made to maintain the inclined posture of the kirigami specimen while stretching it a certain ratio. White light with an irradiance of 0.56 W/cm2 was irradiated using a solar simulator (HAL-320W, Asahi Spectra Co., Ltd., Tokyo, Japan). The temperature distributions of the kirigami specimens were observed with a thermography camera (ETS320, FLIR Systems, Inc., Wilsonville, OR, USA). After waiting for a few minutes for the measurement environment to reach a steady state, 5–9 observations were made at intervals of several tens of seconds, and the median of the maximum temperatures was used. Forced convection was generated by a circulator (KJ-D994, Twinbird Corp. Niigata, Japan) placed at a distance of 60 cm from the center of the kirigami specimen. The air velocity was measured by a hot-wire anemometer (DT-8880, CEM, Shenzhen Everbest Machinery Industry Co., Ltd., Shenzhen, China). The air velocities for both natural and forced convection were measured by installing anemometer sensors in the horizontal direction. The average air velocity was calculated from the results of continuous measurements taken at 1 Hz intervals for ~2 min for each measurement condition.

Fabrication and characterization of the powder EL device

The powder EL device with a bottom-emission structure was fabricated by a previously reported procedure44. First, a high-dielectric polymer paste was prepared by mixing cyanoethyl polyvinyl alcohol (cyanoresin, CR-V, Shin-Etsu Chemical Co., Ltd., Tokyo, Japan) in cyclohexanone (037-05096, Fujifilm Wako Pure Chemical Co., Osaka, Japan) at a weight ratio of 3:7. This polymer paste was laminated on the T-CNF film by an automatic screen-printing machine (TU2020-C, Seritech Co., Ltd., Osaka, Japan) and dried at 80 °C for 6 min. Next, poly(2,3-dihydrothieno-1,4-dioxin)-poly(styrenesulfonate) (PEDOT:PSS, 768650, Sigma-Aldrich Co., LLC., Tokyo, Japan) was laminated and then dried at 80 °C for 10 min. Zinc sulfide phosphor particles (GG45, Osram Sylvania, Wilmington, MA, USA) were then dispersed in the high-dielectric polymer paste at a weight ratio of 4:6. This functional paste was laminated on the transparent electrode and dried at 80 °C for 6 min. BaTiO3 (620-07545, Kishida Chemical Co., Ltd., Osaka, Japan) was then dispersed in the high-dielectric polymer paste at a weight ratio of 4:6, laminated on the phosphor layer, and dried at 80 °C for 6 min. Finally, silver paste (MP-603S, Mino Group Co., Ltd., Gifu, Japan) was laminated on the dielectric layer and dried at 80 °C for 6 min. A TP-HBA3-INV AC inverter with 165 V at 1.2 kHz for the Flex-O-Lite Tracer Plate (TP-A3, Oriental Direct, Tokyo, Japan) was used to emit the EL. The optical fiber sensor of the spectrometer (SEC2020, ALS Co., Ltd., Tokyo, Japan) was held at a distance of 1 cm from the stretching axis of the EL device to measure the tilting angle dependence of the spectral intensity. The voltage dependence of the current and the luminance of the kirigami EL device were measured with an EL measurement system (SX-1152, Iwatsu Electric Co., Ltd., Tokyo, Japan).