Introduction

One of the most remarkable consequences of the application of an external magnetic field to a two-dimensional electron gas is the quantum Hall effect. In this phenomenon, the parabolic band dispersion of the electron gas rearranges into discretized Landau levels1. Moreover, when the Fermi energy is located between two Landau levels, the system is insulating in the bulk and possesses conducting channels on the edge. These channels are so robust to disorder that they can be used for metrology applications2.

The search for such effects in photonic systems has been a very active field in the past decade3. The implementation of Landau levels for photons is a solid-state inspired route to the study of artificial magnetic fields acting on photons. Thanks to the presence of photon nonlinearities in certain optical media, photonic lattices showing Landau levels would be particularly suited for the investigation of the interplay between pseudomagnetism and interactions4,5,6. In addition, the associated unidirectional edge channels are ideal to engineer photonic transport immune to backscattering in a chip3,7. The main difficulty in attaining this regime is that photons are largely insensitive to external magnetic fields.

Beyond the successful observation of chiral edge states for microwave, telecom and near-infrared wavelengths using magneto-optical materials8,9,10, the realization of topological bands for photons has so far relied on the engineering of synthetic magnetic fields. In this way, the Harper–Hofstadter model in coupled micro-resonators11,12 and Landau levels in centimetre-size cavities13 have been realized for photons without the need for any external magnetic field. A very straightforward approach to engineer synthetic magnetic fields was proposed by Guinea and co-workers when considering a layer of graphene14,15: by carefully engineering the strain applied to the honeycomb lattice, a gradient of hopping strength between adjacent sites is obtained, resulting in electrons experiencing a synthetic magnetic field. As time-reversal is not broken, the synthetic magnetic field has opposite signs at each of the two Dirac cones. Since this configuration requires only the local modification of the nearest-neighbour hopping energies across the honeycomb lattice16,17, such strain-induced pseudomagnetic fields can be directly transferred to graphene analogues based on classical waves of different nature18, from the photons/polaritons considered here to phonons. The resulting Landau-level structure is characterized by a zero-energy level (n = 0) and by a set of positive and negative quasi-flat levels (n = ±1, ±2, ±3, …) with a square root energy spacing (\({\it{\epsilon }}_n \sim \pm \sqrt n\)) inherited from the Dirac dispersion, similar to a graphene sheet exposed to a strong perpendicular magnetic field19.

In photonics, this configuration was first implemented by Rechtsman et al. in a lattice of coupled waveguides subject to artificial trigonal strain20. The presence of flat Landau levels was inferred from the localization dynamics of a point-like excitation at the edge of the lattice. However, the observation of the Landau-level spectra and the unique properties of the wavefunctions in the presence of a synthetic field, such as sublattice polarization18,21 and helical propagation22, have remained unexplored. More recently, the implementation of strain-induced Landau levels has been proposed23,24 and reported25 in macroscopic acoustic lattices.

In this article, we report direct evidence of photonic Landau levels and helical edge states emerging from a synthetic magnetic field engineered in strained honeycomb lattices for microcavity polaritons26. Unlike other photonic analogues of graphene, such as coupled waveguides20,27, microwave resonators28,29 and photorefractive crystals30, polaritons allow direct access to the energy band structure and to the spatial distribution of the wavefunction. Taking full advantage of these properties, this system has been a versatile platform to bring to the photonics realm the single-particle physics of quasi-crystals31 and graphene10,32,33,34 and to engineer new types of Dirac cones35 and other two-dimensional lattices36,37. Here, we follow the theoretical proposal of Salerno and co-workers38 and use an artificial uniaxial strain to generate strong synthetic fields for exciton-polaritons. We observe Landau levels at the Dirac cone energy in the s and px,y orbital bands arising, respectively, from the coupling between the fundamental and first excited modes of each micropillar. For both sets of bands, we observe the localization of the n = 0 Landau level wavefunction in one sublattice, illustrating the specificity of pseudomagnetic fields compared to real ones (see ref. 39 for an implementation in the microwave regime). We also report propagating helical edge states associated with the n = 0 Landau level, the synthetic field analogue of the chiral edge states responsible for transport in the quantum Hall effect. In light of the recent progress on polariton quantum effects40,41, our platform provides an alternative route to twisted cavities6,13 for the study of the fractional quantum Hall Laughlin states of strongly interacting photons anticipated in ref. 42.

Results

To fabricate the strained polariton honeycomb lattices, we start from a microcavity grown by molecular beam epitaxy, composed of a λ/2 Ga0.05Al0.95 As cavity embedded in two Bragg mirrors of 28 (top) and 40 (bottom) pairs of λ/4 alternating layers of Ga0.05Al0.95As/Ga0.80Al0.20 As. Twelve 7-nm-wide quantum wells are positioned at the three central maxima of the electromagnetic field. At 10 K, the temperature of our experiments, the strong coupling regime between quantum well excitons and confined photons gives rise to polaritons, light–matter hybrid quasi-particles, characterized by a Rabi splitting of 15 meV. Though the experiments reported here are restricted to the low-power linear regime, the hybrid light–matter nature of polaritons has the potential to reach nonlinear regimes at high excitation densities.

The planar microcavity is processed by electron beam lithography and inductively coupled plasma etching down to the GaAs substrate to form honeycomb lattices of overlapping micropillars. Each micropillar has a diameter of 2.75 μm. Due to the additional lateral confinement provided by the refractive index contrast between the semiconductor and air, the micropillars act as artificial photonic atoms. The fundamental mode of their energy spectrum (s mode) is cylindrically symmetric, similar to the pz orbitals in graphene. The first excited state is made of two antisymmetric modes, px and py, with lobes oriented in orthogonal spatial directions, as sketched in Fig. 1a. During the design of the lithographic mask, an overlap between micropillars is introduced to enable the hopping of polaritons between adjacent sites43. If the hopping from each pillar to its three nearest neighbours is equal and spatially independent (t1 = t2 = t3, unstrained honeycomb lattice), the coupling of s modes gives rise to two bands similar to the π and π* bands of graphene (Fig. 1b), whereas the coupling of p modes results in a set of four bands at higher energy (two of them are dispersive and intersect linearly, giving rise to Dirac cones).

Fig. 1: Strained honeycomb lattice.
figure 1

a Sketch of the real-space distribution of the s and p modes in a single micropillar. b Spectrally resolved far-field emission of an unstrained lattice showing the s and p bands for kx = 4π/3a. \(E_0^s = 1565.25\) meV is the energy of the Dirac points in the s bands. c Scanning electron microscope image of a strained honeycomb lattice with a gradient along the x direction: τ = 0.96 for the s bands and τ = 0.28 for the p bands. d Tight-binding calculation of the band structure of a uniaxially strained ribbon containing Nx = 41 unit cells and a gradient τ = 0.05 along x and zigzag terminations. Periodic boundary conditions are assumed along y. Landau levels appear around the Dirac points K and K′ for \(k_y = \pm 2\pi /3\sqrt 3 a\). The n = 0 Landau level is degenerate with non-propagating trivial edge states on both zigzag edges, while propagating edge states appear only on the right zigzag edge. The colours are defined by the mean position of the wavefunction of each state: magenta for states localized on the right edge, cyan for states localized on the left edge, and black for bulk states

Full size image

To implement artificial strain in our lattices, we design the lithographic mask in such a way that the centre-to-centre distance between the micropillars along the links parallel to x varies continuously between 1.9 and 2.7 μm, while the distance between the other links remains the same over the whole lattice (a = 2.4 μm; t2 = t3, see Fig. 1c). This uniaxial gradient of interpillar distances is designed to yield a linear evolution of the hopping t1(x) in both the s and p bands (see Supplementary material):

$$t_1\left( x \right) = {\it{t}}\left( {1 + \frac{{\it{x}}}{{3a}}\tau } \right)$$
(1)

where t = t2 = t3 and τ quantifies the amount of strain. The strain induces a synthetic vector potential A = (Ax,Ay) with eAx = 0 and \(eA_y\left( x \right) = 2\xi \hbar \tau {\it{x}}/\left( {9a^2} \right)\), where e is the electron charge and ξ = ±1 is the valley index associated with each of the two Dirac points38 K and K′. The resulting artificial magnetic field Bz = ∂xAy is directly proportional to the hopping gradient τ and has opposite signs in the two Dirac valleys K and K′. This is consistent with the fact that the strain-induced pseudomagnetic field does not break the time-reversal symmetry, which is the main difference from a magnetic field applied to graphene. We designed photonic honeycomb lattices with different hopping gradients resulting in artificial magnetic fields corresponding to values up to 3200 T for the s bands and 1000 T for the p bands when considering the electron charge and the lattice parameter of graphene. All the considered lattices share the same design: (i) armchair termination for the bottom and top edges, (ii) zigzag termination for the left and right edges, and (iii) t1 increases from the left to the right edge (i.e., positive gradient τ). Figure 1c shows a scanning electron microscope image of the central region of one of the ribbons employed in the experiments.

Figure 1d shows the energy dispersion of the s bands numerically calculated within a tight-binding model for a ribbon with Nx = 41 unit cells and a weak hopping gradient τ = 0.05 along x. Along y, periodic boundary conditions have been assumed. In the simulation, the black colour indicates modes with a wavefunction localized in the bulk of the lattice, while other colours are used to indicate wavefunctions located either on the right or left edge. Photonic Landau levels n appear in the vicinity of the Dirac cones K and K’, revealing a square root energy structure typical of massless Dirac particles19: \({\it{\epsilon }}_n^s = E_0^s \pm t\sqrt {\tau |n|}\) with \(E_0^s\) the energy of the Dirac points in the s bands. The n = 0 Landau level appears at \(E_0^s\), both at K and K′. At higher and lower energies, higher-order (|n| > 0) Landau levels appear in the simulation. These levels present a small tilt caused by the spatial variation of the Dirac velocity along the horizontal direction44,45. Indeed, high-order Landau levels whose associated wavefunctions are centred in different positions of the lattice have slightly different energies38.

The calculated bands in Fig. 1d also show the presence of edge states, depicted by the cyan and magenta colours. Between K and K′, the coloured line linking the two n = 0 Landau levels corresponds to trivial edge states associated with the zigzag terminations. These states are also present in honeycomb lattices with homogeneous hopping33. Away from the K and K′ points, the n = 0 Landau level evolves into two split bands with energy higher and lower than \(E_0^s\). These bands correspond to propagating states located at the right edge of the lattice, as indicated by the magenta colour. Bearded terminations (not shown here) result in edge states located on the left edge22. Higher-order (|n| > 0) Landau levels give rise to propagating states located at the right and left edges. Since the time-reversal symmetry is preserved, edge states in different valleys propagate in opposite directions, resulting in helical transport. The goal of this article is to report on the experimental implementation of these photonic Landau levels and their associated helical edge states.

The experimental investigation of the lattices is realized by combining reciprocal-space and real-space photoluminescence experiments using a non-resonant continuous wave laser at 740 nm. The laser is focused on an 8 μm diameter spot at the centre of the lattice by an aspherical lens (N.A. = 0.5). The low value of the pump power (0.2 mW) avoids any nonlinear effects. The real-space and momentum-space resolved photoluminescence is measured using an imaging spectrometer coupled to a CCD camera. A polariser in front of the spectrometer selects the emission linearly polarized along the y axis.

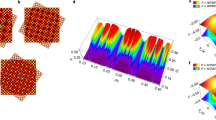
First, we focus on the photonic Landau levels in the s bands. Figure 2a displays the angle-resolved photoluminescence in the middle of the second Brillouin zone (kx = 4π/3a) in a lattice without a hopping gradient (τ = 0; 25 × 18 unit cells). This shows a band structure very similar to the π and π* bands of graphene, with Dirac cones at their crossing. The dispersion is fitted (white line) by a tight-binding model considering nearest-neighbour and next-nearest-neighbour hopping (t = 0.17 meV, \(t^\prime = - 0.08{\it{t}}\)). The latter is an effective hopping that allows us to reproduce the asymmetry between π and π* bands32,33. Physically, it originates from long-distance coupling mediated by the p bands46. Figure 2b shows the measured dispersion of a lattice with artificial strain (τ = 0.56, Bz = 1400 T) when summing the emission over all values of the transverse kx accessible with the collection lens for a given value of ky (parallel to the zigzag edge). The white lines indicate the theoretical dispersion of the discrete bands that originate from the splitting of the bulk bands in our finite lattice (10 × 18 unit cells, 360 micropillars) with a high hopping gradient.

Fig. 2: Photonic Landau levels in s bands.
figure 2

a Spectrally resolved far-field emission of the s bands from an unstrained lattice for kx = 4π/3a. b Spectrally resolved far-field emission summed over all values of kx accessible in our setup for a strained lattice with a gradient τ = 0.56. The corresponding pseudomagnetic field is Bz = 1400 T. The unshifted positions of the Dirac cones K and K’ are indicated by vertical dashed lines. The Dirac energies are located at \(E_0^s = 1565.25\) meV for a and \(E_0^s = 1567.7\) meV for b. For each case, the white solid lines are theoretical fits using the tight-binding Hamiltonian with: t = 0.17 meV and \(t\prime = - 0.08t\) for a; t = 0.17 meV, \({\it{t}}\prime = 0\) meV, a hopping gradient τ = 0.56, and an onsite energy of +1.6t. in the leftmost and rightmost pillar columns to account for the additional confinement of edge micropillars for b. c–e Measured real-space photoluminescence intensity at the energy of the Dirac cone for hopping gradients τ = 0 c, τ = 0.56. d, and τ = 1.26 e. The position of the excitation spot is marked by white dashed lines. The colour scale of each panel has been independently normalized to its maximum value. f Measured density of states for different hopping gradients. For the strained lattices with τ = 0.56, τ = 0.96 and τ = 1.26, the emission of the two leftmost and rightmost columns of micropillars has been excluded to suppress the contribution of the zigzag edge states. Each curve is vertically offset by 0.1 for clarity

Full size image

Figure 2b shows, by the white lines, the calculated dispersion using a tight-binding Hamiltonian that takes into account the nominal variation of t1(x) implemented in the lattice and an onsite energy offset Δ = 1.6t applied to the leftmost and rightmost columns of micropillars in the lattice. This onsite energy accounts for the additional confinement of the s modes in the micropillars located at the edges, which only have two neighbouring pillars instead of three for those in the bulk, and therefore, their eigenfunction is more strongly confined than that in the bulk pillars. To simplify the model, we neglected any next-nearest-neighbour coupling. Close to \(E_0^s\), at the Dirac points, the wavefunctions obtained from the model show spatial localization at the centre of the lattice compatible with the n = 0 Landau level. In contrast, the bands extending away from the Dirac points around \(E_0^s\) correspond to states localized at the zigzag edges. The full colour weighted version of the tight-binding fit, with information on the spatial position of the wavefunctions, is shown in the Supplementary material. Given the significant linewidth of the emission (~200 meV) comparable to the expected gap between the lowest-order Landau levels, the identification of the n = 0 level from momentum-space measurements is challenging.

To clearly identify the zeroth Landau level close to \(E_0^s\), we perform real-space tomography of the wavefunctions at that energy. To do so, the surface of the lattice is imaged on the entrance slit of the spectrometer, and the photoluminescence is resolved in energy and real-space position y for different positions x. The x, y intensity distribution of the wavefunctions for any energy can then be reconstructed from the measured tensor (x, y, E). Figure 2c shows the real-space emission at the energy of the Dirac points for the unstrained lattice (τ = 0). The wavefunction presents a honeycomb pattern that extends over several lattice sites around the excitation spot (indicated by the white dashed line in the images) due to polariton propagation at the Dirac velocity. Figure 2d, e show the emitted intensity at \(E_0^s\) for strained lattices with τ = 0.56 (Bz = 1400 T) and τ = 1.26 (Bz = 3200 T). In contrast to the unstrained case, in which the emission intensities from the A and B sublattices are equivalent, the presence of the pseudomagnetic field changes the intensity ratio between the two sublattices: for τ = 0.56, a clear asymmetry between the A and B sublattices is visible in the bulk of the strained lattice, and for τ = 1.26, the wavefunction is completely localized on the B sublattice. The measured and theoretical dispersions for τ = 1.26 are shown in the Supplementary material.

Sublattice polarization is the main signature of the n = 0 Landau level under a pseudomagnetic field18,38. In the case of a real magnetic field applied perpendicularly to a graphene sheet, the field has the same sign at both Dirac points K and K′. The wavefunction of the n = 0 Landau level at the K point presents a non-zero amplitude in only one of the two sublattices, while at the K′ point, the non-zero amplitude appears in the other sublattice. Overall, the n = 0 Landau level under a real magnetic field is a combination of both Dirac points and is distributed over both sublattices. In the case of a strain-induced pseudomagnetic field, the sign of the effective field at the K point is opposite to that at the K′ point. Therefore, the wavefunction of the n = 0 Landau level is localized in the same sublattice for both Dirac points, and the specific sublattice A or B is determined by the gradient of the strain. For higher-order Landau levels (n ≠ 0), the wavefunctions present a non-zero probability amplitude in both sublattices in both real and strain-induced fields. In the experiments, since an increase in the pseudomagnetic field leads to a larger gap between the n = 0 and higher-n Landau levels, the strongest sublattice polarization is observed for the lattice with the highest hopping gradients τ (Fig. 2d, e). This sublattice polarization provides unambiguous identification of the zeroth Landau level.

The appearance of the zeroth Landau level is also visible in the measured density of states shown in Fig. 2f for different hopping gradients. The density of states is obtained from the integration in space of the emitted light at a given energy. The n = 0 Landau level manifests as a peak of high density of states at \(E_0^s\), as a consequence of the associated degenerate flat band, when the gradient τ increases. To avoid any possible contribution of the trivial zigzag edge states also present at \(E_0^s\), in Fig. 2f, we have excluded from the integration the emission of the two leftmost and two rightmost columns of micropillars in lattices with τ = 0.56, τ = 0.96 and τ = 1.26.

One should also expect the presence of propagating edge states emerging from the pseudomagnetic field at energies between the different Landau levels (see Fig. 1d). However, given the emission linewidth, propagating edge states mix with bulk states and cannot be evidenced in the s bands. For this reason, we turn our attention to the p bands, which are also present in the fabricated lattices. As the nearest-neighbour coupling tL for the p orbitals oriented along the link between adjacent micropillars is four to five times larger than that for the s bands32, we expect a larger Landau-level spacing and the possibility of identifying helical edge states emerging from the artificial magnetic field.

Figure 3a displays the angle-resolved photoluminescence of the p bands when exciting an unstrained lattice (τ = 0, Bz = 0 T) at its centre. The dispersion is recorded as a function of ky for a value of kx = 4π/3a, passing through the centre of the second Brillouin zone. One can observe a flat band at low energy and two intermediate dispersive bands with Dirac crossings at the K and K′ points. By implementing the hopping gradient of Eq. (1) in the longitudinal hopping tL,1(x) for px orbitals parallel to the horizontal links, we expect to engineer an artificial magnetic field around the Dirac points similar to that of the s bands: photonic Landau levels should appear with an energy spectrum \({\it{\epsilon }}_n^p = E_0^p \pm (t_{\mathrm {L}}/2)\sqrt {\tau |n|}\) (see Supplementary material), where \(E_0^p\) is the energy of the Dirac points in the p bands. Figure 3b shows the photoluminescence for the strained lattice shown in Fig. 2b, d) obtained when summing the emission over all accessible values of the transverse kx for each value of ky. For the p bands, the designed centre-to-centre separation results in a hopping gradient of τ = 0.2 (Bz = 500 T). The flat bands around zero energy that are visible for \(k_y \in \left[ { - 2, - 4} \right]k_{y0} \cup \left[ {2,4} \right]k_{y0}\) delimited by the Dirac points at ky = ±2ky0 and ±4ky0 (with \(k_{y0} = 2\pi /3\sqrt 3\)) correspond to the trivial zigzag edge states34, similar to those shown for the s bands in Fig. 1d. At the position of the Dirac points, two energies \({\it{\epsilon }}_{0 - }^p = E_0^p - 0.07\) meV and \({\it{\epsilon }}_{0 + }^p = E_0^p + 0.07\) meV can be defined for the n = 0 Landau level (see the zoomed area in Fig. 3c). This apparent splitting of the Landau level originates from the finite size of the lattice; it is inherent to any experimental realization and should decrease for larger lattices. Interestingly, the n = −1 Landau level is also visible in Fig. 3c. The nearest-neighbour tight-binding simulations shown in Fig. 3b, c as solid lines qualitatively reproduce the observed dispersion for tL = −0.85 meV and assuming that the hopping tT between the p orbitals oriented perpendicular to the link is zero32. Due to the naturally stronger confinement of the p modes in the pillars, in this case, we do not assume any onsite energy offset at the edge micropillars.

Fig. 3: Photonic Landau levels in p bands.
figure 3

a Spectrally resolved far-field emission of the p bands from an unstrained lattice for kx = 4π/3a. b Spectrally resolved far-field emission summed over all values of kx accessible in our setup for a strained lattice with a gradient τ = 0.2. The corresponding pseudomagnetic field is Bz = 500 T. The Dirac energies are located at \(E_0^p = 1568.5\) meV for a and \(E_0^p = 1570.7\) meV for b. The positions of the Dirac cones K and K′ are indicated by vertical dashed lines. c Top panel: zoom on the Landau levels n = 0 and n = −1. The contrast is increased to improve the visibility. The white lines in b, c are theoretical fits using the tight-binding Hamiltonian with tL = −0.85 meV and tT = 0 meV. Bottom panel: coloured version of the theoretical fit used in the top panel. The colour of each state is defined by the mean position of its wavefunction. d Measured density of states for different hopping gradients. For the strained lattices with τ = 0.2, and τ = 0.4, the emission of the two leftmost and rightmost columns of micropillars has been excluded to suppress the contribution of the zigzag edge states. Each curve is vertically offset by 0.08 for clarity. e–g Measured real-space photoluminescence intensity for hopping gradients τ = 0 e, τ = 0.2 f, and τ = 0.4 h at the energy of the Dirac cone. These lattices are, respectively, those shown in Fig. 2c–e. The positions of the excitation spot are identical to those in Fig. 2. The n = 0 Landau level wavefunctions in f and g are characterized by a B sublattice polarization in the bulk. For clarity, circles show the positions of some of the pillars in the lattice. h Measured real-space photoluminescence intensity of the n = −1 Landau level shown in c. The colour scale of each panel has been independently normalized to its maximum value

Full size image

To confirm the identification of the n = 0 Landau level, we compare the wavefunction at the Dirac energy of an unstrained lattice (Bz = 0 T, Fig. 3e) with the wavefunctions recorded at \(E = {\it{\epsilon }}_{0 - }^p\) for τ = 0.2 (Bz = 500 T, Fig. 3f). For zero pseudomagnetic field, the wavefunction is delocalized over several lattice sites and presents a honeycomb pattern made of px,y orbitals. Analogous to that of the s bands, the intensity distribution in the bulk of the strained lattice presents a B sublattice polarization, a clear signature of the n = 0 Landau level emerging from a pseudomagnetic field. One can notice a stronger contribution of px over py orbitals on the left side of the n = 0 Landau level wavefunctions. This feature arises from the larger onsite energy of px orbitals inherited from the stronger confinement of the p orbitals along the direction of the hopping gradient when tL,1(x) < tL. If the hopping gradient is further increased, the sublattice polarization becomes more evident, as displayed in Fig. 3g for τ = 0.4.

The measured density of states shown in Fig. 3d is consistent with the emergence of the degenerate n = 0 Landau level when the strain is increased. The wavefunction of the n = −1 Landau level visible in Fig. 3c is shown in Fig. 3h. As expected, the intensity on sublattices A and B is similar in this case since the sublattice polarization is exclusive to the zeroth Landau level.

The larger hopping strength between the p orbitals than that between the s bands opens up the possibility of observing propagating edge states in the gap between the n = 0 and n = ±1 Landau levels, as shown in the tight-binding simulations in Fig. 1d for the s bands. Figure 3c highlights the presence of two such propagating edge states close to the K point in the gaps between p Landau level n = 0 and Landau levels n = ±1. The tight-binding simulations depicted in the lower panel of Fig. 3c and in the Supplementary material show the continuous evolution of the n = 0 Landau-level bands spatially located in the bulk of the lattice (black colour) into the propagating edge states spatially located on the edge (magenta, right edge), as expected from a two-dimensional system subject to an artificial magnetic field. Similar edge states with opposite group velocities are also visible at the other Dirac cone.

The propagation of these edge states is experimentally studied by placing the excitation spot on the zigzag edges of a strained lattice with a hopping gradient τ = 0.28 (Bz = 700 T). The measured dispersions, centred and zoomed on the zeroth Landau level, are shown in Fig. 4a, b for the excitation on the left and right edges, respectively. The dispersions exhibit very different features depending on the excited edge. Figure 4a displays the dispersion corresponding to the excitation of the left zigzag edge, and it is dominated by a flat band at \(E_0^p\) associated with non-propagating zigzag edge states34. These modes and the n = −1 Landau levels at lower energy are separated by a gap (a similar gap is also visible just above \(E_0^p\)). In contrast, Fig. 4b, which corresponds to the excitation of the right zigzag edge, is dominated by dispersive modes near the Dirac points ky = ±2ky0 with opposite group velocities, lying exactly in the gap between the zeroth and n = −1 Landau levels.

Fig. 4: Zeroth Landau level propagating edge states.
figure 4

a, b Spectrally resolved far-field emission centred and zoomed on the zeroth Landau level (p bands) of a strained lattice with a gradient τ = 0.28 (Bz = 700 T) for a left a and right b edge excitation. \(E_0^p = 1571.3\) meV. The positions of the Dirac cones K and K′ are indicated by vertical dashed lines. c, d Measured real-space photoluminescence intensity pattern for an excitation localized on the left edge c and on the right edge d. The emission at an energy lying in the gap between \({\it{\epsilon }}_{0 - }^p\) and \({\it{\epsilon }}_{ - 1}^p\) (horizontal red line in a and b) is selected. The position of the excitation spot is marked by white dashed lines. e Maximum intensity measured on each pillar above the excitation spot (yellow rectangles in c and d) for left and right edge excitation

Full size image

These dispersive modes correspond to the propagating edge states linked to the zeroth Landau level depicted in Fig. 1d for the s bands. Since they have opposite group velocities in each valley, they propagate in opposite directions. This helical propagation is revealed in Fig. 4d when measuring the emitted intensity for an energy lying between \({\it{\epsilon }}_{0 - }^p\) and \({\it{\epsilon }}_{ - 1}^p\) (horizontal red line in Fig. 4a, b). At the right edge, polaritons flow from the excitation spot towards the top and bottom corners of the lattice. When the left edge is excited, as shown in Fig. 4c, the measured intensity shows a fast exponential decay outside the excitation spot, evidencing the absence of propagating edge states. This left/right propagation asymmetry is well reproduced by driven dissipative calculations (see Supplementary material). The intensity profiles measured along the left and right zigzag edges (yellow rectangles in Fig. 4c, d) are reported in Fig. 4e. The extracted propagation lengths are Ll = 5 μm and Lr = 17 μm for the left and right edges, respectively. Based on the propagation length Lr of the right edge state and the group velocity vg = 2.4 μm s−1 measured in Fig. 4b, we find a lifetime of τ ≈ 7 ps for polaritons in the propagating state, in agreement with previous experiments on polariton honeycomb lattices etched from the same wafer35.

To understand why only the right zigzag edge supports the propagation of the zeroth Landau level, one has to consider the chiral symmetry of our system and the peculiar form of the zeroth Landau level wavefunction emerging from the pseudomagnetic field22. As the zeroth Landau level wavefunction is entirely localized on the B sublattice, its energy is pinned to \(E_0^p\) (the onsite energy of the isolated p orbitals) by the chiral symmetry. Consequently, the only way for the zeroth Landau level to evolve into a dispersive state with \({\it{E}}\, \ne \,{\it{E}}_0^p\) is to combine with other zero-energy edge states localized on the A sublattice. In our case, the role of these zero-energy states is played by trivial edge states associated with the zigzag termination of the honeycomb lattice. These trivial edge states are localized on the A sublattice at the right edge and on the B sublattice at the left edge. For this reason, propagating edge states associated with the n = 0 Landau level appear only at the right edge for the sign of the hopping gradient that we consider. Note that by choosing other terminations, it is possible to engineer propagating edge states only at the left edge, propagating edge states at both edges simultaneously, or no propagating edge states at all22,47. This behaviour is a consequence of the sublattice symmetry breaking of the n = 0 Landau level emerging from the presence of the pseudomagnetic field and is very different from the unidirectional chiral edge states that appear in graphene under an external magnetic field, with a propagation direction independent of the type of edge and robust to local disorder.

Discussion

In this work, we have reported a direct measurement of the n = 0 Landau level wavefunction distributions in a photonic honeycomb lattice subject to a synthetic magnetic field. We have provided unprecedented evidence of the dispersion of helical edge states induced by an artificial magnetic field and their emergence from the fundamental Landau level at the edges of the sample. In combination with recent advances in the enhancement of polariton–polariton interactions40,41,48, our realization is promising for the prospect of studying strongly correlated photonic phases in systems with a higher degeneracy than those in recent reports for microwave49 and twisted cavities6. The helical edge states we have unveiled provide a new playground to design topological photonic channels in a chip without any external magnetic field. They present a high degree of versatility, as their existence is controlled by the termination of the lattice and, similar to the interface states emerging from the valley Hall effect50, they should in principle be free of backscattering in the presence of any disorder that does not mix the two non-equivalent Dirac cones.

Methods

The different hopping gradients τ are designed during the realization of the lithographic mask by accurately tuning the distance d between the centres of the pillars forming a horizontal link. The distance between the centres of the pillars forming angled links with respect to the horizontal direction remains constant (d = 2.4 μm). To determine the dependence of the hopping on the centre-to-centre distance d, we solve the time-independent Schrödinger equation in two dimensions, accounting for the in-plane shape of the micropillars for different centre-to-centre distances51 and assuming infinite potential out of the micropillars. We take D = 2.75 μm as the diameter of the two micropillars, the same as that engineered in the considered strained lattices. From the spectrum of eigenmodes, we extract the bonding–antibonding splitting for both the s and p modes as a function of the centre-to-centre distance. We assume that the splitting for each set of modes corresponds to twice the hopping amplitudes ts and tp in the tight-binding model. We perform a linear fit of ts as a function of d and a parabolic fit for tp, resulting in the following dependences:

$${\it{d}} = - 1.3 \times t_s + 2.73,\,{\mathrm {{for}}}\,{\it{s}}\,{{\mathrm {{bands}}}},$$
$${\it{d}} = - 0.75 \times t_p^2 + 0.34 \times t_p + 2.74,\,{\mathrm{for}}\,{p}\,{\mathrm{bands}}.$$

We use the above equations to design the centre-to-centre distances along the horizontal direction in the considered lattices. Each lattice is designed to have a strictly linear gradient τ either in the s or in the p bands. However, even if a lattice is designed to have a linear hopping gradient in one of the two types of bands, the hopping gradient in the other type of band is also linear to a very good approximation. Supplementary Fig. S1 shows the exact centre-to-centre distances along the horizontal direction employed in the samples used in our study (black dots) along with the s and p bands hopping in the horizontal links expected from the above equations. Lattices (a), (c) and (d) were designed with a linear gradient in the p bands, while (b) was designed with a linear gradient in the s bands. Nevertheless, the expected hopping gradient for the other type of band is also linear to a very good approximation (the figure shows the R2 errors to linear fits). Therefore, our strained lattices implement homogeneous synthetic magnetic fields for both the s and p bands at the Dirac points.