Main

The Solar System is known to be ‘practically stable’, in the sense that none of the known planets is likely to suffer mutual collisions, or be ejected from the Solar System, over the next several billion years3,8,9,10. A more subtle measure of stability is the distinction between regular and chaotic systems. In a regular system, the effect on solutions of small perturbations grows slowly with time, allowing accurate long-term predictability. In contrast, chaotic systems exhibit sensitive dependence on initial conditions, whereby the distance between nearby solutions increases exponentially with time. If the separation at time zero is d(0), then d(t) tends to be approximated by d(0)eλt, where λ is called the Lyapunov exponent. It is often more intuitive to talk of λ−1, called the Lyapunov time. The motion of Pluto is chaotic with a Lyapunov time of 10–20 million years1,11,12, whereas the inner Solar System has a Lyapunov time of about 4–5 million years1,13,14. Pluto’s chaos is caused by the overlap of two-body resonances15. The cause of chaos in the inner Solar System is not yet fully understood16, although secular resonances probably play a role3,14.

The existence of chaos among the jovian planets (Jupiter, Saturn, Uranus and Neptune) is less certain. On one hand, Laskar3,13,14 found that the orbits of the outer planets appeared non-chaotic. This is consistent with the knowledge that there are no two-body resonances among the outer planets2, but nonetheless Laskar’s ‘averaged’ integrations could not detect mean-motion resonances, even if they existed. On the other hand, chaos was found in a non-averaged, full integration of the nine planets by Sussman and Wisdom1. However, their computed Lyapunov time varied with simulation parameters for reasons that were (at the time) unknown.

Two obvious but mutually exclusive explanations can be offered to explain why some investigators find chaos whereas others do not. First, Sussman and Wisdom’s widely varying Lyapunov time might be the result of numerical artefacts, rather than physical effects. This hypothesis was supported at the time by the lack of an explanation for chaos in the outer Solar System. A second plausible explanation is that as Laskar’s averaged equations do not model planet motion, Sussman and Wisdom were observing real chaos, caused by something other than overlap of two-body resonances.

Unfortunately, both explanations have since been convincingly offered, and neither has been disproved so far. On one hand, chaos can be a numerical artefact6, even in an n-body integration7,17. Furthermore, numerous careful integrations of the outer Solar System have been carried out specifically to ensure the accuracy and convergence of the numerical results4,5, and these give a clear indication of no chaos. On the other hand, an explanation for the chaos in terms of three-body mean-motion resonances has been offered by Murray and Holman2. Furthermore, Guzzo18 has carried out carefully tested integrations detecting a web of three-body resonances, precisely where Murray and Holman’s theory predicts them to be. Finally, those who have found chaos also seem to carry out reasonable convergence tests, making it unlikely that the chaos they have found is a numerical artefact. So, we have a quandary: there is apparently unassailable evidence on two sides, demonstrating both that the outer Solar System is chaotic, and that it is not.

The observational uncertainty in the position of the outer planets is a few parts in ten million. In particular, the semi-major axes (which dictate the orbital mean-motion resonances) of Saturn, Uranus and Neptune are known to about 3, 5 and 7 parts in 10 million, respectively19. The resolution of the above paradox is simple: within the observational uncertainty, there exist both chaotic and apparently regular solutions. This is also consistent with Murray and Holman’s theory, as it is known from the study of simpler systems that chaotic ‘zones’ can contain both chaotic and regular-looking trajectories, densely packed among each other, with the chaotic ones having widely varying Lyapunov times20.

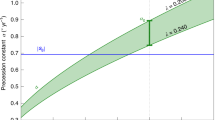
Although it is impossible with a finite-time integration to demonstrate that a trajectory is not chaotic, we abuse the term ‘regular’ to mean a trajectory that shows no evidence of chaos over timescales ranging from 200 Myr (million years) to 1 Gyr (109 years). Figure 1 shows the divergence between initial conditions that initially differ by an infinitesimal amount (1.5 mm in the semi-major axis of Uranus). Chaos manifests itself as exponential divergence between such ‘siblings’, whereas regularity is manifested by polynomial divergence. Our results are consistent across three very different integration schemes, and all schemes agree with each other as long as the time steps are smaller than the convergence threshold.

Figure 1: Divergence between nearby trajectories, integrated with four different numerical integrators.
figure 1

a, A chaotic trajectory with a Lyapunov time of about 12 million years. b, A trajectory showing no evidence of chaos over 200 Myr. Both trajectories are within observational uncertainty of the outer planetary positions.

Full size image

To estimate how the observational uncertainty volume is split between chaotic and regular initial conditions, we chose 31 systems within the observational uncertainty and integrated each for 200 Myr. We found that 21 of the 31 samples (about 70%) were chaotic, and 10 (about 30%) were regular. When integrated for 1 Gyr, the percentage of regular trajectories decreased to less than 10%. However, the spectrum of observed Lyapunov times was enormous (Fig. 2). Furthermore, the ‘canonical’ initial condition, the only one explicitly fitted to all observations in DE40519, shows no evidence of chaos after 1 Gyr.

Figure 2: Menagerie of Lyapunov times.
figure 2

All trajectories originate within observational uncertainty, although the regular one (labelled T1.5, corresponding to an infinite Lyapunov time) is the ‘canonical’ initial condition from the Jet Propulsion Laboratory’s DE405 ephemeris. Finite Lyapunov times range from 11 Myr to 230 Myr; one trajectory even changes Lyapunov time from 50 Myr to 230 Myr after about 500 Myr of evolution.

Full size image

The fine structure observed here does not seem to be explained by existing three-body resonance theory2,18, which makes statements about the existence or absence of chaos on scales of about one part in 106; the structure here is at least one order of magnitude smaller. It could be hypothesized that the structure is due to four-body resonances, but if the fine structure continues to smaller scales, then even four-body resonance theory may not be able to explain it—and there are no five-body resonances in a four-planet system. Thus, it is not clear that extensions to existing resonance theory will fully explain the structure observed in this system.

Methods

We use initial conditions for the Sun and all eight planets (excluding Pluto). The inner planets are deleted, but their effect on the outer planets is crudely accounted for by creating a ‘new’ Sun whose mass is equal to that of the inner Solar System (where ‘inner Solar System’ refers collectively to the real Sun+inner planets), whose position is placed at the barycentre of the inner Solar System, and whose momentum is set equal to that of the inner Solar System. This ensures2 that the positions of the chaotic zones are shifted by no more than about one part in 1011. The system of five bodies is then numerically integrated using only newtonian gravity for 109 years (1 Gyr), or until the distance between siblings saturates. The masses of all objects are held constant. We also ignore many physical effects that may be important to the detailed motion of the planets5,21. However, we believe that none of these effects will alter the chaotic nature of solutions.

Determining the existence of chaos depends critically on the quality of the numerical integration scheme. We ensure that our results are free of numerical artefacts by carrying out our integrations using three different algorithms. First, we use the Wisdom–Holman symplectic mapping12 as implemented in the Mercury 6.2 package22, with time steps ranging from 400 days down to 2 days. We find that using time steps of 16 days or more leads to inconsistent results, in that integrating the same initial condition with different time steps results in significantly different Lyapunov times7. However, for a particular initial condition, results converge to reliable Lyapunov times when the integration time step is 8 days or less. Our second integration scheme is the N-body integrator (NBI) package, which has been used to demonstrate the non-existence of chaos in the outer Solar System4,17,23. We use NBI’s 14th-order Cowell–Störmer integrator with modifications by the University of California, Los Angeles, research group led by William Newman5,23,24, with a time step of 4 days. With these parameters, NBI is known to produce exact solutions to double precision, at each time step; this cannot be improved on without maintaining machine precision while increasing the time step (which would lower the frequency at which the one-roundoff-per-step occurs). Our third and highest precision integration scheme is the Taylor 1.4 package25, which uses a 27th-order Taylor series expansion and a 220-day time step. Taylor integrators are extremely stable when applied to non-stiff problems, with the radius of convergence increasing linearly with integration order, and in our case the time step is well within the radius of convergence26. Arithmetic in Taylor 1.4 is carried out in 19-digit Intel Extended precision. Like NBI, on each step, Taylor 1.4 produces results that are exact to machine precision, except here the step is 220 days rather than 4 days, and the solution is exact to 19 digits rather than 16. In the case of both NBI and Taylor 1.4, the numerical error growth is effectively dominated by a single random roundoff error per step. We have verified the ‘exact to machine precision per step’ properties of both NBI and Taylor by comparison with quadruple-precision integrations that were locally accurate to 30 digits. Once convergence was reached, all integrations had errors that grew (in the absence of chaos) approximately as t3/2, in accordance with Brouwer’s law27. Our 1 Gyr integrations using Taylor 1.4 conserved both energy and angular momentum to almost 13 digits. Numerical error caused the centre of mass of the system to drift by just 0.45 km over 1 Gyr, which is about three orders of magnitude smaller than the current observational error. Thus, the numerical error in our 1 Gyr experiments is negligible compared with current observational error.

Figure 1 uses initial conditions for the Sun and eight planets as listed in the Mercury 6.2 package22, representing the positions of the planets at JD2451000.5. The semi-major axis of Uranus was increased by 2×10−6 AU (Fig. 1b) and 4×10−6 AU (Figure 1a). The percentage of initial conditions that are chaotic come from 31 eight-planet samples from the latest Jet Propulsion Laboratory planetary ephemeris, DE40519. We drew 21 sets of initial conditions starting at 9.5 Jan 1990 (JD2448235), separated by 30-day intervals. This approach samples initial conditions from a span of about 2 years, so it includes samples such that all of the inner planets are sampled at widely varying positions in their orbits (before they are thrown into the Sun). We also drew 10 samples starting at the year 1900, separated by 10-year intervals. Figure 2 uses initial conditions from all of the above sources, and is not intended to represent a uniform sample.

The source code, initial conditions and outputs are all available from the author on request.