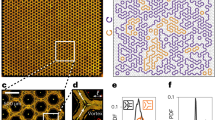
Abstract
We are ‘hairy’ on the inside: beds of passive fibres anchored to a surface and immersed in fluids are prevalent in many biological systems, including intestines, tongues, and blood vessels. These hairs are soft enough to deform in response to stresses from fluid flows. Yet fluid stresses are in turn affected by hair deformation, leading to a coupled elastoviscous problem that is poorly understood. Here we investigate a biomimetic model system of elastomer hair beds subject to shear-driven Stokes flows. We characterize this system with a theoretical model that accounts for the large-deformation flow response of hair beds. Hair bending results in a drag-reducing nonlinearity because the hair tip lowers towards the base, widening the gap through which fluid flows. When hairs are cantilevered at an angle subnormal to the surface, flow against the grain bends hairs away from the base, narrowing the gap. The flow response of angled hair beds is axially asymmetric and amounts to a rectification nonlinearity. We identify an elastoviscous parameter that controls nonlinear behaviour. Our study raises the hypothesis that biological hairy surfaces function to reduce fluid drag. Furthermore, angled hairs may be incorporated in the design of integrated microfluidic components, such as diodes and pumps.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout





Similar content being viewed by others
References
Audoly, B. & Pomeau, Y. Elasticity and Geometry: From Hair Curls to the Non-linear Response of Shells (Oxford Univ. Press, 2010).
Vogel, S. & Davis, K. K. Cats’ Paws and Catapults: Mechanical Worlds of Nature and People (Norton, 2000).
Stoop, N., Lagrange, R., Terwagne, D., Reis, P. M. & Dunkel, J. Curvature-induced symmetry breaking determines elastic surface patterns. Nat. Mater. 14, 337–342 (2015).
Krishnamurthy, D., Katsikis, G., Bhargava, A. & Prakash, M. Schistosoma mansoni cercariae swim efficiently by exploiting an elastohydrodynamic coupling. Nat. Phys. 13, 266–271 (2016).
Wehner, M. et al. An integrated design and fabrication strategy for entirely soft, autonomous robots. Nature 536, 451–455 (2016).
Kwak, M. K., Jeong, H.-E., Kim, T.-i., Yoon, H. & Suh, K. Y. Bio-inspired slanted polymer nanohairs for anisotropic wetting and directional dry adhesion. Soft Matter 6, 1849–1857 (2010).
Guo, P., Weinstein, A. M. & Weinbaum, S. A hydrodynamic mechanosensory hypothesis for brush border microvilli. Am. J. Physiol. 279, F698–F712 (2000).
Harper, C. J., Swartz, S. M. & Brainerd, E. L. Specialized bat tongue is a hemodynamic nectar mop. Proc. Natl Acad. Sci. USA 110, 8852–8857 (2013).
Schwartz, E. A., Leonard, M. L., Bizios, R. & Bowser, S. S. Analysis and modeling of the primary cilium bending response to fluid shear. Am. J. Physiol. 272, F132–F138 (1997).
Young, Y. N., Downs, M. & Jacobs, C. R. Dynamics of the primary cilium in shear flow. Biophys. J. 103, 629–639 (2012).
Weinbaum, S., Zhang, X., Han, Y., Vink, H. & Cowin, S. C. Mechanotransduction and flow across the endothelial glycocalyx. Proc. Natl Acad. Sci. USA 100, 7988–7995 (2003).
Weinbaum, S., Tarbell, J. M. & Damiano, E. R. The structure and function of the endothelial glycocalyx layer. Annu. Rev. Biomed. Eng. 9, 121–167 (2007).
VanTeeffelen, J. W., Brands, J., Stroes, E. S. & Vink, H. Endothelial glycocalyx: sweet shield of blood vessels. Trends Cardiovasc. Med. 17, 101–105 (2007).
Vogel, S. Drag and reconfiguration of broad leaves in high winds. J. Exp. Bot. 40, 941–948 (1989).
Luhar, M. & Nepf, H. M. Flow-induced reconfiguration of buoyant and flexible aquatic vegetation. Limnol. Oceanogr. 56, 2003–2017 (2011).
Albayrak, I., Nikora, V., Miler, O. & O’Hare, M. T. Flow–plant interactions at leaf, stem and shoot scales: drag, turbulence, and biomechanics. Aquat. Sci. 76, 269–294 (2013).
Vollsinger, S., Mitchell, S. J., Byrne, K. E., Novak, M. D. & Rudnicki, M. Wind tunnel measurements of crown streamlining and drag relationships for several hardwood species. Can. J. For. Res. 35, 1238–1249 (2005).
Alben, S., Shelley, M. & Zhang, J. Drag reduction through self-similar bending of a flexible body. Nature 420, 479–481 (2002).
Favier, J., Dauptain, A., Basso, D. & Bottaro, A. Passive separation control using a self-adaptive hairy coating. J. Fluid Mech. 627, 451–483 (2009).
Battiato, I., Bandaru, P. R. & Tartakovsky, D. M. Elastic response of carbon nanotube forests to aerodynamic stresses. Phys. Rev. Lett. 105, 144504 (2010).
Gosselin, F., De Langre, E. & Machado-Almeida, B. Drag reduction of flexible plates by reconfiguration. J. Fluid Mech. 650, 319–341 (2010).
Gosselin, F. P. & de Langre, E. Drag reduction by reconfiguration of a poroelastic system. J. Fluids Struct. 27, 1111–1123 (2011).
Brücker, C. Progress in Turbulence and Wind Energy IV 191–196 (Springer, 2012).
de Langre, E., Gutierrez, A. & Cossé, J. On the scaling of drag reduction by reconfiguration in plants. C. R. Méc. 340, 35–40 (2012).
Schouveiler, L. & Eloy, C. Flow-induced draping. Phys. Rev. Lett. 111, 064301 (2013).
Leclercq, T. & de Langre, E. Drag reduction by elastic reconfiguration of non-uniform beams in non-uniform flows. J. Fluids Struct. 60, 114–129 (2016).
Duprat, C., Protière, S., Beebe, A. Y. & Stone, H. A. Wetting of flexible fibre arrays. Nature 482, 510–513 (2012).
Saintyves, B., Jules, T., Salez, T. & Mahadevan, L. Self-sustained lift and low friction via soft lubrication. Proc. Natl Acad. Sci. USA 113, 5847–5849 (2016).
Fluid-Structure Interactions in Low-Reynolds-Number Flows (eds Duprat, C. & Stone, H.) (RSC Soft Matter Series, The Royal Society of Chemistry, 2016).
Trouilloud, R., Yu, T. S., Hosoi, A. E. & Lauga, E. Soft swimming: exploiting deformable interfaces for low Reynolds number locomotion. Phys. Rev. Lett. 101, 048102 (2008).
Coq, N., du Roure, O., Marthelot, J., Bartolo, D. & Fermigier, M. Rotational dynamics of a soft filament: wrapping transition and propulsive forces. Phys. Fluids 20, 051703 (2008).
Wexler, J. S. et al. Bending of elastic fibres in viscous flows: the influence of confinement. J. Fluid Mech. 720, 517–544 (2013).
Kundu, P. K., Cohen, I. M. & Dowling, D. R. Fluid Mechanics (Elsevier Science, 2015).
Purcell, E. M. Life at low Reynolds number. Am. J. Phys. 45, 3–11 (1977).
Gopinath, A. & Mahadevan, L. Elastohydrodynamics of wet bristles, carpets and brushes. Proc. R. Soc. A 467, 1665–1685 (2011).
Brücker, C., Spatz, J. & Schröder, W. Feasability study of wall shear stress imaging using microstructured surfaces with flexible micropillars. Exp. Fluids 39, 464–474 (2005).
Große, S., Schröder, W. & Brücker, C. Nano-newton drag sensor based on flexible micro-pillars. Meas. Sci. Technol. 17, 2689–2697 (2006).
Brücker, C., Bauer, D. & Chaves, H. Dynamic response of micro-pillar sensors measuring fluctuating wall-shear-stress. Exp. Fluids 42, 737–749 (2007).
Brücker, C. Interaction of flexible surface hairs with near-wall turbulence. J. Phys. Condens. Matter 23, 184120 (2011).
Paek, J. & Kim, J. Microsphere-assisted fabrication of high aspect-ratio elastomeric micropillars and waveguides. Nat. Commun. 5, 3324 (2014).
Brücker, C. Evidence of rare backflow and skin-friction critical points in near-wall turbulence using micropillar imaging. Phys. Fluids 27, 031705 (2015).
Groisman, A. & Quake, S. A microfluidic rectifier: anisotropic flow resistance at low Reynolds numbers. Phys. Rev. Lett. 92, 094501 (2004).
Mates, J. E., Schutzius, T. M., Qin, J., Waldroup, D. E. & Megaridis, C. M. The fluid diode: tunable unidirectional flow through porous substrates. ACS Appl. Mater. Interfaces 6, 12837–12843 (2014).
Li, L., Mo, J. & Li, Z. Nanofluidic diode for simple fluids without moving parts. Phys. Rev. Lett. 115, 134503–134505 (2015).
Nasto, A. et al. Air entrainment in hairy surfaces. Phys. Rev. Fluids 1, 033905 (2016).
Acknowledgements
We thank A. Nasto, A. Helal, A. Gopinath, K. Hood and B. Keshavarz for insightful discussions; K. Broderick for fabrication assistance; F. Frankel for photography of hairs; and S. Lin for tensiometry experiment. A.E.H. acknowledges support from the Defense Advanced Research Projects Agency and US Army Research Office under grant numbers DARPA W31P4Q-13-1-0013 and ARO W911NF-15-1-0166. J.A. acknowledges support the US Army Research Office under grant number W911NF-14-1-0396.
Author information
Authors and Affiliations
Contributions
J.A., J.C. and A.E.H. conceived the project. J.A. performed experiments. J.A., J.C. and E.d.L. contributed to theoretical models. All authors contributed to the paper.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary information
Supplementary information (PDF 895 kb)
Rights and permissions
About this article
Cite this article
Alvarado, J., Comtet, J., de Langre, E. et al. Nonlinear flow response of soft hair beds. Nature Phys 13, 1014–1019 (2017). https://doi.org/10.1038/nphys4225
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nphys4225
This article is cited by
-
The fluidic memristor as a collective phenomenon in elastohydrodynamic networks
Nature Communications (2024)
-
Collective dynamics of dense hairy surfaces in turbulent flow
Scientific Reports (2023)
-
A passive Stokes flow rectifier for Newtonian fluids
Scientific Reports (2021)
-
Bending of Soft Micropatterns in Elastohydrodynamic Lubrication Tribology
Experimental Mechanics (2021)
-
Active hydrodynamic imaging of a rigid spherical particle
Scientific Reports (2020)