Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

  • Article
  • Published:

Determining the quantum expectation value by measuring a single photon

Nature Physics volume 13, pages 1191–1194 (2017)Cite this article

Subjects

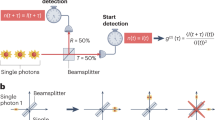
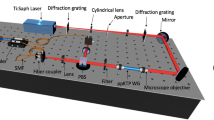
Abstract

One description provides only probabilities for obtaining various eigenvalues of a quantum variable. The eigenvalues and the corresponding probabilities specify the expectation value of a physical observable, which is known to be a statistical property of an ensemble of quantum systems. In contrast to this paradigm, here we demonstrate a method for measuring the expectation value of a physical variable on a single particle, namely, the polarization of a single protected photon. This realization of quantum protective measurements could find applications in the foundations of quantum mechanics and quantum-enhanced measurements.

This is a preview of subscription content, access via your institution

Access options

Buy this article

  • Purchase on Springer Link
  • Instant access to full article PDF
Buy now

Prices may be subject to local taxes which are calculated during checkout

Figure 1: Estimation of the polarization expectation value 〈P〉 by means of a single protective measurement.
Figure 2: Experimental setup.
Figure 3: Illustrative drawing showing the measurement of unprotected and protected photons.
Figure 4: Results obtained for the input state |ψ17π/60〉 = 0.629|H〉 + 0.777|V〉.
Figure 5: Comparison between the uncertainty on P with the PM approach (u(P)) and the one given by projective measurement (uPBS(P)).

Similar content being viewed by others

References

  1. Pusey, M. F., Barrett, J. & Rudolph, T. On the reality of the quantum state. Nat. Phys. 8, 475–478 (2012).

    Article  Google Scholar 

  2. Hardy, L. Are quantum states real? Int. J. Mod. Phys. B 27, 1345012 (2013).

    Article  ADS  MathSciNet  Google Scholar 

  3. Ringbauer, M. et al. Measurements on the reality of the wavefunction. Nat. Phys. 11, 249–254 (2015).

    Article  Google Scholar 

  4. Genovese, M. Interpretations of Quantum Mechanics and the measurement problem. Adv. Sci. Lett. 3, 249–258 (2010).

    Article  Google Scholar 

  5. Aharonov, Y. & Vaidman, L. Measurement of the Schrödinger wave of a single particle. Phys. Lett. A 178, 38–42 (1993).

    Article  ADS  Google Scholar 

  6. Rovelli, C. Comment on ‘Meaning of the wave function’. Phys. Rev. A 50, 2788–2792 (1994).

    Article  ADS  MathSciNet  Google Scholar 

  7. Unruh, W. G. Reality and measurement of the wave function. Phys. Rev. A 50, 882–887 (1994).

    Article  ADS  MathSciNet  Google Scholar 

  8. D’Ariano, G. M. & Yuen, H. P. Impossibility of measuring the wave function of a single quantum system. Phys. Rev. Lett. 76, 2832–2835 (1996).

    Article  ADS  Google Scholar 

  9. Aharonov, Y., Anandan, J. & Vaidman, L. The meaning of protective measurements. Found. Phys. 26, 117–126 (1996).

    Article  ADS  MathSciNet  Google Scholar 

  10. Dass, N. H. & Qureshi, T. Critique of protective measurements. Phys. Rev. A 59, 2590–2601 (1999).

    Article  ADS  Google Scholar 

  11. Uffink, J. How to protect the interpretation of the wave function against protective measurements. Phys. Rev. A 60, 3474–3481 (1999).

    Article  ADS  Google Scholar 

  12. Gao, S. Protective Measurement and Quantum Reality (Cambridge Univ. Press, 2015).

    Google Scholar 

  13. Aharonov, Y., Englert, B. G. & Scully, M. O. Protective measurements and Bohm trajectories. Phys. Lett. A 263, 137–146 (1999).

    Article  ADS  MathSciNet  Google Scholar 

  14. Schlosshauer, M. Measuring the quantum state of a single system with minimum state disturbance. Phys. Rev. A 93, 012115 (2016).

    Article  ADS  Google Scholar 

  15. Aharonov, Y. & Vaidman, L. in Potentiality, Entanglement and Passion-at-a-Distance (eds Cohen, R. S., Horne, M. & Stachel, J.) BSPS 1–8 (Kluwer, 1997).

    Book  Google Scholar 

  16. Misra, B. & Sudarshan, E. C. G. The Zeno’s paradox in quantum theory. J. Math. Phys. 18, 756–763 (1977).

    Article  ADS  MathSciNet  Google Scholar 

  17. Aharonov, Y., Albert, D. Z. & Vaidman, L. How the result of a measurement of a component of the spin of a spin-1/2 particle can turn out to be 100. Phys. Rev. Lett. 60, 1351–1354 (1988).

    ADS  Google Scholar 

  18. Dressel, J., Malik, M., Miatto, F. M., Jordan, A. N. & Boyd, R. W. Understanding quantum weak values: basics and applications. Rev. Mod. Phys. 86, 307–316 (2014).

    Article  ADS  Google Scholar 

  19. Piacentini, F. et al. Measuring incompatible observables by exploiting sequential weak values. Phys. Rev. Lett. 117, 170402 (2016).

    Article  ADS  Google Scholar 

  20. Thekkadath, G. S. et al. Direct measurement of the density matrix of a quantum system. Phys. Rev. Lett. 117, 120401 (2016).

    Article  ADS  Google Scholar 

  21. Itano, W. M., Heinzen, D. J., Bollinger, J. J. & Wineland, D. J. Quantum Zeno effect. Phys. Rev. A 41, 2295–2300 (1990).

    Article  ADS  Google Scholar 

  22. Kwiat, P. G. et al. High-efficiency quantum interrogation measurements via the quantum Zeno effect. Phys. Rev. Lett. 83, 4725–4728 (1999).

    Article  ADS  Google Scholar 

  23. Raimond, J. M. et al. Phase space tweezers for tailoring cavity fields by quantum Zeno dynamics. Phys. Rev. Lett. 105, 213601 (2010).

    Article  ADS  Google Scholar 

  24. Bretheau, L., Campagne-Ibarcq, P., Flurin, E., Mallet, F. & Huard, B. Quantum dynamics of an electromagnetic mode that cannot contain N photons. Science 348, 776–779 (2015).

    Article  ADS  MathSciNet  Google Scholar 

  25. Signoles, A. et al. Confined quantum Zeno dynamics of a watched atomic arrow. Nat. Phys. 10, 715–719 (2014).

    Article  Google Scholar 

  26. Mazzucchi, G., Kozlowski, W., Caballero-Benitez, S. F., Elliott, T. J. & Mekhov, I. B. Quantum measurement-induced dynamics of many-body ultracold bosonic and fermionic systems in optical lattices. Phys. Rev. A 93, 023632 (2016).

    Article  ADS  Google Scholar 

  27. Brida, G. et al. An extremely low-noise heralded single-photon source: a breakthrough for quantum technologies. Appl. Phys. Lett. 101, 221112 (2012).

    Article  ADS  Google Scholar 

  28. Villa, F. et al. CMOS imager with 1024 SPADs and TDCs for single-photon timing and 3-D time-of-flight. IEEE J. Sel. Top. Quantum Electron. 20, 3804810 (2014).

    Google Scholar 

  29. Paris, M. G. A. Quantum estimation for quantum technology. Int. J. Quantum Inf. 7, 125–137 (2009).

    Article  Google Scholar 

  30. Aharonov, Y., Albert, D. Z., Casher, A. & Vaidman, L. Surprising quantum effects. Phys. Lett. A 124, 199–203 (1987).

    Article  ADS  MathSciNet  Google Scholar 

Download references

Acknowledgements

This work has received funding from the European Union’s Horizon 2020 and the EMPIR Participating States in the context of the project EMPIR-14IND05 ‘MIQC2’, and from the INRIM ‘Seed’ project ‘GeQuM’. E.C. was supported by ERC AdG NLST. L.V. acknowledges support of the Israel Science Foundation Grant No. 1311/14 and the German-Israeli Foundation for Scientific Research and Development Grant No. I-1275-303.14. We wish to thank Y. Aharonov, S. Popescu and M. G. A. Paris for helpful discussion.

Author information

Authors and Affiliations

  1. INRIM, Strada delle Cacce 91, I-10135 Torino, Italy

    Fabrizio Piacentini, Alessio Avella, Enrico Rebufello, Marco Gramegna, Giorgio Brida, Ivo P. Degiovanni & Marco Genovese

  2. Politecnico di Torino, Corso Duca degli Abruzzi 24, I-10129 Torino, Italy

    Enrico Rebufello

  3. Dipartimento di Elettronica, Politecnico di Milano, Informazione e Bioingegneria, Piazza Leonardo da Vinci 32, 20133 Milano, Italy

    Rudi Lussana, Federica Villa & Alberto Tosi

  4. H. H. Wills Physics Laboratory, University of Bristol, Tyndall Avenue, Bristol BS8 1TL, UK

    Eliahu Cohen

  5. Raymond and Beverly Sackler School of Physics and Astronomy, Tel-Aviv University, Tel-Aviv 6997801, Israel

    Lev Vaidman

Authors
  1. Fabrizio Piacentini
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Alessio Avella
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Enrico Rebufello
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Rudi Lussana
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Federica Villa
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Alberto Tosi
    View author publications

    You can also search for this author in PubMed Google Scholar

  7. Marco Gramegna
    View author publications

    You can also search for this author in PubMed Google Scholar

  8. Giorgio Brida
    View author publications

    You can also search for this author in PubMed Google Scholar

  9. Eliahu Cohen
    View author publications

    You can also search for this author in PubMed Google Scholar

  10. Lev Vaidman
    View author publications

    You can also search for this author in PubMed Google Scholar

  11. Ivo P. Degiovanni
    View author publications

    You can also search for this author in PubMed Google Scholar

  12. Marco Genovese
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

I.P.D., M.Gramegna, F.P., A.A., M.Genovese (responsible for the laboratories), E.C. and L.V. (both responsible for the theoretical framework) planned the experiment. The experimental realization was achieved (supervised by I.P.D., G.B., M.Gramegna and M.Genovese) by F.P. (leading role), A.A. and E.R. The SPAD camera was developed and optimized for this experiment by R.L., F.V. and A.T. The manuscript was prepared with inputs by all the authors. They also had a fruitful systematic discussion on the progress of the work.

Corresponding author

Correspondence to Fabrizio Piacentini.

Ethics declarations

Competing interests

The authors declare no competing financial interests.

Supplementary information

Supplementary information

Supplementary information (PDF 889 kb)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Piacentini, F., Avella, A., Rebufello, E. et al. Determining the quantum expectation value by measuring a single photon. Nature Phys 13, 1191–1194 (2017). https://doi.org/10.1038/nphys4223

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/nphys4223

This article is cited by

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing