Abstract
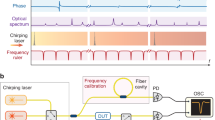
Temporal dissipative Kerr solitons in optical microresonators enable the generation of ultrashort pulses and low-noise frequency combs at microwave repetition rates. They have been demonstrated in a growing number of microresonator platforms, enabling chip-scale frequency combs, optical synthesis of low-noise microwaves and multichannel coherent communications. In all these applications, accessing and maintaining a single-soliton state is a key requirement—one that remains an outstanding challenge. Here, we study the dynamics of multiple-soliton states and report the discovery of a simple mechanism that deterministically switches the soliton state by reducing the number of solitons one by one. We demonstrate this control in Si3N4 and MgF2 resonators and, moreover, we observe a secondary peak to emerge in the response of the system to a pump modulation, an effect uniquely associated with the soliton regime. Exploiting this feature, we map the multi-stability diagram of a microresonator experimentally. Our measurements show the physical mechanism of the soliton switching and provide insight into soliton dynamics in microresonators. The technique provides a method to sequentially reduce, monitor and stabilize an arbitrary state with solitons, in particular allowing for feedback stabilization of single-soliton states, which is necessary for practical applications.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Del’Haye, P. et al. Optical frequency comb generation from a monolithic microresonator. Nature 450, 1214–1217 (2007).
Kippenberg, T. J., Holzwarth, R. & Diddams, S. Microresonator-based optical frequency combs. Science 332, 555–559 (2011).
Kippenberg, T. J., Spillane, S. M. & Vahala, K. J. Kerr-nonlinearity optical parametric oscillation in an ultrahigh-Q toroid microcavity. Phys. Rev. Lett. 93, 083904 (2004).
Savchenkov, A. A. et al. Low threshold optical oscillations in a whispering gallery mode CaF2 resonator. Phys. Rev. Lett. 93, 243905 (2004).
Herr, T. et al. Universal formation dynamics and noise of Kerr-frequency combs in microresonators. Nat. Photon. 6, 480–487 (2012).
Ferdous, F. et al. Spectral line-by-line pulse shaping of on-chip microresonator frequency combs. Nat. Photon. 5, 770–776 (2011).
Huang, S.-W. et al. A low-phase-noise 18 GHz Kerr frequency microcomb phase locked over 65 THz. Sci. Rep. 5, 13355 (2015).
Papp, S. et al. Microresonator frequency comb optical clock. Optica 1, 10–14 (2014).
Pfeifle, J. et al. Coherent terabit communications with microresonator Kerr frequency combs. Nat. Photon. 8, 375–380 (2014).
Pfeifle, J. et al. Full C and L-band transmission at 20 Tbit/s using cavity-soliton Kerr frequency combs. CLEO: 2015 Postdeadline Paper Digest JTh5C.8 (Optical Society of America, 2015).
Cundiff, S. & Weiner, A. Optical arbitrary waveform generation. Nat. Photon. 4, 760–766 (2010).
Del’Haye, P. et al. Phase-coherent microwave-to-optical link with a self-referenced microcomb. Nat. Photon. 10, 516–520 (2016).
Wang, C. et al. Mid-infrared optical frequency combs at 2.5 μm based on crystalline microresonators. Nat. Commun. 4, 1345 (2013).
Griffith, A. et al. Silicon-chip mid-infrared frequency comb generation. Nat. Commun. 6, 6299 (2015).
Herr, T. et al. Temporal solitons in optical microresonators. Nat. Photon. 8, 145–152 (2014).
Brasch, V. et al. Photonic chip–based optical frequency comb using soliton Cherenkov radiation. Science 351, 357–360 (2016).
Akhmediev, N. N. & Ankiewicz, A. Solitons Around Us: Integrable, Hamiltonian and Dissipative Systems 105–126 (Springer, 2003).
Leo, F. et al. Temporal cavity solitons in one-dimensional Kerr media as bits in an all-optical buffer. Nat. Photon. 4, 471–476 (2010).
Bao, C., Chang, W., Yang, C., Akhmediev, N. & Cundiff, S. T. Observation of coexisting dissipative solitons in a mode-locked fiber laser. Phys. Rev. Lett. 115, 253903 (2015).
Lugiato, L. A. & Lefever, R. Spatial dissipative structures in passive optical systems. Phys. Rev. Lett. 58, 2209–2211 (1987).
Barashenkov, I. V. & Smirnov, Y. S. Existence and stability chart for the ac-driven, damped nonlinear Schrödinger solitons. Phys. Rev. E 54, 5707–5725 (1996).
Yi, X., Yang, Q.-F., Yang, K. Y., Suh, M.-G. & Vahala, K. Soliton frequency comb at microwave rates in a high-Q silica microresonator. Optica 2, 1078–1085 (2015).
Wang, P.-H. et al. Intracavity characterization of micro-comb generation in the single-soliton regime. Opt. Express 24, 10890–10897 (2016).
Joshi, C. et al. Thermally controlled comb generation and soliton modelocking in microresonators. Opt. Lett. 41, 2565–2568 (2016).
Liang, W. et al. High spectral purity Kerr frequency comb radio frequency photonic oscillator. Nat. Commun. 6, 7957 (2015).
Akhmediev, N. & Karlsson, M. Cherenkov radiation emitted by solitons in optical fibers. Phys. Rev. A 51, 2602–2607 (1995).
Karpov, M. et al. Raman self-frequency shift of dissipative Kerr solitons in an optical microresonator. Phys. Rev. Lett. 116, 103902 (2016).
Ghelfi, P. et al. A fully photonics-based coherent radar system. Nature 507, 341–345 (2014).
Suh, M.-G., Yang, Q.-F., Yang, K. Y., Yi, X. & Vahala, K. Microresonator soliton dual-comb spectroscopy. Preprint at https://arXiv.org/abs/1607.08222 (2016).
Jost, J. D. et al. Counting the cycles of light using a self-referenced optical microresonator. Optica 2, 706–711 (2015).
Brasch, V., Lucas, E., Jost, J. D., Geiselmann, M. & Kippenberg, T. J. Self-referencing of an on-chip soliton Kerr frequency comb without external broadening. Preprint at https://arXiv.org/abs/1605.02801 (2016).
Grelu, P. & Akhmediev, N. Dissipative solitons for mode-locked lasers. Nat. Photon. 6, 84–92 (2012).
Coen, S., Randle, H., Sylvestre, T. & Erkintalo, M. Modeling of octave-spanning Kerr frequency combs using a generalized mean-field Lugiato–Lefever model. Opt. Lett. 38, 37–39 (2013).
Chembo, Y. & Menyuk, C. Spatiotemporal Lugiato–Lefever formalism for Kerr-comb generation in whispering-gallery-mode resonators. Phys. Rev. A 87, 053852 (2013).
Moss, D., Morandotti, R., Gaeta, L. & Lipson, M. New CMOS-compatible platforms based on silicon nitride and Hydex for nonlinear optics. Nat. Photon. 7, 597–607 (2013).
Foster, M. A. et al. Silicon-based monolithic optical frequency comb source. Opt. Express 19, 14233–14239 (2011).
Ilchenko, V. S., Savchenkov, A. A., Matsko, A. B. & Maleki, L. Nonlinear optics and crystalline whispering gallery mode cavities. Phys. Rev. Lett. 92, 043903 (2004).
Grudinin, I. S. et al. Ultra high Q crystalline microcavities. Opt. Commun. 265, 33–38 (2006).
Liang, W. et al. Generation of near-infrared frequency combs from a MgF2 whispering gallery mode resonator. Opt. Lett. 36, 2290–2292 (2011).
Braginsky, V. B., Gorodetsky, M. L. & Ilchenko, V. S. Quality-factor and nonlinear properties of optical whispering-gallery modes. Phys. Lett. A 137, 393–397 (1989).
Carmon, T., Yang, L. & Vahala, K. Dynamical thermal behavior and thermal self-stability of microcavities. Opt. Express 12, 4742–4750 (2004).
Fomin, A. E., Gorodetsky, M. L., Grudinin, I. S. & Ilchenko, V. S. Nonstationary nonlinear effects in optical microspheres. J. Opt. Soc. Am. B 22, 459–465 (2005).
Lee, C.-C. & Schibli, T. R. Intrinsic power oscillations generated by the backaction of continuum on solitons and its implications on the transfer functions of a mode-locked laser. Phys. Rev. Lett. 112, 223903 (2014).
Wahlstrand, J. K., Willits, J. T., Schibli, T. R., Menyuk, C. R. & Cundiff, S. T. Quantitative measurement of timing and phase dynamics in a mode-locked laser. Opt. Lett. 32, 3426–3428 (2007).
Bao, C., Funk, A. C., Yang, C. & Cundiff, S. T. Pulse dynamics in a mode-locked fiber laser and its quantum limited comb frequency uncertainty. Opt. Lett. 39, 3266–3269 (2014).
Matsko, A. B. & Maleki, L. Feshbach resonances in Kerr frequency combs. Phys. Rev. A 91, 013831 (2015).
Gasch, A., Wedding, B. & Jager, D. Multistability and soliton modes in nonlinear microwave resonators. Appl. Phys. Lett. 44, 1105–1107 (1984).
Yi, X., Yang, Q.-F., Yang, K. Y. & Vahala, K. Active capture and stabilization of temporal solitons in microresonators. Opt. Lett. 41, 2037–2040 (2016).
Pfeiffer, M. H. P. et al. Photonic Damascene process for integrated high-Q microresonator based nonlinear photonics. Optica 3, 20–25 (2016).
Herr, T. et al. Mode spectrum and temporal soliton formation in optical microresonators. Phys. Rev. Lett. 113, 123901 (2014).
Kordts, A., Pfeiffer, M. H. P., Guo, H., Brasch, V. & Kippenberg, T. J. Higher order mode suppression in high-Q anomalous dispersion SiN microresonators for temporal dissipative Kerr soliton formation. Opt. Lett. 41, 452–455 (2016).
Del’Haye, P., Arcizet, O., Gorodetsky, M. L., Holzwarth, R. & Kippenberg, T. J. Frequency comb assisted diode laser spectroscopy for measurement of microcavity dispersion. Nat. Photon. 3, 529–533 (2009).
Acknowledgements
This publication was supported by the Swiss National Science Foundation (SNF) as well as Contract W31P4Q-14-C-0050 from the Defense Advanced Research Projects Agency (DARPA), Defense Sciences Office (DSO). This material is based upon work supported by the Air Force Office of Scientific Research, Air Force Material Command, USAF under Award No. FA9550-15-1-0099. This publication was supported by funding from the European Space Agency (ESA), European Space Research and Technology Centre (ESTEC). G.L., V.E.L. and M.L.G. were supported by the Ministry of Education and Science of the Russian Federation project RFMEFI58516X0005. The authors gratefully acknowledge valuable discussions with M. Geiselmann and J. D. Jost. All samples were fabricated and grown in the Center of MicroNanoTechnology (CMi) at EPFL.
Author information
Authors and Affiliations
Contributions
M.K. designed and performed the experiments and analysed the data. H.G. conceived and initiated the numerical simulations with thermal effects. E.L. performed experiments in MgF2 microresonators and analysed the data. A.K. fabricated the Si3N4 samples and M.H.P.P. developed the fabrication method. V.B. assisted in experiments. G.L. and V.E.L. assisted in simulations. M.L.G. developed the theory and performed the simulations. M.K., H.G., E.L., M.L.G. and T.J.K. discussed all data in the manuscript. M.K. and H.G. wrote the manuscript, with input from E.L., M.L.G. and T.J.K. The project was supervised by T.J.K.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary information
Supplementary information (PDF 535 kb)
Supplementary Movie 1
Supplementary Movie (MOV 8281 kb)
Supplementary Figure 1
Supplementary Figure (GIF 919 kb)
Rights and permissions
About this article
Cite this article
Guo, H., Karpov, M., Lucas, E. et al. Universal dynamics and deterministic switching of dissipative Kerr solitons in optical microresonators. Nature Phys 13, 94–102 (2017). https://doi.org/10.1038/nphys3893
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nphys3893
This article is cited by
-
Genetic algorithm-enhanced microcomb state generation
Communications Physics (2024)
-
Strong interactions between solitons and background light in Brillouin-Kerr microcombs
Nature Communications (2024)
-
Synthetic reflection self-injection-locked microcombs
Nature Photonics (2024)
-
Nozaki–Bekki solitons in semiconductor lasers
Nature (2024)
-
Breaking the efficiency limitations of dissipative Kerr solitons using nonlinear couplers
Science China Physics, Mechanics & Astronomy (2024)