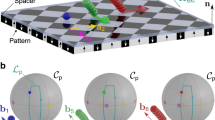
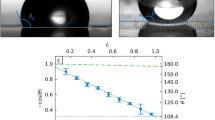
Abstract
Droplets are versatile digital materials; they can be produced at high throughput, perform chemical reactions as miniature beakers and carry biological entities. Droplets have been manipulated with electric, optical, acoustic and magnetic forces, but all these methods use serial controls to address individual droplets. An alternative is algorithmic manipulation based on logic operations that automatically compute where droplets are stored or directed, thereby enabling parallel control. However, logic previously implemented in low-Reynolds-number droplet hydrodynamics is asynchronous and thus prone to errors that prevent scaling up the complexity of logic operations. Here we present a platform for error-free physical computation via synchronous universal logic. Our platform uses a rotating magnetic field that enables parallel manipulation of arbitrary numbers of ferrofluid droplets on permalloy tracks. Through the coupling of magnetic and hydrodynamic interaction forces between droplets, we developed AND, OR, XOR, NOT and NAND logic gates, fanouts, a full adder, a flip-flop and a finite-state machine. Our platform enables large-scale integration of droplet logic, analogous to the scaling seen in digital electronics, and opens new avenues in mesoscale material processing.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout






Similar content being viewed by others
References
Zuse, K. Calculating Space MIT Technical Translation AZT-70-164-GEMIT (Massachusetts Institute of Technology, 1970).
Landauer, R. The physical nature of information. Phys. Lett. A 217, 188–193 (1996).
Wheeler, J. A. Information, physics, quantum: The search for links. Proc. III International Symposium on Foundations of Quantum Mechanics 354–368 (1989).
Floyd, T. Digital Fundamentals 10th edn (Prentice Hall, 2008).
Chee, M. et al. Accessing genetic information with high-density DNA arrays. Science 274, 610–614 (1996).
Winfree, E., Liu, F., Wenzler, L. A. & Seeman, N. C. Design and self-assembly of two-dimensional DNA crystals. Nature 394, 539–544 (1998).
Mao, C., LaBean, T. H., Reif, J. H. & Seeman, N. C. Logical computation using algorithmic self-assembly of DNA triple-crossover molecules. Nature 407, 493–496 (2000).
Teh, S-Y., Lin, R., Hund, L-H. & Lee, A. P. Droplet microfluidics. Lab Chip 8, 198–220 (2008).
Garstecki, P., Fuerstman, M. J., Stone, H. A. & Whitesides, G. M. Formation of droplet and bubbles in a microfluidic T-junction-scaling and mechanism of break-up. Lab Chip 6, 437–446 (2006).
Schwarz, J. A., Vykoukal, J. V. & Gascoyne, P. R. Droplet-based chemistry on a programmable micro-chip. Lab Chip 4, 11–17 (2004).
Song, H., Chen, D. L. & Ismagilov, R. F. Reactions in droplets in microfluidic channels. Angew. Chem. Int. Ed. 45, 7336–7356 (2006).
Schneider, T., Kreutz, J. & Chiu, D. T. The potential impact of droplet microfluidics in biology. Anal. Chem. 85, 3476–3482 (2013).
Gascoyne, P. R. et al. Dielectrophoresis-based programmable fluidic processors. Lab Chip 4, 299–309 (2004).
Link, D. R. et al. Electric control of droplets in microfluidic devices. Angew. Chem. Int. Ed. 45, 2556–2560 (2006).
Ahn, K. et al. Dielectrophoretic manipulation of drops for high-speed microfluidic sorting devices. Appl. Phys. Lett. 88, 024104 (2006).
Brzobohaty, O., Siler, M., Jezek, J., Jakl, P. & Zemanek, P. Optical manipulation of aerosol droplet using a holographic dual and single beam trap. Opt. Lett. 38, 4601–4604 (2013).
Wixforth, A. et al. Acoustic manipulation of small droplets. Anal. Bioanal. Chem. 379, 982–991 (2004).
Pamme, N. Magnetism and microfluidics. Lab Chip 6, 24–38 (2006).
Prakash, M. & Gershenfeld, N. Microfluidic bubble logic. Science 315, 832–835 (2007).
Cheow, L. F., Yobas, L. & Kwong, D-L. Digital microfluidics: Droplet based logic gates. Appl. Phys. Lett. 90, 054107 (2007).
Cybulski, O. & Garstecki, P. Dynamic memory in a microfluidic system of droplets travelling through a simple network of microchannel. Lab Chip 10, 484–493 (2010).
Fuertman, M. J., Gartecki, P. & Whitesides, G. M. Coding/decoding and reversibility of droplet trains in microfluidic networks. Science 315, 828–832 (2007).
Chang, H. Magnetic Bubble Technology: Integrated-Circuit Magnetics for Digital Storage and Processing (IEEE Press and Wiley, 1975).
Romankiw, L., Slusarczuk, M. M. G. & Thompson, D. A. Liquid magnetic bubbles. IEEE Trans. Magn. 11, 25–28 (1975).
Donolato, M. et al. Magnetic domain wall conduits for single cell applications. Lab Chip 11, 2976–2983 (2011).
Lim, B. et al. Magnetophoretic circuits for digital control of single particles and cells. Nature Commun. 5, 3846 (2014).
White, R. Viscous Fluid Flow 3rd edn (McGraw-Hill, 2006).
Rabaud, D. et al. Manipulation of confined bubbles in a thin microchannel: Drag and acoustic Bjerknes forces. Phys. Fluids 23, 042003 (2011).
McCaig, M. & Clegg, A. G. Permanent Magnets in Theory and Practice 2nd edn (Pentech Press, 1985).
Jiles, D. Introduction to Magnetism and Magnetic Materials 2nd edn (CRC Press, 1998).
Dangla, R. 2D Droplet Microfluidics Driven by Confinement Gradients Thesis, Ch. 3 (École Polytechnique 2012).
Nguyen, N-T., Ng, K. M. & Huang, X. Maninulation of ferrofluid droplet using planar coils. Appl. Phys. Lett. 89, 052509 (2006).
Toussaint, R., Akselvoll, J., Helgesen, G., Skjeltorp, A. T. & Flekkoy, E. G. Interaction model for magnetic holes in a ferrofluid layer. Phys. Rev. E 69, 011407 (2004).
Gans, B. J., Blom, C., Philipse, A. P. & Mellema, J. Linear viscoelasticity of an inverse ferrofluid. Phys. Rev. E 60, 4518–4527 (1999).
Gans, B. J., Duin, N. J., van den Ende, D. & Mellema, J. The influence of particle size on the magnetorheological properties of an inverse ferrofluid. J. Chem. Phys. 113, 2032–2042 (2000).
Acknowledgements
We acknowledge all members of the Prakash Lab for useful discussions. G.K. is supported by the Onassis Foundation and the A.G. Leventis Foundation. J.S.C. is supported by a grant from the Gordon and Betty Moore Foundation. M.P. is supported by the Pew Foundation, the Moore Foundation, the Keck Foundation, a Terman Fellowship and a NSF Career Award. We acknowledge S. X. Wang and A. El-Ghazaly for providing an alternating gradient magnetometer and helping with measurements of the Permalloy material. We also acknowledge Z. Hossain with regards to the C and Python codes written to obtain and read the data from the embedded microcontrollers.
Author information
Authors and Affiliations
Contributions
G.K. and M.P. designed the research and planned the experiments. G.K. performed the micro-fabrication, conducted the experiments and developed image processing tools. G.K. and J.S.C. developed the reduced-order models. G.K. derived the scaling laws and developed the logic gates. All authors analysed the data, interpreted the results and wrote the manuscript.
Corresponding author
Ethics declarations
Competing interests
A patent has been filed by Stanford University based on ideas presented here (PCT/US2013/056821).
Supplementary information
Supplementary Information
Supplementary Information (PDF 3748 kb)
Supplementary Movie
Supplementary Movie 1 (MOV 13828 kb)
Supplementary Movie
Supplementary Movie 2 (MOV 2041 kb)
Supplementary Movie
Supplementary Movie 3 (MOV 2444 kb)
Supplementary Movie
Supplementary Movie 4 (MOV 2305 kb)
Supplementary Movie
Supplementary Movie 5 (MOV 5713 kb)
Supplementary Movie
Supplementary Movie 6 (MOV 8722 kb)
Supplementary Movie
Supplementary Movie 7 (MOV 8382 kb)
Supplementary Movie
Supplementary Movie 8 (MOV 2015 kb)
Supplementary Movie
Supplementary Movie 9 (MOV 807 kb)
Supplementary Movie
Supplementary Movie 10 (MOV 1030 kb)
Supplementary Movie
Supplementary Movie 11 (MOV 1686 kb)
Supplementary Movie
Supplementary Movie 12 (MOV 5682 kb)
Supplementary Movie
Supplementary Movie 13 (MOV 4280 kb)
Supplementary Movie
Supplementary Movie 14 (MOV 809 kb)
Supplementary Movie
Supplementary Movie 15 (MOV 5711 kb)
Supplementary Movie
Supplementary Movie 16 (MOV 12134 kb)
Supplementary Movie
Supplementary Movie 17 (MOV 17339 kb)
Supplementary Movie
Supplementary Movie 18 (MOV 3571 kb)
Supplementary Movie
Supplementary Movie 19 (MOV 4167 kb)
Rights and permissions
About this article
Cite this article
Katsikis, G., Cybulski, J. & Prakash, M. Synchronous universal droplet logic and control. Nature Phys 11, 588–596 (2015). https://doi.org/10.1038/nphys3341
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nphys3341
This article is cited by
-
A programmable hybrid digital chemical information processor based on the Belousov-Zhabotinsky reaction
Nature Communications (2024)
-
Droplet-based logic gates simulation of viscoelastic fluids under electric field
Scientific Reports (2024)
-
Artificial microtubules for rapid and collective transport of magnetic microcargoes
Nature Machine Intelligence (2022)
-
A novel algorithm for rapid estimation of magnetic particle trajectory in arbitrary magnetophoretic devices under continuous fluid flow
Microfluidics and Nanofluidics (2022)
-
Mechanical computing
Nature (2021)