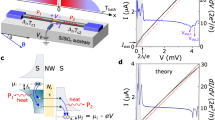
Abstract
Majorana fermions are the only fermionic particles that are expected to be their own antiparticles. Although elementary particles of the Majorana type have not been identified yet, quasi-particles with Majorana-like properties, born from interacting electrons in the solid, have been predicted to exist. Here, we present thorough experimental studies, backed by numerical simulations, of a system composed of an aluminium superconductor in proximity to an indium arsenide nanowire, with the latter possessing strong spin–orbit coupling and Zeeman splitting. An induced one-dimensional topological superconductor, supporting Majorana fermions at both ends, is expected to form. We concentrate on the characteristics of a distinct zero-bias conductance peak and its splitting in energy—both appearing only with a small magnetic field applied along the wire. The zero-bias conductance peak was found to be robustly tied to the Fermi energy over a wide range of system parameters. Although not providing definite proof of a Majorana state, the presented data and the simulations support its existence.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout






Similar content being viewed by others
References
Dirac, P. A. M. Quantum mechanics of many-electron systems. Proc. R. Soc. Lond. Ser. A 123, 714–733 (1929).
Majorana, E. Teoria simmetrica dell elettronee del positrone. Il Nuovo Cimento (1924-1942) 14, 171–184 (1937).
Wilczek, F. Majorana returns. Nature Phys. 5, 614–618 (2009).
Kitaev, A. Y. Unpaired Majorana fermions in quantum wires. Phys.-Usp. 44, 131–136 (2001).
Kopnin, N. & Salomaa, M. Mutual friction in superfluid 3He: Effects of bound states in the vortex core. Phys. Rev. B 44, 9667–9677 (1991).
Alicea, J. New directions in the pursuit of Majorana fermions in solid state systems. Rep. Prog. Phys. 75, 076501 (2012).
Beenakker, C. Search for Majorana fermions in superconductors. Preprint at http://arxiv.org/abs/1112.1950 (2011).
Kitaev, A. Y. Fault-tolerant quantum computation by anyons. Ann. Phys. 303, 2–30 (2003).
Halperin, B. I. et al. Adiabatic manipulations of Majorana fermions in a three-dimensional network of quantum wires. Phys. Rev. B 85, 144501 (2012).
Sau, J. D., Clarke, D. J. & Tewari, S. Controlling non-Abelian statistics of Majorana fermions in semiconductor nanowires. Phys. Rev. B 84, 094505 (2011).
Read, N. & Green, D. Paired states of fermions in two dimensions with breaking of parity and time-reversal symmetries and the fractional quantum Hall effect. Phys. Rev. B 61, 10267 (2000).
Kitaev, A. Anyons in an exactly solved model and beyond. Ann. Phys. 321, 2–111 (2006).
Alicea, J., Oreg, Y., Refael, G., Oppen, F. V. & Fisher, M. P. A. Non-Abelian statistics and topological quantum information processing in 1D wire networks. Nature Phys. 7, 412–417 (2011).
Fu, L. & Kane, C. L. Superconducting proximity effect and Majorana fermions at the surface of a topological insulator. Phys. Rev. Lett. 100, 96407 (2008).
Sau, J. D., Lutchyn, R. M., Tewari, S. & Sarma, S. D. Generic new platform for topological quantum computation using semiconductor heterostructures. Phys. Rev. Lett. 104, 40502 (2010).
Sau, J., Tewari, S., Lutchyn, R. & Stanescu, T. Non-Abelian quantum order in spin-orbit-coupled semiconductors: Search for topological Majorana particles in solid-state systems. Phys. Rev. B 82, 1–59 (2010).
Alicea, J. Majorana fermions in a tunable semiconductor device. Phys. Rev. B 81, 125318 (2010).
Lutchyn, R. M., Sau, J. D. & Sarma, S. D. Majorana fermions and a topological phase transition in semiconductor-superconductor heterostructures. Phys. Rev. Lett. 105, 77001 (2010).
Oreg, Y., Refael, G. & Oppen, F. V. Helical liquids and Majorana bound states in quantum wires. Phys. Rev. Lett. 105, 177002 (2010).
Mourik, V. et al. Signatures of Majorana fermions in hybrid superconductor-semiconductor nanowire devices. Science 336, 1003–1007 (2012).
Deng, M. T. et al. Observation of majorana fermions in a Nb–InSb nanowire-Nb hybrid quantum device. Preprint at http://arxiv.org/abs/1204.4130 (2012).
Potter, A. C. & Lee, P. A. Multichannel generalization of Kitaev’s Majorana end states and a practical route to realize them in thin films. Phys. Rev. Lett. 105, 227003 (2010).
Pientka, F., Kells, G., Romito, A., Brouwer, P. W. & von Oppen, F. Enhanced zero-bias Majorana peak in disordered multi-subband quantum wires. Preprint at http://arxiv.org/abs/1206.0723 (2012).
Rainis, D., Trifunovic, L., Klinovajo, J. & Loss, D. Realistic transport modeling for a superconducting nanowire with Majorana fermions. Preprint at http://arxiv.org/abs/1207.5907v1 (2012).
Bychkov, Y. A. & Rashba, E. Oscillatory effects and the magnetic susceptibility of carriers in inversion layers. J. Phys. C 17, 6039–6045 (1984).
Wimmer, M., Akhmerov, A., Dahlhaus, J. & Beenakker, C. Quantum point contact as a probe of a topological superconductor. New J. Phys. 13, 053016 (2011).
Bolech, C. & Demler, E. Observing majorana bound states in p-wave superconductors using noise measurements in tunneling experiments. Phys. Rev. Lett. 98, 237002 (2007).
Shtrikman, H., Popovitz-Biro, R., Kretinin, A. V. & Kacman, P. GaAs and InAs nanowires for ballistic transport. IEEE J. Selected Top. Quant. Electron. 17, 922–934 (2011).
Das, A. et al. Entangling electrons by splitting Cooper pairs: Two-particle conductance resonance and time coincidence measurements. Preprint at http://arxiv.org/abs/1205.2455 (2012).
Blonder, G., Tinkham, M. & Klapwijk, T. Transition from metallic to tunneling regimes in superconducting microconstrictions: Excess current, charge imbalance, and supercurrent conversion. Phys. Rev. B 25, 4515–4532 (1982).
Lin, C. H., Sau, J. D. & Sarma, S. D. Zero bias conductance peak in Majorana wires made of semiconductor-superconductor hybrid structures. Preprint at http://arxiv.org/abs/1204.3085 (2012).
Qu, C., Zhang, Y., Mao, L. & Zhang, C. Signature of majorana fermions in charge transport in semiconductor nanowires. Preprint at http://arxiv.org/abs/1109.4108 (2011).
Stanescu, T. D., Lutchyn, R. M. & Sarma, S. D. Majorana fermions in semiconductor nanowires. Phys. Rev. B 84, 144522 (2011).
Prada, E., San-Jose, P. & Aguado, R. Transport spectroscopy of NS nanowire junctions with Majorana fermions. Preprint at http://arxiv.org/abs/1203.4488 (2012).
Andreev, A. Thermal conductivity of superconductors intermediate state. Soviet Phys. ZhETP 46, 1823–1828 (1964).
Marmorkos, I., Beenakker, C. & Jalabert, R. Three signatures of phase-coherent Andreev reflection. Phys. Rev. B 48, 2811–2814 (1993).
Kastalsky, A. et al. Observation of pair currents in superconductor-semiconductor contacts. Phys. Rev. Lett. 67, 3026–3029 (1991).
Pillet, J. et al. Andreev bound states in supercurrent-carrying carbon nanotubes revealed. Nature Phys. 6, 965–969 (2010).
Klapwijk, T., Blonder, G. & Tinkham, M. Explanation of subharmonic energy gap structure in superconducting contacts. Physica B + C 109, 1657–1664 (1982).
Goldhaber-Gordon, D. et al. Kondo effect in a single-electron transistor. Nature 391, 156–159 (1998).
Kretinin, A. V. et al. Spin-1/2 Kondo effect in an InAs nanowire quantum dot: Unitary limit, conductance scaling, and Zeeman splitting. Phys. Rev. B 84, 245316 (2011).
Pikulin, D., Dahlhaus, J., Wimmer, M. & Beenakker, C. Zero-voltage conductance peak from weak antilocalization in a Majorana nanowire. Preprint at http://arxiv.org/abs/1206.6687 (2012).
Csonka, S. et al. Giant fluctuations and gate control of the g-factor in InAs nanowire quantum dots. Nano Lett. 8, 3932–3935 (2008).
Kretinin, A. V., Popovitz-Biro, R., Mahalu, D. & Shtrikman, H. Multimode Fabry–Perot conductance oscillations in suspended stacking-faults-free InAs nanowires. Nano Lett. 10, 3439–3445 (2010).
Fasth, C., Fuhrer, A., Samuelson, L., Golovach, V. N. & Loss, D. Direct measurement of the spin-orbit interaction in a two-electron InAs nanowire quantum dot. Phys. Rev. Lett. 98, 266801 (2007).
Kanai, Y. et al. Electrically tuned spin-orbit interaction in an InAs self-assembled quantum dot. Nature Nanotech. 6, 511–516 (2011).
Fu, L. Electron teleportation via Majorana bound states in a mesoscopic superconductor. Phys. Rev. Lett. 104, 56402 (2010).
Jiang, L. et al. Unconventional Josephson signatures of Majorana bound states. Phys. Rev. Lett. 107, 236401 (2011).
Nilsson, J., Akhmerov, A. & Beenakker, C. Splitting of a Cooper pair by a pair of Majorana bound states. Phys. Rev. Lett. 101, 120403 (2008).
Chevallier, D., Sticlet, D., Simon, P. & Bena, C. Mutation of Andreev into Majorana bound states in long superconductor- normal and superconductor-normal-superconductor junctions. Phys. Rev. B 85, 235307 (2012).
Acknowledgements
We thank A. Haim, A. Stern, F. von Oppen and G. Refael for useful discussions. We are grateful to R. Popovitz-Biro and D. Mahalu for their professional contribution and to A. Kretinin for laying the groundwork for nanowire device physics, and S. Ilani and A. Joshua for enabling us to perform the tilted field measurement. M.H. acknowledges the partial support of the Israeli Science Foundation (ISF), the Minerva foundation, the US-Israel Bi-National Science Foundation (BSF) and the European Research Council under the European Community’s Seventh Framework Program (FP7/2007-2013)/ERC Grant agreement # 227716. Y.O. acknowledges the partial support of the DFG, Minerva and that of the BSF. H.S. acknowledges the partial support of the ISF and the Israeli Ministry of Science and Technology (IMOST).
Author information
Authors and Affiliations
Contributions
A.D. and Y.R. contributed to sample design, device fabrication, set-up, data acquisition, analysis and writing of the paper. M.H. contributed to design, data interpretation and writing of the paper. Y.M. contributed to theory, simulations and writing of the paper. Y.O. contributed to theory, simulations, experimental insight and writing of the paper. H.S contributed to the Au-assisted vapour-liquid–solid molecular beam epitaxy growth and structural study of InAs nanowires, discussions and editing of the manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary Information
Supplementary Information (PDF 2893 kb)
Rights and permissions
About this article
Cite this article
Das, A., Ronen, Y., Most, Y. et al. Zero-bias peaks and splitting in an Al–InAs nanowire topological superconductor as a signature of Majorana fermions. Nature Phys 8, 887–895 (2012). https://doi.org/10.1038/nphys2479
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nphys2479
This article is cited by
-
Hosohedral nodal-line superconductivity in hexagonal ABC Dirac semimetals
Communications Physics (2024)
-
Superconducting tunnel junctions with layered superconductors
Quantum Frontiers (2024)
-
In search of Majorana
Nature Physics (2023)
-
Broad and colossal edge supercurrent in Dirac semimetal Cd3As2 Josephson junctions
Nature Communications (2023)
-
Subgap spectroscopy along hybrid nanowires by nm-thick tunnel barriers
Nature Communications (2023)