Abstract
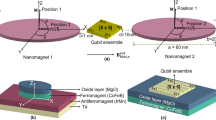
Isolated electronic and nuclear spins in solids are at present being actively explored for potential quantum-computing applications. Spin degrees of freedom provide an excellent quantum memory, owing to their weak magnetic interactions with the environment. For the same reason, however, it is difficult to achieve controlled interactions of spins over distances larger than tens of nanometres. Here we propose a new realization of a quantum data bus for spin qubits where spins are coupled to the motion of magnetized mechanical resonators through magnetic-field gradients. Provided that the mechanical system is charged, the magnetic moments associated with spin qubits can be effectively amplified to enable a coherent spin–spin coupling over long distances through Coulomb forces. Our approach is applicable to a wide class of electronic spin qubits, which can be localized near magnetized tips and can be used for the implementation of hybrid quantum-computing architectures.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout





Similar content being viewed by others
References
Cirac, J. I. & Zoller, P. Quantum computations with cold trapped ions. Phys. Rev. Lett. 74, 4091–4094 (1995).
Leibfried, D. et al. Creation of a six-atom ‘Schrödinger cat’ state. Nature 438, 639–642 (2005).
Hänsel, W. et al. Scalable multiparticle entanglement of trapped ions. Nature 438, 643–646 (2005).
Maunz, P. et al. Entanglement of single-atom quantum bits at a distance. Nature 449, 68–71 (2007).
Jelezko, F. & Wrachtrup, J. Read-out of single spins by optical spectroscopy. J. Phys. Condens. Matter 16, R1089–R1104 (2004).
Jelezko, F. et al. Observation of coherent oscillation of a single nuclear spin and realization of a two-qubit conditional quantum gate. Phys. Rev. Lett. 93, 130501 (2004).
Hanson, R., Mendoza, F. M., Epstein, R. J. & Awschalom, D. D. Polarization and readout of coupled single spins in diamond. Phys. Rev. Lett. 97, 087601 (2006).
Childress, L. et al. Coherent dynamics of coupled electron and nuclear spin qubits in diamond. Science 314, 281–285 (2006).
Dutt, M. V. G. et al. Quantum register based on individual electronic and nuclear spin qubits in diamond. Science 316, 1312–1316 (2007).
Kane, B. E. A silicon-based nuclear spin quantum computer. Nature 393, 133–137 (1998).
Tyryshkin, A. M. et al. Solid-state quantum memory using the P nuclear spin. Nature 455, 1085–1088 (2008).
Tyryshkin, A. M., Lyon, S. A., Astashkin, A. V. & Raitsimring, A. M. Electron spin relaxation times of phosphorus donors in silicon. Phys. Rev. B 68, 193207 (2003).
Stegner, A. R. et al. Electrical detection of coherent 31P spin quantum states. Nature Phys. 2, 835–838 (2006).
Harneit, W. Fullerene-based electron-spin quantum computer. Phys. Rev. A 65, 032322 (2002).
Benjamin, S. C. et al. Towards a fullerene-based quantum computer. J. Phys. Condens. Matter 18, S867–S883 (2006).
Morton, J. J. L. et al. Bang–bang control of fullerene qubits using ultrafast phase gates. Nature Phys. 2, 40–43 (2006).
Churchill, H. O. H. et al. Relaxation and dephasing in a two-electron 13C nanotube double quantum dot. Phys. Rev. Lett. 102, 166802 (2009).
Trauzettel, B., Bulaev, D. V., Loss, D. & Burkard, G. Spin qubits in graphene quantum dots. Nature Phys. 3, 192–196 (2007).
Vrijen, R. et al. Electron–spin-resonance transistors for quantum computing in silicon–germanium heterostructures. Phys. Rev. A 62, 012306 (2000).
Zwanenburg, F. A., van Rijmenam, C. E. W. M., Fang, Y., Lieber, C. M. & Kouwenhoven, L. P. Spin states of the first four holes in a silicon nanowire quantum dot. Nano Lett. 9, 1071–1079 (2009).
Rugar, D., Budakian, R., Mamin, H. J. & Chui, B. W. Single spin detection by magnetic resonance force microscopy. Nature 430, 329–332 (2004).
Mamin, H. J. et al. Isotope-selective detection and imaging of organic nanolayers. Nano Lett. 9, 3020–3024 (2009).
Naik, A. et al. Cooling a nanomechanical resonator with quantum back-action. Nature 443, 193–196 (2006).
Gigan, S. et al. Self-cooling of a micro-mirror by radiation pressure. Nature 444, 67–70 (2006).
Schliesser, A., Riviere, R., Anetsberger, G., Arcizet, O. & Kippenberg, T. J. Resolved-sideband cooling of a micromechanical oscillator. Nature Phys. 4, 415–419 (2008).
Thompson, J. D. et al. Strong dispersive coupling of a high-finesse cavity to a micromechanical membrane. Nature 452, 72–75 (2008).
Teufel, J. D., Harlow, J. W., Regal, C. A. & Lehnert, K. W. Dynamical backaction of microwave fields on a nanomechanical oscillator. Phys. Rev. Lett. 101, 197203 (2008).
Rabl, P. et al. Strong magnetic coupling between an electronic spin qubit and a mechanical resonator. Phys. Rev. B 79, 041302(R) (2009).
Armour, A. D., Blencowe, M. P. & Schwab, K. C. Entanglement and decoherence of a micromechanical resonator via coupling to a Cooper-pair box. Phys. Rev. Lett. 88, 148301 (2002).
Treutlein, P., Hunger, D., Camerer, S., Hänsch, T. W. & Reichel, J. Bose–Einstein condensate coupled to a nanomechanical resonator on an atom chip. Phys. Rev. Lett. 99, 140403 (2007).
Tian, L. & Zoller, P. Coupled ion–nanomechanical systems. Phys. Rev. Lett. 93, 266403 (2004).
Hensinger, W. K. et al. Ion trap transducers for quantum electromechanical oscillators. Phys. Rev. A 72, 041405 (2005).
Balasubramanian, G. et al. Ultralong spin coherence time in isotopically engineered diamond. Nature Mater. 8, 383–387 (2009).
Sorensen, A. & Molmer, K. Quantum computation with ions in thermal motion. Phys. Rev. Lett. 82, 1971–1974 (1999).
Molmer, K. & Sorensen, A. Multiparticle entanglement of hot trapped ions. Phys. Rev. Lett. 82, 1835–1838 (1999).
Wunderlich, C., Figger, H., Meschede, D. & Zimmermann, C. Laser Physics at the Limit (Springer, 2002).
Garca-Ripoll, J. J., Zoller, P. & Cirac, J. I. Speed optimized two-qubit gates with laser coherent control techniques for ion trap quantum computing. Phys. Rev. Lett. 91, 157901 (2003).
Garca-Ripoll, J. J., Zoller, P. & Cirac, J. I. Coherent control of trapped ions using off-resonant lasers. Phys. Rev. A 71, 062309 (2005).
Leggett, A. J. et al. Dynamics of the dissipative two-state system. Rev. Mod. Phys. 59, 1–85 (1987).
Kitagawa, M. & Ueda, M. Squeezed spin states. Phys. Rev. A 47, 5138–5143 (1993).
Dür, W. & Briegel, H. J. Entanglement purification for quantum computation. Phys. Rev. Lett. 90, 067901 (2003).
Jiang, L., Taylor, J. M., Sorensen, A. S. & Lukin, M. D. Distributed quantum computation based on small quantum registers. Phys. Rev. A 76, 062323 (2007).
de Sousa, R. & Das Sarma, S. Theory of nuclear-induced spectral diffusion: Spin decoherence of phosphorus donors in Si and GaAs quantum dots. Phys. Rev. B 68, 115322 (2003).
Coish, W. A. & Loss, D. Hyperfine interaction in a quantum dot: Non-Markovian electron spin dynamics. Phys. Rev. B 70, 195340 (2004).
Maze, J. R., Taylor, J. M. & Lukin, M. D. Electron spin decoherence of single nitrogen-vacancy defects in diamond. Phys. Rev. B 78, 094303 (2008).
Zimmerli, G., Eiles, T. M., Kautz, R. L. & Martinis, J. M. Noise in the Coulomb blockade electrometer. Appl. Phys. Lett. 61, 237–239 (1992).
Haeberlen, U. High Resolution NMR in Solids: Selective Averaging (Academic, 1976).
Uhrig, G. S. Keeping a quantum bit alive by optimized π-pulse sequences. Phys. Rev. Lett. 98, 100504 (2007).
Raussendorf, R. & Briegel, H. J. A one-way quantum computer. Phys. Rev. Lett. 86, 5188–5191 (2001).
Garg, A., Onuchic, J. N. & Ambegaokar, V. Effect of friction on electron transfer in biomolecules. J. Chem. Phys. 83, 4491–4503 (1985).
Acknowledgements
We gratefully acknowledge discussion with M. Aspelmeyer and K. Schwab. This work is supported by ITAMP, NSF, CUA, DARPA and the Packard Foundation. P.Z. acknowledges support by SFB FOQUS and EU Networks.
Author information
Authors and Affiliations
Contributions
P.R. carried out the theoretical analysis of the coupling scheme. All authors contributed to the initial ideas, discussions of the results and writing the manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary Information
Supplementary Information (PDF 281 kb)
Rights and permissions
About this article
Cite this article
Rabl, P., Kolkowitz, S., Koppens, F. et al. A quantum spin transducer based on nanoelectromechanical resonator arrays. Nature Phys 6, 602–608 (2010). https://doi.org/10.1038/nphys1679
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nphys1679
This article is cited by
-
A local probe for many-body physics
Nature Physics (2023)
-
Robust oscillator-mediated phase gates driven by low-intensity pulses
Communications Physics (2023)
-
A novel method to realize quantum spin-phonon Hall insulator in a one-dimensional superconducting resonator lattice
Quantum Information Processing (2023)
-
Quantum parametric amplification of phonon-mediated magnon-spin interaction
Science China Physics, Mechanics & Astronomy (2023)
-
Suppressing laser phase noise in an optomechanical system
Frontiers of Physics (2022)