Main

The experiment3 that observed strong evidence for the long-predicted Efimov effect1,2 has spawned a new level of confidence in our theoretical understanding of the three-body problem with short-range forces. However, even though in some respects the three-body problem is beginning to seem ‘almost solved’, the next step in complexity—to four interacting particles—remains at a primitive stage, comparatively speaking. Although a few studies have been pursued9,10,11,12, the non-perturbative four-boson problem is still largely uncharted territory, especially for processes that begin or end with three or four free particles. Our present study relates most to the pioneering work of Hammer, Platter and Meißner9,10, and of Yamashita et al. 11. It concerns a key question in strongly interacting few-body systems: are universal principles of Efimov physics relevant for the four-boson problem? In the early 1970s, the nuclear physicist Vitaly Efimov1,2 predicted on very general grounds that three neutral bosons, whose mutual interaction is characterized by a large value for the two-body s-wave scattering length a, can form a large number of weakly bound states whenever |a|≫r0, where r0 is the characteristic range of the interaction. Surprisingly, this could happen even when none of the pairs can bind (a<0). Here we provide an analysis that convincingly demonstrates the existence of a class of universal four-boson states that are intimately related to the Efimov effect. Our results connect with and extend previous analyses9,10,11 and provide a more complete landscape of the universal four-boson phenomena. In addition, we demonstrate how four-boson universal states can be seen (and in retrospect, apparently have already been seen) in ultracold-gas experiments.

Our theoretical model hinges on the tunable interaction strength achievable in ultracold-gas experiments. For alkali atoms, when an external B field is placed near a Fano–Feshbach resonance17 a small change of B can cause a to vary from to , allowing for the exploration of a vast range of interatomic interaction strengths. We mimic such variations in a by explicitly modifying the interatomic interactions5. In our framework, the solution of the four-body problem culminates with the solution of the ‘hyper-radial’ Schrödinger equation:

where the hyper-radius R describes the overall size of the system. Here, m is the atomic mass, E the total energy and Fν(R) the hyper-radial wavefunction, with ν representing the set of quantum numbers needed to label each channel. In the hyperspherical adiabatic representation,5,18 most of the complexity of the problem arises in solving the hyperangular equations to determine the effective potentials Wν ν(R) and couplings Wν ν′(R), in this case using a correlated Gaussian hyperspherical approach19. The reduction of the problem to the hyper-radial Schrödinger equation (1) then leads to a simple, intuitive picture: the effective potentials Wν ν(R) support all bound and quasibound states of the system, and the off-diagonal non-adiabatic couplings Wν ν′(R) drive inelastic transitions among different channels.

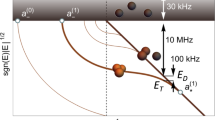
We explore the universality of the four-boson system and its relation to Efimov physics by solving the Schrödinger equation (1) for different model potentials using two complementary numerical techniques: the adiabatic hyperspherical approximation18,20, and the correlated Gaussian basis set expansion14,21. We base our conclusions on the analysis of energies, potential curves and wavefunctions that describe ground and excited states (see Supplementary Information for details) in the universal regime |a|≫r0, where r0 is the characteristic length scale of the two-body interaction, usually associated with the van der Waals length. Figure 1a shows the ‘generalized Efimov plot’10, with all important features that relate two-, three- and four-body physics. Figure 1a shows our numerical results for the four-boson energies (solid black lines) along with the dimer–dimer and dimer–atom–atom break-up thresholds for a>0 (solid red lines) and the energies of the Efimov states (dashed green lines) representing the atom–trimer break-up threshold. Figure 1c,d conveys the geometrical nature of the four-body states taking into account the extremely ‘floppy’ nature of the Efimov trimer states, in which all possible triangular shapes, and even linear configurations, are comparably probable22,23.

Figure 1: Spectrum of energies and geometric structure of four-boson states and their connection to the Efimov physics.
figure 1

a,The energy spectrum of four bosons as a function of the atom–atom scattering length a using the auxiliary function F(x)≡sgn(x)ln(1+|x|) designed to aid the visualization of the full energy landscape. Black solid lines correspond to four-body states, dashed green lines represent atom–trimer dissociation thresholds and red lines correspond to dimer–atom–atom (upper) and dimer–dimer (lower) thresholds. b, Schematic description of dimer–atom–atom Efimov states. c, d, Geometrical nature of ground and excited four-body states, respectively, for different trimer configurations. For the four-boson ground state, the isosurfaces shown are those where the probability of finding the fourth atom is 0.9 and 0.99 of the maximum probability for that particular trimer geometry. The probability isosurface for the first excited four-boson state in the portion of space where the Efimov trimer resides at its most probable equilateral triangular geometry implies that the fourth atom is very weakly bound, and its size exceeds that of the ground state considerably.

Full size image

Figure 1a implies that the four-boson spectrum, throughout the range r0/|a|≪1, is characterized by precisely two tetramer states that are associated with each three-body Efimov state, confirming the ref. 10 prediction. In fact, our extensive numerical tests show that these four-boson energies obey a universal relationship to the corresponding Efimov state energy, which at unitarity () can be expressed as

where E3b(n) is the energy of the nth Efimov state, n=0,1,2,…, and E4b(n,m), m=1 and 2, are the two tetramer energies associated with it (see Supplementary Information for details). Here, we find that the universal relation between three- and four-body energies is characterized by these two universal numbers, equal to: c1≈4.58 and c2∼1.01. For the lowest two four-boson states ref. 10 obtained c1≈5 and c2∼1.01. Similar values, less deep in the universal regime, can be extracted from the small B2 limit of equations (39) and (41) of ref. 9. Our own calculations differ from the universal values if we consider the lowest four-body states (see Supplementary Information). In fact, we believe that the ability of the current method to calculate many more weakly-bound energy levels than previous techniques has been decisive, and it permits us to verify the universal numbers up to 2% accuracy and resolve a previously-existing disagreement in the literature, between the results of Hammer, Platter and Meißner9,10 and those of Yamashita et al.11.

The resolution of this controversy is related to the fundamental question of whether or not an additional ‘four-body parameter’, encapsulating non-universal aspects from the details of the interactions, is required to specify the nature of the four-boson spectrum and scattering observables, akin to the usual three-body parameter1,2,5,6,7,24,25. Specifically, we support the conclusions of refs 9, 10, 12 that no additional four-body parameter enters at leading order in the description of universal four-body spectra and scattering properties. Our result also explains the observations of Yamashita et al. 11 that the energies of more compact four-boson states can vary depending on the details of the interatomic interactions. Our understanding emerges from Fig. 2, showing the four-body effective potentials calculated at unitarity, . We have verified that the effective-potential curves scale with the size of the trimer state. Therefore, if the lowest Efimov state has a size that exceeds r0 only marginally, the minimum of the four-body potential is close enough to r0 and its four-body states are affected by the shape of the two-body interaction, that is, non-universal physics. On the other hand, if the lowest Efimov state is large compared with r0, then the minimum of the four-body potential lies at R≫r0 and the four-boson states probe almost no non-universal effects. Furthermore, the scaling behaviour of the potential curves implies that the three-body Efimov effect controls the four-boson spectrum. As a consequence, the four-boson states follow the same geometric scaling as the three-boson states, with successive energies related by the factor e−2π/s0≈1/515 and successive radii expanding by the factor eπ/s0≈22.7 (where s0≈1.00624). These four-boson states are not true bound states, of course, as was pointed out in ref. 10, so the preceding discussion relates to the real part of their energies.

Figure 2: Effective four-boson potentials for converging at large R to the atom–trimer thresholds.
figure 2

Solid and dashed black lines represent the four-boson states shown in Fig. 1a. The position in R of the minimum of these potentials scales with the size of the Efimov state, indicated in the figure by κi−1 (see Supplementary Information for definition). Therefore, when the trimer state is large the four-boson states associated with it will lie at large R, preventing access to the non-universal region R≲r0, whereby the four-boson states are universal.

Full size image

Parenthetically, we also confirm the existence of a class of four-body states that represent the Efimov effect for three bodies. As pointed out in ref. 8, these four-atom states occur whenever an Efimov state is created for a>0 (see Fig. 1b). In this case the atom–dimer scattering length, aad, has a pole and whenever |aad|≫a a series of four-atom, three-body Efimov states, namely dimer–atom–atom states. Numerically, we observe the emergence of an attractive dipole potential (∝−1/R2) in the dimer–atom–atom channel, for a≪R≪|aad|, confirming the existence of such four-boson states. In fact, the recent observation of an Efimov resonance in ref. 26 in an ultracold atom–molecule mixture could enable the probing of such four-body states experimentally. We also confirm the non-existence of a ‘true Efimov effect’ for four bosons in the spirit of the ref. 27 prediction (see Supplementary Information).

The universal four-body physics discussed above can readily be observed in ultracold quantum gases. In general, weakly bound states deeply affect the collisional properties of ultracold gases, enhancing the atomic and/or molecular losses. The relative importance of four-body processes, however, remains largely unexplored, and we could argue that such processes should be far less likely than two-body or three-body collisions in a typical low-density gas. On the other hand, near the threshold for formation of any four-boson states, the four-body scattering observables should show a resonant enhancement that dramatically affects the collisional behaviour of the gas, even at low densities. The results of the Innsbruck experiment3, realized at atomic densities of n(0)≈3×1012 cm−3, were interpreted under the premise that the atom loss stems just from three-body recombination, B+B+B→B2+B+Erel., which releases enough kinetic energy to eject the collision products. Sure enough, the experimental data show a resonant peak in the three-body recombination rate K3, more specifically at a three-body recombination length of , at a=−850 a.u., in agreement with theoretical expectations for the manifestation of Efimov physics through three-body recombination5,6,7.

Although the experimental Innsbruck data3 are reasonably well understood24,28,29,30, distinguishing three- and four-body losses is difficult, and four-body processes could still be embedded in the observed decay rates. Accordingly, we have reanalysed the Innsbruck data3, looking for possible signatures of new four-boson states. The key observation from our results is that for a<0, when an Efimov state is created, say at a=a3b*, it is accompanied by the creation of two four-boson states at slightly less negative values of a, and those states should enhance four-body processes in this experimentally explored region of a. Moreover, our calculations indicate that, once the scattering length a3b* is known, we know the scattering lengths at which such four-body states appear. This universal relation is determined by the energy spectrum (see Fig. 1a), namely

From our numerical calculations we have found that these relations are approximately fulfilled even when a3b* is not deep in the universal regime, suggesting an insensitivity to non-universal effects.

The main process where such states should appear is four-body recombination, where the four initially free atoms collide to recombine into the dimer–dimer channel, the dimer–atom–atom channel and/or the atom–trimer channel. Figure 3a,b, respectively, depicts this process through the effective potentials at scattering lengths very close to the threshold a3b* for formation of an Efimov state (green dashed line) and just past the point (|a|<|a3b*|) of its creation. When a four-boson state resides energetically close to the collision threshold, we expect a resonant enhancement to the four-body recombination rate, K4. A straightforward Wigner threshold-law analysis demonstrates that K4 approaches a constant as the collision energy is tuned to zero16,31, and thus four-body recombination can indeed potentially compete with three-body recombination in causing atomic losses.

Figure 3: Evidence of signatures of four-boson states through four-body recombination.
figure 3

a, b, Schematic representation of the pathways for four-body recombination when the Efimov state (green dashed line) is above and below the four-body break-up threshold, respectively. In a the only possible decay channels are associated with deeply bound (two- and three-body) states, whereas in b the main decay channel is to the available Efimov state plus a free atom. When a four-boson state crosses the four-body dissociation threshold, it resonantly affects the four-body recombination rate, enhancing the rate of atom losses in an ultracold gas. c, Comparison of ρ3eff (equation (4)) with the experimental data from ref. 3. The shown error bars refer to statistical uncertainty only (see ref. 3 for details). The vertical lines identify the critical scattering lengths where an Efimov state and its associated four-body states are created, respectively, a3b*≈−850 a.u., a4b1*≈0.43a3b*≈−370 a.u. and a4b2*≈0.90a3b*≈−770 a.u. The dashed curve is the 10 nK contribution from K3. d, The atom and trimer densities at 10 nK, demonstrating that four-body recombination can be used to form Efimov trimers.

Full size image

To assess the importance of K4 and quantify our predictions, we have calculated K4 by numerically solving equation (1) using a formula for K4 derived elsewhere31. The main difficulty in comparing our results with data is that existing experiments are probably unable to distinguish three- and four-body losses. We therefore introduce an effective three-body recombination rate, in which both three- and four-body physics are included:

where n(t) is the peak atomic density in the trap at time t, calculated by solving the time evolution rate equations. Figure 3c shows our recombination length5 for t=20 ms. For K3 we use the thermally averaged results of ref. 24 calculated for temperatures of 10, 200 and 250 nK, and adjust it to fit the Efimov resonance at a=a3b*=−850 a.u. and the experimental data for |a|>|a3b*|. Our results show that for this range of a three-body recombination is indeed the dominant loss process. For |a|<|a3b*|, however, we find much better agreement by assuming that four-body recombination is the dominant loss process—the dashed curve in Fig. 3c is the 10 nK contribution from K3. For this range of a, as indicated in Fig. 3c by the vertical dashed lines at a=a4b1* and a=a4b2*, K4 is resonantly enhanced when the two four-boson states are created (see the circled region in Fig. 1a). This agreement strongly suggests that the 2006 Innsbruck experiment3 also offers the first experimental evidence for the universal four-boson states we discussed here, although the agreement with the second resonance predicted for a=a4b2* and 10 nK requires some imagination—and for temperatures of 200 and 250 nK this resonance feature is washed out owing to thermal effects. Nevertheless, the verification of the universal constraint between three- and four-body physics (equation (3)) strengthens the conclusion that the main resonant feature3 at −850 a.u. is indeed an Efimov resonance.

Note also that for |a|>|a3b*| four-body recombination to Efimov states, B+B+B+B→B3+B (see Fig. 3b), is likely to be the dominant four-body decay pathway. Although three-body recombination tends to dominate the atom loss, the formation of Efimov states through four-body recombination is non-negligible. In Fig. 3d we show the atomic density nB and the density of trimers nB3 at 10 nK for a up to −10,000 a.u. Near the threshold for Efimov state formation little energy is released through four-body recombination (approximately the trimer binding energy) and the Efimov state can remain trapped. In this case, our results indicate that about 10% of the atoms will form trimers. For larger |a|, however, the trimer formation is strongly enhanced by a resonance associated with the lowest four-boson state attached to the second Efimov trimer (see Fig. 1a). In this case, we find that about 50% of the atoms will form trimers. Here, however, the energy released through four-body recombination ejects both the atom and the Efimov trimer. Nevertheless, in an experiment where only atoms are visible, the magnetic field could be set to a value such that |a|>|a3b*|, the number of atoms measured and the field ramped back to a value such that |a|<|a3b*|, where no trimers exist. The reappearance of atoms after this ramp would be a convincing signature of the first experimental realization of an ultracold gas of Efimov trimers.

Finally, the Innsbruck group has observed two resonant loss features in four-body recombination32 that satisfy our relations in equation (3), offering stronger experimental evidence of the universal four-body physics demonstrated here.

Methods

Effective three-body recombination.

The idea behind the definition of K3eff(a,t) is to obtain a quantity that encapsulates both three- and four-body contributions. Accordingly to our findings, the atomic losses can indeed be seen as an effective three-body recombination within a characteristic timescale, as explained below. That can presumably facilitate a comparison with the available experimental data.

The key ingredient in the derivation of K3eff(a,t) is the relation between the loss coefficient and the loss rates as given by

where N is the number of atoms involved in the recombination event. Note that K3 (or K4) represents a fundamental few-body entity, namely, the recombination probability per unit time for a single triad (or tetrad) in a unit volume squared (cubed)17. The first factor on the right-hand side of this equation represents the number of atoms lost in the N-atom recombination process, whereas the 1/N! factor accounts for the indistinguishability of the collision partners. The atoms are assumed not to be in a Bose–Einstein condensate.

The rate equation that governs the time evolution of the atomic density can be written as

or alternatively,

from which we define the effective L3eff(a,t). From this definition, and using the relation between LN and KN above, we can easily arrive at our definition of KNeff following the steps below:

We have in fact verified that for times t<∼t0=[n(0)2(K3+n(0)K4/3)]−1 (≈50 ms for our case) the time dependence of n(t) can be described as a result of the effective three-body rate in equation (4), by setting n(t)=n(0). For longer times, n(t) is affected primarily by three- or four-body processes depending on whether or not K3≫n(0)K4.