Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

  • Article
  • Published:

High-order multiphoton Thomson scattering

Nature Photonics volume 11, pages 514–520 (2017)Cite this article

Subjects

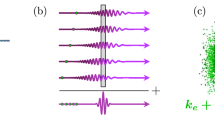
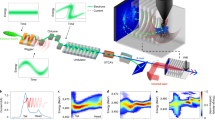
Abstract

Electron–photon scattering, or Thomson scattering, is one of the most fundamental mechanisms in electrodynamics, underlying laboratory and astrophysical sources of high-energy X-rays. After a century of studies, it is only recently that sufficiently high electromagnetic field strengths have been available to experimentally study the nonlinear regime of Thomson scattering in the laboratory. Making use of a high-power laser and a laser-driven electron accelerator, we made the first measurements of high-order multiphoton scattering, in which more than 500 near-infrared laser photons were scattered by a single electron into a single X-ray photon. Both the electron motion and the scattered photons were found to depend nonlinearly on field strength. The observed angular distribution of scattered X-rays permits independent measurement of absolute intensity, in situ, during interactions of ultra-intense laser light with free electrons. Furthermore, the experiment's potential to generate attosecond-duration hard X-ray pulses can enable the study of ultrafast nuclear dynamics.

This is a preview of subscription content, access via your institution

Access options

Buy this article

  • Purchase on Springer Link
  • Instant access to full article PDF
Buy now

Prices may be subject to local taxes which are calculated during checkout

Figure 1: Schematic of the experimental set-up used to study multiphoton TS.
Figure 2: Spatial characteristics of X-rays generated by multiphoton Thomson back-scattering.
Figure 3: Origin of diamond-shaped spatial profile of the scattered X-rays from multiphoton TS.
Figure 4: Angular dependence of X-ray energy distribution for low-order and high-order TS.
Figure 5: Dependence of the Thomson X-ray spectrum on the nonlinearity of the laser–electron interaction.
Figure 6: Scaling of total radiated power and on-axis solid-angle radiated power dP/dΩ.

Similar content being viewed by others

References

  1. Thomson, J. J. On electrical oscillations and the effects produced by the motion of an electrified sphere. Proc. Lond. Math. Soc. 1, 197–219 (1883).

    Article  MathSciNet  Google Scholar 

  2. Evans, D. E. & Katzenstein, J. Laser light scattering in laboratory plasmas. Rep. Prog. Phys. 32, 207–271 (1969).

    Article  ADS  Google Scholar 

  3. Longair, M. S. High-Energy Astrophysics (Cambridge Univ. Press, 2011).

    Google Scholar 

  4. Prunty, S. A primer on the theory of Thomson scattering for high-temperature fusion plasmas. Phys. Scripta 89, 128001 (2014).

    Article  Google Scholar 

  5. Glenzer, S. H. & Redmer, R. X-ray Thomson scattering in high energy density plasmas. Rev. Mod. Phys. 81, 1625–1663 (2009).

    Article  ADS  Google Scholar 

  6. Leemans, W. et al. X-ray based subpicosecond electron bunch characterization using 90° Thomson scattering. Phys. Rev. Lett. 77, 4182–4185 (1996).

    Article  ADS  Google Scholar 

  7. Powers, N. D. et al. Quasi-monoenergetic and tunable X-rays from a laser-driven Compton light source. Nat. Photon. 8, 28–31 (2014).

    Article  ADS  Google Scholar 

  8. Chen, S. et al. MeV-energy X rays from inverse Compton scattering with laser-wakefield accelerated electrons. Phys. Rev. Lett. 110, 155003 (2013).

    Article  ADS  Google Scholar 

  9. Schwoerer, H., Liesfeld, B., Schlenvoigt, H., Amthor, K. & Sauerbrey, R. Thomson-backscattered X rays from laser-accelerated electrons. Phys. Rev. Lett. 96, 014802 (2006).

    Article  ADS  Google Scholar 

  10. Albert, F. & Thomas, A. G. Applications of laser wakefield accelerator-based light sources. Plasma Phys. Control. Fusion 58, 103001 (2016).

    Article  ADS  Google Scholar 

  11. Corde, S. et al. Femtosecond X rays from laser-plasma accelerators. Rev. Mod. Phys. 85, 1–48 (2013).

    Article  ADS  Google Scholar 

  12. Rousse, A. et al. Production of a keV X-ray beam from synchrotron radiation in relativistic laser-plasma interaction. Phys. Rev. Lett. 93, 135005 (2004).

    Article  ADS  Google Scholar 

  13. Terzić, B., Deitrick, K., Hofler, A. S. & Krafft, G. A. Narrow-band emission in Thomson sources operating in the high-field regime. Phys. Rev. Lett. 112, 074801 (2014).

    Article  ADS  Google Scholar 

  14. Nakano, T. et al. Multi-GeV laser-electron photon project at SPring-8. Nucl. Phys. A 684, 71–79 (2001).

    Article  ADS  Google Scholar 

  15. Strickland, D. & Mourou, G. Compression of amplified chirped optical pulses. Opt. Commun. 55, 447–449 (1985).

    Article  ADS  Google Scholar 

  16. Bahk, S. et al. Generation and characterization of the highest laser intensities (1022 W/cm2). Opt. Lett. 29, 2837–2839 (2004).

    Article  ADS  Google Scholar 

  17. Chen, S., Maksimchuk, A. & Umstadter, D. Experimental observation of relativistic nonlinear Thomson scattering. Nature 396, 653–655 (1998).

    Article  ADS  Google Scholar 

  18. Babzien, M. et al. Observation of the second harmonic in Thomson scattering from relativistic electrons. Phys. Rev. Lett. 96, 054802 (2006).

    Article  ADS  Google Scholar 

  19. Sarri, G. et al. Ultrahigh brilliance multi-MeV Îł-ray beams from nonlinear relativistic Thomson scattering. Phys. Rev. Lett. 113, 224801 (2014).

    Article  ADS  Google Scholar 

  20. Khrennikov, K. et al. Tunable all-optical quasimonochromatic Thomson X-ray source in the nonlinear regime. Phys. Rev. Lett. 114, 195003 (2015).

    Article  ADS  Google Scholar 

  21. Phuoc, K. T. et al. All-optical Compton gamma-ray source. Nat. Photon. 6, 308–311 (2012).

    Article  ADS  Google Scholar 

  22. Sakai, Y. et al. Observation of red shifting and harmonic radiation in inverse Compton scattering. Phys. Rev. Spec. Top. Accel. Beams 18, 060702 (2015).

    Article  ADS  Google Scholar 

  23. Lau, Y. Y., He, F., Umstadter, D. P. & Kowalczyk, R. Nonlinear Thomson scattering: a tutorial. Phys. Plasmas 10, 2155–2162 (2003).

    Article  ADS  Google Scholar 

  24. Wiedemann, H. Particle Accelerator Physics (Springer, 2015).

    Book  Google Scholar 

  25. Sarachik, E. S. & Schappert, G. T. Classical theory of the scattering of intense laser radiation by free electrons. Phys. Rev. D 1, 2738–2753 (1970).

    Article  ADS  Google Scholar 

  26. Esarey, E., Ride, S. K. & Sprangle, P. Nonlinear Thomson scattering of intense laser pulses from beams and plasmas. Phys. Rev. E 48, 3003–3021 (1993).

    Article  ADS  Google Scholar 

  27. Koga, J., Esirkepov, T. Z. & Bulanov, S. V. Nonlinear Thomson scattering in the strong radiation damping regime. Phys. Plasmas 12, 093106 (2005).

    Article  ADS  Google Scholar 

  28. Rykovanov, S., Geddes, C., Schroeder, C., Esarey, E. & Leemans, W. Controlling the spectral shape of nonlinear Thomson scattering with proper laser chirping. Phys. Rev. Accel. Beams 19, 030701 (2016).

    Article  ADS  Google Scholar 

  29. Di Piazza, A., Müller, C., Hatsagortsyan, K. Z. & Keitel, C. H. Extremely high-intensity laser interactions with fundamental quantum systems. Rev. Mod. Phys. 84, 1177–1228 (2012).

    Article  ADS  Google Scholar 

  30. Quesnel, B. & Mora, P. Theory and simulation of the interaction of ultraintense laser pulses with electrons in vacuum. Phys. Rev. E 58, 3719–3732 (1998).

    Article  ADS  Google Scholar 

  31. Tajima, T. & Dawson, J. Laser electron accelerator. Phys. Rev. Lett. 43, 267–270 (1979).

    Article  ADS  Google Scholar 

  32. Esarey, E., Schroeder, C. & Leemans, W. Physics of laser-driven plasma-based electron accelerators. Rev. Mod. Phys. 81, 1229–1285 (2009).

    Article  ADS  Google Scholar 

  33. Har-Shemesh, O. & Di Piazza, A. Peak intensity measurement of relativistic lasers via nonlinear Thomson scattering. Opt. Lett. 37, 1352–1354 (2012).

    Article  ADS  Google Scholar 

  34. Pariente, G., Gallet, V., Borot, A., Gobert, O. & Quéré, F. Space–time characterization of ultra-intense femtosecond laser beams. Nat. Photon. 10, 547–553 (2016).

    Article  ADS  Google Scholar 

  35. Heinzl, T., Seipt, D. & Kaempfer, B. Beam-shape effects in nonlinear Compton and Thomson scattering. Phys. Rev. A 81, 022125 (2010).

    Article  ADS  Google Scholar 

  36. Chen, M. et al. Modeling classical and quantum radiation from laser-plasma accelerators. Phys. Rev. Spec. Top. Accel. Beams 16, 030701 (2013).

    Article  ADS  Google Scholar 

  37. Jochmann, A. et al. High resolution energy-angle correlation measurement of hard X rays from laser-Thomson backscattering. Phys. Rev. Lett. 111, 114803 (2013).

    Article  ADS  Google Scholar 

  38. Thomas, A., Ridgers, C., Bulanov, S., Griffin, B. & Mangles, S. Strong radiation-damping effects in a gamma-ray source generated by the interaction of a high-intensity laser with a wakefield-accelerated electron beam. Phys. Rev. X 2, 041004 (2012).

    Google Scholar 

  39. Vranic, M., Martins, J. L., Vieira, J., Fonseca, R. A. & Silva, L. O. All-optical radiation reaction at 1021 W/cm2. Phys. Rev. Lett. 113, 134801 (2014).

    Article  ADS  Google Scholar 

  40. Hartemann, F. V. et al. High-intensity scattering processes of relativistic electrons in vacuum and their relevance to high-energy astrophysics. Astrophys. J. Suppl. Ser. 127, 347–356 (2000).

    Article  ADS  Google Scholar 

  41. Bednarek, W., Kirk, J. & Mastichiadis, A. Production of gamma-rays by inverse Compton scattering in jets. Astron. Astrophys. Suppl. Ser. 120, 571–574 (1996).

    ADS  Google Scholar 

  42. Sambruna, R. M. et al. The high-energy continuum emission of the gamma-ray blazar PKS 0528 134. Astrophys. J. 474, 639–649 (1997).

    Article  ADS  Google Scholar 

  43. Stewart, P. & Sweeney, G. Collective relaxation of ultra-relativistic plasmas. Astron. Astrophys. 44, 1–7 (1975).

    ADS  Google Scholar 

  44. Li, J., Hatsagortsyan, K. Z., Galow, B. J. & Keitel, C. H. Attosecond gamma-ray pulses via nonlinear Compton scattering in the radiation-dominated regime. Phys. Rev. Lett. 115, 204801 (2015).

    Article  ADS  Google Scholar 

  45. Luo, W. et al. Generation of bright attosecond X-ray pulse trains via Thomson scattering from laser-plasma accelerators. Opt. Express 22, 32098–32106 (2014).

    Article  ADS  Google Scholar 

  46. Chung, S., Yoon, M. & Kim, D. E. Generation of attosecond X-ray and gamma-ray via Compton backscattering. Opt. Express 17, 7853–7861 (2009).

    Article  ADS  Google Scholar 

  47. Wu, H. & Meyer-ter-Vehn, J. Giant half-cycle attosecond pulses. Nat. Photon. 6, 304–307 (2012).

    Article  ADS  Google Scholar 

  48. Hartemann, F. V. et al. Nonlinear ponderomotive scattering of relativistic electrons by an intense laser field at focus. Phys. Rev. E 51, 4833–4843 (1995).

    Article  ADS  Google Scholar 

  49. Umstadter, D. Method of aligning a laser-based radiation source. US patent 9,485,847 (2016).

  50. Harvey, C., Heinzl, T. & Ilderton, A. Signatures of high-intensity Compton scattering. Phys. Rev. A 79, 063407 (2009).

    Article  ADS  Google Scholar 

Download references

Acknowledgements

The authors thank K. Brown, J. Mills, C. Petersen, N. Glasco, A. Okelberry, P. Mood and B. Nordell for their contributions. This work was supported primarily by the Air Force Office for Scientific Research, award number FA9550-14-1-0345. Additional support provided by the National Science Foundation, grant no. PHY-1535700 (ultra-low emittance electron beams); the US Department of Energy (DOE), Office of Science, Basic Energy Sciences (BES), award no. DE-FG02-05ER15663 (ultrafast X-ray science); and the Department of Homeland Security Domestic Nuclear Detection Office, competitively awarded contract HSHQDC-13-C-B0036 (low-dose X-ray radiography). This support does not constitute an express or implied endorsement on the part of the Government. M.C. acknowledges the support from the National Science Foundation of China (grant no. 11374209,11421064). Simulations were performed on the Π super computer at Shanghai Jiao Tong University. The authors also thank M. Fuchs from the University of Nebraska, Lincoln and Z.-M. Sheng from Shanghai Jiao Tong University and University of Strathclyde for useful discussions.

Author information

Authors and Affiliations

  1. Department of Physics and Astronomy, University of Nebraska-Lincoln, Lincoln, 68588, Nebraska, USA

    Wenchao Yan, Colton Fruhling, Grigory Golovin, Daniel Haden, Ping Zhang, Baozhen Zhao, Jun Zhang, Cheng Liu, Shouyuan Chen, Sudeep Banerjee & Donald Umstadter

  2. Key Laboratory for Laser Plasmas (Ministry of Education) and School of Physics and Astronomy, Shanghai Jiao Tong University, Shanghai, 200240, China

    Ji Luo & Min Chen

  3. Collaborative Innovation Center of IFSA (CICIFSA), Shanghai Jiao Tong University, Shanghai, 200240, China

    Ji Luo & Min Chen

Authors
  1. Wenchao Yan
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Colton Fruhling
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Grigory Golovin
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Daniel Haden
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Ji Luo
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Ping Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  7. Baozhen Zhao
    View author publications

    You can also search for this author in PubMed Google Scholar

  8. Jun Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  9. Cheng Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  10. Min Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

  11. Shouyuan Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

  12. Sudeep Banerjee
    View author publications

    You can also search for this author in PubMed Google Scholar

  13. Donald Umstadter
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

The experiments were conceived and designed by D.U., W.Y., S.B. and S.C. Experiments were performed by W.Y., C.F., D.H., G.G., P.Z., J.Z., B.Z. and C.L. Data was analysed by G.G., W.Y., D.H. and C.F. Simulation effort was provided by M.C., J.L., C.F. and S.C. The manuscript was written by D.U., W.Y., S.B., G.G., S.C. and M.C.

Corresponding author

Correspondence to Donald Umstadter.

Ethics declarations

Competing interests

The authors declare no competing financial interests.

Supplementary information

Supplementary information

Supplementary information (PDF 596 kb)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Yan, W., Fruhling, C., Golovin, G. et al. High-order multiphoton Thomson scattering. Nature Photon 11, 514–520 (2017). https://doi.org/10.1038/nphoton.2017.100

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/nphoton.2017.100

This article is cited by

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing