Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

Optical clock networks

Nature Photonics volume 11, pages 25–31 (2017)Cite this article

Subjects

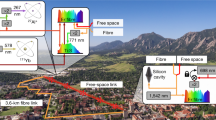
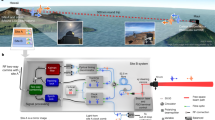
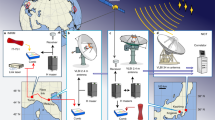
Abstract

Within the last decade, optical atomic clocks have surpassed the best cesium clocks, which are used to realize the unit of time and frequency, in terms of accuracy and stability by about two orders of magnitude. When remote optical atomic clocks are connected by links without degradation in the clock signals, an optical clock network is formed, with distinct advantages for the dissemination of time, geodesy, astronomy and basic and applied research. Different approaches for time and frequency transfer in the microwave and optical regime, via satellites and free-space links, optical fibre links, or transportable optical atomic clocks, can be used to form a hybrid clock network that may allow a future redefinition of the unit of time based on an optical reference transition.

This is a preview of subscription content, access via your institution

Relevant articles

Open Access articles citing this article.

Access options

Rent or buy this article

Get just this article for as long as you need it

$39.95

Learn more

Prices may be subject to local taxes which are calculated during checkout

Figure 1: Schematic of an optical atomic clock where optical transitions in atoms or ions are used to stabilize the frequency of a narrow-band laser to the centre of the transition.
Figure 2: Different methods can be used to compare the frequencies of optical atomic clocks.
Figure 3: Fractional instability of time and frequency transfer and clocks described by the Allan deviation (ADEV) or modified Allan deviation (mod ADEV) as function of the integration time τ.
Figure 4: Fibre links in Europe.

References

  1. Lewandowski, W. & Arias, E. F. GNSS times and UTC. Metrologia 48, S219–S224 (2011).

    ADS  Google Scholar 

  2. International Telecommunication Union ITU-T Recommendation ITU-T G.8271.1/Y.1366.1: Network Limits for Time Synchronization (ITU, 2013).

  3. Dzuba, V. A. et al. Strongly enhanced effects of Lorentz symmetry violation in entangled Yb+ ions. Nat. Phys. 12, 465–468 (2016).

    Google Scholar 

  4. Altschul, B. et al. Quantum tests of the Einstein equivalence principle with the STE–QUEST space mission. Adv. Space Res. 55, 501–524 (2015).

    ADS  Google Scholar 

  5. Uzan, J.-P. Varying constants, gravitation and cosmology. Living Rev. Relativity 14, 2 (2011).

    ADS  MATH  Google Scholar 

  6. Flury, J. Relativistic geodesy. J. Phys. Conf. Ser. 723, 012051 (2016).

    Google Scholar 

  7. Lorimer, D. R. Binary and millisecond pulsars. Living Rev. Relativity 11, 8 (2008).

    ADS  MATH  Google Scholar 

  8. Arzumanian, Z. The NANOGrav nine-year data set: observations, arrival time measurements, and analysis of 37 millisecond pulsars. Astrophys. J. 813, 65 (2015).

    ADS  Google Scholar 

  9. Poli, N., Oates, C. W., Gill, P. & Tino, G. M. Optical atomic clocks. Rivista del Nuovo Cimento 36, 555–624 (2013).

    ADS  Google Scholar 

  10. Ludlow, A. D., Boyd, M. M., Ye, J., Peik, E. & Schmidt, P. O. Optical atomic clocks. Rev. Mod. Phys. 87, 637–701 (2015).

    ADS  Google Scholar 

  11. Nicholson, T. L. et al. Systematic evaluation of an atomic clock at 2 × 10−18 total uncertainty. Nat. Commun. 6, 6896 (2015).

    ADS  Google Scholar 

  12. Ushijima, I., Takamoto, M., Das, M., Ohkubo, T. & Katori, H. Cryogenic optical lattice clocks. Nat. Photon. 9, 185–189 (2015).

    ADS  Google Scholar 

  13. Huntemann, N., Sanner, C., Lipphardt, B., Tamm, C. & Peik, E. Single-ion atomic clock with 3 × 10−18 systematic uncertainty. Phys. Rev. Lett. 116, 063001 (2016).

    ADS  Google Scholar 

  14. Hinkley, N. et al. An atomic clock with 10−18 instability. Science 341, 1215–1218 (2013).

    ADS  Google Scholar 

  15. Al-Masoudi, A., Dörscher, S., Häfner, S., Sterr, U. & Lisdat, C. Noise and instability of an optical lattice clock. Phys. Rev. A 92, 063814 (2015).

    ADS  Google Scholar 

  16. Fortier, T. M. et al. Precision atomic spectroscopy for improved limits on variation of the fine structure constant and local position invariance. Phys. Rev. Lett. 98, 070801 (2007).

    ADS  Google Scholar 

  17. Blatt, S. et al. New limits on coupling of fundamental constants to gravity using 87Sr optical lattice clocks. Phys. Rev. Lett. 100, 140801 (2008).

    ADS  Google Scholar 

  18. Godun, R. M. et al. Frequency ratio of two optical clock transitions in 171Yb+ and constraints on the time-variation of fundamental constants. Phys. Rev. Lett. 113, 210801 (2014).

    ADS  Google Scholar 

  19. Huntemann, N. et al. Improved limit on a temporal variation of m p /m e from comparisons of Yb+ and Cs atomic clocks. Phys. Rev. Lett. 113, 210802 (2014).

    ADS  Google Scholar 

  20. Bjerhammar, A. On a relativistic geodesy. Bull. Géodésique 59, 207–220 (1985).

    ADS  Google Scholar 

  21. Gill, P. Is the time right for a redefinition of the second by optical atomic clocks? J. Phys. Conf. Ser. 723, 012053 (2016).

    Google Scholar 

  22. Grebing, C. et al. Realization of a timescale with an accurate optical lattice clock. Optica 3, 563–569 (2015).

    ADS  Google Scholar 

  23. Ido, T., Hachisu, H., Nakagawa, F. & Hanado, Y. Rapid evaluation of time scale using an optical clock. J. Phys. Conf. Ser. 723, 012041 (2016).

    Google Scholar 

  24. Droste, S. et al. Characterization of a 450 km baseline GPS carrier-phase link using an optical fiber link. New J. Phys. 17, 083044 (2015).

    ADS  Google Scholar 

  25. Petit, G. et al. 1 × 10−16 frequency transfer by GPS PPP with integer ambiguity resolution. Metrologia 52, 301–309 (2015).

    ADS  Google Scholar 

  26. Bauch, A. Time and frequency comparisons using radiofrequency signals from satellites. C. R. Physique 16, 471–479 (2015).

    Google Scholar 

  27. Hachisu, H. et al. Direct comparison of optical lattice clocks with an intercontinental baseline of 9000 km. Opt. Lett. 39, 4072–4075 (2014).

    ADS  Google Scholar 

  28. Margolis, H. et al. International timescales with optical clocks. In Proc. 2013 Joint UFFC, EFTF PFM Symp. 908–911 (IEEE, 2013).

    Google Scholar 

  29. Fujieda, M. et al. Carrier-phase two-way satellite frequency transfer over a very long baseline. Metrologia 51, 253–262 (2014).

    ADS  Google Scholar 

  30. Fujieda, M., Gotoh, T. & Amagai, J. Advanced two-way satellite frequency transfer by carrier-phase and carrier-frequency measurements. J. Phys. Conf. Ser. 723, 012036 (2016).

    Google Scholar 

  31. Laurent, Ph., Massonnet, D., Cacciapuoti, L. & Salomon, C. The ACES/PHARAO space mission: La mission spatiale ACES/Pharao. C.R. Physique 16, 540–552 (2015).

    Google Scholar 

  32. Schäfer, W. & Feldmann, T. Perspectives of time and frequency transfer via satellite. J. Phys. Conf. Ser. 723, 012038 (2016).

    Google Scholar 

  33. Huang, Y.-J. & Tsao, H.-W. Design and evaluation of an open-loop receiver for TWSTFT applications. IEEE Trans. Instrum. Meas. 64, 1553–1558 (2015).

    Google Scholar 

  34. Samain, E. et al. Time transfer by laser link: a complete analysis of the uncertainty budget. Metrologia 52, 423–432 (2015).

    ADS  Google Scholar 

  35. Rovera, G. D. et al. A direct comparison between two independently calibrated time transfer techniques: T2L2 and GPS common-views. J. Phys. Conf. Ser. 723, 012037 (2016).

    Google Scholar 

  36. Schreiber, K. U. et al. Ground-based demonstration of the European Laser Timing (ELT) experiment. IEEE Trans. Ultrasonics, Ferroelectrics, Frequency Control 57, 728–737 (2010).

    Google Scholar 

  37. Cacciapuoti, L. & Salomon, C. Atomic clock ensemble in space. J. Phys. Conf. Ser. 327, 012049 (2011).

    Google Scholar 

  38. Djerroud, K. et al. Coherent optical link through the turbulent atmosphere. Opt. Lett. 35, 1479–1481 (2010).

    ADS  Google Scholar 

  39. Robert, C., Conan, J.-M. & Wolf, P. Impact of turbulence on high-precision ground-satellite frequency transfer with two-way coherent optical links. Phys. Rev. A 93, 033860 (2016).

    ADS  Google Scholar 

  40. Deschênes, J.-D. et al. Synchronization of distant optical clocks at the femtosecond level. Phys. Rev. X 6, 021016 (2016).

    Google Scholar 

  41. Yamaguchi, A. et al. Direct comparison of distant optical lattice clocks at the 10−16 uncertainty. Appl. Phys. Express 4, 082203 (2011).

    ADS  Google Scholar 

  42. Predehl, K. et al. A 920-kilometer optical fiber link for frequency metrology at the 19th decimal place. Science 336, 441–444 (2012).

    ADS  Google Scholar 

  43. Lopez, O. et al. Ultra-stable long distance optical frequency distribution using the Internet fiber network. Opt. Express 20, 23518–23526 (2012).

    ADS  Google Scholar 

  44. Rost, M. et al. Time transfer through optical fibers over a distance of 73 km with an uncertainty below 100 ps. Metrologia 49, 772–778 (2012).

    ADS  Google Scholar 

  45. Śliwczyński, Ł., Krehlik, P., Czubla, A., Buczek, Ł. & Lipiński, M. Dissemination of time and RF frequency via a stabilized fibre optic link over a distance of 420 km. Metrologia 50, 133–145 (2013).

    ADS  Google Scholar 

  46. Calonico, D. et al. High-accuracy coherent optical frequency transfer over a doubled 642-km fiber link. Appl. Phys. B 117, 979–986 (2014).

    ADS  Google Scholar 

  47. Chiodo, N. et al. Cascaded optical fiber link using the Internet network for remote clocks comparison. Opt. Express 23, 33927–33937 (2015).

    ADS  Google Scholar 

  48. Raupach, S. M. F., Koczwara, A. & Grosche, G. Brillouin amplification supports 10−20 accuracy in optical frequency transfer over 1400 km of underground fibre. Phys. Rev. A 92, 021801(R) (2015).

    ADS  Google Scholar 

  49. Ma, L.-S., Jungner, P., Ye, J. & Hall, J. L. Delivering the same optical frequency at two places: accurate cancellation of phase noise introduced by optical fiber or other time-varying path. Opt. Lett. 19, 1777–1779 (1994).

    ADS  Google Scholar 

  50. Jiang, H. et al. Transfer of an optical frequency over an urban fiber link. J. Opt. Soc. Am. B 25, 2029–2035 (2008).

    ADS  Google Scholar 

  51. Williams, P. A., Swann, W. C. & Newbury, N. R. High-stability transfer of an optical frequency over long fiber-optic links. J. Opt. Soc. Am. B 25, 1284–1293 (2008).

    ADS  Google Scholar 

  52. Grosche, G. et al. Optical frequency transfer via 146 km fiber link with 10−19 relative accuracy. Opt. Lett. 34, 2270–2272 (2009).

    ADS  Google Scholar 

  53. Akatsuka, T. et al. 30-km-long optical fiber link at 1397 nm for frequency comparison between distant strontium optical lattice clocks. Jpn. J. Appl. Phys. 53, 032801 (2014).

    ADS  Google Scholar 

  54. Lopez, O. et al. Simultaneous remote transfer of accurate timing and optical frequency over a public fiber network. Appl. Phys. B 110, 3–6 (2013).

    ADS  Google Scholar 

  55. Lisdat, C. et al. A clock network for geodesy and fundamental science. Nat. Commun. 7, 12443 (2016).

    ADS  Google Scholar 

  56. Bercy, A. et al. Two-way optical frequency comparisons at 5 × 10−21 relative stability over 100-km telecommunication network fibers. Phys. Rev. A 90, 061802(R) (2014).

    ADS  Google Scholar 

  57. Vogt, S. et al. A transportable optical lattice clock. J. Phys. Conf. Ser. 723, 012020 (2016).

    Google Scholar 

  58. Poli, N. et al. A transportable strontium optical lattice clock. Appl. Phys. B 117, 1107–1116 (2014).

    ADS  Google Scholar 

  59. Bongs, K. et al. Development of a strontium optical lattice clock for the SOC mission on the ISS. C.R. Physique 16, 553–564 (2015).

    Google Scholar 

  60. Cao, J. et al. A transportable 40Ca+ single-ion clock with 7.7 × 10−17 systematic uncertainty. Preprint at http://lanl.arxiv.org/abs/1607.03731v1 (2016).

  61. Hong, F.-L. et al. Measuring the frequency of a Sr optical lattice clock using a 120 km coherent optical transfer. Opt. Lett. 34, 692–694 (2009).

    ADS  Google Scholar 

  62. Akatsuka, T. et al. 30-km-long optical fiber link at 1397 nm for frequency comparison between distant strontium optical lattice clocks. Jpn. J. Appl. Phys. 53, 032801 (2014).

    ADS  Google Scholar 

  63. Ye, J. et al. Delivery of high-stability optical and microwave frequency standards over an optical fiber network. J. Opt. Soc. Am. B 20, 1459–1467 (2003).

    ADS  Google Scholar 

  64. Williams, P. A., Swann, W. C. & Newbury, N. R. High-stability transfer of an optical frequency over long fiber-optic links. J. Opt. Soc. Am. B 25, 1284–1293 (2008).

    ADS  Google Scholar 

  65. Bureau International des Poids et Mesures http://www.bipm.org/en/bipm-services/timescales/time-ftp/Circular-T.html (last download July 2016).

  66. Bureau International des Poids et Mesures Recommendation 1 (CI-2006): Concerning Secondary Representations of the Second 249–250 (95th meeting, October 2006); http://www.bipm.org/en/committees/cipm/publications-cipm.html (last download 1 June 2016).

  67. Gill, P. When should we change the definition of the second? Phil. Trans. R. Soc. A 369, 4109–4130 (2012).

    ADS  Google Scholar 

  68. Riehle, F. Towards a redefinition of the second based on optical atomic clocks. C.R. Physique 16, 506–515 (2015).

    Google Scholar 

  69. Wolf, P. et al. Comparing high accuracy frequency standards via TAI. In 20th European Frequency Time Forum 476–485 (IEEE, 2006).

    Google Scholar 

  70. Le Targat, R. et al. Experimental realization of an optical second with strontium lattice clocks. Nat. Commun. 4, 2109 (2013).

    ADS  Google Scholar 

  71. Grebing, C. et al. Realization of a timescale with an accurate optical lattice clock. Optica 3, 563–569 (2016).

    ADS  Google Scholar 

  72. Ido, T., Hachisu, H., Nakagawa, F. & Hanado, Y. Rapid evaluation of time scale using an optical clock. J. Phys. Conf. Ser. 723, 012041 (2016).

    Google Scholar 

  73. Pavlis, N. K. & Weiss, M. A. The relativistic redshift with 3 × 10−17 uncertainty at NIST, Boulder, Colorado, USA. Metrologia 40, 66–73 (2003).

    ADS  Google Scholar 

  74. Grosche, G. Eavesdropping time and frequency: phase noise cancellation along a time-varying path, such as an optical fiber. Opt. Lett. 39, 2545–2548 (2014).

    ADS  Google Scholar 

  75. Ludlow, A. et al. Sr lattice clock at 1 × 10−16 fractional uncertainty by remote optical evaluation with a Ca clock. Science 319, 1805–1808 (2008).

    ADS  Google Scholar 

  76. Chanteau, B. et al. Mid-infrared laser phase-locking to a remote near-infrared frequency reference for high-precision molecular spectroscopy. New J. Phys. 15, 073003 (2013).

    ADS  Google Scholar 

  77. Lopez, O. et al. Frequency and time transfer for metrology and beyond using telecommunication network fibres. C.R. Physique 16, 531–539 (2015).

    Google Scholar 

  78. Calonico, D. et al. High-accuracy coherent optical frequency transfer over a doubled 642-km fiber link. Appl. Phys. B 117, 979–986 (2014).

    ADS  Google Scholar 

  79. Friebe, J. et al. Remote frequency measurement of the 1S0 -3P1 transition in laser-cooled 24Mg. New J. Phys. 13, 125010 (2011).

    ADS  Google Scholar 

  80. Matveev, A. et al. Precision measurement of the hydrogen 1S-2S frequency via a 920-km fiber link. Phys. Rev. Lett. 110, 230801 (2013).

    ADS  Google Scholar 

  81. Chou, C. W., Hume, D. B., Rosenband, T. & Wineland, D. J. Optical clocks and relativity. Science 329, 1630–1633 (2010).

    ADS  Google Scholar 

  82. Soffel, M. et al. The IAU 2000 resolutions for astrometry, celestial mechanics, and metrology in the relativistic framework: explanatory supplement. Astron. J. 126, 2687–2706 (2003).

    ADS  Google Scholar 

  83. Takano, T. et al. Geopotential measurements with synchronously linked optical lattice clocks. Nat. Photon. 10, 662–666 (2016).

    ADS  Google Scholar 

  84. King, M. A. et al. Lower satellite-gravimetry estimates of Antarctic sea-level contribution. Nature 491, 586–589 (2012).

    ADS  Google Scholar 

  85. Pail, R. et al. GOCE-only gravity field model derived from 8 months of GOCE data. In Proc. 4th Int. GOCE User Workshop SP-696 (ed. Ouwehand, L.) 17–23 (European Space Agency, 2011).

    Google Scholar 

  86. Sheard, B. et al. Intersatellite laser ranging instrument for the GRACE Follow-on mission. J. Geod. 86, 1083–1095 (2012).

    ADS  Google Scholar 

  87. Filippi, G. et al. The ALMA high speed optical communication link is here. An essential component for reliable present and future operations; https://www.eso.org/sci/libraries/SPIE2016/9913-83.pdf

  88. Doeleman, S. et al. Adapting a cryogenic sapphire oscillator for very long baseline interferometry. Publ. Astron. Soc. Pacif. 123, 582–595 (2011).

    ADS  Google Scholar 

  89. Clivati, C. et al. A coherent fiber link for very large baseline interferometry. IEEE Trans. Ultrasonics Ferroelectrics Frequency Control 62, 1907–1912 (2015).

    Google Scholar 

  90. ESA's Deep Space Tracking Network (European Space Agency); http://download.esa.int/esoc/estrack/esa_estrack_brochure_2015_EN.pdf

  91. Schiller, S. Feasibility of giant fiber-optic gyroscopes. Phys. Rev. A 87, 033823 (2013).

    ADS  Google Scholar 

  92. Clivati, C. et al. Large-area fiber-optic gyroscopes on a multiplexed fiber network. Opt. Lett. 38, 1092–1094 (2013).

    ADS  Google Scholar 

  93. Derevianko, A. & Pospelov, M. Hunting for topological dark matter with atomic clocks. Nat. Phys. 10, 933–936 (2014).

    Google Scholar 

  94. Stadnik, Y. V. & Flambaum, V. V. Enhanced effects of variation of the fundamental constants in laser interferometers and application to dark matter detection. Phys. Rev. A 93, 063630 (2016).

    ADS  Google Scholar 

  95. Matei, D. G. et al. A second generation of low thermal noise cryogenic silicon resonators. J. Phys. Conf. Ser. 723, 012031 (2016).

    Google Scholar 

  96. Abbott, B. P. et al. (LIGO Scientific Collaboration and Virgo Collaboration) Observation of gravitational waves from a binary black hole merger. Phys. Rev. Lett. 116, 061102 (2016).

    ADS  MathSciNet  Google Scholar 

  97. Pustelny, S. et al. The Global Network of Optical Magnetometers for Exotic Physics (GNOME): a novel scheme to search for physics beyond the standard model. Ann. Phys. 525, 659–670 (2013).

    Google Scholar 

  98. Kómár, P. et al. A quantum network of clocks. Nat. Phys. 10, 582–587 (2014).

    Google Scholar 

  99. Polzik, E. & Ye, J. Entanglement and spin squeezing in a network of distant optical lattice clocks. Phys. Rev. A 93, 021404(R) (2016).

    ADS  Google Scholar 

  100. Kolkowitz, S. et al. Gravitational wave detection with optical lattice atomic clocks. Preprint at http://lanl.arxiv.org/abs/1606.01859v2 (2016).

  101. Ma, L.-S. et al. International comparisons of femtosecond laser frequency combs. IEEE Trans. Instrum. Meas. 54, 746–749 (2005).

    Google Scholar 

  102. Rosenband, T. et al. Frequency ratio of Al+ and Hg+ single-ion optical clocks; metrology at the 17th decimal place. Science 319, 1808–1812 (2008).

    ADS  Google Scholar 

  103. Yamanaka, K., Ohmae, N., Ushijima, I., Takamoto, M. & Katori, H. Frequency ratio of 199Hg and 87Sr optical lattice clocks beyond the SI limit. Phys. Rev. Lett. 114, 230801 (2015).

    ADS  Google Scholar 

  104. Nemitz, N. et al. Frequency ratio of Yb and Sr clocks with 5 × 10−17 uncertainty at 150 s averaging time. Nat. Photon. 10, 258–261 (2016).

    ADS  Google Scholar 

  105. Sullivan, D., Allan, D., Howe, D. & Walls, F. (eds) NIST Technical Note 1337: Characterization of Clocks and Oscillators (NIST, US Department of Commerce, National Institute of Standards and Technology, 1990); http://tf.boulder.nist.gov/general/pdf/868.pdf

    Google Scholar 

  106. Rubiola, E. On the measurement of frequency and of its sample variance with high-resolution counters. Rev. Sci. Instrum. 76, 054703 (2005).

    ADS  Google Scholar 

  107. Itano, W. M. et al. Quantum projection noise: population fluctuations in two-level systems. Phys. Rev. A 47, 3554–3570 (1993).

    ADS  Google Scholar 

Download references

Acknowledgements

The author thanks H. Schnatz, N. Huntemann, D. Piester, G. Grosche and U. Sterr for their valuable comments and suggestions.

Author information

Authors and Affiliations

  1. Physikalisch-Technische Bundesanstalt, Bundesallee 100, Braunschweig, 38116, Germany

    Fritz Riehle

Authors
  1. Fritz Riehle
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Fritz Riehle.

Ethics declarations

Competing interests

The author declares no competing financial interests.

Rights and permissions

Reprints and Permissions

About this article

Verify currency and authenticity via CrossMark

Cite this article

Riehle, F. Optical clock networks. Nature Photon 11, 25–31 (2017). https://doi.org/10.1038/nphoton.2016.235

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/nphoton.2016.235

This article is cited by

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing