Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

Direct measurement of Kramers turnover with a levitated nanoparticle

Nature Nanotechnology volume 12, pages 1130–1133 (2017)Cite this article

Subjects

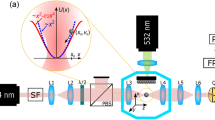
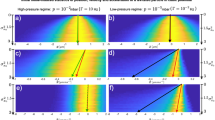
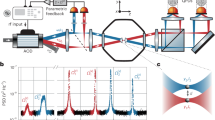
Abstract

Understanding the thermally activated escape from a metastable state is at the heart of important phenomena such as the folding dynamics of proteins1,2, the kinetics of chemical reactions3 or the stability of mechanical systems4. In 1940, Kramers calculated escape rates both in the high damping and low damping regimes, and suggested that the rate must have a maximum for intermediate damping5. This phenomenon, today known as the Kramers turnover, has triggered important theoretical and numerical studies6. However, as yet, there is no direct and quantitative experimental verification of this turnover. Using a nanoparticle trapped in a bistable optical potential, we experimentally measure the nanoparticle's transition rates for variable damping and directly resolve the Kramers turnover. Our measurements are in agreement with an analytical model that is free of adjustable parameters. The levitated nanoparticle presented here is a versatile experimental platform for studying and simulating a wide range of stochastic processes and testing theoretical models and predictions.

This is a preview of subscription content, access via your institution

Relevant articles

Open Access articles citing this article.

Access options

Rent or buy this article

Get just this article for as long as you need it

$39.95

Learn more

Prices may be subject to local taxes which are calculated during checkout

Figure 1: Double-trap set-up.
Figure 2: Transition kinetics.
Figure 3: Experimentally measured jumping rate R as a function of gas pressure Pgas (black dots) compared with the analytical model of equation (3).

References

  1. Best, R. & Hummer, G. Diffusive model of protein folding dynamics with Kramers turnover in rate. Phys. Rev. Lett. 96, 228104 (2006).

    Article  Google Scholar 

  2. Chung, H. S., Piana-Agostinetti, S., Shaw, D. E. & Eaton, W. A. Structural origin of slow diffusion in protein folding. Science 349, 1504–1510 (2015).

    Article  CAS  Google Scholar 

  3. Garcìa-Müller, P. L., Borondo, F., Hernandez, R. & Benito, R. M. Solvent-induced acceleration of the rate of activation of a molecular reaction. Phys. Rev. Lett. 101, 178302 (2008).

    Article  Google Scholar 

  4. Badzey, R. L. & Mohanty, P. Coherent signal amplification in bistable nanomechanical oscillators by stochastic resonance. Nature 437, 995–998 (2005).

    Article  CAS  Google Scholar 

  5. Kramers, H. Brownian motion in a field of force and the diffusion model of chemical reactions. Physica 7, 284–304 (1940).

    Article  CAS  Google Scholar 

  6. Hänggi, P., Talkner, P. & Borkovec, M. Reaction-rate theory: fifty years after Kramers. Rev. Mod. Phys. 62, 251–341 (1990).

    Article  Google Scholar 

  7. Silvestrini, P., Pagano, S., Cristiano, R., Liengme, O. & Gray, K. E. Effect of dissipation on thermal activation in an underdamped Josephson junction: first evidence of a transition between different damping regimes. Phys. Rev. Lett. 60, 844–847 (1988).

    Article  CAS  Google Scholar 

  8. Turlot, E. et al. Escape oscillations of a Josephson junction switching out of the zero-voltage state. Phys. Rev. Lett. 62, 1788–1791 (1989).

    Article  CAS  Google Scholar 

  9. Schroeder, J., Troe, J. & Vöhringer, P. Photoisomerization of trans-stilbene in compressed solvents: Kramers-turnover and solvent induced barrier shift. Z. Phys. Chem. 188, 287–306 (1995).

    Article  CAS  Google Scholar 

  10. McCann, L. I., Dykman, M. & Golding, B. Thermally activated transitions in a bistable three-dimensional optical trap. Nature 402, 785–787 (1999).

    Article  CAS  Google Scholar 

  11. Li, T., Kheifets, S., Medellin, D. & Raizen, M. G. Measurement of the instantaneous velocity of a Brownian particle. Science 328, 1673–1675 (2010).

    Article  CAS  Google Scholar 

  12. Gieseler, J., Deutsch, B., Quidant, R. & Novotny, L. Subkelvin parametric feedback cooling of a laser-trapped nanoparticle. Phys. Rev. Lett. 109, 103603 (2012).

    Article  Google Scholar 

  13. Gieseler, J., Quidant, R., Dellago, C. & Novotny, L. Dynamic relaxation of a levitated nanoparticle from a non-equilibrium steady state. Nat. Nanotech. 9, 358–364 (2014).

    Article  CAS  Google Scholar 

  14. Millen, J., Deesuwan, T., Barker, P. & Anders, J. Nanoscale temperature measurements using non-equilibrium Brownian dynamics of a levitated nanosphere. Nat. Nanotech. 9, 425–429 (2014).

    Article  CAS  Google Scholar 

  15. Chandler, D. Statistical mechanics of isomerization dynamics in liquids and the transition state approximation. J. Chem. Phys. 68, 2959–2970 (1978).

    Article  CAS  Google Scholar 

  16. Dellago, C. & Bolhuis, P. G. in Advanced Computer Simulation Approaches for Soft Matter Sciences III (eds Holm, P. C. & Kremer, P. K.) 167–233 (Springer, 2009).

  17. Mel'nikov, V. I. The Kramers problem: fifty years of development. Phys. Rep. 209, 1–71 (1991).

    Article  Google Scholar 

  18. Pollak, E. & Ankerhold, J. Improvements to Kramers turnover theory. J. Chem. Phys. 138, 164116 (2013).

    Article  Google Scholar 

  19. Hershkovitz, E. & Pollak, E. Multidimensional generalization of the Pollak–Grabert–Haenggi turnover theory for activated rate processes. J. Chem. Phys. 106, 7678–7699 (1997).

    Article  CAS  Google Scholar 

  20. Han, S., Lapointe, J. & Lukens, J. E. Thermal activation in a two-dimensional potential. Phys. Rev. Lett. 63, 1712–1715 (1989).

    Article  CAS  Google Scholar 

  21. Bowman, R. W. & Padgett, M. J. Optical trapping and binding. Rep. Prog. Phys. 76, 026401 (2013).

    Article  Google Scholar 

  22. Dykman, M. I. & Ryvkine, D. Activated escape of periodically modulated systems. Phys. Rev. Lett. 94, 070602 (2005).

    Article  CAS  Google Scholar 

  23. Gammaitoni, L., Hänggi, P., Jung, P. & Marchesoni, F. Stochastic resonance. Rev. Mod. Phys. 70, 223–287 (1998).

    Article  CAS  Google Scholar 

  24. Ricci, F. et al. Optically levitated nanoparticle as a model system for stochastic bistable dynamics. Nature Commun. 8, 15141 (2017).

    Article  CAS  Google Scholar 

  25. Kiesel, N. et al. Cavity cooling of an optically levitated submicron particle. Proc. Natl Acad. Sci. USA 110, 14180–14185 (2013).

    Article  CAS  Google Scholar 

  26. Fonseca, P. Z. G., Aranas, E. B., Millen, J., Monteiro, T. S. & Barker, P. F. Nonlinear dynamics and strong cavity cooling of levitated nanoparticles. Phys. Rev. Lett. 117, 173602 (2016).

    Article  CAS  Google Scholar 

  27. Jain, V. et al. Direct measurement of photon recoil from a levitated nanoparticle. Phys. Rev. Lett. 116, 243601 (2016).

    Article  Google Scholar 

  28. Guantes, R., Vega, J. L., Miret-Artes, S. & Pollak, E. Kramers turnover theory for diffusion of Na atoms on a Cu(001) surface measured by He scattering. J. Chem. Phys. 119, 2780–2791 (2003).

    Article  CAS  Google Scholar 

  29. Toyabe, S., Sagawa, T., Ueda, M., Muneyuki, E. & Sano, M. Experimental demonstration of information-to-energy conversion and validation of the generalized Jarzynski equality. Nat. Phys. 6, 988–992 (2010).

    Article  CAS  Google Scholar 

  30. Bérut, A. et al. Experimental verification of Landauer's principle linking information and thermodynamics. Nature 483, 187–189 (2012).

    Article  Google Scholar 

  31. Dechant, A., Kiesel, N. & Lutz, E. All-optical nanomechanical heat engine. Phys. Rev. Lett. 114, 183602 (2015).

    Article  Google Scholar 

Download references

Acknowledgements

This research was supported by the Swiss National Science Foundation (no. 200021L_169319) in cooperation with the Austrian Science Fund (no. I 3163), ERC-QMES (no. 338763), CoG ERC-QnanoMECA (no. 64790), Fundació Privada CELLEX and the severo Ochoa programme. L.R. acknowledges support from an ETH – Marie Curie Cofund Fellowship. The authors thank M. Frimmer, V. Jain, E. Hebestreit, C. Moritz, P. Mestres, E. Pollak and P. Bharadwaj for discussions and experimental support.

Author information

Author notes

  1. Loïc Rondin

    Present address: Laboratoire Aimé Cotton, CNRS, Université Paris-Sud, ENS Cachan, Université Paris-Saclay, 91405, Orsay Cedex, France

  2. Jan Gieseler

    Present address: Physics Department, Harvard University, Cambridge, Massachusetts, 02318, USA

Authors and Affiliations

  1. ETH Zürich, Photonics Laboratory, Zürich, 8093, Switzerland

    Loïc Rondin, Jan Gieseler & Lukas Novotny

  2. ICFO-Institut de Ciencies Fotoniques, The Barcelona Institute of Science and Technology, Castelldefels (Barcelona), 08860, Spain

    Francesco Ricci & Romain Quidant

  3. ICREA-Institució Catalana de Recerca i Estudis Avançats, Barcelona, 08010, Spain

    Romain Quidant

  4. Faculty of Physics, University of Vienna, Boltzmanngasse 5, Wien, 1090, Austria

    Christoph Dellago

  5. Erwin Schrödinger International Institute for Mathematics and Physics, University of Vienna, Boltzmanngasse 9, Wien, 1090, Austria

    Christoph Dellago

Authors
  1. Loïc Rondin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jan Gieseler
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Francesco Ricci
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Romain Quidant
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Christoph Dellago
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Lukas Novotny
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

L.R. and L.N. designed and conceived the experiment. L.R. performed the experiment and analysed the data, with input from J.G., C.D. and L.N. All authors discussed the results and contributed to writing the manuscript.

Corresponding author

Correspondence to Lukas Novotny.

Ethics declarations

Competing interests

The authors declare no competing financial interests.

Supplementary information

Supplementary information

Supplementary information (PDF 691 kb)

Rights and permissions

Reprints and Permissions

About this article

Verify currency and authenticity via CrossMark

Cite this article

Rondin, L., Gieseler, J., Ricci, F. et al. Direct measurement of Kramers turnover with a levitated nanoparticle. Nature Nanotech 12, 1130–1133 (2017). https://doi.org/10.1038/nnano.2017.198

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/nnano.2017.198

This article is cited by

Search

Advanced search

Quick links

Find nanotechnology articles, nanomaterial data and patents all in one place. Visit Nano by Nature Research