Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

  • Article
  • Published:

Probabilistic density maps to study global endomembrane organization

Nature Methods volume 7, pages 560–566 (2010)Cite this article

Subjects

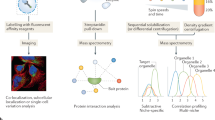
Abstract

We developed a computational imaging approach that describes the three-dimensional spatial organization of endomembranes from micromanipulation-normalized mammalian cells with probabilistic density maps. Applied to several well-known marker proteins, this approach revealed the average steady-state organization of early endosomes, multivesicular bodies or lysosomes, endoplasmic reticulum exit sites, the Golgi apparatus and Golgi-derived transport carriers in crossbow-shaped cells. The steady-state organization of each tested endomembranous population was well-defined, unique and in some cases depended on the cellular adhesion geometry. Density maps of all endomembrane populations became stable when pooling several tens of cells only and were reproducible in independent experiments, allowing construction of a standardized cell model. We detected subtle changes in steady-state organization induced by disruption of the cellular cytoskeleton, with statistical significance observed for just 20 cells. Thus, combining micropatterning with construction of endomembrane density maps allows the systematic study of intracellular trafficking determinants.

This is a preview of subscription content, access via your institution

Access options

Buy this article

  • Purchase on Springer Link
  • Instant access to full article PDF
Buy now

Prices may be subject to local taxes which are calculated during checkout

Figure 1: Analysis of CD63-positive endomembranes from 35 cells on crossbow-shaped patterns.
Figure 2: Analysis of Rab5-, Sec13- and Rab6-positive endomembranes on crossbow-shaped patterns.
Figure 3: Comparison of 'characteristic territories' and 'characteristic volumes' of endomembranes on crossbow-shaped patterns.
Figure 4: Analysis of CD63- and Rab6-positive endomembranes in circular cells.
Figure 5: Changes of Rab6-positive endomembranes upon cytoskeletal disruption.

Similar content being viewed by others

References

  1. Bornens, M. Organelle positioning and cell polarity. Nat. Rev. Mol. Cell Biol. 9, 874–886 (2008).

    Article  CAS  Google Scholar 

  2. Caviston, J.P. & Holzbaur, E.L. Microtubule motors at the intersection of trafficking and transport. Trends Cell Biol. 16, 530–537 (2006).

    Article  CAS  Google Scholar 

  3. Lanzetti, L. Actin in membrane trafficking. Curr. Opin. Cell Biol. 19, 453–458 (2007).

    Article  CAS  Google Scholar 

  4. Ross, J.L., Ali, M.Y. & Warshaw, D.M. Cargo transport: molecular motors navigate a complex cytoskeleton. Curr. Opin. Cell Biol. 20, 41–47 (2008).

    Article  CAS  Google Scholar 

  5. Insall, R.H. & Machesky, L.M. Actin dynamics at the leading edge: from simple machinery to complex networks. Dev. Cell 17, 310–322 (2009).

    Article  CAS  Google Scholar 

  6. Schmoranzer, J. et al. Par3 and dynein associate to regulate local microtubule dynamics and centrosome orientation during migration. Curr. Biol. 19, 1065–1074 (2009).

    Article  CAS  Google Scholar 

  7. Egea, G., Lazaro-Dieguez, F. & Vilella, M. Actin dynamics at the Golgi complex in mammalian cells. Curr. Opin. Cell Biol. 18, 168–178 (2006).

    Article  CAS  Google Scholar 

  8. Rivero, S., Cardenas, J., Bornens, M. & Rios, R.M. Microtubule nucleation at the cis-side of the Golgi apparatus requires AKAP450 and GM130. EMBO J. 28, 1016–1028 (2009).

    Article  CAS  Google Scholar 

  9. Semenova, I. et al. Actin dynamics is essential for myosin-based transport of membrane organelles. Curr. Biol. 18, 1581–1586 (2008).

    Article  CAS  Google Scholar 

  10. Taunton, J. Actin filament nucleation by endosomes, lysosomes and secretory vesicles. Curr. Opin. Cell Biol. 13, 85–91 (2001).

    Article  CAS  Google Scholar 

  11. Sachs, K., Perez, O., Pe'er, D., Lauffenburger, D.A. & Nolan, G.P. Causal protein-signaling networks derived from multiparameter single-cell data. Science 308, 523–529 (2005).

    Article  CAS  Google Scholar 

  12. Sigal, A. et al. Variability and memory of protein levels in human cells. Nature 444, 643–646 (2006).

    Article  CAS  Google Scholar 

  13. Snijder, B. et al. Population context determines cell-to-cell variability in endocytosis and virus infection. Nature 461, 520–523 (2009).

    Article  CAS  Google Scholar 

  14. Liu, W.F. & Chen, C.S. Cellular and multicellular form and function. Adv. Drug Deliv. Rev. 59, 1319–1328 (2007).

    Article  CAS  Google Scholar 

  15. Thery, M., Pepin, A., Dressaire, E., Chen, Y. & Bornens, M. Cell distribution of stress fibres in response to the geometry of the adhesive environment. Cell Motil. Cytoskeleton 63, 341–355 (2006).

    Article  CAS  Google Scholar 

  16. Thery, M. et al. Anisotropy of cell adhesive microenvironment governs cell internal organization and orientation of polarity. Proc. Natl. Acad. Sci. USA 103, 19771–19776 (2006).

    Article  CAS  Google Scholar 

  17. Racine, V. et al. Visualization and quantification of vesicle trafficking on a three-dimensional cytoskeleton network in living cells. J. Microsc. 225, 214–228 (2007).

    Article  Google Scholar 

  18. Bowman, A.W. & Foster, P. Density based exploration of bivariate data. Stat. Comput. 3, 171–177 (1993).

    Article  Google Scholar 

  19. Hyndman, R. Computing and graphing highest density regions. Am. Stat. 50, 120–126 (1996).

    Google Scholar 

  20. Pols, M.S. & Klumperman, J. Trafficking and function of the tetraspanin CD63. Exp. Cell Res. 315, 1584–1592 (2009).

    Article  CAS  Google Scholar 

  21. Chavrier, P., Parton, R.G., Hauri, H.P., Simons, K. & Zerial, M. Localization of low molecular weight GTP binding proteins to exocytic and endocytic compartments. Cell 62, 317–329 (1990).

    Article  CAS  Google Scholar 

  22. Tang, B.L. et al. The mammalian homolog of yeast Sec13p is enriched in the intermediate compartment and is essential for protein transport from the endoplasmic reticulum to the Golgi apparatus. Mol. Cell. Biol. 17, 256–266 (1997).

    Article  CAS  Google Scholar 

  23. Antony, C. et al. The small GTP-binding protein rab6p is distributed from medial Golgi to the trans-Golgi network as determined by a confocal microscopic approach. J. Cell Sci. 103, 785–796 (1992).

    CAS  PubMed  Google Scholar 

  24. Grigoriev, I. et al. Rab6 regulates transport and targeting of exocytotic carriers. Dev. Cell 13, 305–314 (2007).

    Article  CAS  Google Scholar 

  25. White, J. et al. Rab6 coordinates a novel Golgi to ER retrograde transport pathway in live cells. J. Cell Biol. 147, 743–760 (1999).

    Article  CAS  Google Scholar 

  26. Gretton, A., Borgwardt, K.M., Rasch, M.J., Schoelkopf, B. & Smola, A. A kernel method for the two-sample problem. Advances in Neural Information Processing Systems 19: Proceedings of the 2006 Conference 513–520 (MIT Press, Cambridge, Masssachusetts, USA, 2007).

  27. Wodarz, A. & Näthke, I. Cell polarity in development and cancer. Nat. Cell Biol. 9, 1016–1024 (2007).

    Article  CAS  Google Scholar 

  28. Rodriguez Boulan, E. & Sabatini, D.D. Asymmetric budding of viruses in epithelial monlayers: a model system for study of epithelial polarity. Proc. Natl. Acad. Sci. USA 75, 5071–5075 (1978).

    Article  CAS  Google Scholar 

  29. Snider, J. et al. Intracellular actin-based transport: how far you go depends on how often you switch. Proc. Natl. Acad. Sci. USA 101, 13204–13209 (2004).

    Article  CAS  Google Scholar 

  30. Azioune, A., Storch, M., Bornens, M., Théry, M. & Piel, M. Simple and rapid process for single cell micro-patterning. Lab Chip 9, 1640–1642 (2009).

    Article  CAS  Google Scholar 

  31. Sibarita, J.B. Deconvolution microscopy. Adv. Biochem. Eng. Biotechnol. 95, 201–243 (2005).

    PubMed  Google Scholar 

  32. Simonoff, J.S. Smoothing Methods for Statistics. (Springer, New York, 1996).

    Book  Google Scholar 

  33. Duong, T. & Hazelton, M.L. Plug-in bandwidth matrices for bivariate kernel density estimations. J. Nonparametr. Stat. 17, 17–30 (2003).

    Article  Google Scholar 

Download references

Acknowledgements

We acknowledge L. Sengmanivong of the Nikon Imaging Centre at Institut Curie–Centre National de la Recherche Scientifique and V. Fraisier of the Plate-forme Imagerie Cellulaire et Tissulaire–Infrastructures en Biologie Santé et Agronomie Imaging Facility for their extensive help with microscopes and in particular their help using the deconvolution service of the facility. We thank J.-B. Sibarita for advice on image analysis including use of the multidimensional image analysis program and fruitful discussion during early phases of the project; I. Brito for statistical advice; W. Hong (Institute of Molecular and Cell Biology, Singapore) for providing the Sec13 antibody; M. Piel, A. Azioune and J. Fink for help with microprinting; and G. Egea, S. Miserey, A. Echard and J. Enninga for critical reading of the manuscript. K.S. received funding from the Fondation pour la Recherche Médicale en France and Association pour la Recherche sur le Cancer. This project was supported by grants from the Centre National de la Recherche Scientifique and Institut Curie.

Author information

Authors and Affiliations

  1. Unité Mixte de Recherche 144, Centre National de la Recherche Scientifique, Institut Curie, Laboratory Molecular Mechanisms of Intracellular Transport, Paris, France

    Kristine Schauer, Tarn Duong, Sabine Bardin, Michel Bornens & Bruno Goud

  2. Institut Pasteur, Groupe Imagerie et Modélisation, Unité de Recherche Associée 2582, Centre National de la Recherche Scientifique, Paris, France

    Tarn Duong

  3. Mines ParisTech, Centre for Computational Biology, Institut Curie, Institut National de la Santé Et de la Recherche Médicale U900, Paris, France

    Kevin Bleakley

Authors
  1. Kristine Schauer
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Tarn Duong
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Kevin Bleakley
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Sabine Bardin
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Michel Bornens
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Bruno Goud
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

K.S. and B.G. designed the research, K.S. performed the experiments and analysis and wrote the manuscript, T.D. developed the density calculation, K.B. developed the statistical analysis and edited the manuscript, S.B. adjusted patterning techniques and M.B. contributed to the conception of the work.

Corresponding authors

Correspondence to Kristine Schauer or Bruno Goud.

Ethics declarations

Competing interests

The authors declare no competing financial interests.

Supplementary information

Supplementary Text and Figures

Supplementary Figures 1–6, Supplementary Table 1 and Supplementary Notes 1–2 (PDF 2412 kb)

Supplementary Video 1

Maximum intensity projection of the deconvolved fluorescence of GFP-Rab6-positive cells (n = 82) under control conditions. (AVI 2507 kb)

Supplementary Video 2

Maximum intensity projection of the deconvolved fluorescence of GFP-Rab6-positive cells (n = 47) after nocodazole treatment. (AVI 1383 kb)

Supplementary Video 3

Maximum intensity projection of the deconvolved fluorescence of GFP-Rab6-positive cells (n = 50) after cytochalasin D treatment. (AVI 1667 kb)

Rights and permissions

Reprints and permissions

About this article

Cite this article

Schauer, K., Duong, T., Bleakley, K. et al. Probabilistic density maps to study global endomembrane organization. Nat Methods 7, 560–566 (2010). https://doi.org/10.1038/nmeth.1462

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/nmeth.1462

This article is cited by

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing