Abstract
Defects usually play an important role in tailoring various properties of two-dimensional materials. Defects in two-dimensional monolayer molybdenum disulphide may be responsible for large variation of electric and optical properties. Here we present a comprehensive joint experiment–theory investigation of point defects in monolayer molybdenum disulphide prepared by mechanical exfoliation, physical and chemical vapour deposition. Defect species are systematically identified and their concentrations determined by aberration-corrected scanning transmission electron microscopy, and also studied by ab-initio calculation. Defect density up to 3.5 × 1013 cm−2 is found and the dominant category of defects changes from sulphur vacancy in mechanical exfoliation and chemical vapour deposition samples to molybdenum antisite in physical vapour deposition samples. Influence of defects on electronic structure and charge-carrier mobility are predicted by calculation and observed by electric transport measurement. In light of these results, the growth of ultra-high-quality monolayer molybdenum disulphide appears a primary task for the community pursuing high-performance electronic devices.
Similar content being viewed by others
Introduction
The success of graphene1,2 offers a paradigm for the exploration of novel low-dimensional physical phenomena3 and physical properties in two-dimensional (2D) crystal systems4,5. However, its intrinsic shortcoming lies in its zero bandgap, which strongly hinders its application in logical electronic devices. Among the post-graphene development, 2D semiconducting molybdenum disulphide and other transition metal dichalcogenides6,7 have recently appeared on the horizon of materials science and condensed matter physics. Monolayer MoS2 is a direct-gap semiconductor that exhibits a substantially improved efficiency in its photoluminescence8,9. Valley polarization occurs due to significant spin–orbit coupling and leads to optical circular dichroism10,11,12 in the monolayer system. The electronic transport of MoS2-based field effect transistors (FETs) shows steep sub-threshold swing of 70 mV dec−1 (refs 13, 14, 15) and a high on/off ratio up to 108 (ref. 16). A metal-insulator transition happens when carrier densities reaches 1013 cm−2, which also increases the effective mobility17,18,19. Owing to its unique optical and electric properties, MoS2 is believed to be a promising candidate as a building block for future applications in nanoelectronics and optoelectronics6.
Wafer-scale production of atomically thin layers is paramount for MoS2 to be used as a candidate channel material for electronic and optoelectronic devices13,14,15,16,17,18,19. Among the currently available preparation methods, mechanical exfoliation (ME) is deemed less efficient for these large-scale applications, even though it produces the highest-quality samples exhibiting the best electric performance. Physical and chemical vapour deposition17,20,21,22,23,24,25 methods are more compatible for the scalable growth of high-quality samples. However, the experimentally attainable mobility is still one or two order-of-magnitude lower than the theoretical value of 410 cm2 V−1 s−1 (refs 13, 18, 26, 27, 28, 29). For back-gated m-MoS2 FET devices, the highest mobility reported so far reaches 81 cm2 V−1 s−1 for ME sample28, 45 cm2 V−1 s−1 for chemical vapour deposition (CVD)17 and<1 cm2 V−1 s−1 for physical vapour deposition (PVD)25. The major scattering mechanism for the mobility deterioration has been recently suggested as due to the presence of plentiful localized band tail states30 caused by short-range disordered structural defects (such as vacancies28,31 and grain boundaries32), and Coulomb traps33,34. However, the roles played by various defects in electric and optoelectronic properties are yet to be explicitly understood. There have been few investigations reported on point defects, mostly vacancies, and grain boundaries in m-MoS2 (refs 28, 30, 31, 32, 35, 36). These studies are, however, often performed with samples made by preparatory methods, for example, ME or CVD, which essentially limits the scope of those studies.
Here we present a systematic investigation of the point defects in distinctly prepared m-MoS2 by combining atomically resolved annular dark-field scanning transmission electron microscopy (ADF-STEM) imaging, density functional theory (DFT) calculation and electric transport measurements. We observe, for the first time, that antisite defects with molybdenum replacing sulphur are dominant point defects in PVD-grown MoS2, while the sulphur vacancies are predominant in ME and CVD specimens. These experimental observations are further supported qualitatively by the growth mechanism and quantitatively by the defects’ formation energies calculations. The DFT calculations, in addition, predict the electronic structures and magnetic properties of m-MoS2 with antisite defects. We also discuss the influence of defects on the phonon-limited carrier mobility theoretically, and further examine them by electric transport in defective m-MoS2-based FETs. Our systematic investigation of point defects, especially antisites, will further deepen our understanding of this novel 2D atomically thin semiconductor and pave the way for the scalable electronic application of the family of atomically thin transition metal chalcogenides.
Results
Statistics of point defects
For the purpose of the analysis of defects and their concentration, we have chosen about ten samples prepared under the optimized fabrication condition (see the Methods section and Supplementary Note 1 for the details of sample synthesis) from each method (ME, PVD and CVD) and then transferred each sample onto at least two TEM grids independently for ADF-STEM characterizations. The crystalline quality and the choice of samples for statistical analysis are presented in Supplementary Figs 1–9. Figure 1 summarized the most significant results obtained from our analysis on these MoS2 samples. For the PVD specimen, antisite defects with one Mo atom replacing one or two S atoms (MoS or MoS2) are frequently observed, marked with red dashed circles shown in Fig. 1a, while the dominant defects for the ME and CVD samples are S vacancies with one (VS) or two (VS2) S atoms absent, as marked by green dashed circles in Fig. 1b. As the STEM’s Z-contrast mechanism37, that is, I~Z1.6–2.0 (I and Z are the image contrast and atomic number, respectively), predicts, Mo and S atoms can be unambiguously discriminated, with Mo (Z=44) showing bright contrast and two superposed S atoms showing dim contrast in the lattice of m-MoS2. Following a similar argument and quantitative image analysis, various defects, for example, MoS or VS where the lattice image presents abnormal intensity variation, can be clearly identified individually through direct imaging and their atomic structures further verified by ab-initio calculations (please refer to Supplementary Table 1 for details of each atomic defect).
(a) Antisite defects in PVD MoS2 monolayers. Scale bar, 1 nm. (b) Vacancies including VS and VS2 observed in ME monolayers, similar to that observed for CVD sample. Scale bar, 1 nm. (c,d) Histograms of various point defects in PVD, CVD and ME monolayers. Error estimates are given for the dominant defects (more details on the statistics can be found in Supplementary Fig. 6). ME data are in green, PVD data in red and CVD in blue.
We show the relative importance of each type of point defects in Fig. 1c,d. Figure 1c presents the total counts of different point defects based on over 70 atomically resolved ADF-STEM images for each type, that is, ME, PVD or CVD MoS2 samples. It is found that the dominant type of point defects in each sample highly depends on the specific sample preparation method. The VS vacancy is the predominant point defects in ME and CVD samples, with its concentration of about (1.2±0.4) × 1013 cm−2 (Supplementary Fig. 6), close to the results reported previously30. Atomic defects VMo (one Mo atom missing) and SMo (one S atom replacing Mo site) were also found, but with much lower concentrations as shown in Fig. 1c. In contrast, the histogram also shows that antisite defects MoS2 and MoS are dominant in PVD samples, with their concentrations higher than that of VS. The density of MoS2 and MoS reaches (2.8±0.3) × 1013 and 7.0 × 1012 cm−2, corresponding to an atomic percent of 0.8% and 0.21%, respectively (counted on the total number of all Mo and S atoms). Such a defect concentration is surprisingly high if the defects were regarded as impurity doping, which is usually only achieved in degenerate semiconductor38 (for instance 10−2~10−4). It is, therefore, of vital importance to understand how they modify the electronic properties of m-MoS2 as elucidated below.
Structural characterization of point defects
So far, there have been few reports concerning the structures of sulphur vacancies31,39 and their impacts on the electronic transport properties30,40 of m-MoS2; by contrast, there is still a lack of detailed knowledge on the antisite defects, which is at such an unexpected high doping level in PVD samples. Hence, we focus more on antisite defects. In Fig. 2a–e, we highlight all the images of the experimentally observed antisite defects in m-MoS2 (see also Supplementary Fig. 10), which can be grouped into two categories. One is the antisite defects with Mo atom(s) substituting S atom(s), including MoS, MoS2 and  (Fig. 2a–c). The other category is the antisite defects with S atom occupying the site of Mo, namely SMo and
(Fig. 2a–c). The other category is the antisite defects with S atom occupying the site of Mo, namely SMo and  (Fig. 2d,e). The experimental identification of these antisite defects can be further unambiguously supported by the quantitative image simulation based on DFT-predicted atomic structures of all antisite defects. The fully relaxed DFT-predicted atomic structures of antisite defects were shown in Fig. 2k–t, where the atomic displacement and structure deformation are explicitly observable, especially for antisites MoS2 and
(Fig. 2d,e). The experimental identification of these antisite defects can be further unambiguously supported by the quantitative image simulation based on DFT-predicted atomic structures of all antisite defects. The fully relaxed DFT-predicted atomic structures of antisite defects were shown in Fig. 2k–t, where the atomic displacement and structure deformation are explicitly observable, especially for antisites MoS2 and  . Associated side views of these relaxed structures are available in Fig. 2p–t. The ADF image simulations (Fig. 2f–j) based on the calculated structures fit quite well with the experimental images shown in Fig. 2a–e, respectively, especially for the off-centre feature observable in antisites MoS2 and
. Associated side views of these relaxed structures are available in Fig. 2p–t. The ADF image simulations (Fig. 2f–j) based on the calculated structures fit quite well with the experimental images shown in Fig. 2a–e, respectively, especially for the off-centre feature observable in antisites MoS2 and  , which can be tentatively attributed to Jahn–Teller distortions.
, which can be tentatively attributed to Jahn–Teller distortions.
(a–c) High-resolution STEM–ADF images of antisite MoS, MoS2 and  , respectively. The former two antisites (highlighted by the red dashed rectangle in k) are dominant in PVD-synthesized MoS2 single layers. Scale bar, 0.5 nm (d,e) Atomic structures of antisite defects SMo and
, respectively. The former two antisites (highlighted by the red dashed rectangle in k) are dominant in PVD-synthesized MoS2 single layers. Scale bar, 0.5 nm (d,e) Atomic structures of antisite defects SMo and  , respectively. (f–j) Simulated STEM images based on the theoretically relaxed structures of the corresponding point defects in (a–e), using simulation software QSTEM49. (k–t) Relaxed atomic model of all antisite defects in a–e through DFT calculation, with top and side views, respectively. Light blue, Mo atoms; gold, S atoms. For ease of comparison, we have presented the simulated ADF images before the atomistic schematics of the DFT calculated structures.
, respectively. (f–j) Simulated STEM images based on the theoretically relaxed structures of the corresponding point defects in (a–e), using simulation software QSTEM49. (k–t) Relaxed atomic model of all antisite defects in a–e through DFT calculation, with top and side views, respectively. Light blue, Mo atoms; gold, S atoms. For ease of comparison, we have presented the simulated ADF images before the atomistic schematics of the DFT calculated structures.
Energetics of predominant point defects in different m-MoS2
The distribution of different atomic defects in m-MoS2 certainly depends on their preparation process. A full exploration of the growth dynamics requires a comprehensive experiment–theory joint investigation, which is beyond the scope of the present work, although it is of fundamental interest. Here we provide a qualitative explanation, based on our DFT calculations, to reveal the microscopic physical mechanism of the preparation-process-dependent defect formation. The formation energy (ΔEForm) for all the point defects are calculated and summarized in Table 1, as consistent with a previous report35. Here, chemical potentials of elements Mo and S were employed to calculate the formation enthalpy (ΔHForm) of defects. To account for a range of different possible reservoirs (for example, bulk element or bulk MoS2), each enthalpy is given with a range, as listed in Table 1. Two widely used DFT codes (CASTEP41 and VASP (Vienna Ab-initio Simulation Package)42) are adopted to exploit the full range of functionality available and demonstrate the consistency of the calculated results in Table 1.
An ME sample is exfoliated from MoS2 natural mineral. After the MoS2 mineral was formed and/or extracted, either element S or Mo of MoS2 is prone to reach a solid–gas phase equilibrium. Owing to a higher saturated vapour pressure of S, the mineral-form MoS2 has to release more S than Mo atoms into the gas phase and thus S is prone to be deficient in MoS2. Reflecting this fact, vacancies VS and VS2 have the lowest ΔEForm of 2.12 eV and 4.14 eV, respectively, among all the defects. The formation energies of all the antisite defects are higher than 5 eV, indicating that the S-deficient mineral-form MoS2 favours the formation of S vacancies, leading to the observation of the most common defect of VS, followed by VS2, and almost no antisite defect in ME samples.
In a typical PVD process, MoS2 precursor is sublimated into the gas phase with clusters and atoms, carried by Ar gas (mixed with H2), and then condensed into a solid-phase MoS2. Sulphur has a larger saturated vapour pressure so that more S atoms in the gas phase will leave the preparation chamber, thus establishing a S-deficient and Mo-rich condition. These clusters and atoms are highly mobile and are thus prone to form an ordered structure of MoS2 in the lowest total energy. Considering n+1 Mo and 2n-1S atoms for example, they have two options, namely, forming (i) n MoS2 units with one MoS antisite or (ii) n+1 MoS2 units with three VS vacancies. The exact value of n does not affect the energetic difference between these two types of defects. We thus arbitrarily instantiate n as 107, namely 108 Mo and 213 S atoms in total. The total energy of the antisite option is −1,774.83 eV, while that for the vacancy case is −1,774.26 eV which is 0.57 eV less stable than the former. A similar relation also applies to antisite defect MoS2 with an energy gain of 0.92 eV. A sample with one antisite MoS or MoS2 shares the same number of Mo and S atoms with another sample that has three or four S vacancies, respectively. The formation energies of antisites MoS and MoS2 were, therefore, divided by three and four, respectively, to make these energies quantitatively comparable with a single S vacancy, as required by the comparison with Boltzmann distribution. We have renormalized ΔE′Form(MoS)=1.93 eV, ΔE′Form(MoS2)=1.89 eV, which give rise to a ratio of p(MoS2):p(MoS):p(VS)=10.5:6.7:1 at the growth temperature of 1,100 K. This ratio is comparable with the experimental probability density ratio of MoS2:MoS:VS=9:2.3:1 in PVD samples.
The CVD process is distinctly different from ME or PVD. Extra S vapour is supplied to replace O in the MoO3 precursor under an S-rich condition. We suspect that there are small amount of residual O atoms taking the position of S atoms in the resulting MoS2 sheets, due to the competition between Mo–O and Mo–S bonding in the reaction chamber. Our ab-initio calculation, not shown here, suggests that these O atoms are 1.99 eV less stable than corresponding 2S and usually tend to desorb into the gas phase leaving vacancies at the S sites, that is, S vacancies. It is argued that Mo atoms may jump into the S vacancies and form Mo antisites. Despite of the S-rich condition, even if Mo is rich in a certain local environment, Mo atoms may be firmly bonded with oxygen in the precursor, which strongly limits the diffusion of Mo, making the formation of Mo antisite from mobile Mo atom and S vacancy much less likely.
Electronic structures
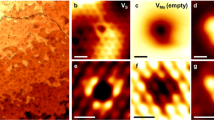
The electronic structure of point defects plays a crucial role in determining the electric properties of these defective m-MoS2. Vacancy VS and its effect on electronic structures have been recently reported30,35; we thus focus on the less-studied antisite defects (please refer to Supplementary Fig. 11 for our ADF imaging and DFT calculation of VS). Figure 3 shows the theoretically predicted band structures and projected density-of-states of two primary antisites MoS and MoS2. Our results give a bandgap of 1.73 eV for a defect-free m-MoS2 (Supplementary Fig. 12), close to the experimentally observed optical bandgap of 1.8 eV (ref. 8). Defect states with nearly flat band dispersion for MoS2 and MoS reside inside the band gap of a perfect m-MoS2 (Fig. 3a,d). These states mostly comprises the d orbitals of four Mo atoms around the defect. In addition, the orbital hybridization of Mo and S atoms results in extended wavefunctions involving the surrounding atoms, forming a ‘superatom’ with a radius of roughly 6 Å, as shown in Fig. 3b,c,f.
(a) Band structure and corresponding density of states (DOS) of antisite defect MoS2.The grey bands are from normal lattice sites, similar to conduction band and valence band of perfect monolayer, while the discrete red bands show the localized defects states. The DOS is projected onto the atoms around the defect (defect) and those in the middle plane of two adjacent defects (pure), respectively. The grey dash line indicates the position of the Fermi Level. (b,c) Real-space distribution of the wave functions of the two defect states below and above the Fermi energy. (d) The band structure and DOS of antisite MoS, with a similar colour scheme of a, but the two spin components are coloured in red (spin-up) and blue (spin-down), respectively. (e) Spin density of antisite MoS, defined as ρup−ρdown, charge densities ρup and ρdown are spin-resolved for spin-up and -down components, which are represented by yellow and blue isosurfaces, respectively. (f) Spin-resolved real-space distribution of the wave function of the two marked defect states (State 3) in d. The isosurface value in b,c,e,f is 0.001e Bhor−3.
Magnetic properties of m-MoS2 have not been reported yet, as it is believed to be a non-magnetic material. Nevertheless, we did find a local magnetic moment of 2 μB in antisite MoS, while the values for other defects, for example, VS, VS2 and MoS2, are smaller than 0.1 μB, and hence are negligible. The magnetic moment of 2 μB for a MoS antisite is not localized only on the central Mo atom, with the surrounding atoms contributing roughly 20% of the total moment probably due to the strong hybridization among these atoms in a ‘superatom’, as shown in the visualized total spin density (Fig. 3e). Detailed distribution of magnetic moment is available in Supplementary Fig. 13. The spin-resolved real-space distribution of a defect-induced state (state 3) marked in Fig. 3d was plotted in Fig. 3f to illustrate the origin of the magnetism. The occupied spin-up component (yellow isosurface) is mainly composed of the dxy and dx2–y2 orbitals of the antisite Mo atom, while the unoccupied spin-down component (cyan isosurface) is projected onto the dxy and dz2 orbitals of surrounding Mo atoms, consistent with the total spin charge density shown in Fig. 3e. More detailed discussion on the magnetic property of antisites are presented in Supplementary Fig. 14 and Supplementary Note 2.
Carrier mobility in defective samples
As there have been plentiful reports on the transport of ME MoS2-based FETs with electron mobility 1~81 cm2 V−1 s−1 (refs 15, 16, 18, 26, 28, 43, 44), we focus on the transport properties of CVD and PVD monolayers. Figure 4a–d presents the output and transfer characteristics of fabricated FETs based on PVD and CVD MoS2, respectively. Our transport measurements (in Fig. 4a–d) of defective MoS2-based FETs reveal that the PVD and CVD MoS2 has electron mobility 0.5 and 11 cm2 V−1 s−1, respectively. All these results are well comparable with the reported mobilities of m-MoS2 of 1~81 cm2 V−1 s−1 for ME15,16,18,26,28,43,44, 5~45 cm2 V−1 s−1 for CVD17,29 and the reported values of <1 cm2 V−1 s−1 for PVD m-MoS2 (ref. 25) respectively.
Theoretically, we focus on the effect of the defects on the phonon-limited carrier mobilities45,46,47. Table 2 lists the calculated effective masses, deformation potentials and estimated mobilities derived based on the predicted electron mobility of 410 cm2 V−1 s−1 in a perfect m-MoS2 (ref. 48). MoS2 samples are usually n-type, we thus primarily focus on the electron mobility. It is found that the phonon-limited mobility of electrons flowing in the intrinsic conduction band is, exceptionally, nearly unaffected by the presence of vacancies (VS or VS2), but reduced by three times in the samples with antisite defects, whereas the phonon-limited mobility of holes carried by the intrinsic valence band is more sensitive to these defects and reduces roughly three times for vacancy and more than four times for antisite. Both vacancy and antisite are strong electron-scattering centres that the mobility derived from the defect states (d–e and d–h) for either electron or hole is fairly small, mostly smaller than 1 and 10 cm2 V−1 s−1, respectively. The defect states strongly affect, but not overwhelmingly dominate, the overall carrier mobility of the samples, owing to the relative low density of defects and the strongly localized defect states. On the other hand, in a real FET device the measured mobility can be affected by the contact resistance45 or the carrier density19. Furthermore, the trapped charges would act as a scattering centre15,40. A hopping transport caused by localized disorder is also observed30,33. Both can effectively reduce the mobility of the device. Nevertheless, our theory shows that the measured mobility is, most likely, correlated with the primary type of defects in a sample.
Discussion
We have to address the possible influence of electron beam irradiation on the formation of atomic defects and to distinguish the native from irradiation-induced defects. The observed antisite defects are believed to be native, not caused by electron beam irradiation. We envisage that two steps are involved in the formation of a MoS defect from a well-prepared sample, namely the formation of a VS vacancy and Mo adatom, followed by the capture of the Mo adatom by the S vacancy. Although sulphur vacancy could be created by electron beam sputtering31,39, the formation of a Mo adatom and adjacent Mo vacancy need substantially high energy transfer from electron irradiation, which is less likely. The in-situ experiments show that the Mo adatoms are very mobile, but rarely jump into S vacancies to form antisite defects (Supplementary Note 3 and Supplementary Figs 15 and 16). On considering these experimental and simulation results, the observed MoS and MoS2 antisites should be confidently regarded as intrinsic defects. In terms of S vacancies, as suggested by early studies30,31, the concentration of sulphur vacancies may be slightly overestimated due to beam damage even if the microscope works at low accelerating voltage (Supplementary Fig. 17).
MoS2 sheets are extensively adopted in electronic devices. These point defects, as localized disorders, are significant scattering centres of carriers, which may reduce the mobility of charge carriers through the intrinsic conduction or valence band, especially for samples with antisite defects. Therefore, growth of ultra-high-quality m-MoS2 is of crucial importance to fabricate high-performance electronic devices. On the other side, the presence of defects may provide us novel routes to tailor the properties of m-MoS2. We predicted theoretically for the first time that antisite MoS shows a magnetic moment of 2 μB. Our prediction of local magnetic moments may promote further investigations on the magnetic properties of defective MoS2 monolayers. Given the strong optoelectronic response of MoS2 layers with MoS defects, it is a probable material that is capable for optical manipulation of local magnetic moment. If the defect density goes sufficiently high, it may expect an appreciably large magnetic exchange interaction between defects, and thus become a promising model system for the studies of dilute-magnetic semiconductors and 2D magnetism.
Based on these findings, we propose an application-oriented strategy for fabricating atomically thin MoS2. In terms of electric applications, extra S should be introduced into the growth process or post-growth treatment, to restrain the formation of antisite defects for PVD specimen or to heal the abundant S vacancies for CVD and ME specimens, whereas in respect to magnetism, varied pressure of S in the PVD growth could produce different densities of MoSx antisites that remain to be explored for magnetic applications.
In summary, our systematic investigation of geometric and electronic structures of antisites and vacancies of m-MoS2 by ADF-STEM imaging and DFT calculation has led to a considerable progress in our understanding of the variation of the electric and magnetic properties induced by these point defects. We have demonstrated that minimizing point defects, especially antisites, is paramount for electric transport applications, while controllably introduced antisites may produce atomic size local magnetic moments. All these results considerably improve the understanding of point defect in atomically thin transition metal dichalcogenides and should benefit their potential applications in optoelectronic and nanoelectronic devices.
Methods
Sample preparations and transfer
ME m-MoS2 was prepared by micro-cleavage8 of natural bulk crystal (SPI Supplies) using scotch tapes. The monolayer was identified from the optical contrast of thin flakes under an optical microscope (Zeiss A2m) and then transferred onto copper TEM grids covered with holey carbon films.
CVD monolayers were synthesized through the reduction of precursor MoO3 by sulphur vapour flow at ambient pressures following the previously reported method21,32. PVD MoS2 monolayers used in this study were synthesized by thermal evaporation of MoS2 powders (Sigma-Aldrich, 99%) at a temperature of 950 °C. Ar (2 s.c.c.m.) and H2 (0.5 s.c.c.m.) were used as the carriers gases, following the reported method in ref. 25. The pressure of the growth chamber was about 8 Pa and the growth time was usually 10 min. In terms of sample synthesis, the advantages and disadvantages of these methods and liquid-phase exfoliation are compared in Supplementary Table 2.
PVD and CVD MoS2 monolayers were transferred onto the TEM grid as follows: first, the SiO2 substrates with monolayer samples were covered with polymethyl methacrylate (PMMA) film after spin coating and then dried in air at 120 °C for 5 min. The substrates were immersed into the boiling sodium hydroxide solution (1 mol l−1), which was heated up to 200 °C to etch away the underneath SiO2 layers. The floating PMMA film was picked up with a clean glass slide and then transferred into the distilled water for several cycles to wash away surface residues. In the next step, the PMMA film was lifted out by a TEM grid covered with lacey carbon film and then dried naturally in ambient. This TEM grid was heated at 120 °C for 5 min in air before immersion into hot acetone for about 24 h, to remove the PMMA. Before the ADF-STEM characterization, all the monolayer specimens on TEM grids were annealed at 200 °C in air for 10 min to reduce surface residues and/or contaminations.
STEM characterization and image simulation
Most of the structural characterizations of m-MoS2 were carried out with a probe-corrected Titan ChemiSTEM (FEI, USA). We operated this microscope at an acceleration voltage of 80 kV to alleviate specimen damage induced by beam radiation. A low probe current was selected (<70 pA) and the convergence angle was set to be 22 mrad. Under such a condition, the probe size was estimated to be close to 1.5 Å. To enhance the contrast of the sulphur sublattices, the so-called medium-range ADF mode rather than the high-angle ADF mode was used by adjusting the camera length properly. Some experiments (such as Figs 1a and 2a–c) were done with an ARM 200CF (JEOL, Japan), equipped with a cold field-emission gun. The advantage of higher energy resolution (0.3 eV) and smaller probe size (<1.2 Å) provides higher resolution, thus giving rise to sharper contrast of the atomic images. All the experimental images shown in the main text and Supplementary Information were filtered through the standard Wiener deconvolution to partially remove the background noise for a better display (Supplementary Fig. 10).
It should be noted that intrinsic adatom defects were seldom observed experimentally and, therefore, they are not considered here. All the image statistics were done on the clean regions of the examined samples by ADF–STEM. On considering the good homogeneity of these samples prepared under the optimized conditions (see Supplementary Information), it shall not lead any large variations in the analysed defect population.
STEM–ADF image simulations of relaxed antisite defects were done by software QSTEM49. The input parameters were set according to the experimental conditions. Probe size, convergence angle and acceptance angle of the ADF detector are critical and accounted for in the image simulation.
DFT calculations
The defect formation enthalpy for the first column of Table 1 was calculated using the total energy method with the plane-wave pseudopotential DFT code CASTEP41. The basic methodology is well known and has been widely used before for defect calculations. In this study, the Perdew-Burke-Ernzerhof-generalized gradient approximation50 with ultrasoft potentials is used, as supplied in the CASTEP library. In addition, the dispersion interactions were added using the semi-empirical scheme of Grimme51. Structural optimizations of both ionic positions and cell vectors are performed using a modified Broyden-Fletcher-Goldfarb-Shanno-like scheme. The calculations were performed in a slab geometry of a 6 × 6 supercell of m-MoS2 with a 15 Å vacuum space in the c axis direction perpendicular to the monolayer. A comparative study of the defect formation enthalpy and energy, together with the electronic and magnetic properties were also done by VASP simulation code42 using the same slab model. The projector augmented-wave method52 combined with a plane wave basis is adopted in the calculations. The energy cutoff for plane wave is 400 eV in structural relaxation and increases to 500 eV while calculating the energy and electronic properties. The optB86b exchange functional53 together with the vdW correlation54,55 was adopted for exchange-correlation functional. The Brillouin zone of the supercell is sampled by a 3 × 3 × 1 k-mesh. All these structures are fully relaxed until the residual force for each atom is less than 0.02 eV Å−1.
Estimation of formation energy and enthalpy
The formation energy was defined as, ΔEForm=ESystem–NS × ES_ML–NMo × EMo_ML, where ES_ML=ES(single)+EBond, EMo_ML=EMo(single)+2EBond and EBond=(EML–EMo(single)−2ES(single))/3. Formation enthalpy of defects is defined as  , where μRemoved and μAdded are the chemical potentials of the removed and added atoms to form a defect, respectively. Chemical potentials of Mo and S in MoS2 fulfill the equation
, where μRemoved and μAdded are the chemical potentials of the removed and added atoms to form a defect, respectively. Chemical potentials of Mo and S in MoS2 fulfill the equation  , where μ*Mo is the chemical potential of Mo in the bulk form, μ*S is the chemical potential of S in the α-phase crystal form and
, where μ*Mo is the chemical potential of Mo in the bulk form, μ*S is the chemical potential of S in the α-phase crystal form and  is the formation enthalpy of MoS2. Although it is difficult to obtain the exact values of μMo and μS, the range of them can be deduced as
is the formation enthalpy of MoS2. Although it is difficult to obtain the exact values of μMo and μS, the range of them can be deduced as  ,
,  .
.
There are two formation enthalpy values in Table 1, the former one was computed by choosing μMo and μS equal to μ*Mo and μ*S, respectively, indicating that the removed (added) atoms come from (go to) the pure bulk form of Mo and S. For the latter value, we set  and
and  . In this case, the source and drain of defect atoms are pure MoS2 ML. For antisite defects MoS2 and
. In this case, the source and drain of defect atoms are pure MoS2 ML. For antisite defects MoS2 and  , both schemes give the same result.
, both schemes give the same result.
Estimation of ‘phonon-limited’ carrier mobility
In 2D, the carrier mobility is given by the expression45,46,47

where  is the effective mass in the transport direction and md is the average effective mass determined by
is the effective mass in the transport direction and md is the average effective mass determined by  . The term E1 represents the deformation potential constant of the valence-band maximum for holes or conduction-band minimum for electrons along the transport direction, defined by
. The term E1 represents the deformation potential constant of the valence-band maximum for holes or conduction-band minimum for electrons along the transport direction, defined by  . Here ΔVi is the energy change of the ith band under proper cell compression and dilatation, l0 is the lattice constant in the transport direction and Δl is the deformation of l0.
. Here ΔVi is the energy change of the ith band under proper cell compression and dilatation, l0 is the lattice constant in the transport direction and Δl is the deformation of l0.
FET fabrication and transport
The monolayer MoS2-based FET devices are fabricated through the following process. First, source (S) and drain (D) electrodes of the devices were defined via e-beam lithography and a 5/45 nm Ti/Au film was then evaporated followed by a standard lift-off process. In addition, the back-gated MoS2 FETs were then finished. Second, the top-gated devices were begun with forming gate insulator. Gate oxide layer (30 nm HfO2 film) was grown under 90 °C through Atomic Layer Deposition (Cambridge NanoTech Inc.). Lastly, the gate electrode window was also defined by e-beam lithography, followed by evaporation of 5 nm Ti and 45 nm Au thin film, and the top-gated MoS2 FETs are finished after a lift-off process. The as-fabricated devices were measured through Keithley 4200 semiconductor analyser on a probe station at room temperature and in air. An example of the device architecture is shown in Supplementary Fig. 18.
Additional information
How to cite this article: Hong, J. et al. Exploring atomic defects in molybdenum disulphide monolayers. Nat. Commun. 6:6293 doi: 10.1038/ncomms7293 (2015).
References
Novoselov, K. S. et al. Two-dimensional gas of massless Dirac fermions in graphene. Nature 438, 197–200 (2005).
Geim, A. K. & Novoselov, K. S. The rise of graphene. Nat. Mater. 6, 183–191 (2007).
Zhang, Y. B., Tan, Y. W., Stormer, H. L. & Kim, P. Experimental observation of the quantum Hall effect and Berry’s phase in graphene. Nature 438, 201–204 (2005).
Novoselov, K. S. et al. Electronic properties of graphene. Phys. Stat. Sol. B 244, 4106–4111 (2007).
Li, X. et al. Large-area synthesis of high-quality and uniform graphene films on copper foils. Science 324, 1312–1314 (2009).
Wang, Q. H., Kalantar-Zadeh, K., Kis, A., Coleman, J. N. & Strano, M. S. Electronics and optoelectronics of two-dimensional transition metal dichalcogenides. Nat. Nanotechnol. 7, 699–712 (2012).
Ataca, C., Sahin, H. & Ciraci, S. Stable single-layer MX2 transition-metal oxides and dichalcogenides in a honeycomb-like structure. J. Phys. Chem. C. 116, 8983–8999 (2012).
Mak, K. F., Lee, C., Hone, J., Shan, J. & Heinz, T. F. Atomically thin MoS2: a new direct-gap semiconductor. Phys. Rev. Lett. 105, 136805 (2010).
Splendiani, A. et al. Emerging photoluminesence in monolayer MoS2 . Nano Lett. 10, 1271–1275 (2010).
Zeng, H., Dai, J., Yao, W., Xiao, D. & Cui, X. Valley polarization in MoS2 monolayers by optical pumping. Nat. Nanotechnol. 7, 490–493 (2012).
Mak, K. F., He, K., Shan, J. & Heinz, T. F. Control of valley polarization in monolayer MoS2 by optical helicity. Nat. Nanotechnol. 7, 494–498 (2012).
Cao, T. et al. Valley-selective circular dichroism of monolayer molybdenum disulphide. Nat. Commun. 3, 887 (2012).
Radisavljevic, B., Radenovic, A., Brivio, J., Giacometti, V. & Kis, A. Single-layer MoS2 transistors. Nat. Nanotechnol. 6, 147–150 (2011).
Kim, S. et al. High-mobility and low-power thin-film transistors based on multilayer MoS2 crystals. Nat. Commun. 3, 1011 (2012).
Perera, M. M. et al. Improved carrier mobility in few-layer MoS2 field-effect transistors with ionic-liquid gating. ACS Nano 7, 4449–4458 (2013).
Zhang, Y. J., Ye, J. T., Matsuhashi, Y. & Iwasa, Y. Ambipolar MoS2 thin flake transistors. Nano Lett. 12, 1136–1140 (2012).
Schmidt, H. et al. Transport properties of monolayer MoS2 grown by chemical vapor deposition. Nano Lett. 14, 1909–1913 (2014).
Zou, X. M. et al. Interface engineering for high-performance top-gated MoS2 field-effect transistors. Adv. Mater. 26, 6255–6261 (2014).
Radisavljevic, B. & Kis, A. Mobility engineering and a metal-insulator transition in monolayer MoS2 . Nat. Mater. 12, 815–820 (2013).
Wang, X., Feng, H., Wu, Y. & Jiao, L. Controlled synthesis of highly crystalline MoS2 flakes by chemical vapor deposition. J. Am. Chem. Soc. 135, 5304–5307 (2013).
Lee, Y.-H. et al. Synthesis of large-area MoS2 atomic layers with chemical vapor deposition. Adv. Mater. 24, 2320–2325 (2012).
Liu, K.-K. et al. Growth of large-area and highly crystalline MoS2 thin layers on insulating substrates. Nano Lett. 12, 1538–1544 (2012).
Shi, Y. et al. van der Waals epitaxy of MoS2 layers using graphene as growth templates. Nano Lett. 12, 2784–2791 (2012).
Wu, S. et al. Vapor-solid growth of high optical quality MoS2 monolayers with near-unity valley polarization. ACS Nano 7, 2768–2772 (2013).
Feng, Q. et al. Growth of large-area 2D MoS2(1-x) Se2x semiconductor alloys. Adv. Mater. 26, 2648–2653 (2014).
Fuhrer, M. S. & Hone, J. Measurement of mobility in dual-gated MoS2 transistors. Nat. Nanotechnol. 8, 146–147 (2013).
Radisavljevic, B. & Kis, A. Measurement of mobility in dual-gated MoS2 transistors. Nat. Nanotechnol. 8, 147–148 (2013).
Yu, Z. et al. Towards intrinsic charge transport in monolayer molybdenum disulfide by defect and interface engineering. Nat. Commun. 5, 5290 (2014).
Liu, H. et al. Statistical study of deep submicron dual-gated field-effect transistors on monolayer chemical vapor deposition molybdenum disulfide films. Nano Lett. 13, 2640–2646 (2013).
Qiu, H. et al. Hopping transport through defect-induced localized states in molybdenum disulphide. Nat. Commun. 4, 2642 (2013).
Komsa, H.-P. et al. Two-dimensional transition metal dichalcogenides under electron irradiation: defect production and doping. Phys. Rev. Lett. 109, 035503 (2012).
Najmaei, S. et al. Vapour phase growth and grain boundary structure of molybdenum disulphide atomic layers. Nat. Mater. 12, 754–759 (2013).
Ghatak, S., Pal, A. N. & Ghosh, A. Nature of electronic states in atomically thin MoS2 field-effect transistors. ACS Nano 5, 7707–7712 (2011).
Li, S.-L. et al. Thickness-dependent interfacial Coulomb scattering in atomically thin field-effect transistors. Nano Lett. 13, 3546–3552 (2013).
Zhou, W. et al. Intrinsic structural defects in monolayer molybdenum disulfide. Nano Lett. 13, 2615–2622 (2013).
van der Zande, A. M. et al. Grains and grain boundaries in highly crystalline monolayer molybdenum disulphide. Nat. Mater. 12, 554–561 (2013).
Pennycook, S. J. et al. Scanning Transmission Electron Microscopy-Imaging and Analysis Springer (2011).
Sze, S. M. & Ng, K. K. Physics of Semiconductor Devices John Wiley & Sons, Inc.: New Jersey, (2007).
Komsa, H. P., Kurasch, S., Lehtinen, O., Kaiser, U. & Krasheninnikov, A. V. From point to extended defects in two-dimensional MoS2: evolution of atomic structure under electron irradiation. Phys. Rev. B 88, 035301 (2013).
Zhu, W. et al. Electronic transport and device prospects of monolayer molybdenum disulphide grown by chemical vapour deposition. Nat. Commun. 5, 3087 (2014).
Clark, S. J. et al. First principles methods using CASTEP. Z. Kristallogr. 220, 567–570 (2005).
Kresse, G. & Furthmuller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 54, 11169–11186 (1996).
Baugher, B. W. H., Churchill, H. O. H., Yang, Y. & Jarillo-Herrero, P. Intrinsic electronic transport properties of high-quality monolayer and bilayer MoS2 . Nano Lett. 13, 4212–4216 (2013).
Bao, W. Z., Cai, X. H., Kim, D., Sridhara, K. & Fuhrer, M. S. High mobility ambipolar MoS2 field-effect transistors: substrate and dielectric effects. Appl. Phys. Lett. 102, 042104 (2013).
Takagi, S.-i., Toriumi, A., Iwase, M. & Tango, H. On the universality of inversion layer mobility in Si MOSFET's: part I-effects of substrate impurity concentration. IEEE Trans. Electr. Dev. 41, 2357–2362 (1994).
Qiao, J. S. et al. High-mobility transport anisotropy and linear dichroism in few-layer black phosphorus. Nat. Commun. 5, 4475 (2014).
Fiori, G. & Iannaccone, G. Multiscale modeling for graphene-based nanoscale transistors. Proc. IEEE 101, 1653–1669 (2013).
Kaasbjerg, K., Thygesen, K. S. & Jacobsen, K. W. Phonon-limited mobility in n-type single-layer MoS2 from first principles. Phys. Rev. B 85, 115317 (2012).
Koch, C. T. Determination of Core Structure Periodicity and Point Defect Density Along Dislocations PhD thesisArizona State Univ. (2002).
Perdew, J. P., Burke, K. & Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 77, 3865–3868 (1996).
Grimme, S. Semiempirical GGA-type density functional constructed with a long-range dispersion correction. J. Comput. Chem. 27, 1787–1799 (2006).
Blochl, P. E. Projector augmented-wave method. Phys. Rev. B 50, 17953–17979 (1994).
Klimes, J., Bowler, D. R. & Michaelides, A. Van der waals density functionals applied to solids. Phys. Rev. B 83, 195131 (2011).
Dion, M., Rydberg, H., Schroder, E., Langreth, D. C. & Lundqvist, B. I. Van der Waals density functional for general geometries. Phys. Rev. Lett. 92, 246401 (2004).
Lee, K., Murray, E. D., Kong, L., Lundqvist, B. I. & Langreth, D. C. Higher-accuracy van der waals density functional. Phys. Rev. B 82, 081101 (2010).
Acknowledgements
This work was financially supported by the National Basic Research Program of China under grant numbers 2012CB932704, 2014CB932500 and 2015CB921000; National Science Foundation of China under grant numbers 51222202, 11004244, 11274380, 91433103 and 51472215; and the Fundamental Research Funds for the Central Universities under grant numbers 2014XZZX003-07 (ZJU), 12XNLJ03 and 14XNH062 (RUC). JY and MP acknowledge the EPSRC (UK) funding (EP/G070326, EP/J022098 and EP/K013564). WJ was supported by the Program for New Century Excellent Talents in Universities. The work on electron microscopy was mainly done at the Center for Electron Microscopy of Zhejiang University. VASP Calculations were performed at the Physics Lab of High-Performance Computing of Renmin University of China and Shanghai Supercomputer Center, and Castep calculations were done in York. JY acknowledges Pao Yu-Kong International Foundation for a Chair Professorship. We thank Zheng Meng, Haiyan Nan and Zhenhua Ni for their assistance on PL measurements, Fang Lin for her assistance on provding the codes for Wiener filtering, and Yanfeng Zhang and Liying Jiao for providing high-quality CVD MoS2 samples. We acknowledge Shengbai Zhang for his advice on formation mechanism of different defects and other calculations, and Wang Yao for his advices about the spin-valley effects in m-MoS2.
Author information
Authors and Affiliations
Contributions
J.H. and Z.H. contributed equally to this work. C.J., J.Y. and W.J. conceived the research. J.H., D.L. and N.M. contributed to the sample preparations. J.H. and C.J did most of the STEM characterizations, with the assistance from K.L., X.Y., L.G. and X.Z., J.H., J.Y. and C.J. were responsible for the STEM data analysis and image simulations. Z.H., W.J. and M.P. did the DFT calculations. N.M., L.X. and J.H. contributed to the synthesis, measurement and analysis of PL spectra and the PVD devices of PVD samples. D.W. and Z.Z. contributed to the FET device fabrications and electric transport measurements on CVD samples. All authors discussed the results and contributed to the preparation of the manuscript.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary Information
Supplementary Figures 1-18, Supplementary Tables 1-2, Supplementary Notes 1-3 and Supplementary References (PDF 2181 kb)
Rights and permissions
This work is licensed under a Creative Commons Attribution 4.0 International License. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in the credit line; if the material is not included under the Creative Commons license, users will need to obtain permission from the license holder to reproduce the material. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/
About this article
Cite this article
Hong, J., Hu, Z., Probert, M. et al. Exploring atomic defects in molybdenum disulphide monolayers. Nat Commun 6, 6293 (2015). https://doi.org/10.1038/ncomms7293
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/ncomms7293
This article is cited by
-
Atomically engineering metal vacancies in monolayer transition metal dichalcogenides
Nature Synthesis (2024)
-
Accurate atomic scanning transmission electron microscopy analysis enabled by deep learning
Nano Research (2024)
-
Excitonic devices based on two-dimensional transition metal dichalcogenides van der Waals heterostructures
Frontiers of Chemical Science and Engineering (2024)
-
Atomic-level polarization in electric fields of defects for electrocatalysis
Nature Communications (2023)
-
Towards the realisation of high permi-selective MoS2 membrane for water desalination
npj Clean Water (2023)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.