Introduction

Spin-orbit coupling (SOC) is a manifestation of Einstein’s theory of relativity in condensed matter systems. Recently, SOC has attracted a great deal of attention since it is a main ingredient for spintronics applications1,2, induces novel quantum phases3,4 and generates new particles and elementary excitations5,6. Moreover, when incorporated with electron correlations, SOC can give rise to even more fascinating phenomena7,8. In the iridium oxide family, where the IrO6 octahedron is the essential building block, various quantum phases have been predicted or verified according to the electron correlation strength on top of the large SOC of the Ir 5d t2g orbital: topological band insulator for weak coupling9,10, Weyl semi-metal, axion insulator, non-Fermi liquid and TI* phases for intermediate coupling11,12,13,14,15, and topological Mott insulator and quantum spin liquid phases for strong coupling7,16,17.

Emergence of the spin-orbital entangled jeff states induced by SOC18,19 is the key feature to host all the above phases, yet the existence of such states is limited to a small number of iridate compounds only. Here, the series of lacunar spinel compounds20,21, GaM4X8, where early 4d or 5d transition metal atoms occupy the M-site, are found to provide the molecular form of the jeff basis in their low-energy electronic structures. The idealness of the molecular jeff state is guaranteed by the formation of the M4 metal cluster and the large SOC. Combined with the ability to control the electron correlation from the weak to strong coupling limit, the lacunar spinels can manifest themselves as the best candidates to demonstrate this so-called jeff physics.

Results

Formation of the molecular jeff states in GaTa4Se8

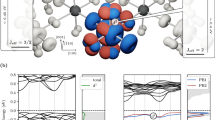
The chemical formula and crystal structure of the GaM4X8 lacunar spinels are easily deduced from the spinel with half-deficient Ga atoms, that is, Ga0.5M2X4. Due to the half removal of the Ga atoms, the transition metal atoms are strongly distorted into the tetrahedral center as denoted by the red arrows in Fig. 1a, and a tetramerized M4 cluster appears. The M4 cluster yields a short intra-cluster M–M distance, naturally inducing the molecular states residing on the cluster as basic building blocks for the low-energy electronic structure. On the other hand, the large inter-cluster distance results in a weak inter-cluster bonding and a narrow bandwidth of the molecular states.

Figure 1: Molecular form of spin-orbital entangled jeff states in GaTa4Se8.
figure 1

(a) The connectivity between the neighbouring M4 clusters and the local distortion of each cluster. (b) Band structure and PDOS of GaTa4Se8 without SOC. (c) Three Wannier orbitals constructed from the triplet molecular orbital bands near the Fermi level. (d) Band structure and DOS with SOC, projected onto the jeff=1/2 and 3/2 subspaces. The size of the circle in the band structure shows the weight of each subspace in each Bloch state.

Full size image

As a representative example of the lacunar spinels, we investigate the electronic structure of GaTa4Se8 (Fig. 1b–d). Figure 1b shows the band structure and the projected density of states (PDOS) of GaTa4Se8 in the absence of SOC. In consistency with previous studies21,22,23, the triply degenerate molecular t2 bands occupied by one electron are located near the Fermi level with a small bandwidth of ~0.75 eV. As shown in the PDOS plot, the molecular t2 bands are dominated by Ta t2g orbital components; the small admixture of Se 5p and the strong tetramerization imply that the molecular t2 states consist of direct bonding between Ta t2g states.

The molecular nature of the low-energy electronic structure can be visualized by adopting the maximally localized Wannier function scheme24,25. The three molecular t2 Wannier functions depicted in Fig. 1c read

where Dα and dα denote the molecular t2 and atomic t2g states, respectively, and i is a site index indicating the four corners of the M4 cluster. Each Dα originates from a σ-type strong bonding between the constituent t2g orbitals in the M4 cluster. (See Supplementary Note 1, Supplementary Fig. 1 and Supplementary Table 1 for details on the molecular t2 Hamiltonian.) Owing to the exact correspondence between the molecular t2 and the atomic t2g states, as revealed in equation 1, the molecular t2 triplet carries the same effective orbital angular momentum leff=1 as the atomic t2g orbital18. By virtue of SOC, the leff=1 states are entangled with the s=1/2 spin, and two multiplets designated by the effective total angular momentum jeff=1/2 and 3/2 emerge. The band structure and PDOS of GaTa4Se8 in the presence of SOC verify the above jeff picture (Fig. 1d); the molecular t2 bands split into upper jeff=1/2 and lower jeff=3/2 bands. The separation between the two jeff subbands is almost perfect owing to the large SOC of the Ta atoms as well as the small bandwidth of the molecular t2 band. An alternative confirmation of the jeff picture can also be given by constructing the Wannier function from each of the jeff subbands, which shows a 99% agreement with the ideal molecular jeff states. (See Supplementary Fig. 2.) Consequently, the electronic structure of GaTa4Se8 can be labelled as a quarter-filled jeff=3/2 system on a face-centered cubic lattice.

Robust jeff-ness in the GaM4X8 series

The aforementioned jeff-ness in GaTa4Se8 remains robust in the GaM4X8 series with a neighbouring 5d transition metal (M=W) as well as the 4d counterparts (M=Nb and Mo). Among the series, M=W compounds have not been reported previously in experiments; thus we use optimized lattice parameters by structural relaxations. In Fig. 2a–d, the electronic structures of GaTa4Se4Te426, GaW4Se4Te4, GaNb4Se821 and GaMo4Se827 are shown—band structure, PDOS and Fermi surface with projection onto the molecular jeff states. In Fig. 2a,b, one can see the clear separation and identification of the higher jeff=1/2 doublet and the lower jeff=3/2 quartet driven by the large SOC of the 5d transition metal atoms. The overall band dispersions are quite similar, except for the location of the Fermi level; the M=Ta and M=W lacunar spinels are well characterized by the quarter-filled jeff=3/2 and the half-filled jeff=1/2 systems, respectively. In 4d compounds, the separation between the jeff subbands is reduced due to the smaller SOC compared with that of the 5d systems (Fig. 2c,d). Nevertheless, there is a discernible splitting between the jeff=1/2 and 3/2 bands, which is comparable to or even better than that in the prototype jeff compounds, Sr2IrO4 and Ba2IrO428.

Figure 2: jeff-ness in the GaM4X8 series.
figure 2

The molecular jeff-projected band structures, DOS and the Fermi surfaces of (a) GaTa4Se4Te4, (b) GaW4Se4Te4, (c) GaNb4Se8 and (d) GaMo4Se8 are presented. (e) The relation between the external hydrostatic pressure, lattice constant and bandwidth of the molecular t2 bands in the absence of SOC.

Full size image

To acquire a well-identified jeff band, we need the jeff state as a local basis, and the inter-orbital hopping terms between the jeff subspaces should be suppressed. Hence, there are three important conditions to realize the ideal jeff system: high symmetry protecting the leff=1 threefold orbital degeneracy, small bandwidth minimizing the inter-orbital mixing and large SOC fully entangling the spin and orbital degrees of freedom. The lacunar spinel compounds comfortably satisfy the above conditions; the tetrahedral symmetry of the M4 cluster protects the orbital degeneracy, the long inter-cluster distance leads to the small bandwidth and a large SOC is inherent in 4d and 5d transition metal atoms.

Figure 2e introduces one important controlling parameter—the bandwidth. By changing the inter-cluster distance via external pressure and/or by substituting chalcogen atoms, the bandwidth of the molecular t2 band can be tuned over a wide range. In the M=Ta series, for example, the bandwidth varies from 0.4 to 1.1 eV. Consequently, the effective electron correlation strength, given by the ratio between the bandwidth and the on-site Coulomb interactions, can be controlled to reach from the weak to the strong coupling regime. In fact, the bandwidth-controlled insulator-to-metal transitions were observed in GaTa4Se4 and GaNb4Se423,29, implying that both the weakly and strongly interacting limits are accessible in a single compound.

Effective Hamiltonian

From the apparent separation between the jeff subbands, as well as the similar band dispersions, the GaM4X8 series are governed by a common effective Hamiltonian composed of two independent jeff=1/2 and 3/2 subspaces, that is, . (See Supplementary Notes 2 and 3.) Therefore, the compounds with M=Nb/Ta and M=Mo/W are described by the quarter-filled and the half-filled systems, respectively. The nearest-neighbor hopping terms for each subspace are written as

where S1/2 and S3/2 are the jeff=1/2 and 3/2 pseudospin matrices, respectively, and Γ are the 5-component Dirac Gamma matrices. t0 and tQ’s are even, and tD’s are odd functions under the spatial inversion; tD’s are allowed by the inversion asymmetry of the M4 cluster. The pseudospin-dependent hopping terms tD and tQ can be interpreted as the effective magnetic dipolar and quadrupolar fields acting on the hopping electron, respectively.

DFT+SOC+U calculations

So far, we have discussed about the jeff-ness without containing electron correlations, which provides a valid picture in the weak coupling regime. Once taking electron correlations into account, one important question arises on the robustness of the molecular jeff states under the influence of the on-site Coulomb interaction. To answer this question, we perform DFT+SOC+U calculations for GaTa4Se4Te4, GaW4Se4Te4, GaNb4Se8 and GaMo4Se8. We consider two simplest magnetic configurations, ferromagnetic and antiferromagnetic order, and the antiferromagnetic solutions for each compound are shown in Fig. 3. In the 5d compounds, the molecular jeff states remain robust with developing a SOC-assisted Mott gap within each jeff subspace (Fig. 3a,b). For the 4d compounds, the jeff character is enhanced from the non-interacting cases in Fig. 2c,d; the occupied states in GaNb4Se8 (Fig. 3c) and the unoccupied states in GaMo4Se8 (Fig. 3d) are dominated by jeff=3/2 and 1/2 characters, respectively. The strengthened jeff character by the cooperation with electron correlations is consistent with the recent theoretical results on Sr2IrO428,30. See the Supplementary Note 4, Supplementary Figs 3–6 and Supplementary Tables 2–5 for more details.

Figure 3: DFT+SOC+U calculations.
figure 3

The jeff-projected band structure and DOS of (a) GaTa4Se4Te4, (b) GaW4Se4Te4, (c) GaNb4Se8 and (d) GaMo4Se8 with the presence of electron correlations and antiferromagnetic order.

Full size image

Discussion

The effective Hamiltonian of the lacunar spinel series has intriguing implications both in the weak and strong coupling regimes. As suggested in previous studies3,9,31, the effective fields exerted on the hopping electron can induce a topological insulating phase in the weak coupling regime. In fact, a non-trivial band topology is realized within the molecular jeff bands in-thin film geometries: the monolayer (Fig. 4a) and the bilayer thin film (Fig. 4b) of the M4 clusters normal to the (111) direction. Each system corresponds to the triangular and honeycomb lattice, respectively, and the inter-layer coupling enhanced by a factor of three is adopted in the bilayer system. Non-trivial gaps emerge in the half-filled jeff=3/2 bands in the monolayer and the half-filled jeff=1/2 bands in the bilayer system. A two-dimensional (2D) topological insulator phase is indicated by an odd number of edge Dirac cones at time-reversal invariant momenta in ribbon geometries (Fig. 4a,b). Such 2D geometries might be feasible with the help of the state-of-the-art epitaxial technique prevailing in oxide perovskite compounds32, or by mechanically cleaving the single crystal to get clean surfaces as done in previous studies on GaTa4Se833,34.

Figure 4: Topological insulating phases and anisotropic spin model.
figure 4

The one-dimensional band structure of (a) half-filled jeff=3/2 monolayer and (b) half-filled jeff=1/2 bilayer M4 ribbons (20 unit cell width). The insets show schematic top view of each system, where the thin grey and the thick red lines represent the intra- and the inter-planar bonding, respectively. The thickness of the coloured fat lines in the band structure represent the weights on the edge. (c) Two mirror planes (blue and red) existing in between the neighbouring M4 clusters determine the direction of illustrated as green arrow. (d) Magnitudes of Heisenberg (dark red), Dzyaloshinskii–Moriya (green) and pseudodipolar (blue) exchange interactions as a function of |t0/tD|. The magnitude of |t0/tD| for each of the M=Mo/W compounds is marked on the horizontal axis. (e) The 90°- and (f) the 60°-compass interactions are realized on (001) and (111) M4 monolayers, respectively.

Full size image

In the strong coupling regime, the large on-site Coulomb terms are added to the kinetic Hamiltonian, and the hopping terms are treated as perturbations. The localized jeff pseudospins become low-energy degrees of freedom and exchange interactions between the neighbouring jeff moments emerge. In the simplest example, the one-band Hubbard model within the half-filled , the resulting spin Hamiltonian for the jeff=1/2 moments is written as35,36

among the exchange interaction terms, the Dzyaloshinskii–Moriya Dij and the pseudodipolar interaction Aij depend on , whose direction is determined by the two mirror planes, as illustrated in Fig. 4c (details in Supplementary Note 5). As shown in Fig. 4d, the relative magnitude of each exchange term is changed with different chalcogen atoms, so that systematic study of the anisotropic Hamiltonian in equation 3 can be made in the M=Mo/W compounds. Especially, GaMo4S8 and GaW4Se8 satisfies the limit of |t0/tD|→0, where the spin Hamiltonian becomes highly anisotropic and bond direction dependent such that

with . In addition to the Heisenberg term, the Hamiltonian contains the bond-dependent and Ising-like pseudodipolar interaction, called as a Heisenberg-compass model37. It can be further reduced to distinct 2D spin models in thin-film geometries. Figure 4e,f shows two examples—the (001) and (111) monolayer lead to the 90°- and 60°-compass model with the Heisenberg exchange term on a square and a triangular lattice, respectively.

The jeff=3/2 systems in the strong coupling limit could also have a significant implication in terms of unconventional multipolar orders38,39,40. On top of the nonmagnetic insulating behaviour, the weak tetragonal superstructure and the anomalous magnetic response observed in GaNb4S8 at T~31 K41 could give some clues on the quadrupolar ordered phase as well as the spin liquid phase suggested in ref. 39, which promptly calls for further research on the jeff=3/2 spin model.

The formation of the M4 cluster and SOC are the essential requisites to realize the molecular jeff state in these 3D intermetallic compounds. The strong tetramerization sustains the isolated molecular bands with threefold orbital degeneracy and narrow bandwidth, and the large SOC fully entangles the spin and orbital components. The existence of the pure quantum state has been shedding light on studying the ideal quantum model systems in strongly correlated physics; the Hubbard Hamiltonian or the frustrated spin Hamiltonian based on the pure spin-half state has been realized in several organic compounds42,43,44. Likewise, the molecular form of the ideal jeff state as a pure quantum state might be of great use to explore the emergent phenomena in the spin-orbit-coupled correlated electron systems.

Methods

First-principles calculations

Structural optimizations were done with the projector augmented wave potentials and the PBEsol45 generalized gradient approximation as implemented in the Vienna ab initio Simulation Package46,47. Momentum space integrations were performed on a 12 × 12 × 12 Monkhorst-Pack grid, and a 300-eV energy cutoff was used for the plane-wave basis set. The force criterion was 10−3 eV Å−1, and the pressures exerted were estimated by using the Birch–Murnaghan fit.

For the electronic structure calculations, we used OPENMX code48 based on the linear-combination-of-pseudo-atomic-orbital basis formalism. Four hundred Rydberg units of energy cutoff was used for the real-space integration. SOC was treated via a fully relativistic j-dependent pseudopotential in a non-collinear scheme. Simplified DFT+U formalism by Dudarev et al.49, implemented in OPENMX code50, was adopted in the DFT+SOC+U calculations. Ueff≡U−J=2.5 and 2.0 eV was used for the 4d and 5d compounds, respectively.

Additional information

How to cite this article: Kim, H.-S. et al. Spin-orbital entangled molecular jeff states in lacunar spinel compounds. Nat. Commun. 5:3988 doi: 10.1038/ncomms4988 (2014).