Abstract
The 1s exciton—the ground state of a bound electron-hole pair—is central to understanding the photoresponse of monolayer transition metal dichalcogenides. Above the 1s exciton, recent visible and near-infrared investigations have revealed that the excited excitons are much richer, exhibiting a series of Rydberg-like states. A natural question is then how the internal excitonic transitions are interrelated on photoexcitation. Accessing these intraexcitonic transitions, however, demands a fundamentally different experimental tool capable of probing optical transitions from 1s ‘bright’ to np ‘dark’ states. Here we employ ultrafast mid-infrared spectroscopy to explore the 1s intraexcitonic transitions in monolayer MoS2. We observed twofold 1s→3p intraexcitonic transitions within the A and B excitons and 1s→2p transition between the A and B excitons. Our results revealed that it takes about 0.7 ps for the 1s A exciton to reach quasi-equilibrium; a characteristic time that is associated with a rapid population transfer from the 1s B exciton, providing rich characteristics of many-body exciton dynamics in two-dimensional materials.
Similar content being viewed by others
Introduction
Photogenerated electron-hole (e–h) pairs in solids create bound states, whose elementary quasiparticle state is called 1s exciton in a Wannier–Mott exciton model. Since the optoelectronic response is governed by the light-induced dynamic behaviour of this elementary ground state, knowledge of the 1s exciton response to the optical stimuli has been a crucial issue in many optoelectronic applications, such as phototransistors1,2, photovoltaics3, light-emitting diodes4,5, van der Waals heterostructure-based optoelectronics6,7,8 and valleytronic device applications5,9,10,11,12. In transition metal dichalcogenides (TMDCs), this is particularly the case as the two-dimensional (2D) materials approach a monolayer limit, where the reduced dielectric screening results in a strong Coulomb interaction13,14,15,16,17,18,19,20,21, leading to an unusually large 1s exciton binding energy Ebind, typically a few hundreds of meV below the electronic bandgap of a few eV (refs 16, 22, 23, 24, 25, 26, 27).
Above the fundamental 1s exciton, theories predicted the presence of densely spaced exciton states in monolayer MoS2 with 1s exciton Ebind of 0.4–0.54 eV (refs 18, 19, 20, 21, 28, 29, 30, 31), whose (s-like) bright and (p-like) dark exciton characters were later confirmed by a series of seminal experiments via linear one-photon absorption21,22, two-photon photoluminescence excitation (PLE)22,23,24,32 and nonlinear wave-mixing spectroscopy25,32, whereby Ebind was experimentally measured to be between 0.22 (ref. 26) and 0.44 eV (refs 23, 26); the reported Ebind, however, shows somewhat discrepancy depending on the measurement methods and is varied from samples to samples23,26,27. These experimental techniques, although they are appropriate to clarify the optical state of the excitons, may address indirectly the dynamic transient information between the 1s ‘bright’ and the excited np ‘dark’ exciton (n is the principle quantum number); we denoted the exciton states in analogy to the hydrogen series21. By contrast, if one measures the 1s→np transitions, then the data should describe the internal excitonic transients, directly providing the transient optical nature of the fundamental 1s exciton dynamics. This, so called intraexcitonic spectroscopy33, fundamentally differs from band-to-band and other time-resolved spectroscopies8,34,35,36, and the technique can not only explain the transient response of the 1s exciton, but more importantly, may provide experimental manoeuvre in exploiting the photoinduced excitonic responses to the TMDC-based optoelectronic devices. For example, knowledge of the 1s and np exciton energies and their associated dynamics afford the first-order quantitative information on the exciton dissociation energy, where in an ideal case at least Ebind/e (e is the electron charge) of an external or internal potential is required to dissociate the bound e–h pairs. In addition, because intraexcitonic spectroscopy can access the p-like dark excitons, one may design a scheme of coupling an infrared (IR) light to the 2D TMDC materials, via below-gap two-photon excitation, for the light-harnessing applications.
Here we explore the 1s intraexcitonic transient dynamics in monolayer MoS2 by using time-resolved mid-IR spectroscopy. Inspired by a theoretical GW–Bethe–Salpeter result19, where the fundamental 1s→2p transitions are predicted to be 0.32 and 0.3 eV for the A and B exciton in isolated, suspended monolayer MoS2, we employed an ultrafast mid-IR spectroscopy (0.23–0.37 eV probe) in conjunction with an ultrafast white-light continuum spectroscopy (Fig. 1a). The mid-IR measurements show that there are two 1s→3p transitions for A and B exciton and 1s→2p between 1s A and 2p B exciton. The time-dependent IR absorption rapidly subdues over broad probe–photon energies, representing the transient absorption from the 1s to the quasi-continuum states after pump excitation.
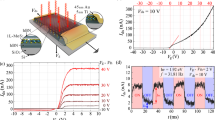
(a) Schematic illustration of the ultrafast intraexcitonic (green) and conventional band-to-band (red) interband spectroscopy. The 3.1 eV optical pump (blue arrow) creates e–h pairs from ground to quasi-continuum states or C-band. The mid-IR pulse (green) measures the 1s→np transitions, while the white-light continuum pulse measures the ground-to-1s transition. (b) Transient dynamics of ΔT/T0 measured at two representative energies of 1.86 eV (red) and 0.35 eV (green). The positive sign of ΔT/T0 is observed for 1.86 eV probe, while 0.35 eV probe exhibits a negative sign. A clear temporal delay (∼0.2 ps) between the two rising transients was observed. The experimentally determined temporal error bar for each time-zero (20 fs for 0.35 eV and 8 fs for 1.86 eV) is discussed in Supplementary Fig. 8.
Results
Time-resolved intraexcitonic and band-to-band dynamics
The samples used in our experiment were monolayer MoS2, grown by chemical vapour deposition method, and were transferred to a CaF2 substrate (see Supplementary Note 1 for the sample characterization). As schematically shown in Fig. 1a, the sample was non-resonantly excited by a 70 fs, 3.1 eV pump pulse, and the corresponding differential-transmission changes ΔT/T0 were measured in a vacuum cryostat (Methods). The 3.1 eV pump excites carriers into the quasi-continuum of the A and B excitons37,38 or into the band-nesting C-band near the Γ point39,40. The former generates the unbound e–h plasma above the A and B excitons and the latter case experiences a rapid inter-valley scattering into K and K′ valley. Nevertheless, both cases generate carriers in much higher energy compared with the A or B exciton resonance. Figure 1b shows a direct comparison of two representative data measured by mid-IR probe (0.35 eV) and interband A-exciton probe (1.86 eV) with the same pump fluence F=24.4 μJ cm−2 (equivalent to e–h pair density of 7.4 × 1012 cm−2 given 15% absorption)8,41 measured at 77 K. The polarization of pump and probe beam are linear and orthogonal with respect to each other, such that we do not account for the recently discovered valley-exciton-locked selection rule32. The fact that two ΔT/T0 transients exhibit an opposite sign implies the kinetic origin of the photoresponses is indeed different. For the 1.86 eV dynamics, the increased probe transmission is typically attributed to the ground-state bleaching35,42,43, where the increased occupation probability of electrons in conduction band and holes in the valance band leads to the reduced probe absorption, that is, increased ΔT/T0>0. On the other hand, given that the 0.35 eV probe is far below the band-to-band A-exciton energy, one may attribute the decreased ΔT/T0<0 (increased probe absorption) to the transition within the bands, that is, intraband free-carrier absorption. However, considering that the mid-IR peak signal is only 36.2% of the 1.86 eV one, we can exclude this possibility because the intraband oscillator strength is much smaller than the interband one, usually by an order of magnitude, as revealed by prior works on the quasi-2D quantum wells44 or recent 2D MoS2 (ref. 45); as discussed later in Figs 2, 3, 4, we provide compelling experimental evidences to support our rationale (see also Supplementary Note 2 (refs 46, 47, 48)). The increased probe absorption of the mid-IR suggests that there exists an occupied state below the electronic gap.
Left panel is the mid-IR transient and right panel is the IR transient dynamics. The transient spectra (red dots) are fitted by three Lorentzian oscillators (blue, orange and green) for the mid-IR range and a step function (red) for the IR range. The red solid in left panel is the sum of three intraexcitonic oscillators. We note that it was not possible to fit all the mid-IR spectra if only two oscillators were used.
(a) Energy diagram of the ground state (G), and the fundamental excitons (1s,A and 1s,B) and the higher excited np dark excitons in shown. Transition energies of three oscillators are indicated by blue (0.27 eV), orange (0.31 eV) and green (0.36 eV) arrows. The transient band-to-band dynamics (b) is directly compared with the intraexcitonic absorption dynamics (c–e). Transient dynamics of the intraexcitonic spectral weight parameter S1s→np for each three oscillator are shown at each row: (c) 1s,A→3p,A, (d) 1s,B→3p,B and (e) 1s,A→2p,B, respectively. Dashed lines show the maximum S1s→np peak for each intraexcitonic transition.
(a) Temperature-dependent −ΔT/T0 dynamics measured at 0.6 eV. Inset: the peak value of −ΔT/T0 is plotted as a function of the temperature. No temperature-dependent dynamics were observed, thereby the free-carrier absorption can be excluded in the analysis of Figs 1, 2, 3. We performed fitting using a biexponential function. The summarized results are shown in b for the fast τ1 and in c for the slow decay component τ2, where both components are temperature-independent. (d) Fluence-dependent −ΔT/T0 dynamics measured at 0.6 eV probe. Inset: the peak −ΔT/T0 shows a linear fluence dependence, such that no higher-order nonlinear exciton dynamics were observed. Fluence-dependent fast τ1 (e) and slow decay component τ2 (f). Solid line for each trace is the corresponding biexponential fit. Both τ1 and τ2 are independent of the pump fluence, implying that no absorption occurs from the defect states.
We find that there exists a clear time departure between the two rising dynamics, where the onset of the 0.35 eV probe peak appears ∼0.2 ps later than the 1.86 eV probe (dashed line in Fig. 1b). Immediately after the pump, the 1.86 eV probe rapidly increases, while the 0.35 eV dynamics emerge rather slowly. We observed that this rapid upsurge of the 1.86 eV is not a local spectral behaviour, but being presented in a broad range of high-energy probes (Supplementary Notes 3 and 4), evidencing the quasi-instantaneous ground-state bleaching35. Understanding this high-energy dynamics has been a subject to debate; different investigations have proposed different kinetic origins of the 1s exciton, such as exciton linewidth broadening35, stimulated emission42, dynamic bandgap renormalization49 and biexciton formation38. As discussed, more details in Supplementary Note 4, both earlier50,51,52 and recent studies35,42,53, have shown that the 3.1 eV photoexcitation into the quasi-continuum of unbound states generates a significant amount of free-carriers. Because the exciton formation occurs after exciton–free carrier scattering, the initial decaying kinetics of mid-IR is slightly delayed compared with the rising transient of the interband one, explaining the observed time-delay between the two transients of Fig. 1b. Since the mid-IR probe can resonantly measure the internal exciton dynamics, the measured intraexcitonic transients are expected to provide pure population dynamics of the ground 1s exciton33,54.
Temperally and spectrally resolved intraexcitonic dynamics
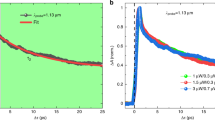
Figure 2 is the temporally and spectrally resolved mid-IR dynamics. Here we probed not only the broad mid-IR transients (0.23–0.37 eV), but also measured the IR dynamics (0.47–0.67 eV). This scheme affords a simultaneous access to the dynamic transitions from the 1s ground exciton to the higher lying np excitons or quasi-continuum states. The measured −ΔT/T0 spectra show peculiar energy-dependent behaviours. At Δt≤0.4 ps, the −ΔT/T0 spectra are strongly reshaped, exhibiting a relatively small increase of differential absorption (not absolute absorption) near 0.27 eV compared with the increased absorption around 0.3–0.5 eV. The increased absorption is more prominent at Δt>0.4 ps, where one can see that −ΔT/T0 is gradually larger near 0.27 eV with increasing Δt, and the differential absorption at 0.3–0.5 eV is concurrently smaller with increasing Δt. Between 0.4<Δt≤0.9 ps, −ΔT/T0 above 0.3 eV is rapidly vanished, while the absorption resonance below 0.3 eV is accordingly increased. After Δt>0.9 ps, the absorption resonance below 0.3 eV keeps reserved and it slowly subdues with featureless IR spectra above 0.3 eV.
For a quantitative analysis of the observed transient spectra, we use the following model consisted of multi-oscillator components55:

The term of summation represents the intraexcitonic absorption from 1s to either A or B excitonic np state and the second term Θ(E−Ebind) is a step-like transition21 from 1s to the continuum with Ebind of 0.44 eV. In the equation, ɛ (=4.2) (refs 14, 27) and ɛ0 are the dielectric constant of monolayer MoS2 and the vacuum dielectric constant, respectively. There, the absorption amplitude S1s→np, or the spectral weight of the intraexcitonic 1s→np transition, is proportional to the product of the oscillator strength f1s→np and the ground exciton density n1s (refs 39, 40, 55, 56). Because the 3.1 eV pump excitation creates e–h plasma in the band nesting resonance, an accurate estimation of n1s requires both theoretical study of intervalley scatterings and the corresponding ultrafast measurements, which is beyond the scope of our ultrafast mid-IR intraexcitonic spectroscopy. In fact, the spectral weight from 1s→np is not only proportional to the population, but also depends on the probability of finding an empty final np state. As discussed about the transient spectra dynamics above, the photoexcited unbound e–h pairs experience rapid relaxation and start to form a ground-state exciton within ∼0.4 ps. It is strictly true that the np exciton population is negligible only at Δt⩾0.4 ps. Similar studies on 1D and quasi-2D quantum-well structures have shown that the contribution from np→continuum is negligible33,54,56,57,58. We found that the spectral fit matches well the measured data when we used up to three mid-IR oscillators, with the following transition energy En of E1=0.27 eV, E2=0.31 eV, and E3=0.36 eV. On the basis that the observed Ens do not vary Δt, we fix En to fit the time-resolved mid-IR spectra, but vary S1s→np and the phenomenological exciton broadening parameter Γ. For the IR transients, the spectra are featureless representing the step-function-like 1s to the continuum transition21; this featureless IR spectrum is deviated from the step-like absorption at Δt≤0.4 ps, which might be due to the time-dependent thermalization process after the pump excitation.
Given that the magnitude of S1s→np differs only by a factor of 2 for each En, these transitions cannot simply be assigned to the phenomena taking place within single exciton Rydberg series of the A (or B) exciton branch. This is because even when the nonhydrogenic excitonic nature of a monolayer TMDC is considered, where a strongly (weakly) screened Coulomb potential is dominant when n≤2 (n⩾3) (ref. 21), the spectral weight should be substantially decreased by nearly an order of magnitude with increasing n, which is too large to account for our measurement results. Of course, care should be taken to estimate the precise strength of intraexcitonic transition in a monolayer TMDC because the nonhydrogenic exciton is dominant when n≤2, thus the 1s→np transition can deviate from the hydrogenic excitonic nature for n⩾3. This is because any intraexcitonic 1s→np transition depends both on the wavefunction of the nth exciton as well as the 1s exciton state, and the latter certainly deviates from the 2D-hydrogen model. Recent PLE23 revealed that the energy levels of the exciton Rydberg series are 1.88 eV (1s), 2.05 eV (2s) and 2.15 eV (3s) for A exciton and 2.03 eV (1s), 2.24 eV (2s) and 2.34 eV (3s) for B exciton. By considering 0.15 eV energy splitting between A and B excitons and the difference of reduced exciton masses of 0.25m0 (A exciton) and 0.28m0 (B exciton)18, we estimated the intraexcitonic transition energies of 0.27 eV for E1A→3A, 0.31 eV for E1B→3B and 0.36 eV for E1A→2B, which are exactly matched our measured intraexcitonic transition energy En. Interestingly, these values are somewhat deviated from the GW–Bethe–Salpeter prediction19, possibly due to the substrate dielectric screening effect. Nevertheless, our measurements agree well with the experimental PLE investigation due to similar dielectric constant of CaF2 and fused silica23 as a substrate. Although there is a small difference (∼20 meV) for the A exciton energy between PLE (1.88 eV) and our photocurrent spectra and ultrafast absorption measurement (1.86 eV, Supplementary Notes 1 and 3), the difference is very marginal19,28,30,31 and the intraexcitonic spectroscopy can measure the energy difference between 1s and np, regardless of the A-exciton resonance. In accordance with PLE and our mid-IR measurements, we expect the fundamental 1s→2p would be 0.17 eV for the A exciton and 0.21 eV for the B exciton, and this is beyond our capability of tuning the mid-IR spectrum. Therefore, as schematically shown in Fig. 3a, we understand our intraexcitonic transition energy of E1 as 1s,A→3p,A within A exciton, E2 as 1s,B→3p,B within B exciton and E3 as 1s,A→2p,B between A and B exciton. Indeed, our energy assignment well-corroborates a recent many-body Bethe–Salpeter prediction on the nonhydrogenic characters of excited excitons19,20,28,30,31, underscoring a distinct capability of our intraexcitonic spectroscopy in measuring the relative energy difference between 1s and np. For the exciton broadening parameter Γ, since the effective mass of A and B exciton is different, Γ (=28.2 meV) for 1s,A→3p,A, Γ (=37.4 meV) for 1s,B→3p,B and Γ (=30 meV) for 1s,A→2p,B are slightly different due to the different exciton dispersion.
Dynamics of 1s→np intraexcitonic spectral weights
For further analysis, we show the temporal dynamics of S1s,A→3p,A (Fig. 3c, blue), S1s,B→3p,B (Fig. 3d, orange) and S1s,A→3p,A (Fig. 3e, green). We identify three different kinetic regimes: immediately after the pump, the rising transients of all three spectral weights show similar behaviours, representing the hot-carrier relaxation from the quasi-continuum to the A and B exciton branch. This kinetics clearly differs from the dynamics of 1.86 eV probe (Fig. 3b), where the latter arises from the quasi-instantaneous bleaching dynamics. At 0.4≤Δt≤0.7 ps, the dynamics of S1s,B→3p,B rapidly decrease, while the peak S1s,A→3p,Aand S1s,A→3p,B emerge ∼0.3 ps later. Because the 1s B exciton is 0.15 eV higher than that of A exciton (Fig. 3a), the 1s B exciton serves as a population supplier to the energetically lower 1s A exciton, thereby the two transients show a complementary dynamics. At longer Δt>0.7 ps, because the 1s A excitons are thermalized and reaches a quasi-equilibrium condition, the dynamics of S1s,A→3p,A nearly follows that of S1s,A→2p,B. This highlights that although S1s,A→3p,A and S1s,A→3p,B are spectrally separated apart, that is 0.27 and 0.36 eV, respectively, both transients are closely interrelated because these absorptions originate from the same 1s,A ground state exciton.
Discussion
At an elevated temperature, the free-carrier absorption from 1s, 2s, 2p, 3s, 3p… may contribute to the increased probe absorption with ΔT/T0<0. This scenario typically shows a strong temperature dependence of the relaxation rate, in which the higher temperature the larger the electron–phonon scattering rate, resulting in the dynamics to be highly temperature dependent. Here given that the formation time scale of the 1s exciton is very fast within 0.4 ps (see Figs 2 and 3) and the Drude scattering rate cannot be extended to the mid-IR range (Supplementary Note 2), the contribution of np→continuum transition may be very insignificant to the temperature-dependent mid-IR intraexcitonic response. Figure 4a shows that our mid-IR transients, fitted by a biexponential function, exhibit nearly temperature independent of the relaxation components (Fig. 4b,c). This implies that the effect of free-carrier absorption is negligible. We additionally show in Fig. 4b that the recombination of excitons arises on sub-ps and tens of ps time scale. At T=77 K, the mid-IR peak |ΔT/T0| linearly increases with F up to 32.5 μJ cm−2 (equivalent to e–h pair density of 9.86 × 1012 cm−2) (refs 8, 41). The linear F-dependence reflects that there exists no high-order nonlinear excitonic interaction, ensuring that our mid-IR transients represent the first-order population dynamics. A recent below-gap-probe study45 reported very similar relaxation times to our results. These time components were explained using defect-assisted exciton recombination. Given that we observed negligible F-dependent relaxation dynamics (Fig. 4e,f), we can infer that our mid-IR decay transients do not arise from the photoinduced absorption of filled e–h pair in the localized states, but arise from the exciton capture into the defects.
In summary, we report the experimental observation of the 1s intraexcitonic transition. Recently, Poellmann et al.47 investigated a similar investigation of intraexcitonic transition in monolayer WSe2, reporting the presence of strong absorption in a 2D TMDC, whose fundamental optical absorption originates from the 1s ground exciton. Our ultrafast mid-IR measurements reveal twofold 1s→3p transition energies to be 0.27 eV and 0.31 eV for A and B exciton, respectively. We additionally uncover an intraexcitonic relaxation channel of 1s→2p to be 0.36 eV between 1s A and 2p B exciton. The large exciton-binding energy due to the non-local dielectric screening ensures not only 1s→2p transition47 to be observable, but also a higher-order transition of 1s→3p in a monolayer 2D TMDC at an elevated temperature, which cannot be accessible using conventional interband spectroscopy, or any in quasi-2D quantum-well structures. In addition, looking to the future, the availability of electric-gate tuning may enable to investigate the coherent many-body inter-excitonic correlations among exciton, biexciton38,59 and trion12,13,18,48 in a time-resolved controlled manner, which is non-trivial to study in other low-dimensional inorganic semiconductor structures.
Methods
Ultrafast optical pump–probe spectroscopy
Using 250 kHz, 50 fs Ti/sapphire laser system (Coherent RegA 9050), optical parametric amplifier (Coherent OPA 9850) yields signal (0.77–1.12 eV) and idler (0.47 eV–0.67 eV) pulses that are used to generate mid-IR pulse (0.23–0.37 eV) via difference frequency generator (Coherent DFG). The idler and DFG output serve as the probe pulse in the IR and mid-IR range, respectively. The chirp of mid-IR pulse is discussed in Supplementary Note 5. High-energy interband response was measured by using a white-light continuum (1.76–2.03 eV) generated by focusing 1.55 eV pulses into a 1 mm sapphire disk. For the group-delay dispersion (GDD) of the white-light continuum pulse, we compensated using a pair of prism, and further checked the GDD-induced delay via cross-correlation of the white-light pulse and 1.55 eV pulse, whose details are explained in the Supplementary Note 3. The 3.1 eV pump pulse was created by second harmonic generation of 1.55 eV pulse in a 1-mm-thick beta barium borate (BBO) crystal. Due to the combination of OPA and DFG, where both signal and idler from OPA were used to generate the mid-IR DFG output, the only available seed pulse for 3.1 eV pump pulse was 1.55 eV in our system, so that the mid-IR measurement with resonant pump excitation at either A or B 1s exciton was not possible in our current system. For the each mid-IR or IR measurement, pump pulse and probe pulse are simultaneously focused on the sample in the cryostat equipped with two CaF2 windows, and pump–probe delay is controlled by a mechanical delay stage (Newport M-IMS300LM). The spot size of our pump and probe beams were 100 μm, and 50 μm, respectively, which were simultaneously focused using f=50 mm lens before the temperature-controlled vacuum cryostat. In our optical geometry, the 3.1 eV pump passes through a mechanical delay stage, so called ‘pump delay’. Because the pump delay is recorded in a computer as an absolute length, we performed cross-correlation measurement to estimate the probe delay using BBO (visible upconversion) and KTA (mid-IR upconversion) crystals. More detailed information for determining the pump–probe ‘time-zero’ is explained in Supplementary Note 6. Differential transmission signal (ΔT/T0) was recorded in a lock-in amplifier (Stanford Research Systems SR850) with 10 kHz chopping frequency (Scitec 300CD). The schematics of mid-IR and IR setup are illustrated in the Supplementary Fig. 9.
Additional information
How to cite this article: Cha, S. et al. 1s intraexcitonic dynamics in monolayer MoS2 probed by ultrafast mid-infrared spectroscopy. Nat. Commun. 7:10768 doi: 10.1038/ncomms10768 (2016).
References
Lopez-Sanchez, O., Lembke, D., Kayci, M., Radenovic, A. & Kis, A. Ultrasensitive photodetectors based on monolayer MoS2 . Nat. Nano 8, 497–501 (2013).
Wang, Q. H., Kalantar-Zadeh, K., Kis, A., Coleman, J. N. & Strano, M. S. Electronics and optoelectronics of two-dimensional transition metal dichalcogenides. Nat. Nano 7, 699–712 (2012).
Furchi, M. M., Pospischil, A., Libisch, F., Burgdorfer, J. & Mueller, T. Photovoltaic effect in an electrically tunable van der Waals heterojunction. Nano Lett. 14, 4785–4791 (2014).
Cheng, R. et al. Electroluminescence and Photocurrent generation from atomically sharp WSe2/MoS2 heterojunction p–n diodes. Nano Lett. 14, 5590–5597 (2014).
Zhang, Y., Oka, T., Suzuki, R., Ye, J. & Iwasa, Y. Electrically switchable chiral light-emitting transistor. Science 344, 725–728 (2014).
Britnell, L. et al. Strong light-matter interactions in heterostructures of atomically thin films. Science 340, 1311–1314 (2013).
Lee, C.-H. et al. Atomically thin pn junctions with van der Waals heterointerfaces. Nat. Nano 9, 676–681 (2014).
He, J. et al. Electron transfer and coupling in graphene–tungsten disulfide van der Waals heterostructures. Nat. Commun. 5, 5622 (2014).
Gong, Z. et al. Magnetoelectric effects and valley-controlled spin quantum gates in transition metal dichalcogenide bilayers. Nat. Commun. 4, 2053 (2013).
Xu, X., Yao, W., Xiao, D. & Heinz, T. F. Spin and pseudospins in layered transition metal dichalcogenides. Nat. Phys. 10, 343–350 (2014).
Mak, K. F., McGill, K. L., Park, J. & McEuen, P. L. The valley Hall effect in MoS2 transistors. Science 344, 1489–1492 (2014).
Jones, A. M. et al. Optical generation of excitonic valley coherence in monolayer WSe2 . Nat. Nano 8, 634–638 (2013).
Mak, K. F., Lee, C., Hone, J., Shan, J. & Heinz, T. F. Atomically thin MoS2: a new direct-gap semiconductor. Phys. Rev. Lett. 105, 136805 (2010).
Cheiwchanchamnangij, T. & Lambrecht, W. R. Quasiparticle band structure calculation of monolayer, bilayer, and bulk MoS2 . Phys. Rev. B 85, 205302 (2012).
Mak, K. F. et al. Tightly bound trions in monolayer MoS2 . Nat. Mater. 12, 207–211 (2013).
Ugeda, M. M. et al. Giant bandgap renormalization and excitonic effects in a monolayer transition metal dichalcogenide semiconductor. Nat. Mater. 13, 1091–1095 (2014).
Zhang, Y. et al. Direct observation of the transition from indirect to direct bandgap in atomically thin epitaxial MoSe2 . Nat. Nano 9, 111–115 (2014).
Berkelbach, T. C., Hybertsen, M. S. & Reichman, D. R. Theory of neutral and charged excitons in monolayer transition metal dichalcogenides. Phys. Rev. B 88, 045318 (2013).
Qiu, D. Y., Felipe, H. & Louie, S. G. Optical spectrum of MoS2: many-body effects and diversity of exciton states. Phys. Rev. Lett. 111, 216805 (2013).
Berghäuser, G. & Malic, E. Analytical approach to excitonic properties of MoS2 . Phys. Rev. B 89, 125309 (2014).
Chernikov, A. et al. Exciton binding energy and nonhydrogenic rydberg series in monolayer WS2 . Phys. Rev. Lett. 113, 076802 (2014).
He, K. et al. Tightly bound excitons in monolayer WSe2 . Phys. Rev. Lett. 113, 026803 (2014).
Hill, H. M. et al. Observation of excitonic Rydberg states in monolayer MoS2 and WS2 by photoluminescence excitation spectroscopy. Nano Lett. 15, 2992–2997 (2015).
Ye, Z. et al. Probing excitonic dark states in single-layer tungsten disulphide. Nature 513, 214–218 (2014).
Wang, G. et al. Giant enhancement of the optical second-harmonic emission of WSe 2 monolayers by laser excitation at exciton resonances. Phys. Rev. Lett. 114, 097403 (2015).
Zhang, C., Johnson, A., Hsu, C.-L., Li, L.-J. & Shih, C.-K. Direct imaging of band profile in single layer MoS2 on graphite: quasiparticle energy gap, metallic edge states, and edge band bending. Nano Lett. 14, 2443–2447 (2014).
Klots, A. R. et al. Probing excitonic states in suspended two-dimensional semiconductors by photocurrent spectroscopy. Sci. Rep. 4, 6608 (2014).
Berkelbach, T. C., Hybertsen, M. S. & Reichman, D. R. Bright and dark singlet excitons via linear and two-photon spectroscopy in monolayer transition metal dichalcogenides, Preprint at http://arxiv.org/abs/1505.07127 (2015).
Stroucken, T. & Koch, S. W. Evidence for optically bright p-excitons in transition-metal dichalchogenides, Preprint at http://arxiv.org/abs/1404.4238v2 (2014).
Wu, F., Qu, F. & MacDonald, A. H. Exciton band structure of monolayer MoS2 . Phys. Rev. B 91, 075310 (2015).
Chaves, A., Low, T., Avouris, P., Çakır, D. & Peeters, F. Anisotropic exciton Stark shift in black phosphorus. Phys. Rev. B 91, 155311 (2015).
Xiao, J. et al. Optical selection rule based on valley-exciton locking for 2D valleytronics, Preprint at http://arxiv.org/abs/1504.04947 (2014).
Kaindl, R. A., Carnahan, M. A., Hägele, D., Lövenich, R. & Chemla, D. S. Ultrafast terahertz probes of transient conducting and insulating phases in an electron–hole gas. Nature 423, 734–738 (2003).
Shi, H. et al. Exciton dynamics in suspended monolayer and few-layer MoS2 2D crystals. ACS Nano 7, 1072–1080 (2013).
Sim, S. et al. Exciton dynamics in atomically thin MoS2: interexcitonic interaction and broadening kinetics. Phys. Rev. B 88, 075434 (2013).
Hong, X. et al. Ultrafast charge transfer in atomically thin MoS2/WS2 heterostructures. Nat. Nano 9, 682–686 (2014).
Cabo, A. G. et al. Observation of ultrafast free carrier dynamics in single layer MoS2 . Nano Lett. 15, 5883–5887 (2015).
Sie, E. J., Frenzel, A. J., Lee, Y.-H., Kong, J. & Gedik, N. Intervalley biexcitons and many-body effects in monolayer MoS2 . Phys. Rev. B 92, 125417 (2015).
Carvalho, A., Ribeiro, R. & Neto, A. C. Band nesting and the optical response of two-dimensional semiconducting transition metal dichalcogenides. Phys. Rev. B 88, 115205 (2013).
Kozawa, D. et al. Photocarrier relaxation pathway in two-dimensional semiconducting transition metal dichalcogenides. Nat. Commun. 5, 4543 (2014).
Li, Y. et al. Measurement of the optical dielectric function of monolayer transition-metal dichalcogenides: MoS2, MoSe2, WS2, and WSe2 . Phys. Rev. B 90, 205422 (2014).
Mai, C. et al. Many-body effects in valleytronics: direct measurement of valley lifetimes in single-layer MoS2 . Nano Lett. 14, 202–206 (2013).
Mai, C. et al. Exciton valley relaxation in a single layer of WS2 measured by ultrafast spectroscopy. Phys. Rev. B 90, 041414 (2014).
Klimov, V., Schwarz, C. J., McBranch, D., Leatherdale, C. & Bawendi, M. Ultrafast dynamics of inter-and intraband transitions in semiconductor nanocrystals: Implications for quantum-dot lasers. Phys. Rev. B 60, R2177 (1999).
Wang, H., Zhang, C. & Rana, F. Ultrafast dynamics of defect-assisted electron–hole recombination in monolayer MoS2 . Nano Lett. 15, 339–345 (2015).
Shen, C.-C. et al. Charge dynamics and electronic structures of monolayer MoS2 films grown by chemical vapor deposition. Appl. Phys. Exp. 6, 125801 (2013).
Poellmann, C. et al. Resonant internal quantum transitions and femtosecond radiative decay of excitons in monolayer WSe2 . Nat. Mater. 14, 889–893 (2015).
Lui, C. H. et al. Trion-induced negative photoconductivity in monolayer MoS2 . Phys. Rev. Lett. 113, 166801 (2014).
Wang, Q. et al. Valley carrier dynamics in monolayer molybdenum disulfide from helicity-resolved ultrafast pump–probe spectroscopy. ACS Nano 7, 11087–11093 (2013).
Wake, D. R., Yoon, H. W., Wolfe, J. P. & Morkoç, H. Response of excitonic absorption spectra to photoexcited carriers in GaAs quantum wells. Phys. Rev. B 46, 13452–13460 (1992).
Knox, W. H. et al. Femtosecond excitation of nonthermal carrier populations in GaAs quantum wells. Phys. Rev. Lett. 56, 1191–1193 (1986).
Knox, W. H., Chemla, D. S., Livescu, G., Cunningham, J. E. & Henry, J. E. Femtosecond carrier thermalization in dense Fermi seas. Phys. Rev. Lett. 61, 1290–1293 (1988).
Chernikov, A., Ruppert, C., Hill, H. M., Rigosi, A. F. & Heinz, T. F. Population inversion and giant bandgap renormalization in atomically thin WS2 layers. Nat. Photon. 9, 466–470 (2015).
Wang, J., Graham, M. W., Ma, Y., Fleming, G. R. & Kaindl, R. A. Ultrafast spectroscopy of midinfrared internal exciton transitions in separated single-walled carbon nanotubes. Phys. Rev, Lett. 104, 177401 (2010).
Jörger, M., Fleck, T., Klingshirn, C. & Von Baltz, R. Midinfrared properties of cuprous oxide: High-order lattice vibrations and intraexcitonic transitions of the 1s paraexciton. Phys. Rev. B 71, 235210 (2005).
Huber, R., Kaindl, R. A., Schmid, B. A. & Chemla, D. S. Broadband terahertz study of excitonic resonances in the high-density regime in GaAs. AlxGa1− xAs quantum wells. Phys. Rev. B 72, 161314 (2005).
Suzuki, T. & Shimano, R. Time-resolved formation of excitons and electron-hole droplets in Si studied using terahertz spectroscopy. Phys. Rev. Lett. 103, 057401 (2009).
Kaindl, R. A., Hägele, D., Carnahan, M. A. & Chemla, D. S. Transient terahertz spectroscopy of excitons and unbound carriers in quasi-two-dimensional electron-hole gases. Phys. Rev. B 79, 045320 (2009).
You, Y. et al. Observation of biexcitons in monolayer WSe2 . Nat. Phys. 11, 477–481 (2015).
Acknowledgements
S. Cha, S. Sim, J. Park and H. Choi were supported by the National Research Foundation of Korea (NRF) through the government of Korea (MSIP) (Grants No. NRF-2011-0013255, NRF-2009-0083512, NRF-2015R1A2A1A10052520), Global Frontier Program (2014M3A6B3063709), the Yonsei University Yonsei-SNU Collaborative Research Fund of 2014 and the Yonsei University Future-leading Research Initiative of 2014. J.H.S., H.H. and M.-H.J. were supported by Institute for Basic Science (IBS), Korea under the contract number of IBS-R014-G1-2016-a00.
Author information
Authors and Affiliations
Contributions
S.C. and H.C. conceived the idea and designed the experiments. H.H., J.H.S. and M.-H.J. fabricated and characterized vapour-phase-grown MoS2 monolayer crystals; and S.C., S.S. and J.P. conducted the ultrafast optical pump–probe spectroscopy. S.C., J.H.S. and S.S. analysed the results. All authors discussed the results and prepared the manuscript.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary Information
Supplementary Figures 1-10, Supplementary Notes 1-8 and Supplementary References. (PDF 1004 kb)
Rights and permissions
This work is licensed under a Creative Commons Attribution 4.0 International License. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in the credit line; if the material is not included under the Creative Commons license, users will need to obtain permission from the license holder to reproduce the material. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/
About this article
Cite this article
Cha, S., Sung, J., Sim, S. et al. 1s-intraexcitonic dynamics in monolayer MoS2 probed by ultrafast mid-infrared spectroscopy. Nat Commun 7, 10768 (2016). https://doi.org/10.1038/ncomms10768
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/ncomms10768
This article is cited by
-
Erratum to: Terahertz spectroscopy of high temperature superconductors and their photonic applications
Journal of the Korean Physical Society (2022)
-
Terahertz spectroscopy of high temperature superconductors and their photonic applications
Journal of the Korean Physical Society (2022)
-
Ultrafast transient sub-bandgap absorption of monolayer MoS2
Light: Science & Applications (2021)
-
The ultrafast onset of exciton formation in 2D semiconductors
Nature Communications (2020)
-
Enhanced interlayer neutral excitons and trions in trilayer van der Waals heterostructures
npj 2D Materials and Applications (2018)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.