Abstract
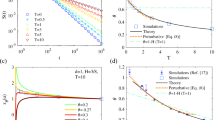
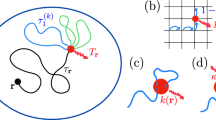
The first-passage time, defined as the time a random walker takes to reach a target point in a confining domain, is a key quantity in the theory of stochastic processes1. Its importance comes from its crucial role in quantifying the efficiency of processes as varied as diffusion-limited reactions2,3, target search processes4 or the spread of diseases5. Most methods of determining the properties of first-passage time in confined domains have been limited to Markovian (memoryless) processes3,6,7. However, as soon as the random walker interacts with its environment, memory effects cannot be neglected: that is, the future motion of the random walker does not depend only on its current position, but also on its past trajectory. Examples of non-Markovian dynamics include single-file diffusion in narrow channels8, or the motion of a tracer particle either attached to a polymeric chain9 or diffusing in simple10 or complex fluids such as nematics11, dense soft colloids12 or viscoelastic solutions13,14. Here we introduce an analytical approach to calculate, in the limit of a large confining volume, the mean first-passage time of a Gaussian non-Markovian random walker to a target. The non-Markovian features of the dynamics are encompassed by determining the statistical properties of the fictitious trajectory that the random walker would follow after the first-passage event takes place, which are shown to govern the first-passage time kinetics. This analysis is applicable to a broad range of stochastic processes, which may be correlated at long times. Our theoretical predictions are confirmed by numerical simulations for several examples of non-Markovian processes, including the case of fractional Brownian motion in one and higher dimensions. These results reveal, on the basis of Gaussian processes, the importance of memory effects in first-passage statistics of non-Markovian random walkers in confinement.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout



Similar content being viewed by others
References
Redner, S. A Guide to First- Passage Processes (Cambridge Univ. Press, 2001)
ben Avraham, D. & Havlin, S. Diffusion and Reactions in Fractals and Disordered Systems (Cambridge Univ. Press, 2000)
Bénichou, O. & Voituriez, R. From first-passage times of random walks in confinement to geometry-controlled kinetics. Phys. Rep. 539, 225–284 (2014)
Shlesinger, M. F. Mathematical physics: search research. Nature 443, 281–282 (2006)
Lloyd, A. L. & May, R. M. Epidemiology—how viruses spread among computers and people. Science 292, 1316–1317 (2001)
Condamin, S., Bénichou, O., Tejedor, V., Voituriez, R. & Klafter, J. First-passage times in complex scale-invariant media. Nature 450, 77–80 (2007)
Bénichou, O., Chevalier, C., Klafter, J., Meyer, B. & Voituriez, R. Geometry-controlled kinetics. Nat. Chem. 2, 472–477 (2010)
Wei, Q.-H., Bechinger, C. & Leiderer, P. Single-file diffusion of colloids in one-dimensional channels. Science 287, 625–627 (2000)
Panja, D. Anomalous polymer dynamics is non-Markovian: memory effects and the generalized Langevin equation formulation. J. Stat. Mech. 2010, P06011 (2010)
Franosch, T. et al. Resonances arising from hydrodynamic memory in Brownian motion. Nature 478, 85–88 (2011)
Turiv, T. et al. Effect of collective molecular reorientations on Brownian motion of colloids in nematic liquid crystal. Science 342, 1351–1354 (2013)
Démery, V., Bénichou, O. & Jacquin, H. Generalized Langevin equations for a driven tracer in dense soft colloids: construction and applications. New J. Phys. 16, 053032 (2014)
Ernst, D., Hellmann, M., Köhler, J. & Weiss, M. Fractional Brownian motion in crowded fluids. Soft Matter 8, 4886–4889 (2012)
Mason, T. G. & Weitz, D. Optical measurements of frequency-dependent linear viscoelastic moduli of complex fluids. Phys. Rev. Lett. 74, 1250 (1995)
Holcman, D. & Schuss, Z. The narrow escape problem. SIAM Rev. 56, 213–257 (2014)
Bénichou, O., Meyer, B., Tejedor, V. & Voituriez, R. Zero constant formula for first-passage observables in bounded domains. Phys. Rev. Lett. 101, 130601–130604 (2008)
Guérin, T., Bénichou, O. & Voituriez, R. Non-markovian polymer reaction kinetics. Nat. Chem. 4, 568–573 (2012)
Bénichou, O., Guérin, T. & Voituriez, R. Mean first-passage times in confined media: from Markovian to non-Markovian processes. J. Phys. A 48, 163001 (2015)
Kou, S. & Xie, X. S. Generalized Langevin equation with fractional Gaussian noise: subdiffusion within a single protein molecule. Phys. Rev. Lett. 93, 180603 (2004)
Wilemski, G. & Fixman, M. Diffusion-controlled intrachain reactions of polymers. 1. Theory. J. Chem. Phys. 60, 866–877 (1974)
Hanggi, P. & Talkner, P. First-passage time problems for non-Markovian processes. Phys. Rev. A 32, 1934–1937 (1985)
Masoliver, J., Lindenberg, K. & West, B. J. First-passage times for non-Markovian processes: correlated impacts on bound processes. Phys. Rev. A 34, 2351–2363 (1986)
Krug, J. et al. Persistence exponents for fluctuating interfaces. Phys. Rev. E 56, 2702 (1997)
Molchan, G. Maximum of a fractional Brownian motion: probabilities of small values. Commun. Math. Phys. 205, 97–111 (1999)
Bray, A. J., Majumdar, S. N. & Schehr, G. Persistence and first-passage properties in nonequilibrium systems. Adv. Phys. 62, 225–361 (2013)
Noh, J. D. & Rieger, H. Random walks on complex networks. Phys. Rev. Lett. 92, 118701 (2004)
Ochab-Marcinek, A. & Hołyst, R. Scale-dependent diffusion of spheres in solutions of flexible and rigid polymers: mean square displacement and autocorrelation function for FCS and DLS measurements. Soft Matter 7, 7366–7374 (2011)
Mandelbrot, B. B. & Wallis, J. R. Noah, Joseph, and operational hydrology. Wat. Resour. Res. 4, 909–918 (1968)
Cutland, N. J., Kopp, P. E. & Willinger, W. Stock price returns and the Joseph effect: a fractional version of the Black–Scholes model. In Seminar on Stochastic Analysis, Random Fields and Applications 327–351 (Springer, 1995)
Burnecki, K. et al. Universal algorithm for identification of fractional Brownian motion. A case of telomere subdiffusion. Biophys. J. 103, 1839–1847 (2012)
Acknowledgements
This work was supported by ERC grant FPTOpt-277998.
Author information
Authors and Affiliations
Contributions
All authors contributed equally to this work.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Additional information
Reviewer Information Nature thanks K. Lindenberg and the other anonymous reviewer(s) for their contribution to the peer review of this work.
Supplementary information
Supplementary Information
This file contains Supplementary Text and Data and Supplementary Figures 1-6. (PDF 1387 kb)
Rights and permissions
About this article
Cite this article
Guérin, T., Levernier, N., Bénichou, O. et al. Mean first-passage times of non-Markovian random walkers in confinement. Nature 534, 356–359 (2016). https://doi.org/10.1038/nature18272
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nature18272
This article is cited by
-
Random Walks on Quasi-One-Dimensional Lattices
Journal of Statistical Physics (2023)
-
Everlasting impact of initial perturbations on first-passage times of non-Markovian random walks
Nature Communications (2022)
-
Quantum model for understanding protein misfolding behavior—phase diagram and manual intervention
Science China Physics, Mechanics & Astronomy (2021)
-
Stochastic process-based degradation modeling and RUL prediction: from Brownian motion to fractional Brownian motion
Science China Information Sciences (2021)
-
Rapid onset of molecular friction in liquids bridging between the atomistic and hydrodynamic pictures
Communications Physics (2020)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.