Abstract
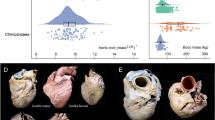
For more than three-quarters of a century it has been assumed1 that basal metabolic rate increases as body mass raised to some power p. However, there is no broad consensus regarding the value of p: whereas many studies have asserted that p is 3/4 (refs 1–4; ‘Kleiber’s law’), some have argued that it is 2/3 (refs 5–7), and others have found that it varies depending on factors like environment and taxonomy6,8,9,10,11,12,13,14,15,16. Here we show that the relationship between mass and metabolic rate has convex curvature on a logarithmic scale, and is therefore not a pure power law, even after accounting for body temperature. This finding has several consequences. First, it provides an explanation for the puzzling variability in estimates of p, settling a long-standing debate. Second, it constitutes a stringent test for theories of metabolic scaling. A widely debated model17 based on vascular system architecture fails this test, and we suggest modifications that could bring it into compliance with the observed curvature. Third, it raises the intriguing question of whether the scaling relation limits body size.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout



Similar content being viewed by others
References
Kleiber, M. Body size and metabolism. Hilgardia 6, 315–353 (1932)
Bartels, H. Metabolic rate of mammals equals the 0.75 power of their body weight. Exp. Biol. Med. 7, 1–11 (1982)
Feldman, H. A. & McMahon, T. A. The 3/4 mass exponent for energy metabolism is not a statistical artifact. Respir. Physiol. 52, 149–163 (1983)
Savage, V. M. et al. The predominance of quarter-power scaling in biology. Funct. Ecol. 18, 257–282 (2004)
Heusner, A. A. Energy metabolism and body size. I. Is the 0.75 mass exponent of Kleiber's equation a statistical artifact? Respir. Physiol. 48, 1–12 (1982)
Dodds, P. S., Rothman, D. H. & Weitz, J. S. Re-examination of the “3/4-law'' of metabolism. J. Theor. Biol. 209, 9–27 (2001)
White, C. R. & Seymour, R. S. Mammalian basal metabolic rate is proportional to body mass2/3 . Proc. Natl Acad. Sci. USA 100, 4046–4049 (2003)
McNab, B. K. An analysis of the factors that influence the level and scaling of mammalian BMR. Comp. Biochem. Physiol. A 151, 5–28 (2008)
Glazier, D. S. A unifying explanation for diverse metabolic scaling in animals and plants. Biol. Rev. Camb. Phil. Soc. 85, 111–138 (2009)
White, C. R., Phillips, N. F. & Seymour, R. S. The scaling and temperature dependence of vertebrate metabolism. Biol. Lett. 2, 125–127 (2006)
McNab, B. K. Complications inherent in scaling the basal rate of metabolism in mammals. Q. Rev. Biol. 63, 25–54 (1988)
Bokma, F. Evidence against universal metabolic allometry. Funct. Ecol. 18, 184–187 (2004)
Lovegrove, B. G. The zoogeography of mammalian basal metabolic rate. Am. Nat. 156, 201–219 (2000)
Lovegrove, B. G. The influence of climate on the metabolic rate of small mammals: a slow-fast metabolic continuum. J. Comp. Physiol. B 173, 87–112 (2003)
Heusner, A. A. Size and power in mammals. J. Exp. Biol. 160, 25–54 (1991)
Sieg, A. E. et al. Mammalian metabolic allometry: do intraspecific variation, phylogeny, and regression models matter? Am. Nat. 174, 720–733 (2009)
West, G. B., Brown, J. H. & Enquist, B. J. A general model for the origin of allometric scaling laws in biology. Science 276, 122–126 (1997)
Lindstedt, S. L. & Calder, W. A. Body size, physiological time, and the longevity of homeothermic mammals. Q. Rev. Biol. 56, 1–16 (1981)
Schmidt-Nielsen, K. Scaling: Why Is Animal Size So Important? (Cambridge Univ. Press, 1983)
Brown, J. H., Gillooly, J. F., Allen, A. P., Savage, V. M. & West, G. B. Toward a metabolic theory of ecology. Ecology 85, 1771–1789 (2004)
McMahon, T. A. & Bonner, J. T. On Size and Life (Scientific American Library, 1983)
Peters, R. H. The Ecological Implications of Body Size (Cambridge Univ. Press, 1983)
Calder, W. A. Size, Function, and Life History (Dover, 1996)
Heusner, A. A. Energy metabolism and body size. II. Dimensional analysis and energetic non-similarity. Respir. Physiol. 48, 13–25 (1982)
Robinson, W. R., Peters, R. H. & Zimmermann, J. The effects of body size and temperature on metabolic rate of organisms. Can. J. Zool. 61, 281–288 (1983)
Gillooly, J. F. & Allen, A. P. Changes in body temperature influence the scaling of VO2,max and aerobic scope in mammals. Biol. Lett. 3, 99–102 (2007)
Alberts, B. et al. Molecular Biology of the Cell 4th edn (Garland Science, 2002)
White, C. R., Blackburn, T. M. & Seymour, R. S. Phylogenetically informed analysis of the allometry of mammalian basal metabolic rate supports neither geometric nor quarter-power scaling. Evolution 63, 2658–2667 (2009)
Grafen, A. The phylogenetic regression. Phil. Trans. R. Soc. Lond. B 326, 119–157 (1989)
Savage, V. M., Deeds, E. J. & Fontana, W. Sizing up allometric scaling theory. PLoS Comp. Biol. 4, e1000171 (2008)
Acknowledgements
We thank the staff of the library of the Museum of Comparative Zoology at Harvard University for their assistance and access to their resources. A. Duncan assisted in indexing and copying original printed materials.
Author Contributions T.K. carried out the statistical analysis, performed the analytical calculations, and annotated the data of ref. 8; T.K., V.S. and E.J.D. extended and analysed vascular scaling models; T.K., V.S., E.J.D. and W.F. interpreted the results and wrote the paper.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary Information
This Supplementary Information file comprises: 1 Regression Coefficients for Various Data Sets; 2 Regression Diagnostics; 3 Linear Regression and the Slope of a Quadratic Function; 4 Extending the West-Brown-Enquist model. It also includes Supplementary Figures 1-5 with legends and Supplementary References. (PDF 4758 kb)
Supplementary Data
This file contains curvature data. (XLS 295 kb)
Rights and permissions
About this article
Cite this article
Kolokotrones, T., Van Savage, Deeds, E. et al. Curvature in metabolic scaling. Nature 464, 753–756 (2010). https://doi.org/10.1038/nature08920
Received:
Accepted:
Issue Date:
DOI: https://doi.org/10.1038/nature08920
This article is cited by
-
Minke whale feeding rate limitations suggest constraints on the minimum body size for engulfment filtration feeding
Nature Ecology & Evolution (2023)
-
Solving the grand challenge of phenotypic integration: allometry across scales
Genetica (2022)
-
Wide size dispersion and use of body composition and maturation improves the reliability of allometric exponent estimates
Journal of Pharmacokinetics and Pharmacodynamics (2022)
-
Intra- and inter-specific scaling laws of plants and animals
Acta Mechanica Sinica (2021)
-
An non-loglinear enzyme-driven law of photosynthetic scaling in two representative crop seedlings under different water conditions
Scientific Reports (2020)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.