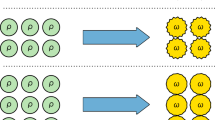
Abstract
Traditionally, entanglement was considered to be a quirk of microscopic objects that defied a common-sense explanation. Now, however, entanglement is recognized to be ubiquitous and robust. With the realization that entanglement can occur in macroscopic systems — and with the development of experiments aimed at exploiting this fact — new tools are required to define and quantify entanglement beyond the original microscopic framework.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout



Similar content being viewed by others
References
Amico, L., Fazio, R., Osterloh, A. & Vedral, V. Many-body entanglement. Rev. Mod. Phys. 80, 517–576 (2008).
Osterloh, A. et al. Scaling of entanglement close to a quantum phase transition. Nature 416, 608–610 (2002).
Osborne, T. J. & Nielsen, M. A. Entanglement in a simple quantum phase transition. Phys. Rev. A 66, 032110 (2002).
Arnesen, M. C., Bose, S. & Vedral, V. Natural thermal and magnetic entanglement in 1D Heisenberg model. Phys. Rev. Lett. 87, 017901 (2001).
Vedral, V. High temperature macroscopic entanglement. New J. Phys. 6, 102 (2004).
Brukner, C., Vedral, V. & Zeilinger, A. Crucial role of entanglement in bulk properties of solids. Phys. Rev. A 73, 012110 (2006).
Vedral, V. A better than perfect match. Nature 439, 397 (2006).
Zeilinger, A., Weihs, G., Jennewein, T. & Aspelmeyer, M. Happy centenary, photon. Nature 433, 230–238 (2005).
Einstein, A., Podolsky, B. & Rosen, N. Can quantum-mechanical description of physical reality be considered complete? Phys. Rev. 47, 777–780 (1935).
Werner, R. F. Quantum states with Einstein–Podolsky–Rosen correlations admitting a hidden-variable model. Phys. Rev. A 40, 4277–4281 (1989).
Brukner, C. & Vedral, V. Macroscopic thermodynamical witnesses of quantum entanglement. Preprint at <http://arxiv.org/abs/quant-ph/0406040> (2004).
Toth, G. & Guhne, O. Detecting genuine multipartite entanglement with two local measurements. Phys. Rev. Lett. 94, 060501 (2004).
Narnhoffer, H. Separability for lattice systems at high temperature. Phys. Rev. A 71, 052326 (2005).
Schrödinger, E. Die gegenwärtige Situation in der Quantenmechanik. Naturwissenschaften 23, 807–812; 823–828; 844–849 (1935).
Horodecki, M., Horodecki, P. & Horodecki, R. Separability of mixed states: necessary and sufficient conditions. Phys. Lett. A 223, 1–8 (1996).
Anders J. & Vedral, V. Macroscopic entanglement and phase transitions. Open Sys. Inform. Dyn. 14, 1–16 (2007).
Horodecki, M. Entanglement measures. Quant. Inform. Comput. 1, 3–26 (2001).
Vedral V. et al. Quantifying entanglement. Phys. Rev. Lett. 78, 2275–2279 (1997).
Greenberger, D., Horne, M. A. & Zeilinger, A. in Bell's Theorem, Quantum Theory, and Conceptions of the Universe (ed. Kafatos, M.) 73–76 (Kluwer Academic, Dordrecht, 1989).
Dur, W., Vidal, G. & Cirac, J. I. Three qubits can be entangled in two inequivalent ways. Phys. Rev. A 62, 062314 (2000).
Leggett, A. J. Macroscopic quantum systems and the quantum theory of measurement. Prog. Theor. Phys. Suppl. 69, 80–100 (1980).
Anders, J. & Winter, A. Entanglement and separability of quantum harmonic oscillator systems at finite temperature. Quant. Inform. Comput. 8, 0245–0262 (2008).
Vedral, V. Entanglement in the second quantisation formalism. Cent. Eur. J. Phys. 2, 289–306 (2003).
Anderson, P. W. Resonating valence bonds: a new kind of insulator? Mater. Res. Bull. 81, 53–60 (1973).
Raussendorf, R. & Briegel, H. J. A one-way quantum computer. Phys. Rev. Lett. 86, 5188–5191 (2001).
Chandran, A., Kaszlikowski, D., Sen De, A., Sen, U. & Vedral, V. Regional versus global entanglement in resonating-valence-bond states. Phys. Rev. Lett. 99, 170502 (2007).
Page, D. N. & Wootters, W. K. Evolution without evolution: dynamics described by stationary observables. Phys. Rev. D 27, 2885–2892 (1983).
Haffner, H. et al. Scalable multiparticle entanglement of trapped ions. Nature 438, 643–646 (2005).
Lu, C.-Y. et al. Experimental entanglement of six photons in graph states. Nature Phys. 3, 91–95 (2007).
Baugh, J. et al. Quantum information processing using nuclear and electron magnetic resonance: review and prospects. Preprint at <http://arxiv.org/abs/0710.1447> (2007).
de Chiara, G. et al. A scheme for entanglement extraction from a solid. New J. Phys. 8, 95 (2006).
Toth, G. Entanglement detection in optical lattices of bosonic atoms with collective measurements. Phys. Rev. A 69, 052327 (2004).
Heaney, L., Anders, J., Kaszlikowski, D. & Vedral, V. Spatial entanglement from off-diagonal long-range order in a Bose–Einstein condensate. Phys. Rev. A 76, 053605 (2007).
Recher, P. & Loss, D. Superconductor coupled to two Luttinger liquids as an entangler for spin electrons. Phys. Rev. B 65, 165327 (2002).
Verstraete, F. & Cirac, J. I. Quantum nonlocality in the presence of superselection rules and data hiding protocols. Phys. Rev. Lett. 91, 010404 (2003).
Acknowledgements
I am grateful for funding from the Engineering and Physical Sciences Research Council, the Wolfson Foundation, the Royal Society and the European Union. My work is also supported by the National Research Foundation (Singapore) and the Ministry of Education (Singapore). I thank J. A. Dunningham, A. J. Leggett, D. Markham, E. Rieper, W. Son and M. Williamson for discussions of this and related subjects. W. Son's help with illustrations is also gratefully acknowledged.
Author information
Authors and Affiliations
Ethics declarations
Competing interests
The author declares no competing financial interests.
Additional information
Correspondence should be addressed to the author (vlatko.vedral@quantuminfo.org).
Rights and permissions
About this article
Cite this article
Vedral, V. Quantifying entanglement in macroscopic systems. Nature 453, 1004–1007 (2008). https://doi.org/10.1038/nature07124
Published:
Issue Date:
DOI: https://doi.org/10.1038/nature07124
This article is cited by
-
Witnessing light-driven entanglement using time-resolved resonant inelastic X-ray scattering
Nature Communications (2023)
-
Evolution of the entanglement, photon statistics and quantum Fisher information of a single qubit parity deformed JCM
Optical and Quantum Electronics (2023)
-
The QBIT Theory: Consciousness from Entangled Qubits
Integrative Psychological and Behavioral Science (2022)
-
The relationship between single-particle commuting observables lz, sz entangled states and the spin–orbit coupling
Quantum Information Processing (2020)
-
Indirect Entanglement Transfer and Steering Between Two Atomic Ensembles in a Multi-Mode Hybrid Optomechanical System
International Journal of Theoretical Physics (2019)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.