Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

  • Letter
  • Published:

Non-saturating magnetoresistance in heavily disordered semiconductors

Nature volume 426, pages 162–165 (2003)Cite this article

Abstract

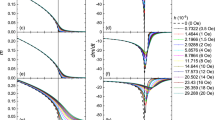
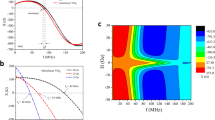
The resistance of a homogeneous semiconductor increases quadratically with magnetic field at low fields and, except in very special cases, saturates at fields much larger than the inverse of the carrier mobility, a number typically of the order of 1 T (refs 1, 2). A surprising exception to this behaviour has recently been observed in doped silver chalcogenides3,4,5, which exhibit an anomalously large, quasi-linear magnetoresistive response that extends down to low fields and survives, even at extreme fields of 55 T and beyond. Here we present a simple model of a macroscopically disordered and strongly inhomogeneous semiconductor that exhibits a similar non-saturating magnetoresistance. In addition to providing a possible explanation for the behaviour of doped silver chalcogenides, our model suggests potential routes for the construction of magnetic field sensors with a large, controllable and linear response.

This is a preview of subscription content, access via your institution

Access options

Buy this article

  • Purchase on Springer Link
  • Instant access to full article PDF
Buy now

Prices may be subject to local taxes which are calculated during checkout

Figure 1: Four-terminal network resistor unit, and a schematic diagram of an N × M resistor network.
Figure 2: Normalized magnetoresistance ΔR(H)/R(0) as a function of dimensionless magnetic field β for different sized N × N uniform networks.
Figure 3: Visualization of currents and voltages at large magnetic field in a 10 × 10 random network of disks with radii 1 (arbitrary units), where the potential difference U = -1 V.
Figure 4: Average normalized magnetoresistance ΔR(H)/R(0) as a function of dimensionless magnetic field H/H0 of 20 × 20 random resistor networks for different mobility distributions, where H0 = 1 kOe is a typical field scale.

Similar content being viewed by others

References

  1. Kittel, C. Quantum Theory of Solids (Wiley, New York, 1963)

    MATH  Google Scholar 

  2. Smith, R. A. Semiconductors 2nd edn (Cambridge Univ. Press, Cambridge, 1978)

    MATH  Google Scholar 

  3. Xu, R. et al. Large magnetoresistance in non-magnetic silver chalcogenides. Nature 390, 57–60 (1997)

    Article  ADS  CAS  Google Scholar 

  4. Lee, M., Rosenbaum, T. F., Saboungi, M.-L. & Schnyders, H. S. Band-gap tuning and linear magnetoresistance in the silver chalcogenides. Phys. Rev. Lett. 88, 066602 (2002)

    Article  ADS  CAS  Google Scholar 

  5. Husmann, A. et al. Megagauss sensors. Nature 417, 421–424 (2002)

    Article  ADS  CAS  Google Scholar 

  6. Dalven, R. & Gill, R. Energy gap in β-Ag2Se. Phys. Rev. 159, 645–649 (1967)

    Article  ADS  CAS  Google Scholar 

  7. Tokura, Y. (ed.) Colossal Magnetoresistive Oxides (Gordon and Breach Science, New York, 2000)

  8. Kapitza, P. L. The change of electrical conductivity in strong magnetic fields. Proc. R. Soc. Lond. A 123, 292–372 (1929)

    Article  ADS  Google Scholar 

  9. Abrikosov, A. A. Quantum magnetoresistance. Phys. Rev. B 58, 2788–2794 (1998)

    Article  ADS  CAS  Google Scholar 

  10. Büttiker, M. Magnetoresistance of very pure simple metals. Phys. Rev. B 42, 3197–3200 (1990)

    Article  ADS  Google Scholar 

  11. Bruls, G. J. C. L., Bass, J., van Gelder, A. P., van Kempen, H. & Wyder, P. Linear magnetoresistance caused by sample thickness variations. Phys. Rev. Lett. 46, 553–555 (1981)

    Article  ADS  Google Scholar 

  12. Beer, A. C. Solid State Physics Supplement 4: Galvanomagnetic Effects in Semiconductors (Academic, New York, 1963)

    Google Scholar 

  13. Reynolds, J. A. & Hough, J. M. Formulae for dielectric constant of mixtures. Proc. Phys. Soc. Lond. B 70, 769–775 (1957)

    Article  ADS  Google Scholar 

  14. Balagurov, B. Ya. Conductivity of inhomogeneous media in strong magnetic fields. Sov. Phys. Solid State 28, 1694–1698 (1986)

    Google Scholar 

  15. Stroud, D. & Pan, F. P. Effect of isolated inhomogeneities on the galvanomagnetic properties of solids. Phys. Rev. B 13, 1434–1438 (1976)

    Article  ADS  CAS  Google Scholar 

  16. Bergman, D. J. & Stroud, D. G. High-field magnetotransport in composite conductors: Effective medium approximation. Phys. Rev. B 62, 6603–6613 (2000)

    Article  ADS  CAS  Google Scholar 

  17. Herring, C. Effect of random inhomogeneities on electrical and galvanomagnetic measurements. J. Appl. Phys. 31, 1939–1953 (1960)

    Article  ADS  Google Scholar 

  18. Dreizin, Yu. A. & Dykhne, A. M. Anomalous conductivity of inhomogeneous media in a strong magnetic field. Sov. Phys. JETP 36, 127–136 (1973)

    ADS  Google Scholar 

  19. Solin, S. A., Thio, T., Hines, D. R. & Heremans, J. J. Enhanced room-temperature geometric magnetoresistance in inhomogeneous narrow-gap semiconductors. Science 289, 1530–1532 (2000)

    Article  ADS  CAS  Google Scholar 

  20. Solin, S. A., Thio, T. & Hines, D. R. Controlled GMR enhancement from conducting inhomogeneities in non-magnetic semiconductors. Physica B 279, 37–40 (2000)

    Article  ADS  CAS  Google Scholar 

  21. Ogorelec, Z., Hamzic, A. & Basletic, M. On the optimisation of the large magnetoresistance of Ag2Se. Europhys. Lett. 46, 56–61 (1999)

    Article  ADS  Google Scholar 

Download references

Acknowledgements

P.B.L. thanks the NHMFL for hospitality during the final drafting of this Letter. The NHMFL is supported by the National Science Foundation, the state of Florida and the US Department of Energy. This work was supported by the Association of Commonwealth Universities and the Cambridge Commonwealth Trust.

Author information

Authors and Affiliations

  1. Cavendish Laboratory, University of Cambridge, Cambridge, CB3 0HE, UK

    M. M. Parish & P. B. Littlewood

  2. National High Magnetic Field Laboratory, Pulsed Field Facility, LANL, Los Alamos, New Mexico, 87545, USA

    P. B. Littlewood

Authors
  1. M. M. Parish
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. P. B. Littlewood
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to M. M. Parish.

Ethics declarations

Competing interests

The authors declare that they have no competing financial interests.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Parish, M., Littlewood, P. Non-saturating magnetoresistance in heavily disordered semiconductors. Nature 426, 162–165 (2003). https://doi.org/10.1038/nature02073

Download citation

  • Received:

  • Accepted:

  • Issue Date:

  • DOI: https://doi.org/10.1038/nature02073

Comments

By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing